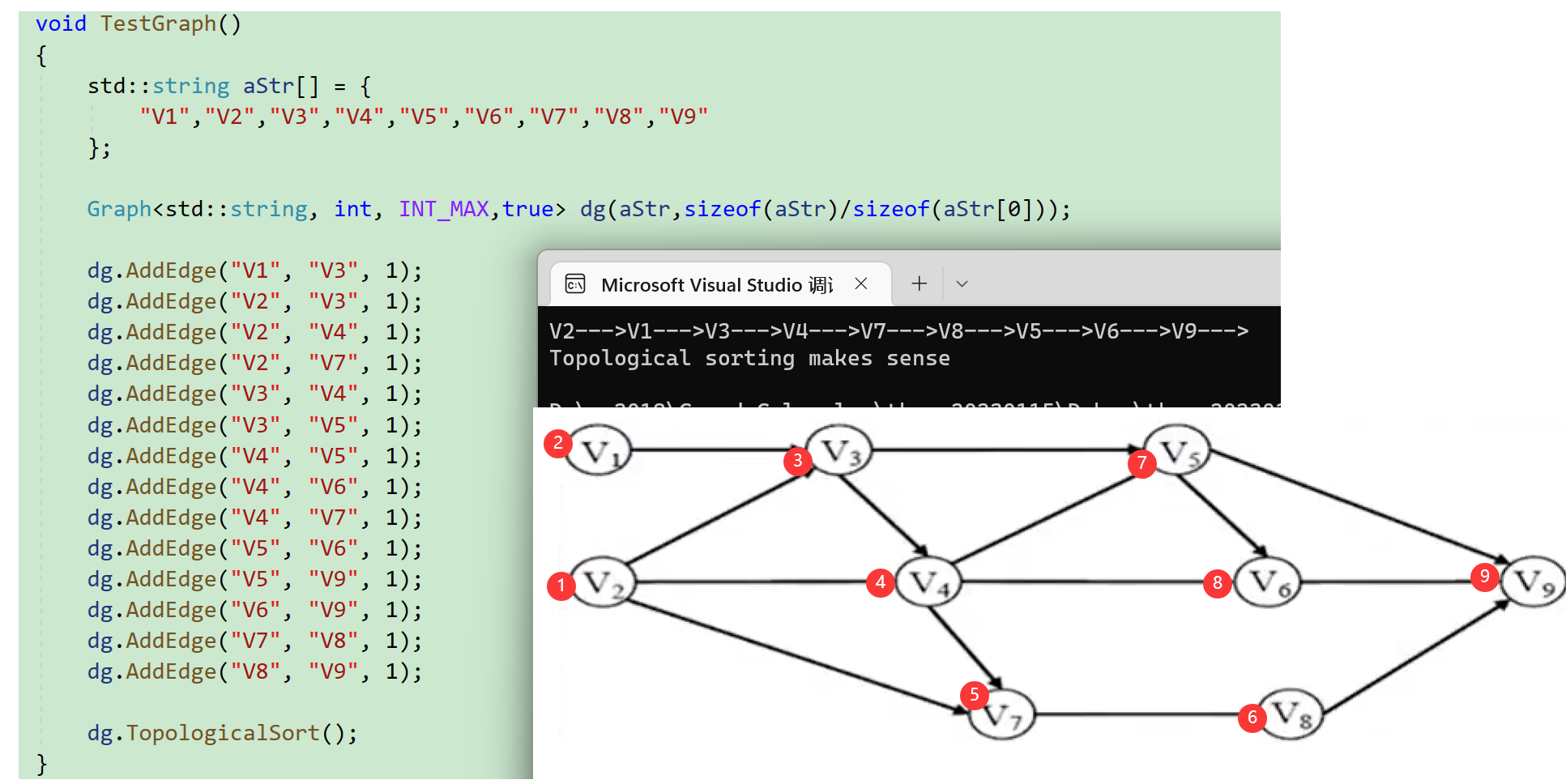
A - Sequence of Strings

Problem Statement

You are given N strings S1​,S2​,…,SN​ in this order.

Print SN​,SN−1​,…,S1​ in this order.

Constraints

• 1≤N≤10
• N is an integer.
• Si​ is a string of length between 1 and 10, inclusive, consisting of lowercase English letters, uppercase English letters, and digits.

---

Input

The input is given from Standard Input in the following format:

N
S1​
S2​
......
SN​

Output

Print N lines. The i-th (1≤i≤N) line should contain SN+1−i​.

---

Sample Input 1

3

Takahashi

Aoki

Snuke

Sample Output 1

Snuke

Aoki

Takahashi

We have N=3 .S1​= Takahashi,S2​= Aoki, and S3​= Snuke.

Thus, you should print Snuke, Aoki, and Takahashi in this order.

---

Sample Input 2

4

2023

Year

New
Happy

Sample Output 2

Happy

New

Year

2023

The given strings may contain digits.

AC Code:

逆序输出即可!

#include <iostream>
#include <cstring>
#include <stack>
using namespace std;
stack<string> sta;
string s;
int n;
int main()
{
    ios::sync_with_stdio(false);
    cin.tie(nullptr), cout.tie(nullptr);
    cin >> n;
    while (n--)
    {
        cin >> s;
        sta.push(s);
    }
    while (!sta.empty())
    {
        cout << sta.top() << '\n';
        sta.pop();
    }
    return 0;
}

#include<bits/stdc++.h>
using namespace std;
int main() {
    int n;
    cin >> n;
    vector <string> s(n);
    for (int i = 0; i < n; i++) cin >> s[i];
    for (int i = n - 1; i >= 0; i--) cout << s[i] << '\n';
    return 0;
}

B - Multi Test Cases

Problem Statement

In this problem, an input file contains multiple test cases.
You are first given an integer T. Solve the following problem for TT test cases.

• We have N positive integers A1​,A2​,...,AN​. How many of them are odd?

Constraints

• 1≤T≤100
• 1≤N≤100
• 1≤Ai​≤10^9
• All values in the input are integers.

---

Input

The input is given from Standard Input in the following format, where test-i​ represents the i-th test case:

T
test1​
test2​
......
testT​

Each test case is in the following format:

N
A1......AN​

Output

Print T lines. The i-th line should contain the answer for the i-th test case.

---

Sample Input 1

4

3

1 2 3

2

20 23

10

6 10 4 1 5 9 8 6 5 1

1

1000000000

Sample Output 1

2

1

5

0

AC Code:

#include <iostream>
#include <cstring>
#include <stack>
using namespace std;
const int N = 1e2 + 5;
int a[N], sum;
int t, n;
int main()
{
    ios::sync_with_stdio(false);
    cin.tie(nullptr), cout.tie(nullptr);
    cin >> t;
    while (t--)
    {
        sum = 0;
        cin >> n;
        for (int i = 0; i < n; i++)
            cin >> a[i];
        for (int i = 0; i < n; i++)
            if (a[i] & 1)
                sum++;
        cout << sum << '\n';
    }
    return 0;
}

C - Count Connected Components

Problem Statement

You are given a simple undirected graph with N vertices numbered 1 to N and M edges numbered 1 to M. Edge i connects vertex ui​ and vertex vi​.
Find the number of connected components in this graph.

Notes

A simple undirected graph is a graph that is simple and has undirected edges.
A graph is simple if and only if it has no self-loop or multi-edge.

A subgraph of a graph is a graph formed from some of the vertices and edges of that graph.
A graph is connected if and only if one can travel between every pair of vertices via edges.
A connected component is a connected subgraph that is not part of any larger connected subgraph.

Constraints

• 1≤N≤100
• The given graph is simple.
• All values in the input are integers.

---

Input

The input is given from Standard Input in the following format:

N M
u1 v1
u2 v2
......
uM vM

Output

Print the answer.

---

Sample Input 1

5 3
1 2
1 3
4 5

Sample Output 1

2

The given graph contains the following two connected components:

• a subgraph formed from vertices 1, 2, 3, and edges 1, 2;
• a subgraph formed from vertices 4, 5, and edge 3.

---

Sample Input 2

5 0

Sample Output 2

5

---

Sample Input 3

4 6
1 2
1 3
1 4
2 3
2 4
3 4

Sample Output 3

1

连通块的数量

AC Code:

#include <bits/stdc++.h>
#define int long long
using namespace std;
const int N = 5e5 + 10;

int n, m, p[N];

int find(int x)
{
    if (p[x] != x)
        p[x] = find(p[x]);
    return p[x];
}
signed main()
{
    cin >> n >> m;

    for (int i = 1; i <= n; i++)
        p[i] = i;

    while (m--)
    {
        int u, v;
        cin >> u >> v;
        u = find(u), v = find(v);
        if (u != v)
            p[u] = v;
    }

    int ans = 0;
    for (int i = 1; i <= n; i++)
        ans += find(i) == i;

    cout << ans << '\n';
}

D - Happy New Year 2023

Problem Statement

There is an integer N. It is known that N can be represented as N=p^2*q using two different prime numbers p and q. Find p and q.You have T test cases to solve.

---

Input

The input is given from Standard Input in the following format, where test-i​ represents the i-th test case:​

T
test1
test2
test3
......
testT

1<=T<=10.

1<=N<=9*10^18.

Each test case is in the following format:

N

Output

Print T lines.

---

Sample Input 1

3
2023
63
1059872604593911

Sample Output 1

17 7
3 7
104149 97711

AC Code:

#include <bits/stdc++.h>
using namespace std;
#define int long long
const int N = 3e6 + 10;
int n, cnt, primes[N];
bool st[N];
void get_primes()
{
    for (int i = 2; i < 3e6; i++)
    {
        if (!st[i])
            primes[cnt++] = i;
        for (int j = 0; primes[j] * i < 3e6; j++)
        {
            st[primes[j] * i] = true;
            if (i % primes[j] == 0)
                break;
        }
    }
}
void solve()
{
    cin >> n;
    for (int i = 0; i < cnt; i++)
    {
        if (n % (primes[i] * primes[i]) == 0)
        {
            cout << primes[i] << " " << n / (primes[i] * primes[i]) << '\n';
            return;
        }
        if (n % primes[i] == 0)
        {
            int num = n / primes[i];
            int x = (int)sqrt(num);
            cout << x << " " << primes[i] << '\n';
            return;
        }
    }
}
signed main()
{
    get_primes();
    st[1] = 1;
    int T;
    cin >> T;
    while (T--)
        solve();
}