题目链接

1766C - Hamiltonian Wall Rating：1300

题目描述

Sir Monocarp Hamilton is planning to paint his wall. The wall can be represented as a grid, consisting of 2 rows and m columns. Initially, the wall is completely white.

Monocarp wants to paint a black picture on the wall. In particular, he wants cell (i,j) (the j-th cell in the i-th row) to be colored black, if ci,j= ‘B’, and to be left white, if ci,j= ‘W’. Additionally, he wants each column to have at least one black cell, so, for each j, the following constraint is satisfied: c1,j, c2,j or both of them will be equal to ‘B’.

In order for the picture to turn out smooth, Monocarp wants to place down a paint brush in some $cell(x1,y1)$ and move it along the path $(x1,y1),(x2,y2),\dots ,(xk,yk)$ so that:

• for each i, (xi,yi) and (xi+1,yi+1) share a common side;
• all black cells appear in the path exactly once;
• white cells don’t appear in the path.

Determine if Monocarp can paint the wall.

Input

The first line contains a single integer $t$ ($1\le t\le 10^4$) — the number of testcases.

The first line of each testcase contains a single integer $m(1\le m\le 2\cdot 10^5)$ — the number of columns in the wall.

The i-th of the next two lines contains a string ci, consisting of m characters, where each character is either ‘B’ or ‘W’. ci,j is ‘B’, if the $cell(i,j)$ should be colored black, and ‘W’, if the $cell(i,j)$ should be left white.

Additionally, for each j, the following constraint is satisfied: c1,j, c2,j or both of them are equal to ‘B’.

The sum of m over all testcases doesn’t exceed $2\cdot 10^5$.

Output

For each testcase, print "YES"if Monocarp can paint a wall. Otherwise, print "NO".

inputCopy

6
3
WBB
BBW
1
B
B
5
BWBWB
BBBBB
2
BW
WB
5
BBBBW
BWBBB
6
BWBBWB
BBBBBB

outputCopy

YES
YES
NO
NO
NO
YES

大致题意：
每次给你一个 2行 m列 的字符数组s。$s[i][j]={=}^{\prime }{B}^{\prime }$ 代表这一块是黑色，$s[i][j]={=}^{\prime }{W}^{\prime }$ 代表这一块是白色。

问是否存在一条路径包括了所有的黑色块，每个黑色块只在这条路径上出现一次。存在就打印 YES，否则打印NO。

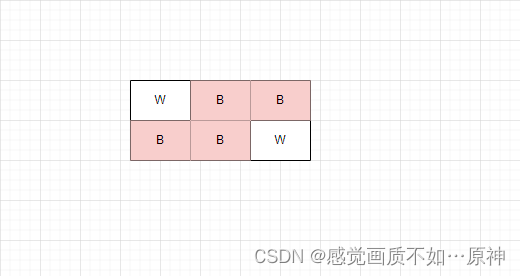
这个例子存在这样的一条路径，所以打印 YES:

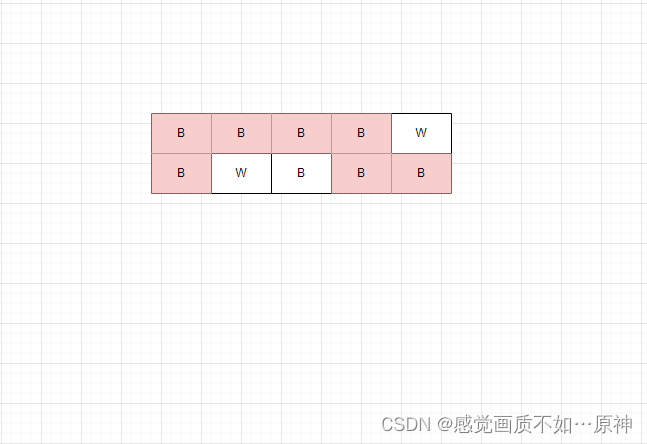
以下这个例子就不存在，所以打印NO：

我们定义 $f(i,j)(1<=i<=2,1<=j<=n)$ 为到达点 $(i,j)$的路径中包含的黑色块的数量。

最后我们只需要判断 $max(f(1,n),f(2,n))==总的黑色块数量$，如果等于说明确实存在这样一条路径；否则不存在。

分情况讨论：

当 $s[1][j]={=}^{\prime }{B}^{\prime }$ 时，有以下三种情况：

• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }andf[2][j-1]={=}^{\prime }{B}^{\prime }$时，$f[1][j]=f[1][j-1]+1$
• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }$时，$f[1][j]=f[1][j-1]+1$
• 当 $f[2][j-1]={=}^{\prime }{B}^{\prime }$时，这种情况不符合要求，忽略。

当 $s[2][j]={=}^{\prime }{B}^{\prime }$ 时，有以下三种情况：

• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }andf[2][j-1]={=}^{\prime }{B}^{\prime }$时，$f[2][j]=f[2][j-1]+1$
• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }$时，这种情况不符合要求，忽略。
• 当 $f[2][j-1]={=}^{\prime }{B}^{\prime }$时，$f[2][j]=f[2][j-1]+1$

当 $f[1][j-1]={=}^{\prime }{B}^{\prime }andf[2][j-1]={=}^{\prime }{B}^{\prime }$时，有以下四种情况：

• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }andf[2][j-1]={=}^{\prime }{B}^{\prime }$时，
• • $f[1][j]=max(f[1][j-1]+1,f[2][j-1]+2)$
• • $f[2][j]=max(f[1][j-1]+2,f[2][j-1]+1)$
• 当 $f[1][j-1]={=}^{\prime }{B}^{\prime }$时，
• • $f[1][j]=f[1][j-1]+1$
• • $f[2][j]=f[1][j-1]+2$
• 当 $f[2][j-1]={=}^{\prime }{B}^{\prime }$时，
• • $f[1][j]=f[2][j-1]+2$
• • $f[2][j]=f[2][j-1]+1$

时间复杂度： $O(n)$

代码：

#include <iostream>
#include<algorithm>
#include<vector>
#include<cstring>


using namespace std;

const int N = 2e5+10;
char s[3][N];
int f[3][N];

void solve(){
    int n;
    scanf("%d",&n);
    for(int i = 1;i <= 2;i++){
        scanf("%s",s[i] + 1);
    }
    
    memset(f,0,sizeof f);
    
    int cnt = 0;
    for(int i = 1;i <= 2;i++){
        for(int j = 1;j <= n;j++){
            if(s[i][j] == 'B') cnt++;
        }
    }

    if(s[1][1] == 'B'&& s[2][1] == 'B'){
        f[1][1] = 1;
        f[2][1] = 1;
    }
    
    for(int j = 2;j <= n;j++){
        if(s[1][j] == 'B' && s[2][j] == 'B'){
            if(s[1][j-1] == 'B' && s[2][j-1] == 'B'){
                f[1][j] = max(f[1][j-1] + 1,f[2][j-1] + 2);
                f[2][j] = max(f[1][j-1] + 2,f[2][j-1] + 1);
            }
            else if(s[1][j-1] == 'B'){
                f[1][j] = f[1][j-1] + 1;
                f[2][j] = f[1][j-1] + 2;
            }
            else if(s[2][j-1] == 'B'){
                f[1][j] = f[2][j-1] + 2;
                f[2][j] = f[2][j-1] + 1;
            }
        }
        else if(s[1][j] == 'B'){
            if(s[1][j-1] == 'B' && s[2][j-1] == 'B') f[1][j] = f[1][j-1]+1;
            else if(s[1][j-1] == 'B') f[1][j] = f[1][j-1] + 1;
        }
        else if(s[2][j] == 'B'){
            if(s[1][j-1] == 'B' && s[2][j-1] == 'B') f[2][j] = f[2][j-1]+1;
            else if(s[2][j-1] == 'B') f[2][j] = f[2][j-1] + 1;
        }
    }

    
    int ans = max(f[1][n],f[2][n]);
    //ans 相当于是连接 这个cnt个黑色块的边数 所以要+1 才是黑色块的数量
    if(cnt == ans+1) puts("YES");
    
    else puts("NO");
}

int main() {
  int t;
  cin>>t;
  while(t--){
      solve();
  }
  return 0;
}