左神算法中级提升（3）

news2024/11/29 22:31:45

目录

【案例1】

【题目描述】【2018阿里巴巴面试题】

 【思路解析】

【代码实现】

【案例2】

【题目描述】

 【思路解析1】

 【思路解析2】

【代码实现】

 【案例3】

【题目描述】

 【思路解析】

 【代码实现】

【案例4】

【题目描述】

 【思路解析】

【代码实现】

【案例5】

【题目描述】

 【思路解析】

【代码实现】

【案例6】

【题目描述】

【思路解析】

【代码实现】

【案例7】

【题目描述】

【思路解析】

【代码实现】

【案例1】

【题目描述】【2018阿里巴巴面试题】

 【思路解析】

对每个位置，它都有一个至少需要移动多少轮，能使这个地方局部平分，这所有位置中的最大轮数就是我们需要返回的。

（1） 如果数组求和不能被数组.length整除，直接返回-1

说明  a和b可正可负，正代表需要扔出衣服，负代表需要接收衣服

i位置的左侧部分a，右侧部分b

（2）【1】如果a和b均为负，因为一次只能移动一件，所以需要|a+b|轮。

【2】如果a和b均为正，需要max{|a|，|b|}轮

【3】如果a和b不同号，需要max{|a|，|b|}轮

【代码实现】

/**
 * @ProjectName: study3
 * @FileName: Ex1
 * @author:HWJ
 * @Data: 2023/7/11 7:49
 */
public class Ex1 {
    public static void main(String[] args) {
        int[] arr = {100,0,0,0};
        System.out.println(getMin(arr));
    }

    public static int getMin(int[] arr){
        int sum = 0;
        for (int i = 0; i < arr.length; i++) {
            sum += arr[i];
        }
        if (sum % arr.length != 0){
            return -1;
        }
        int n = sum / arr.length;
        int rightSum = sum;
        int leftSum = 0;
        int leftSize = 0;
        int rightSize = arr.length - 1;
        int min = 0;
        for (int i = 0; i < arr.length; i++) {
            rightSum -= arr[i];
            int left = Math.abs(leftSum - (n * leftSize));
            int right = Math.abs(rightSum - (rightSize * n));
            if (rightSum - (rightSize * n) < 0 && leftSum - (n * leftSize) < 0){
                min = Math.max(left + right, min);
            }else {
                min = Math.max(Math.max(left, right), min);
            }
            leftSum += arr[i];
            leftSize ++;
            rightSize --;
        }
        return min;
    }

}

【案例2】

【题目描述】

 【思路解析1】

循环打印，再触碰边界时考虑如何转弯即可。此方法容易想到（就是模仿打印顺序的实现），但不容易实现。

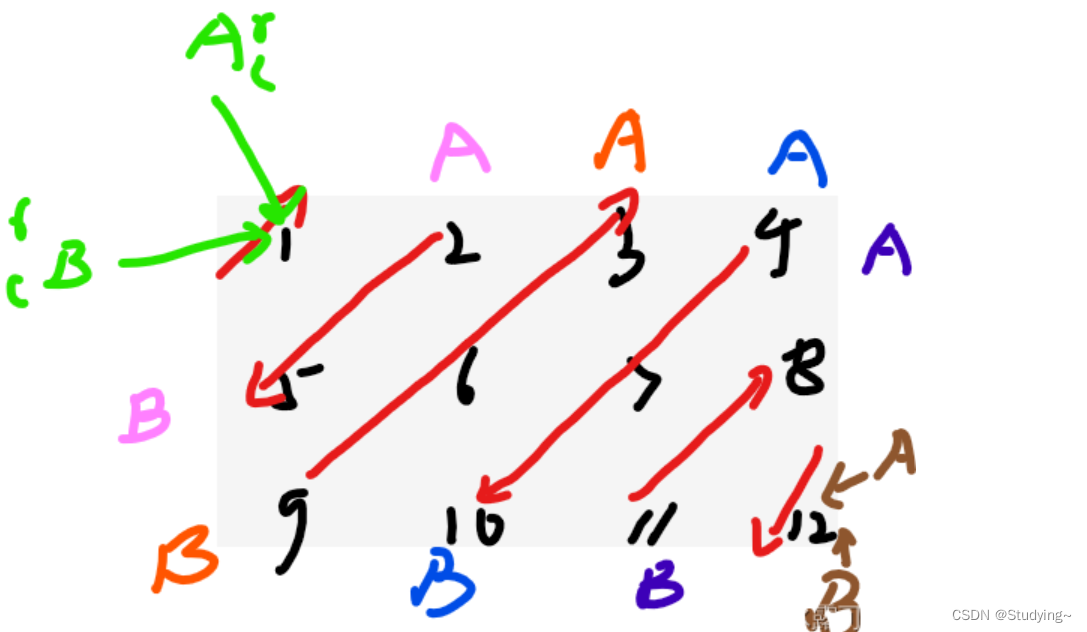
 【思路解析2】

使用宏观调度的思路，让A和B初始为矩阵的左上角，然后如果A和B指向同一个就直接打印，否则就打印斜线，每次B往下移动，如果不能再往下了，就往右移动，每次A往右移动，如果不能再往右了就往下移动。

【代码实现】

/**
 * @ProjectName: study3
 * @FileName: Ex2
 * @author:HWJ
 * @Data: 2023/7/11 11:44
 */
public class Ex2 {
    public static void main(String[] args) {
        int[][] arr = {{0, 1, 2, 3}, {4, 5, 6, 7}, {8, 9, 10, 11}};
        print2(arr);
    }

    public static void print(int[][] arr) {
        int N = arr.length;
        int M = arr[0].length;
        int i = 0;
        int j = 0;
        int k = 0; // k == 0 打印顺序向右上遍历， k == 1 打印顺序向左下遍历
        while (true) {

            System.out.print(arr[i][j] + " ");
            if (i == N - 1 && j == M - 1) {
                break;
            }
            if (k == 0) {
                i--;
                j++;
            } else {
                i++;
                j--;
            }


            if (i < 0 || i > N - 1 || j < 0 || j > M - 1) {
                if (k == 0) { // 当左上和右下顺序遍历越界时，调整方向
                    k = 1;
                    i++;
                    if (j == M && i != N) {
                        j--;
                        i++;
                    }
                } else {
                    k = 0;
                    j++;
                    if (i == N && j != M) {
                        i--;
                        j++;
                    }
                }
            }

        }

    }

    public static void print2(int[][] arr) {
        int ar = 0;
        int ac = 0;
        int br = 0;
        int bc = 0;
        boolean from = false;
        int endR = arr.length - 1;
        int endC = arr[0].length - 1;
        while (ar != endR + 1) {
            printM(arr, ar, ac, br, bc, from);
            ar = ac == endC ? ar + 1 : ar;
            ac = ac == endC ? ac : ac + 1;
            bc = br == endR ? bc + 1 : bc;
            br = br == endR ? br : br + 1;

            from = !from;
        }
    }

    public static void printM(int[][] arr, int ar, int ac, int br, int bc, boolean from) {
        if (from) {
            while (ar <= br) {
                System.out.print(arr[ar++][ac--] + " ");
            }
        } else {
            while (ar <= br) {
                System.out.print(arr[br--][bc++] + " ");
            }
        }
    }

}

 【案例3】

【题目描述】

 【思路解析】

螺旋打印的思路很好写，但是实现比较复杂，我们怎么使用宏观调度的方法是一个难题。

使用A和B确定两个边界，这两个边界确定一个长方形，然后我们只用螺旋打印长方形上的点，然后打印完后，边界A往右下移动，边界B往左上移动，然后再次打印这个长方形上的点。要求B不能越过A。

 【代码实现】

/**
 * @ProjectName: study3
 * @FileName: Ex3
 * @author:HWJ
 * @Data: 2023/7/11 12:19
 */
public class Ex3 {
    public static void main(String[] args) {
        int[][] arr = {{0, 1, 2, 3}, {4, 5, 6, 7}, {8, 9, 10, 11}};
        print(arr);
    }

    public static void print(int[][] map) {
        int a = 0;
        int b = 0;
        int c = map.length - 1;
        int d = map[0].length - 1;
        while (a <= c && b <= d) {
            process(map, a++, b++, c--, d--);
        }

    }

    public static void process(int[][] map, int a, int b, int c, int d) {
        if (a == c) { // A 和 B 同行
            for (int i = b; i <= d; i++) {
                System.out.print(map[a][i] + " ");
            }
        } else if (b == d) { // A 和 B 同列
            for (int i = a; i <= c; i++) {
                System.out.print(map[i][b] + " ");
            }
        } else { // 常规矩形的情况
            int curR = a; // 当前行
            int curC = b; // 当前列
            for (curC = b; curC < d; curC++) {
                System.out.print(map[a][curC] + " ");
            }
            for (curR = a; curR < c; curR++) {
                System.out.print(map[curR][d] + " ");
            }
            for (curC = d; curC > b; curC--) {
                System.out.print(map[c][curC] + " ");
            }
            for (curR = c; curR > a; curR--) {
                System.out.print(map[curR][b] + " ");
            }
        }
    }

}

【案例4】

【题目描述】

 【思路解析】

因为给定的矩阵是正方形，然后实现矩阵就可以分成多层正方形，矩阵中每个位置的数顺时针转动90度，它只会在它的那层正方形上转动。所以我们只用实现每层正方形转动完成即可。

【代码实现】

/**
 * @ProjectName: study3
 * @FileName: Ex4
 * @author:HWJ
 * @Data: 2023/7/11 13:07
 */
public class Ex4 {
    public static void main(String[] args) {
        int[][] matrix = {{0,1,2,3},{4,5,6,7},{8,9,10,11},{12,13,14,15}};
        rotate(matrix);
    }

    public static void rotate(int[][] matrix) {
        int a = 0;
        int b = 0;
        int c = matrix.length - 1;
        int d = matrix.length - 1;
        while (a <= c) {
            rotateEdge(matrix, a++, b++, c--, d--);
        }
        printMatrix(matrix); // 旋转完成后打印矩阵
    }

    public static void rotateEdge(int[][] matrix, int a, int b, int c, int d) {
        for (int i = 0; i < d - b; i++) { // 将正方形分组旋转
            int tmp = matrix[a][b + i];
            matrix[a][b + i] = matrix[c - i][b];
            matrix[c - i][b] = matrix[c][d - i];
            matrix[c][d - i] = matrix[a + i][d];
            matrix[a + i][d] = tmp;
        }
    }

    public static void printMatrix(int[][] matrix) {  // 打印矩阵
        int n = matrix.length;
        for (int i = 0; i < n; i++) {
            for (int j = 0; j < n; j++) {
                System.out.print(matrix[i][j] + " ");
            }
            System.out.println();
        }
    }
}

【案例5】

【题目描述】

 【思路解析】

（1）如果n是质数，则只调用操作2，就是它的最好结果

（2）如果n不是质数，假设是某一些质数相乘。n = x*y*z*r*q;

先只使用操作2来达到x，后面对于y、z、r、q都是先第一步使用操作1，剩余( i-2)步使用操作2，这里的i是泛指。

【代码实现】

/**
 * @ProjectName: study3
 * @FileName: Ex5
 * @author:HWJ
 * @Data: 2023/7/11 15:12
 */
public class Ex5 {
    public static void main(String[] args) {

    }

    public static int[] sumAndCount(int n) {
        int sum = 0;
        int count = 0;
        for (int i = 2; i <= n; i++) {
            while (n % i == 0) {
                count++;
                sum += i;
                n /= i;
            }
        }
        return new int[] {sum, count};
    }

    public static int gewWays(int n){
        if (n < 2){
            return  0;
        }
        if (isPrime(n)){
            return n-1;
        }
        return sumAndCount(n)[0] - sumAndCount(n)[1];
    }
    
    public static boolean isPrime(int n){
        if (n < 2){
            return false;
        }
        if (n == 2){
            return true;
        }
        for (int i = 2; i <= Math.sqrt(n); i++) {
            if (n % i == 0){
                return false;
            }
        }
        return true;
    }
}

【案例6】

【题目描述】

【思路解析】

 先对数组arr进行词频统计，然后规定小根堆的大小为k个，当大小未达到k个，就直接加入；否则，与堆顶比较，大的值进入小根堆。

【代码实现】

import java.util.Comparator;
import java.util.HashMap;
import java.util.PriorityQueue;

/**
 * @ProjectName: study3
 * @FileName: Ex6
 * @author:HWJ
 * @Data: 2023/7/11 15:37
 */
public class Ex6 {
    public static void main(String[] args) {
        String[] strings = {"abc", "abc","abc","as","as","bck","bck","bck"};
        topK(strings,2);
    }

    public static void topK(String[] str, int k) {
        if (k == 0) {
            return;
        }
        // 词频统计
        HashMap<String, Integer> strs = new HashMap<>();
        for (int i = 0; i < str.length; i++) {
            if (strs.containsKey(str[i])) {
                strs.put(str[i], strs.get(str[i]) + 1);
            } else {
                strs.put(str[i], 1);
            }
        }
        
        // 构建小根堆
        PriorityQueue<Str> strs1 = new PriorityQueue<>(new Comparator<Str>() {
            @Override
            public int compare(Str o1, Str o2) {
                return o1.count - o2.count;
            }
        });
        
        // 将词频统计后的数据，加入小根堆
        for (String s : strs.keySet()) {
            Str str1 = new Str(s, strs.get(s));
            if (strs1.size() == k) {
                if (str1.count > strs1.peek().count) {
                    strs1.poll();
                    strs1.add(str1);
                }
            } else {
                strs1.add(str1);
            }

        }
        
        // 将小根堆的数据打印出来
        while (!strs1.isEmpty()){
            System.out.println(strs1.poll().s);
        }

    }

    public static class Str {
        public String s;
        public int count = 0;

        public Str(String s, int count) {
            this.s = s;
            this.count = count;
        }
    }

}

【案例7】

【题目描述】

假设用户让我们实现某个结构，这个结构可以随时接收一个字符串，也可以随时打印当前出现次数最多的前k个字符串。

【思路解析】

这道题和上题类似，但是需要动态更新，所以需要一个结构来存放它在小根堆的位置来实现动态更新。

【代码实现】

import java.util.HashMap;

/**
 * @ProjectName: study3
 * @FileName: Ex7
 * @author:HWJ
 * @Data: 2023/7/11 16:16
 */
public class Ex7 {
    public static void main(String[] args) {

    }


    public static class Str {
        public String s;
        public int count = 0;

        public Str(String s, int count) {
            this.s = s;
            this.count = count;
        }
    }

    public static class topK{
        public HashMap<Str, Integer> strIndex;
        public HashMap<String, Str> strMap;
        int heapSize;
        Str[] strs;

        public topK(int K){
            this.heapSize = 0;
            this.strs = new Str[K]; // 大小为k的小根堆
            this.strMap = new HashMap<>(); // 词频统计的表结构
            this.strIndex = new HashMap<>();  // 此数据在堆中的位置结构
        }

        public void add(String s){
            int preIndex = -1;
            Str cur;
            if (!strMap.containsKey(s)){ // 如果这个字符串的信息没有出现过
                cur = new Str(s, 1); // 新建
                strMap.put(s, cur);
                strIndex.put(cur, -1); // 位置信息为-1
            }else { // 如果有，直接取出它的信息，然后词频++
                cur = strMap.get(s);
                cur.count++;
                preIndex = strIndex.get(cur);
            }
            if (preIndex == -1){// 如果位置信息为-1，说明他们不在小根堆中
                if (heapSize == strs.length){ // 如果小根堆已经满了
                    if (cur.count > strs[0].count){ // 大于堆顶就让它替换堆顶，并且修改他们的位置信息，然后调整为小根堆
                        strIndex.put(strs[0], -1);
                        strIndex.put(cur, 0);
                        strs[0] = cur;
                        heapify(strs, 0, heapSize);
                    }
                }else { // 没满直接加入，修改位置信息，然后修改为小根堆
                    strIndex.put(cur, heapSize);
                    strs[heapSize] = cur;
                    heapInsert(strs, heapSize++);
                }
            }else {
                // 如果它在小根堆中，更新小根堆
                heapify(strs, preIndex, heapSize);
            }
        }

        public void printTop(){
            System.out.println("TopK: ");
            for (int i = 0; i < heapSize; i++) {
                if(strs[i] == null){
                    break;
                }
                System.out.println("String: " + strs[i].s);
                System.out.println("Count: " + strs[i].count);
            }
        }

        public void heapify(Str[] strs, int index, int heapSize){ // 往下整理
            int left = index * 2 + 1;
            while (left < heapSize){
                int smallest = left + 1 < heapSize && strs[left].count > strs[left + 1].count ? left + 1: left;
                if (strs[index].count > strs[smallest].count){
                    swap(strs, index, smallest);
                    index = smallest;
                    left = 2 * index + 1;
                }else {
                    break;
                }
            }
        }

        public void heapInsert(Str[] strs, int index){ // 往上整理
            int father = (index - 1) / 2;
            while (strs[index].count < strs[father].count){
                swap(strs, index, father);
                index = father;
                father = (index - 1) / 2;
            }
        }

        public void swap(Str[] strs, int i, int j){
            Str tmp = strs[i];
            strs[i] = strs[j];
            strs[j] = tmp;
            strIndex.put(strs[i], j);
            strIndex.put(strs[j], i);
        }

    }
}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.coloradmin.cn/o/741313.html

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈，一经查实，立即删除！

相关文章

无人机禁飞区地图更新！图新地球全国限飞区自定义地图免费分享！

无人机禁飞区地图更新！图新地球全国限飞区自定义地图免费分享！

随着无人机的普及&#xff0c;人们越来越容易拥有一台无人机。但很多用户并不了解无人机的飞行规则和禁飞限制。对于没有严格遵守规定的人来说&#xff0c;无人机飞行往往会构成公共安全和私人财产的潜在危害。 为此&#xff0c;政府和航空管理机构陆续出台了一系列限制无人机…
阅读更多...
247个Python练习案例附源码(百看不如一练)

247个Python练习案例附源码(百看不如一练)

众所周知&#xff0c;我们在学习Pyhont过程中&#xff0c;大都看书枯燥、看视频进度慢&#xff0c;网上查找的学习案例又比较凌乱不成体系。。。 百看不如一练&#xff0c;今天为大家搜集了一份Python从入门到进阶的实战案例合集&#xff0c;共计247个案例&#xff0c;185页内…
阅读更多...
【数据仓库】BI看板DataEase入坑指南

【数据仓库】BI看板DataEase入坑指南

开头夸夸国产开源BI软件DataEase&#xff0c;支持常见各种报表&#xff0c;还支持图表联动和上下级钻取&#xff0c;超赞有木有&#xff01;&#xff01;&#xff01; 再来为什么说入坑&#xff0c;源码启动各种不服啊。本地用的maven3.5一直导入不了Java项目backend。后来看了…
阅读更多...
【Linux后端服务器开发】缓冲区

【Linux后端服务器开发】缓冲区

目录 一、缓冲区概述 二、语言层面缓冲区 三、C语言模拟实现stdio库 一、缓冲区概述 Linux缓冲区是指在内存中开辟的一块区域&#xff0c;用于存储输入输出数据的临时存储区域。 当应用程序向文件或设备进行读写操作时&#xff0c;数据会先被存储到缓冲区中&#xff0c;然…
阅读更多...
MYSQL学习第一天

MYSQL学习第一天

1.创建数据库&#xff0c;删除数据库&#xff0c;查询创建数据的语句&#xff0c;使用数据库&#xff0c;查询当前默认的数据库以及使用的编码方式校验规则 1.1 创建数据库 create database db_name; 1.2 使用数据库 use db_name; 1.3 查询当前使用的数据库 select datab…
阅读更多...
学校公寓管理系统

学校公寓管理系统

学校公寓管理系统是学校管理的重要组成部分&#xff0c;它的主要任务是通过数字化和自动化的方式提高公寓管理的效率&#xff0c;同时为学生提供更优质的服务。这个系统能够处理学生住宿的申请、房间的分配、住宿费用的收取以及公寓设施的使用等各项工作。 首先&#xff0c;学校…
阅读更多...
【MR设备】燧光MR设备极简教程（使用篇）

【MR设备】燧光MR设备极简教程（使用篇）

燧光MR设备极简教程（使用篇） 一、硬件的基础使用二、定位信标三、投屏直播1、第三人称视角同步MR2、第一人称视角无线投屏相关文章：燧光MR设备极简教程（开发篇） 一、硬件的基础使用 官方使用文档：https://developer.ximmerse.com/#/example?id=6 1. 开机： 长按Home…
阅读更多...
漫谈大数据时代的个人信息安全（一）——“按图索骥”

漫谈大数据时代的个人信息安全（一）——“按图索骥”

大数据时代的个人信息安全系列——“按图索骥” 一、寻找王珞丹二、啥是Exif &#xff1f;三、个人信息保护小贴士 近日&#xff0c;某高校毕业生在校期间窃取学校内网数据&#xff0c;收集全校学生个人隐私信息的新闻引发了人们对大数据时代个人信息安全问题的再度关注。在大数…
阅读更多...
今日教会你录播课实时翻译怎么弄

今日教会你录播课实时翻译怎么弄

在数字时代的浪潮中&#xff0c;视频教学是一种重要的教学方式。无论在网络教学平台&#xff0c;还是在大学教育或公司的培训中&#xff0c;录制课程都以其灵活、方便的特点&#xff0c;给广大师生提供了极大的便利。但是&#xff0c;随着国际间交往的不断深入&#xff0c;语言…
阅读更多...
【第七章】习题

【第七章】习题

1、 下列代码创建了几个对象 public class stringPool {public static void main(String[] args) {String s1 new String("abc");String s2 new String("abc");if (s1 s2) {System.out.println("在堆中只创建了一个对象");} else {System.out…
阅读更多...
(vue)整个页面添加背景视频

(vue)整个页面添加背景视频

(vue)整个页面添加背景视频 App.vue <template><div id"app" :class"[platform]"><video src"./assets/images/top/bg-video-711.mp4" autoplay muted loop class"bg"></video><router-view /></di…
阅读更多...
智能优化算法——灰狼优化算法（PythonMatlab实现）

智能优化算法——灰狼优化算法（PythonMatlab实现）

目录 1 灰狼优化算法基本思想 2 灰狼捕食猎物过程 2.1 社会等级分层 2.2 包围猎物 2.3 狩猎 2.4 攻击猎物 2.5 寻找猎物 3 实现步骤及程序框图 3.1 步骤 3.2 程序框图 4 Python代码实现 5 Matlab实现 1 灰狼优化算法基本思想 灰狼优化算法是一种群智能优化算法&#xff0c;它的…
阅读更多...
JAVA8 实体类集合多个字段组合排序

JAVA8 实体类集合多个字段组合排序

实体类集合多个字段组合排序&#xff0c;自由组合升降序&#xff0c;下面是参考代码示例&#xff1a; import lombok.Data;Data public class DbjrdmxDTO {private String djbh;private String rq; //订单日期private String ckdm;private String ckmc;private String newKhSig…
阅读更多...
Coggle 30 Days of ML（23年7月）任务九：学会Bert基础，transformer库基础使用

Coggle 30 Days of ML（23年7月）任务九：学会Bert基础，transformer库基础使用

Coggle 30 Days of ML&#xff08;23年7月&#xff09;任务九&#xff1a;学会Bert基础&#xff0c;transformer库基础使用 任务九&#xff1a;学会Bert基础&#xff0c;transformer库基础使用 说明&#xff1a;在这个任务中&#xff0c;你将学习Bert模型的基础知识&#xff…
阅读更多...
【使用字符串转换时间问题？】Tue Jul 11 23:59:59 CST 2023

【使用字符串转换时间问题？】Tue Jul 11 23:59:59 CST 2023

问题展示&#xff1a;想要去除多余显示只显示&#xff08;时分秒&#xff1a;23:59:59&#xff09; 解决办法&#xff1a; 问题解决 实现代码&#xff1a; String dateString "2023-07-11 23:59:59";SimpleDateFormat inputFormat new SimpleDateFormat("…
阅读更多...
异常处理一例

异常处理一例

1.现象 代码片段&#xff1a; uint8_t CmdListener(char c) { #define CMD_SIZE 5static uint8_t cmdQueue[9];static uint8_t cmdReset[] { !, b, o, o, t};static uint8_t cmdYModem[] { 0x01, 0x00, 0xff };static uint8_t cmdIdx 0;int i;xlog("%c", 0xcc);…
阅读更多...
【LeetCode】HOT 100（27）

【LeetCode】HOT 100（27）

题单介绍&#xff1a; 精选 100 道力扣&#xff08;LeetCode&#xff09;上最热门的题目&#xff0c;适合初识算法与数据结构的新手和想要在短时间内高效提升的人&#xff0c;熟练掌握这 100 道题&#xff0c;你就已经具备了在代码世界通行的基本能力。 目录 题单介绍&#…
阅读更多...
【Linux】ELK 企业级日志分析系统

【Linux】ELK 企业级日志分析系统

提示&#xff1a;文章写完后&#xff0c;目录可以自动生成&#xff0c;如何生成可参考右边的帮助文档 ELK 企业级日志分析系统 ELK 概述1、ELK 简介2、为什么要使用 ELK3、完整日志系统基本特征4、ELK 的工作原理 ELK Elasticsearch 集群部署&#xff08;在Node1、Node2节点上操…
阅读更多...
stm32（串口知识点）

stm32（串口知识点）

HAL串口发送/接收函数&#xff1a; HAL_UART_Transmit(); 串口发送数据&#xff0c;使用超时管理机制HAL_UART_Receive(); 串口接收数据&#xff0c;使用超时管理机制HAL_UART_Transmit_IT(); 串口中断模式发送 HAL_UART_Receive_IT(); 串口中断模式接收 HAL_UART_Transmit(…
阅读更多...
ByteBuddy学习笔记

ByteBuddy学习笔记

ByteBuddy 1.ByteBuddy的用途 ByteBuddy通过修改字节码来新增、修改、删除Java类的现有功能&#xff0c;主要用于分离功能代码和非功能代码&#xff0c;比如 比如非功能代码如下&#xff1a; public double calculatePrice(){double discount getDiscount();double price …
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023