Problem - 1817B - Codeforces

给定一个具有n个节点和m条边的简单无向图。请注意,该图不一定是连通的。节点从1到n标记。
如果图包含具有特殊节点u的简单循环,则定义图为Fish Graph。除循环中的边之外,图应恰好有2条额外的边。两条边都应连接到节点u,但它们不应连接到循环的任何其他节点。
确定图是否包含子图,其中包含Fish图,并且如果是这样,请找到任何此类子图。
在本问题中,我们将子图定义为通过取原始图的任意边集获得的图像。
输入:
第一行输入整数t(1≤t≤1000),表示测试用例数量。接下来是测试用例的描述。
每个测试用例的第一行包含两个整数n和m(1≤n,m≤2000)——节点数和边数。
接下来的m行中的每一行都包含一条边的描述。每行包含两个整数ui和vi(1≤ui,vi≤n,ui≠vi)——一条边连接节点ui到节点vi。
保证没有两条边连接相同的无序节点对。
此外,保证所有测试用例的n总和和m总和均不超过2000。
输出:
对于每个测试用例,如果图包含子图,则输出“YES”,其为Fish图,否则打印“NO”。如果答案是“YES”,则在以下行中输出子图的说明。
说明的第一行包含一个整数k——子图的边数。
在接下来的k行中,输出所选子图的边缘。每个k行都应包含两个整数u和v(1≤u,v≤n,u≠v)——连接u和v的边属于子图。打印u和v的顺序无所谓,只要两个节点由原始图中的一条边连接即可。无论如何打印边缘的顺序都没有关系,只要结果子图是Fish Graph。
如果有多个解决方案,请打印任何一个。
示例:
Example
Input
Copy
3
7 8
1 2
2 3
3 4
4 1
4 5
4 6
4 2
6 7
7 7
6 7
1 2
2 3
3 4
4 1
1 3
3 5
4 4
1 3
3 4
4 1
1 2
Output
Copy
YES 6 5 4 6 4 4 3 1 4 2 1 3 2 YES 5 5 3 2 3 3 1 4 3 1 4 NO
题解:
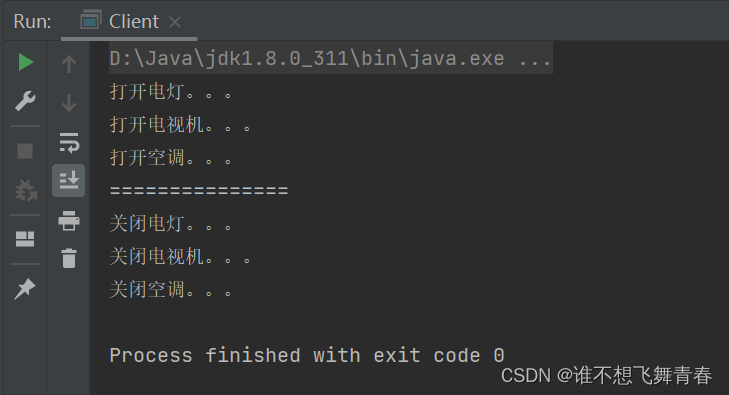
如果有这样的子图,其中一个点度数一定>= 4,一个环上的点外连两条枝干,即使这两条枝干也属于一个环,也没问题,因为我们找的是子图,只要拿特定的边即可
因此我们只需要搜索每个度数>=4的点,看他是否有环,有环就一定是
具体细节代码中都有注释
#include <cstdio>
#include <cstring>
#include <algorithm>
#include<iostream>
#include<vector>
#include<set>
#include<map>
#include<cmath>
#include<queue>
using namespace std;
typedef long long ll;
#define int long long
typedef pair<int,int> PII;
typedef unsigned long long ULL;
const int N = 4e5 + 10;
int mod = 1e9 + 7;
vector<int> p[N];
vector<int> ans;
int st[N];//看当前点是否走过
int vis[N];//标记目标节点相邻的节点
int dfs(int u,int f)
{
if(st[u])
return 0;
ans.push_back(u);//存点
st[u] = 1;
if(vis[u]&&u != f)
return 1;//如果碰到了,与目标节点相邻的节点,并且不是我们最开始的搜索起点,说明存在环
for(auto ne:p[u])
{
if(dfs(ne,f))
return 1;
}
ans.pop_back();//如果无环清空
return 0;
}
void solve()
{
int n,m;
cin >> n >> m;
for(int i = 1;i <= m;i++)
{
int x,y;
cin >> x >> y;
p[x].push_back(y);
p[y].push_back(x);
}
int f = 0;
ans.clear();
for(int i = 1;i <= n;i++)
{
if(p[i].size() < 4)
continue;
for(int j = 1;j <= n;j++)
{
st[j] = 0;
vis[j] = 0;
}
st[i] = 1;
for(auto ne:p[i])
{
vis[ne] = 1;
}
for(auto ne:p[i])
{
if(dfs(ne,ne))
{
f = i;
break;
}
}
if(f)
break;
}
if(f)
{
cout <<"YES\n";
cout << ans.size() + 3 <<"\n";
cout << f <<" "<<ans[0] <<"\n";
cout << f <<" "<<ans[ans.size() - 1] <<"\n";
for(int i = 0;i < ans.size() - 1;i++)
cout << ans[i] <<" " << ans[i + 1] <<"\n";
int c = 0;
for(auto ne:p[f])
{
if(c == 2)
break;
if(ne != ans[0]&&ne != ans[ans.size() - 1])
{
cout <<f <<" " <<ne <<"\n";
c++;
}
}
}
else
{
cout <<"NO\n";
}
for(int i = 1;i <= n;i++)
{
p[i].clear();
}
}
signed main()
{
ios::sync_with_stdio(0 );
cin.tie(0);cout.tie(0);
int t = 1;
cin >> t;
while(t--)
{
solve();
}
}