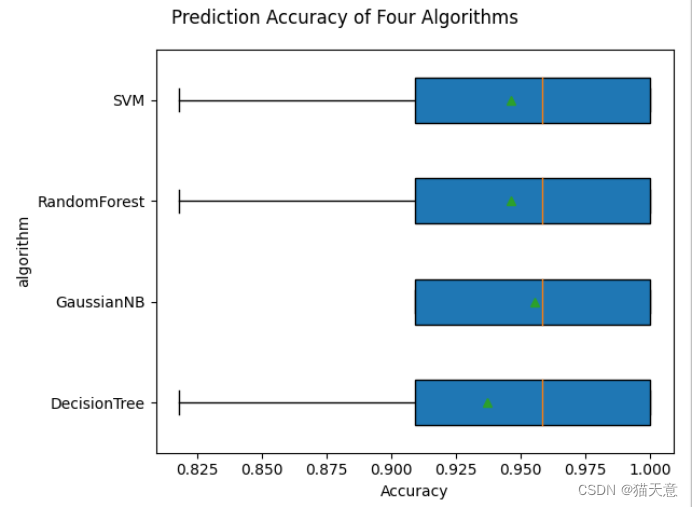
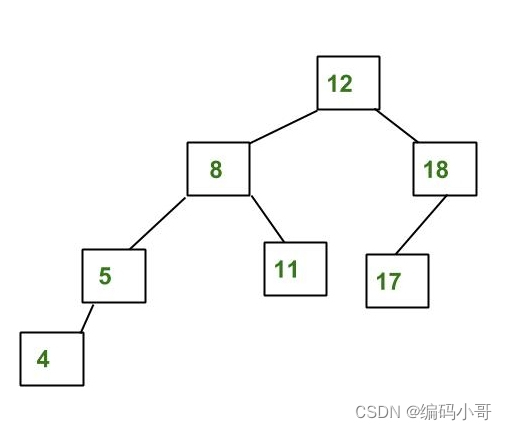
AVL 是一种自平衡二叉搜索树,其中任何节点的左右子树的高度之差不能超过 1。
AVL树的特点:
1、它遵循二叉搜索树的一般属性。
2、树的每个子树都是平衡的,即左右子树的高度之差最多为1。
3、当插入新节点时,树会自我平衡。因此,插入操作非常耗时。
AVL Tree的应用:
1、大多数内存中集和字典都使用 AVL 树进行存储。
2、数据库应用程序(插入和删除不太常见,但需要频繁的数据查找)也经常使用 AVL 树。
3、除了数据库应用程序之外,它还用于其他需要更好搜索的应用程序。
4、有序关联容器(集合、多集、映射和多映射)的大多数 STL 实现都使用红黑树而不是 AVL树。
#include <stdio.h>
#include <stdlib.h>
struct AVLnode
{
int key;
struct AVLnode *left;
struct AVLnode *right;
int height;
};
typedef struct AVLnode avlNode;
int max(int a, int b) { return (a > b) ? a : b; }
avlNode *newNode(int key)
{
avlNode *node = (avlNode *)malloc(sizeof(avlNode));
if (node == NULL)
printf("!! Out of Space !!\n");
else
{
node->key = key;
node->left = NULL;
node->right = NULL;
node->height = 0;
}
return node;
}
int nodeHeight(avlNode *node)
{
if (node == NULL)
return -1;
else
return (node->height);
}
int heightDiff(avlNode *node)
{
if (node == NULL)
return 0;
else
return (nodeHeight(node->left) - nodeHeight(node->right));
}
/* 返回左子树中最小值的节点*/
avlNode *minNode(avlNode *node)
{
avlNode *temp = node;
while (temp->left != NULL) temp = temp->left;
return temp;
}
void printAVL(avlNode *node, int level)
{
int i;
if (node != NULL)
{
printAVL(node->right, level + 1);
printf("\n\n");
for (i = 0; i < level; i++) printf("\t");
printf("%d", node->key);
printAVL(node->left, level + 1);
}
}
avlNode *rightRotate(avlNode *z)
{
avlNode *y = z->left;
avlNode *T3 = y->right;
y->right = z;
z->left = T3;
z->height = (max(nodeHeight(z->left), nodeHeight(z->right)) + 1);
y->height = (max(nodeHeight(y->left), nodeHeight(y->right)) + 1);
return y;
}
avlNode *leftRotate(avlNode *z)
{
avlNode *y = z->right;
avlNode *T3 = y->left;
y->left = z;
z->right = T3;
z->height = (max(nodeHeight(z->left), nodeHeight(z->right)) + 1);
y->height = (max(nodeHeight(y->left), nodeHeight(y->right)) + 1);
return y;
}
avlNode *LeftRightRotate(avlNode *z)
{
z->left = leftRotate(z->left);
return (rightRotate(z));
}
avlNode *RightLeftRotate(avlNode *z)
{
z->right = rightRotate(z->right);
return (leftRotate(z));
}
avlNode *insert(avlNode *node, int key)
{
if (node == NULL)
return (newNode(key));
/*二叉搜索树插入*/
if (key < node->key)
node->left =
insert(node->left, key); /*递归插入左子树*/
else if (key > node->key)
node->right =
insert(node->right, key); /*递归插入右子树*/
/*计算节点高度*/
node->height = (max(nodeHeight(node->left), nodeHeight(node->right)) + 1);
/*检查平衡性*/
int balance = heightDiff(node);
/*左左*/
if (balance > 1 && key < (node->left->key))
return rightRotate(node);
/*右右*/
if (balance < -1 && key > (node->right->key))
return leftRotate(node);
/*左右*/
if (balance > 1 && key > (node->left->key))
{
node = LeftRightRotate(node);
}
/*右左*/
if (balance < -1 && key < (node->right->key))
{
node = RightLeftRotate(node);
}
return node;
}
avlNode *delete (avlNode *node, int queryNum)
{
if (node == NULL)
return node;
if (queryNum < node->key)
node->left =
delete (node->left, queryNum); /*Recursive deletion in L subtree*/
else if (queryNum > node->key)
node->right =
delete (node->right, queryNum); /*Recursive deletion in R subtree*/
else
{
/*单节点或者没有子节点*/
if ((node->left == NULL) || (node->right == NULL))
{
avlNode *temp = node->left ? node->left : node->right;
/*没有子节点*/
if (temp == NULL)
{
temp = node;
node = NULL;
}
else /*单节点*/
*node = *temp;
free(temp);
}
else
{
/*两个孩子节点*/
/*获取右子树最小值的节点*/
avlNode *temp = minNode(node->right);
node->key = temp->key; /*拷贝到根节点*/
node->right =
delete (node->right,
temp->key); /*删除右子树最小值的节点*/
}
}
/*单节点*/
if (node == NULL)
return node;
/*更新高度*/
node->height = (max(nodeHeight(node->left), nodeHeight(node->right)) + 1);
int balance = heightDiff(node);
/*左左*/
if ((balance > 1) && (heightDiff(node->left) >= 0))
return rightRotate(node);
/*左右*/
if ((balance > 1) && (heightDiff(node->left) < 0))
{
node = LeftRightRotate(node);
}
/*右右*/
if ((balance < -1) && (heightDiff(node->right) >= 0))
return leftRotate(node);
/*右左*/
if ((balance < -1) && (heightDiff(node->right) < 0))
{
node = RightLeftRotate(node);
}
return node;
}
avlNode *findNode(avlNode *node, int queryNum)
{
if (node != NULL)
{
if (queryNum < node->key)
node = findNode(node->left, queryNum);
else if (queryNum > node->key)
node = findNode(node->right, queryNum);
}
return node;
}
void printPreOrder(avlNode *node)
{
if (node == NULL)
return;
printf(" %d ", (node->key));
printPreOrder(node->left);
printPreOrder(node->right);
}
void printInOrder(avlNode *node)
{
if (node == NULL)
return;
printInOrder(node->left);
printf(" %d ", (node->key));
printInOrder(node->right);
}
void printPostOrder(avlNode *node)
{
if (node == NULL)
return;
printPostOrder(node->left);
printPostOrder(node->right);
printf(" %d ", (node->key));
}
int main()
{
int choice;
int flag = 1;
int insertNum;
int queryNum;
avlNode *root = NULL;
avlNode *tempNode;
while (flag == 1)
{
printf("\n\nEnter the Step to Run : \n");
printf("\t1: Insert a node into AVL tree\n");
printf("\t2: Delete a node in AVL tree\n");
printf("\t3: Search a node into AVL tree\n");
printf("\t4: printPreOrder (Ro L R) Tree\n");
printf("\t5: printInOrder (L Ro R) Tree\n");
printf("\t6: printPostOrder (L R Ro) Tree\n");
printf("\t7: printAVL Tree\n");
printf("\t0: EXIT\n");
scanf("%d", &choice);
switch (choice)
{
case 0:
{
flag = 0;
printf("\n\t\tExiting, Thank You !!\n");
break;
}
case 1:
{
printf("\n\tEnter the Number to insert: ");
scanf("%d", &insertNum);
tempNode = findNode(root, insertNum);
if (tempNode != NULL)
printf("\n\t %d Already exists in the tree\n", insertNum);
else
{
printf("\n\tPrinting AVL Tree\n");
printAVL(root, 1);
printf("\n");
root = insert(root, insertNum);
printf("\n\tPrinting AVL Tree\n");
printAVL(root, 1);
printf("\n");
}
break;
}
case 2:
{
printf("\n\tEnter the Number to Delete: ");
scanf("%d", &queryNum);
tempNode = findNode(root, queryNum);
if (tempNode == NULL)
printf("\n\t %d Does not exist in the tree\n", queryNum);
else
{
printf("\n\tPrinting AVL Tree\n");
printAVL(root, 1);
printf("\n");
root = delete (root, queryNum);
printf("\n\tPrinting AVL Tree\n");
printAVL(root, 1);
printf("\n");
}
break;
}
case 3:
{
printf("\n\tEnter the Number to Search: ");
scanf("%d", &queryNum);
tempNode = findNode(root, queryNum);
if (tempNode == NULL)
printf("\n\t %d : Not Found\n", queryNum);
else
{
printf("\n\t %d : Found at height %d \n", queryNum,
tempNode->height);
printf("\n\tPrinting AVL Tree\n");
printAVL(root, 1);
printf("\n");
}
break;
}
case 4:
{
printf("\nPrinting Tree preOrder\n");
printPreOrder(root);
break;
}
case 5:
{
printf("\nPrinting Tree inOrder\n");
printInOrder(root);
break;
}
case 6:
{
printf("\nPrinting Tree PostOrder\n");
printPostOrder(root);
break;
}
case 7:
{
printf("\nPrinting AVL Tree\n");
printAVL(root, 1);
break;
}
default:
{
flag = 0;
printf("\n\t\tExiting, Thank You !!\n");
break;
}
}
}
return 0;
}