对于同一层次中的指标，通过两两比较，构造出判断矩阵，记为A。

aij表示因素i对因素j的重要性比较结果，可采用1-9的量化比例标度来反映其重要程度。

1：指标两个相比，A比B同等重要

3：指标两个相比，A比B稍微重要

5：指标两个相比，A比B明显重要

7：指标两个相比，A比B强烈重要

8：指标两个相比，A比B极端重要

计算权重向量（使用算数平均法计算）：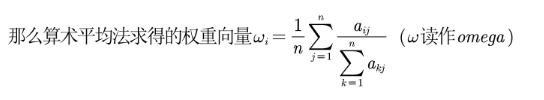 

  (3)一致性检验：

λm:判断矩阵A的最大特征值

CI：一致性检验指标，n是判断矩阵的阶数

CR：检验系数，当CR小于0.1时视为通过

代码：

%输入：比较矩阵
%输出：w为各权重值,CI,CR为一致性检验


%Output prompt information
disp('INPUT A');
%Input comparison matrix
A=input('A=');
[n,n]=size(A);
x=ones(n,100);
y=ones(n,100);
m=zeros(1,100);
m(1)=max(x(:,1));
y(:,1)=x(:,1);
x(:,2)=A*y(:,1);
m(2)=max(x(:,2));
y(:,2)=x(:,2)/m(2);
p=0.0001;i=2;k=abs(m(2)-m(1));
%Calculate weight
while  k>p
  i=i+1;
  x(:,i)=A*y(:,i-1);
  m(i)=max(x(:,i));
  y(:,i)=x(:,i)/m(i);
  k=abs(m(i)-m(i-1));
end
a=sum(y(:,i));
w=y(:,i)/a;
t=m(i);
disp(w);
%Consistency inspection
CI=(t-n)/(n-1);RI=[0 0 0.52 0.89 1.12 1.26 1.36 1.41 1.46 1.49 1.52 1.54 1.56 1.58 1.59];
CR=CI/RI(n);
%Output inspection results
if CR<0.10
    disp('The consistency of this matrix is acceptable!');
    disp('CI=');disp(CI);
    disp('CR=');disp(CR);
end