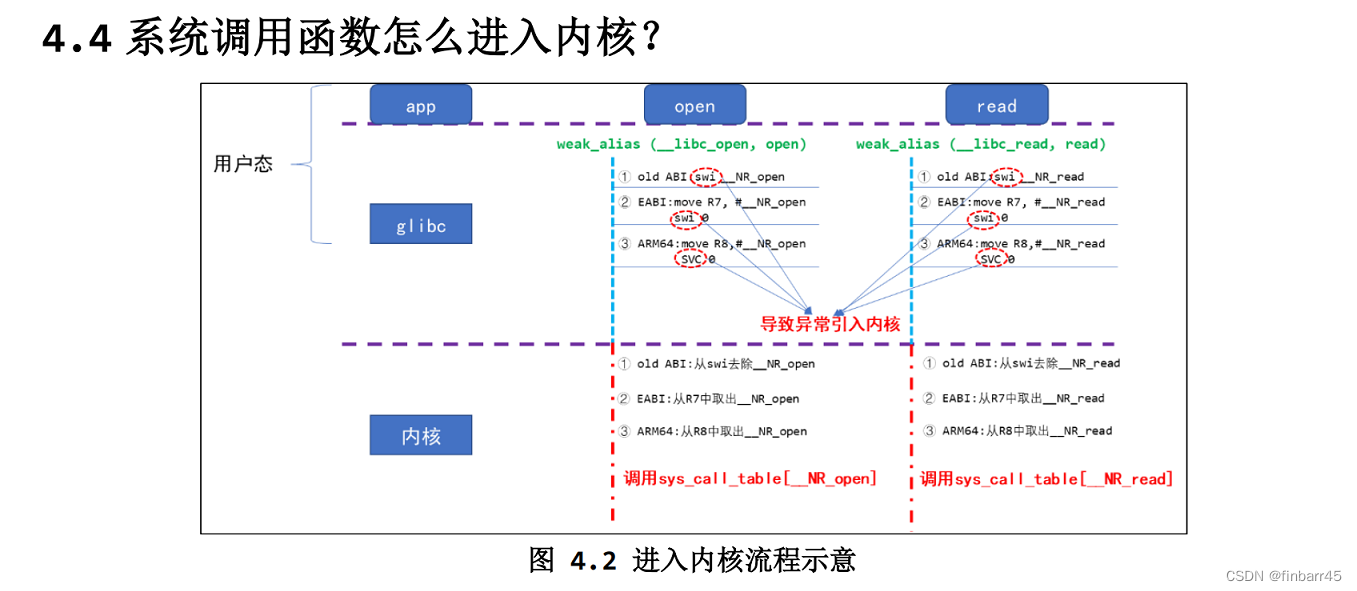
1、题目
给定一个数组 prices ,它的第 i 个元素 prices[i] 表示一支给定股票第 i 天的价格。
你只能选择 某一天 买入这只股票,并选择在 未来的某一个不同的日子 卖出该股票。设计一个算法来计算你所能获取的最大利润。
返回你可以从这笔交易中获取的最大利润。如果你不能获取任何利润,返回 0 。
2、示例
示例1:
输入:[7,1,5,3,6,4] 输出:5 解释:在第 2 天(股票价格 = 1)的时候买入,在第 5 天(股票价格 = 6)的时候卖出,最大利润 = 6-1 = 5 。 注意利润不能是 7-1 = 6, 因为卖出价格需要大于买入价格;同时,你不能在买入前卖出股票。
示例2:
输入:prices = [7,6,4,3,1] 输出:0 解释:在这种情况下, 没有交易完成, 所以最大利润为 0。
3、解题思路
该题有两种解决方法:
1.暴力法:
通过遍历取出数组中的每一个元素,并跟剩下的元素进行求差的结果再与最大利润进行比较,如此循环找出最大利润值。
2.动态规划法(优解):
首先假设第i天是获取最大的利益值,那么购入时候肯定是在集合[0,i-1]的范围里面找到其中的最小值,然后两者的价格相减就是我们要的最大利益。
4、LeetCode代码与案例代码
1.暴力法
LeetCode代码:
class Solution {
public int maxProfit(int[] prices) {
int maxProfit = 0;
for (int i=0;i< prices.length-1;i++){
for (int j=i+1;j< prices.length;j++){
if (prices[j] - prices[i]>maxProfit){
maxProfit = prices[j] - prices[i];
}
}
}
return maxProfit;
}
}案例代码:
package LettCode05;
public class javaDemo {
public static void main(String[] args) {
int nums[] =new int[]{7,6,4,3,1};
// 暴力解法
int maxProfit = 0;
for (int i=0;i< nums.length-1;i++){
for (int j=i+1;j< nums.length;j++){
if (nums[j] - nums[i]>maxProfit){
maxProfit = nums[j] - nums[i];
}
}
}
System.out.println("最大利润为"+maxProfit);
}
}

总结:时间复杂度为O(n^2),空间复杂度为O(1);
2.动态规划法
LeetCode代码:
class Solution {
public int maxProfit(int[] prices) {
int lowPrice = Integer.MAX_VALUE;
int max_profit= 0;
for(int i=0;i<prices.length;i++){
if (prices[i]<lowPrice){
lowPrice = prices[i];
}else if(prices[i] - lowPrice >max_profit){
max_profit = prices[i] - lowPrice;
}
}
return max_profit;
}
}案例代码:
package LeetCode06;
public class javaDemo {
public static void main(String[] args) {
int prices[] = new int[]{7,1,5,3,6,4};
// 动态规划
int max_profit = 0;
int lowPrice = Integer.MAX_VALUE;
for (int i=0;i<prices.length;i++){
// 找到第i天前的最小值
if (prices[i]<lowPrice){
lowPrice = prices[i];
// 某天的值减去这天前的最小值就是这天的最大利益
// 通过比较每一天的利益大小得到最大利益
}else if (prices[i]-lowPrice>max_profit){
max_profit = prices[i]-lowPrice;
}
}
System.out.println("最大利润为"+max_profit);
}
}
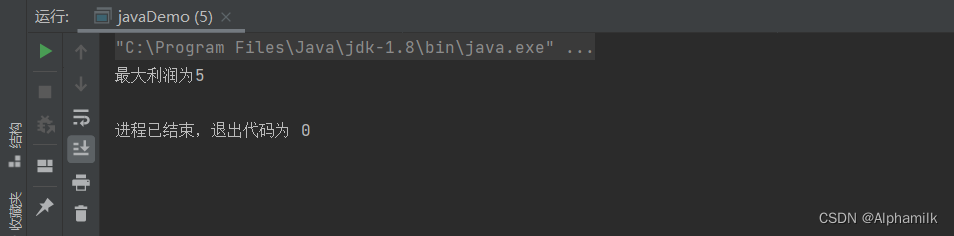
总结:该方法的时间复杂度为O(n),空间复杂度为O(1)