35.利用fminsearch解多元变量无约束条件下的函数最小值（matlab程序）

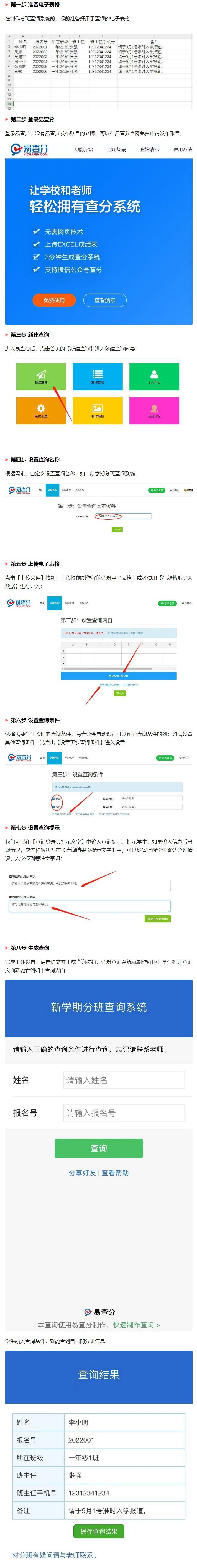
1.简述

1.fminsearch函数基本语法

函数功能：使用无导数法计算无约束多变量函数的最小值

语法
x = fminsearch(fun,x0)
x = fminsearch(fun,x0,options)
x = fminsearch(problem)
[x,fval] = fminsearch(___)
[x,fval,exitflag] = fminsearch(___)
[x,fval,exitflag,output] = fminsearch(___)
说明
非线性规划求解器。搜索由以下公式指定的问题的最小值：
min f(x)
f(x) 是返回标量的函数，x 是向量或矩阵；请参阅矩阵参数。
x = fminsearch(fun,x0) 在点 x0 处开始并尝试求 fun 中描述的函数的局部最小值 x。
x = fminsearch(fun,x0,options) 使用 options 所指定的优化选项执行最小化。使用 optimset 可设置这些选项。
x = fminsearch(problem) 求 problem 的最小值，它是 problem 中所述的一个结构体。
[x,fval] = fminsearch(___)，对任何上述输入语法，在 fval 中返回目标函数 fun 在解 x 处的值。
[x,fval,exitflag] = fminsearch(___) 还返回描述退出条件的值 exitflag。
[x,fval,exitflag,output] = fminsearch(___) 还会返回结构体 output 以及有关优化过程的信息。

2.代码

主程序：

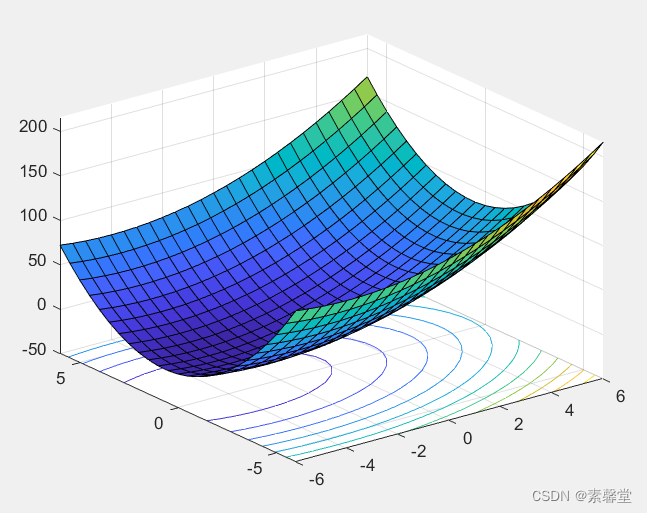
% 通过绘图确定一个初始值；然后进行迭代找到真正的最小值；
clc
clear
[x,y]=meshgrid(-6:.5:6);
f= 8*x-4*y +x.^2+3*y.^2;
surfc(x,y,f)
x0=[0,0];
%[x,fval,exitflag]=fminunc(@(x)(8*x(1)-4*x(2) +x(1).^2+3*x(2).^2),x0)
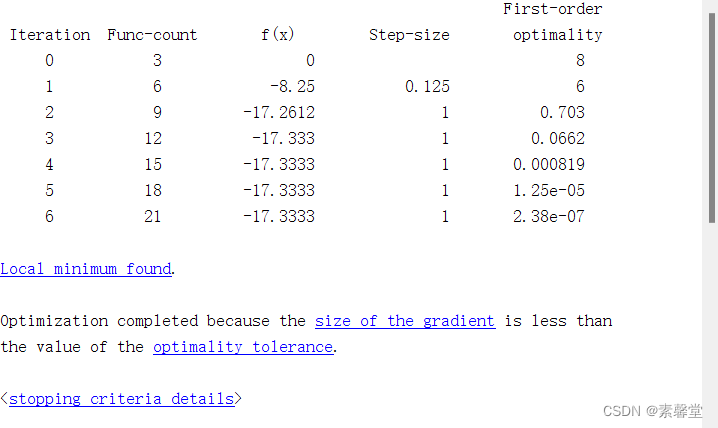
options=optimset('display','iter','Tolx',1e-8);
[x,fval,exitflag]=fminunc(@(x)(8*x(1)-4*x(2) +x(1).^2+3*x(2).^2),x0,options)
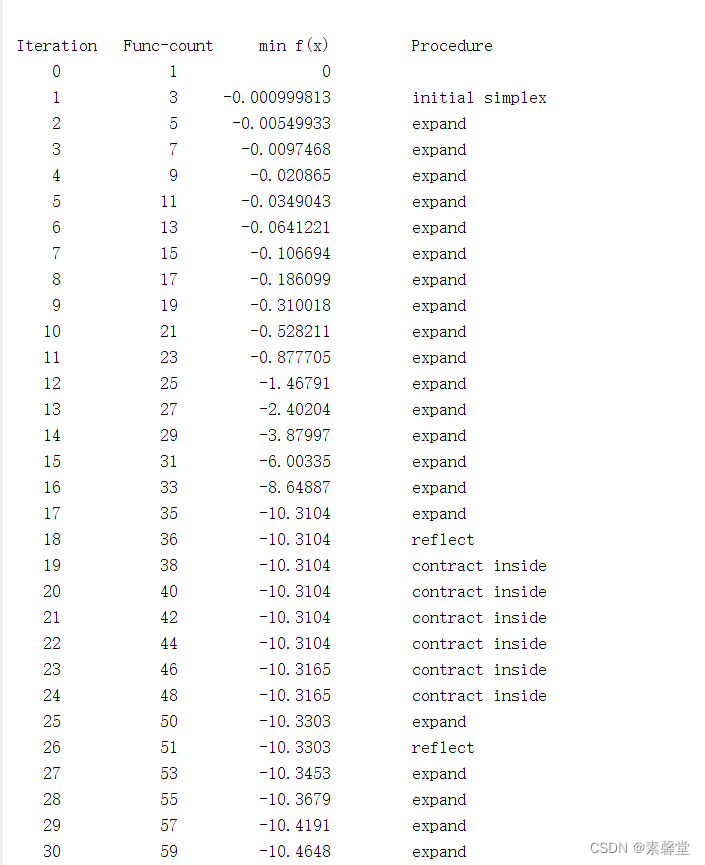
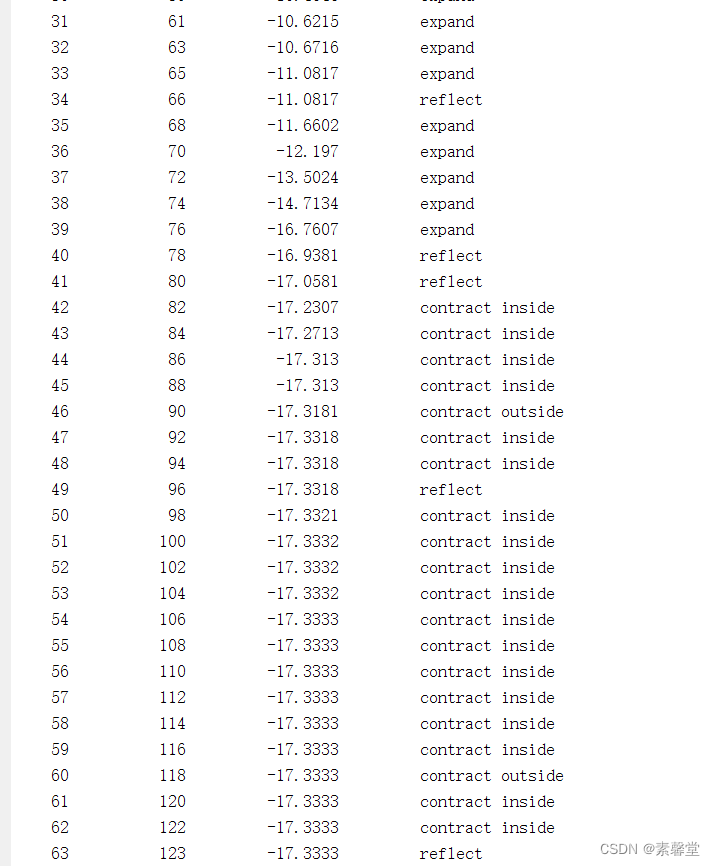
[x_fminsearch,fval_fminsearch,exitflag]=fminsearch(@(x)(8*x(1)-4*x(2) +x(1).^2+3*x(2).^2),x0,options)

子程序：

function [x,fval,exitflag,output] = fminsearch(funfcn,x,options,varargin)
%FMINSEARCH Multidimensional unconstrained nonlinear minimization (Nelder-Mead).
% X = FMINSEARCH(FUN,X0) starts at X0 and attempts to find a local minimizer
% X of the function FUN. FUN is a function handle. FUN accepts input X and
% returns a scalar function value F evaluated at X. X0 can be a scalar, vector
% or matrix.
%
% X = FMINSEARCH(FUN,X0,OPTIONS) minimizes with the default optimization
% parameters replaced by values in the structure OPTIONS, created
% with the OPTIMSET function. See OPTIMSET for details. FMINSEARCH uses
% these options: Display, TolX, TolFun, MaxFunEvals, MaxIter, FunValCheck,
% PlotFcns, and OutputFcn.
%
% X = FMINSEARCH(PROBLEM) finds the minimum for PROBLEM. PROBLEM is a
% structure with the function FUN in PROBLEM.objective, the start point
% in PROBLEM.x0, the options structure in PROBLEM.options, and solver
% name 'fminsearch' in PROBLEM.solver.
%
% [X,FVAL]= FMINSEARCH(...) returns the value of the objective function,
% described in FUN, at X.
%
% [X,FVAL,EXITFLAG] = FMINSEARCH(...) returns an EXITFLAG that describes
% the exit condition. Possible values of EXITFLAG and the corresponding
% exit conditions are
%
% 1 Maximum coordinate difference between current best point and other
% points in simplex is less than or equal to TolX, and corresponding
% difference in function values is less than or equal to TolFun.
% 0 Maximum number of function evaluations or iterations reached.
% -1 Algorithm terminated by the output function.
%
% [X,FVAL,EXITFLAG,OUTPUT] = FMINSEARCH(...) returns a structure
% OUTPUT with the number of iterations taken in OUTPUT.iterations, the
% number of function evaluations in OUTPUT.funcCount, the algorithm name
% in OUTPUT.algorithm, and the exit message in OUTPUT.message.
%
% Examples
% FUN can be specified using @:
% X = fminsearch(@sin,3)
% finds a minimum of the SIN function near 3.
% In this case, SIN is a function that returns a scalar function value
% SIN evaluated at X.
%
% FUN can be an anonymous function:
% X = fminsearch(@(x) norm(x),[1;2;3])
% returns a point near the minimizer [0;0;0].
%
% FUN can be a parameterized function. Use an anonymous function to
% capture the problem-dependent parameters:
% f = @(x,c) x(1).^2+c.*x(2).^2; % The parameterized function.
% c = 1.5; % The parameter.
% X = fminsearch(@(x) f(x,c),[0.3;1])
%
% FMINSEARCH uses the Nelder-Mead simplex (direct search) method.
%
% See also OPTIMSET, FMINBND, FUNCTION_HANDLE.

% Reference: Jeffrey C. Lagarias, James A. Reeds, Margaret H. Wright,
% Paul E. Wright, "Convergence Properties of the Nelder-Mead Simplex
% Method in Low Dimensions", SIAM Journal of Optimization, 9(1):
% p.112-147, 1998.

defaultopt = struct('Display','notify','MaxIter','200*numberOfVariables',...
'MaxFunEvals','200*numberOfVariables','TolX',1e-4,'TolFun',1e-4, ...
'FunValCheck','off','OutputFcn',[],'PlotFcns',[]);

% If just 'defaults' passed in, return the default options in X
if nargin == 1 && nargout <= 1 && strcmpi(funfcn,'defaults')
x = defaultopt;
return
end

if nargin < 3, options = []; end

% Detect problem structure input
if nargin == 1
if isa(funfcn,'struct')
[funfcn,x,options] = separateOptimStruct(funfcn);
else % Single input and non-structure
error('MATLAB:fminsearch:InputArg',...
getString(message('MATLAB:optimfun:fminsearch:InputArg')));
end
end

if nargin == 0
error('MATLAB:fminsearch:NotEnoughInputs',...
getString(message('MATLAB:optimfun:fminsearch:NotEnoughInputs')));
end

% Check for non-double inputs
if ~isa(x,'double')
error('MATLAB:fminsearch:NonDoubleInput',...
getString(message('MATLAB:optimfun:fminsearch:NonDoubleInput')));
end

n = numel(x);
numberOfVariables = n;

% Check that options is a struct
if ~isempty(options) && ~isa(options,'struct')
error('MATLAB:fminsearch:ArgNotStruct',...
getString(message('MATLAB:optimfun:commonMessages:ArgNotStruct', 3)));
end

printtype = optimget(options,'Display',defaultopt,'fast');
tolx = optimget(options,'TolX',defaultopt,'fast');
tolf = optimget(options,'TolFun',defaultopt,'fast');
maxfun = optimget(options,'MaxFunEvals',defaultopt,'fast');
maxiter = optimget(options,'MaxIter',defaultopt,'fast');
funValCheck = strcmp(optimget(options,'FunValCheck',defaultopt,'fast'),'on');

% In case the defaults were gathered from calling: optimset('fminsearch'):
if ischar(maxfun) || isstring(maxfun)
if strcmpi(maxfun,'200*numberofvariables')
maxfun = 200*numberOfVariables;
else
error('MATLAB:fminsearch:OptMaxFunEvalsNotInteger',...
getString(message('MATLAB:optimfun:fminsearch:OptMaxFunEvalsNotInteger')));
end
end
if ischar(maxiter) || isstring(maxiter)
if strcmpi(maxiter,'200*numberofvariables')
maxiter = 200*numberOfVariables;
else
error('MATLAB:fminsearch:OptMaxIterNotInteger',...
getString(message('MATLAB:optimfun:fminsearch:OptMaxIterNotInteger')));
end
end

switch printtype
case {'notify','notify-detailed'}
prnt = 1;
case {'none','off'}
prnt = 0;
case {'iter','iter-detailed'}
prnt = 3;
case {'final','final-detailed'}
prnt = 2;
case 'simplex'
prnt = 4;
otherwise
prnt = 1;
end
% Handle the output
outputfcn = optimget(options,'OutputFcn',defaultopt,'fast');
if isempty(outputfcn)
haveoutputfcn = false;
else
haveoutputfcn = true;
xOutputfcn = x; % Last x passed to outputfcn; has the input x's shape
% Parse OutputFcn which is needed to support cell array syntax for OutputFcn.
outputfcn = createCellArrayOfFunctions(outputfcn,'OutputFcn');
end

% Handle the plot
plotfcns = optimget(options,'PlotFcns',defaultopt,'fast');
if isempty(plotfcns)
haveplotfcn = false;
else
haveplotfcn = true;
xOutputfcn = x; % Last x passed to plotfcns; has the input x's shape
% Parse PlotFcns which is needed to support cell array syntax for PlotFcns.
plotfcns = createCellArrayOfFunctions(plotfcns,'PlotFcns');
end

header = ' Iteration Func-count min f(x) Procedure';

% Convert to function handle as needed.
funfcn = fcnchk(funfcn,length(varargin));
% Add a wrapper function to check for Inf/NaN/complex values
if funValCheck
% Add a wrapper function, CHECKFUN, to check for NaN/complex values without
% having to change the calls that look like this:
% f = funfcn(x,varargin{:});
% x is the first argument to CHECKFUN, then the user's function,
% then the elements of varargin. To accomplish this we need to add the
% user's function to the beginning of varargin, and change funfcn to be
% CHECKFUN.
varargin = [{funfcn}, varargin];
funfcn = @checkfun;
end

n = numel(x);

% Initialize parameters
rho = 1; chi = 2; psi = 0.5; sigma = 0.5;
onesn = ones(1,n);
two2np1 = 2:n+1;
one2n = 1:n;

% Set up a simplex near the initial guess.
xin = x(:); % Force xin to be a column vector
v = zeros(n,n+1); fv = zeros(1,n+1);
v(:,1) = xin; % Place input guess in the simplex! (credit L.Pfeffer at Stanford)
x(:) = xin; % Change x to the form expected by funfcn
fv(:,1) = funfcn(x,varargin{:});
func_evals = 1;
itercount = 0;
how = '';
% Initial simplex setup continues later

% Initialize the output and plot functions.
if haveoutputfcn || haveplotfcn
[xOutputfcn, optimValues, stop] = callOutputAndPlotFcns(outputfcn,plotfcns,v(:,1),xOutputfcn,'init',itercount, ...
func_evals, how, fv(:,1),varargin{:});
if stop
[x,fval,exitflag,output] = cleanUpInterrupt(xOutputfcn,optimValues);
if prnt > 0
disp(output.message)
end
return;
end
end

% Print out initial f(x) as 0th iteration
if prnt == 3
disp(' ')
disp(header)
fprintf(' %5.0f %5.0f %12.6g %s\n', itercount, func_evals, fv(1), how);
elseif prnt == 4
formatsave.format = get(0,'format');
formatsave.formatspacing = get(0,'formatspacing');
% reset format when done
oc1 = onCleanup(@()set(0,'format',formatsave.format));
oc2 = onCleanup(@()set(0,'formatspacing',formatsave.formatspacing));
format compact
format short e
disp(' ')
disp(how)
disp('v = ')
disp(v)
disp('fv = ')
disp(fv)
disp('func_evals = ')
disp(func_evals)
end
% OutputFcn and PlotFcns call
if haveoutputfcn || haveplotfcn
[xOutputfcn, optimValues, stop] = callOutputAndPlotFcns(outputfcn,plotfcns,v(:,1),xOutputfcn,'iter',itercount, ...
func_evals, how, fv(:,1),varargin{:});
if stop % Stop per user request.
[x,fval,exitflag,output] = cleanUpInterrupt(xOutputfcn,optimValues);
if prnt > 0
disp(output.message)
end
return;
end
end

% Continue setting up the initial simplex.
% Following improvement suggested by L.Pfeffer at Stanford
usual_delta = 0.05; % 5 percent deltas for non-zero terms
zero_term_delta = 0.00025; % Even smaller delta for zero elements of x
for j = 1:n
y = xin;
if y(j) ~= 0
y(j) = (1 + usual_delta)*y(j);
else
y(j) = zero_term_delta;
end
v(:,j+1) = y;
x(:) = y; f = funfcn(x,varargin{:});
fv(1,j+1) = f;
end

% sort so v(1,:) has the lowest function value
[fv,j] = sort(fv);
v = v(:,j);

how = 'initial simplex';
itercount = itercount + 1;
func_evals = n+1;
if prnt == 3
fprintf(' %5.0f %5.0f %12.6g %s\n', itercount, func_evals, fv(1), how)
elseif prnt == 4
disp(' ')
disp(how)
disp('v = ')
disp(v)
disp('fv = ')
disp(fv)
disp('func_evals = ')
disp(func_evals)
end
% OutputFcn and PlotFcns call
if haveoutputfcn || haveplotfcn
[xOutputfcn, optimValues, stop] = callOutputAndPlotFcns(outputfcn,plotfcns,v(:,1),xOutputfcn,'iter',itercount, ...
func_evals, how, fv(:,1),varargin{:});
if stop % Stop per user request.
[x,fval,exitflag,output] = cleanUpInterrupt(xOutputfcn,optimValues);
if prnt > 0
disp(output.message)
end
return;
end
end
exitflag = 1;

% Main algorithm: iterate until
% (a) the maximum coordinate difference between the current best point and the
% other points in the simplex is less than or equal to TolX. Specifically,
% until max(||v2-v1||,||v3-v1||,...,||v(n+1)-v1||) <= TolX,
% where ||.|| is the infinity-norm, and v1 holds the
% vertex with the current lowest value; AND
% (b) the corresponding difference in function values is less than or equal
% to TolFun. (Cannot use OR instead of AND.)
% The iteration stops if the maximum number of iterations or function evaluations
% are exceeded
while func_evals < maxfun && itercount < maxiter
if max(abs(fv(1)-fv(two2np1))) <= max(tolf,10*eps(fv(1))) && ...
max(max(abs(v(:,two2np1)-v(:,onesn)))) <= max(tolx,10*eps(max(v(:,1))))
break
end

% Compute the reflection point

% xbar = average of the n (NOT n+1) best points
xbar = sum(v(:,one2n), 2)/n;
xr = (1 + rho)*xbar - rho*v(:,end);
x(:) = xr; fxr = funfcn(x,varargin{:});
func_evals = func_evals+1;

if fxr < fv(:,1)
% Calculate the expansion point
xe = (1 + rho*chi)*xbar - rho*chi*v(:,end);
x(:) = xe; fxe = funfcn(x,varargin{:});
func_evals = func_evals+1;
if fxe < fxr
v(:,end) = xe;
fv(:,end) = fxe;
how = 'expand';
else
v(:,end) = xr;
fv(:,end) = fxr;
how = 'reflect';
end
else % fv(:,1) <= fxr
if fxr < fv(:,n)
v(:,end) = xr;
fv(:,end) = fxr;
how = 'reflect';
else % fxr >= fv(:,n)
% Perform contraction
if fxr < fv(:,end)
% Perform an outside contraction
xc = (1 + psi*rho)*xbar - psi*rho*v(:,end);
x(:) = xc; fxc = funfcn(x,varargin{:});
func_evals = func_evals+1;

if fxc <= fxr
v(:,end) = xc;
fv(:,end) = fxc;
how = 'contract outside';
else
% perform a shrink
how = 'shrink';
end
else
% Perform an inside contraction
xcc = (1-psi)*xbar + psi*v(:,end);
x(:) = xcc; fxcc = funfcn(x,varargin{:});
func_evals = func_evals+1;

if fxcc < fv(:,end)
v(:,end) = xcc;
fv(:,end) = fxcc;
how = 'contract inside';
else
% perform a shrink
how = 'shrink';
end
end
if strcmp(how,'shrink')
for j=two2np1
v(:,j)=v(:,1)+sigma*(v(:,j) - v(:,1));
x(:) = v(:,j); fv(:,j) = funfcn(x,varargin{:});
end
func_evals = func_evals + n;
end
end
end
[fv,j] = sort(fv);
v = v(:,j);
itercount = itercount + 1;
if prnt == 3
fprintf(' %5.0f %5.0f %12.6g %s\n', itercount, func_evals, fv(1), how)
elseif prnt == 4
disp(' ')
disp(how)
disp('v = ')
disp(v)
disp('fv = ')
disp(fv)
disp('func_evals = ')
disp(func_evals)
end
% OutputFcn and PlotFcns call
if haveoutputfcn || haveplotfcn
[xOutputfcn, optimValues, stop] = callOutputAndPlotFcns(outputfcn,plotfcns,v(:,1),xOutputfcn,'iter',itercount, ...
func_evals, how, fv(:,1),varargin{:});
if stop % Stop per user request.
[x,fval,exitflag,output] = cleanUpInterrupt(xOutputfcn,optimValues);
if prnt > 0
disp(output.message)
end
return;
end
end
end % while

x(:) = v(:,1);
fval = fv(:,1);

output.iterations = itercount;
output.funcCount = func_evals;
output.algorithm = 'Nelder-Mead simplex direct search';

% OutputFcn and PlotFcns call
if haveoutputfcn || haveplotfcn
callOutputAndPlotFcns(outputfcn,plotfcns,x,xOutputfcn,'done',itercount, func_evals, how, fval, varargin{:});
end

if func_evals >= maxfun
msg = getString(message('MATLAB:optimfun:fminsearch:ExitingMaxFunctionEvals', sprintf('%f',fval)));
if prnt > 0
disp(' ')
disp(msg)
end
exitflag = 0;
elseif itercount >= maxiter
msg = getString(message('MATLAB:optimfun:fminsearch:ExitingMaxIterations', sprintf('%f',fval)));
if prnt > 0
disp(' ')
disp(msg)
end
exitflag = 0;
else
msg = ...
getString(message('MATLAB:optimfun:fminsearch:OptimizationTerminatedXSatisfiesCriteria', ...
sprintf('%e',tolx), sprintf('%e',tolf)));
if prnt > 1
disp(' ')
disp(msg)
end
exitflag = 1;
end

output.message = msg;

%--------------------------------------------------------------------------
function [xOutputfcn, optimValues, stop] = callOutputAndPlotFcns(outputfcn,plotfcns,x,xOutputfcn,state,iter,...
numf,how,f,varargin)
% CALLOUTPUTANDPLOTFCNS assigns values to the struct OptimValues and then calls the
% outputfcn/plotfcns.
%
% state - can have the values 'init','iter', or 'done'.

% For the 'done' state we do not check the value of 'stop' because the
% optimization is already done.
optimValues.iteration = iter;
optimValues.funccount = numf;
optimValues.fval = f;
optimValues.procedure = how;

xOutputfcn(:) = x; % Set x to have user expected size
stop = false;
state = char(state);
% Call output functions
if ~isempty(outputfcn)
switch state
case {'iter','init'}
stop = callAllOptimOutputFcns(outputfcn,xOutputfcn,optimValues,state,varargin{:}) || stop;
case 'done'
callAllOptimOutputFcns(outputfcn,xOutputfcn,optimValues,state,varargin{:});
end
end
% Call plot functions
if ~isempty(plotfcns)
switch state
case {'iter','init'}
stop = callAllOptimPlotFcns(plotfcns,xOutputfcn,optimValues,state,varargin{:}) || stop;
case 'done'
callAllOptimPlotFcns(plotfcns,xOutputfcn,optimValues,state,varargin{:});
end
end

%--------------------------------------------------------------------------
function [x,FVAL,EXITFLAG,OUTPUT] = cleanUpInterrupt(xOutputfcn,optimValues)
% CLEANUPINTERRUPT updates or sets all the output arguments of FMINBND when the optimization
% is interrupted.

% Call plot function driver to finalize the plot function figure window. If
% no plot functions have been specified or the plot function figure no
% longer exists, this call just returns.
callAllOptimPlotFcns('cleanuponstopsignal');

x = xOutputfcn;
FVAL = optimValues.fval;
EXITFLAG = -1;
OUTPUT.iterations = optimValues.iteration;
OUTPUT.funcCount = optimValues.funccount;
OUTPUT.algorithm = 'Nelder-Mead simplex direct search';
OUTPUT.message = getString(message('MATLAB:optimfun:fminsearch:OptimizationTerminatedPrematurelyByUser'));

%--------------------------------------------------------------------------
function f = checkfun(x,userfcn,varargin)
% CHECKFUN checks for complex or NaN results from userfcn.

f = userfcn(x,varargin{:});
% Note: we do not check for Inf as FMINSEARCH handles it naturally.
if isnan(f)
error('MATLAB:fminsearch:checkfun:NaNFval',...
getString(message('MATLAB:optimfun:fminsearch:checkfun:NaNFval', localChar( userfcn ))));
elseif ~isreal(f)
error('MATLAB:fminsearch:checkfun:ComplexFval',...
getString(message('MATLAB:optimfun:fminsearch:checkfun:ComplexFval', localChar( userfcn ))));
end

%--------------------------------------------------------------------------
function strfcn = localChar(fcn)
% Convert the fcn to a character array for printing

if ischar(fcn)
strfcn = fcn;
elseif isstring(fcn) || isa(fcn,'inline')
strfcn = char(fcn);
elseif isa(fcn,'function_handle')
strfcn = func2str(fcn);
else
try
strfcn = char(fcn);
catch
strfcn = getString(message('MATLAB:optimfun:fminsearch:NameNotPrintable'));
end
end

3.运行结果