问题链接 AcWing 106. 动态中位数

问题描述

分析
推荐b站董晓算法视频讲解对顶堆
这道题应该用树状数组、平衡树也能解决，这里用对顶堆来做，对顶堆能够用维护第K位置的数，K是固定的，在这道题中，维护两个堆，一个大根堆一个小根堆

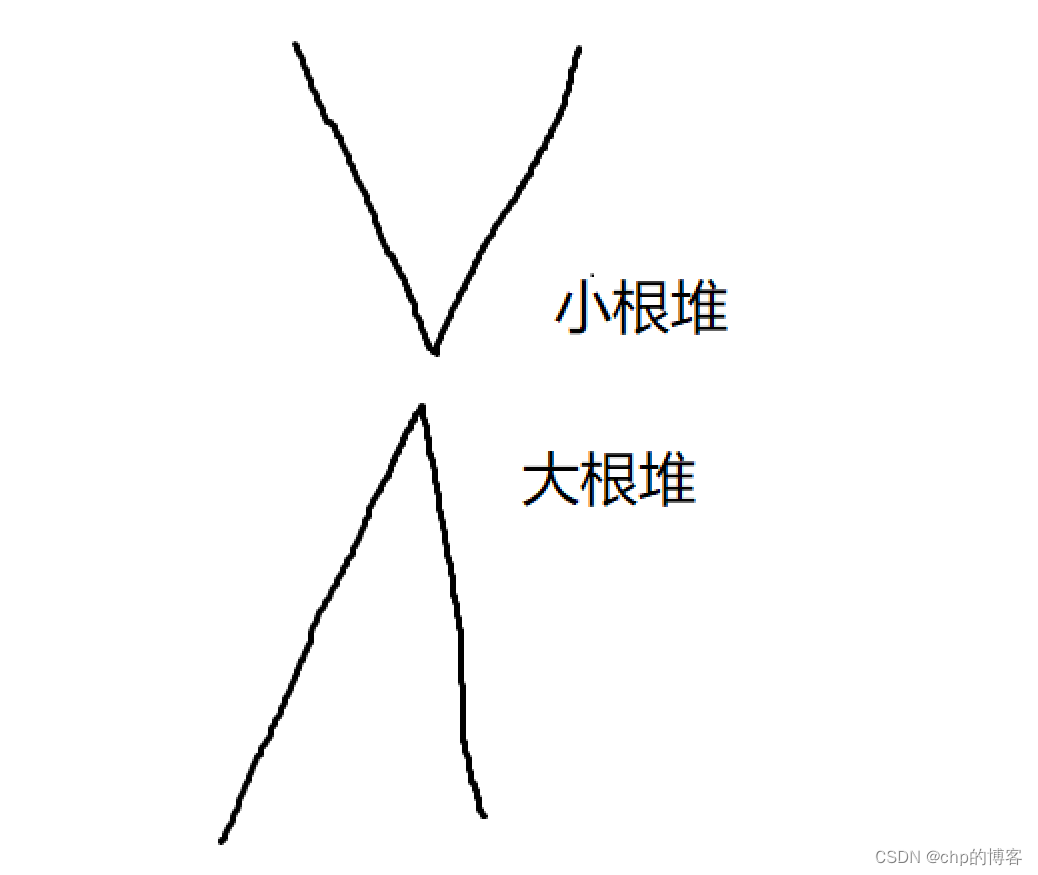
stl 中有堆，不用自己写，假设a为小根堆，b为大根堆

1.大小关系:
让小根堆中存的元素最小值，大于大根堆元素的最大值，也就是
a.top()>=b.top()

2.数量关系
除了维护大小关系也要维护两个堆内元素的数量关系
b.size()最多比a.size()多1
a.size()最多和b.size()相等
也就是只有两种情况
b.size()-a.size()=1;
b.size()-a.size()=0;

维护好这两堆之后，那么b.top()就是当前序列的中位数

代码如下

#include<cstdio>
#include<iostream>
#include<algorithm>
#include<cstring>
#include<queue>
using namespace std;

int main(){
    int p;
    cin>>p;
    while(p--){
        int k,m;
        cin>>k>>m;
        cout<<k<<" "<<(m+1)/2<<endl;
        priority_queue<int,vector<int>,greater<int> > a;//小根堆
        priority_queue<int,vector<int>,less<int> > b;//大根堆
        for(int i=1;i<=m;i++){
            int x;
            scanf("%d",&x);
            if(!a.empty()&&a.top()<=x) a.push(x);
            else b.push(x);
            if(i%2==1&&b.size()<a.size()){
                b.push(a.top());
                a.pop();
            }
            if(i%2==0&&a.size()<b.size()){
                a.push(b.top());
                b.pop();
            }
            if(i&1) printf("%d ",b.top());
            if(i%20==0) cout<<endl;
        }
        cout<<endl;
    }
    return 0;
}