【题目来源】
https://www.luogu.com.cn/problem/P5018
https://www.acwing.com/problem/content/478/
【题目描述】
一棵有点权的有根树如果满足以下条件,则被轩轩称为对称二叉树:
1.二叉树;
2.将这棵树所有节点的左右子树交换,新树和原树对应位置的结构相同且点权相等。
下图中节点内的数字为权值,节点外的 id 表示节点编号。

备注:图片来源于洛谷 https://www.luogu.com.cn/problem/P5018
现在给出一棵二叉树,希望你找出它的一棵子树,该子树为对称二叉树,且节点数最多。请输出这棵子树的节点数。
注意:只有树根的树也是对称二叉树。本题中约定,以节点 T 为子树根的一棵“子树”指的是:节点T和它的全部后代节点构成的二叉树。
【输入格式】
第一行一个正整数 n,表示给定的树的节点的数目,规定节点编号1∼n,其中节点 1 是树根。
第二行 n 个正整数,用一个空格分隔,第 i 个正整数 vi 代表节点 i 的权值。
接下来 n 行,每行两个正整数 li,ri,分别表示节点 i 的左右孩子的编号。如果不存在左/右孩子,则以 −1 表示。两个数之间用一个空格隔开。
【输出格式】
输出文件共一行,包含一个整数,表示给定的树的最大对称二叉子树的节点数。
【数据范围】
vi≤1000,n≤10^6
【输入输出样例】
input:
10
2 2 5 5 5 5 4 4 2 3
9 10
-1 -1
-1 -1
-1 -1
-1 -1
-1 2
3 4
5 6
-1 -1
7 8
output:
3【样例解析】
根据上述样例所得的二叉树如下所示。可知最大的对称二叉子树为以节点 7 为树根的子树,节点数为 3。

备注:图片来源于洛谷 https://www.luogu.com.cn/problem/P5018
【算法代码】
#include<bits/stdc++.h>
using namespace std;
const int maxn=1e6+5;
int v[maxn],lid[maxn],rid[maxn];
int ans;
bool flag=0;
int getN(int x,int y) {
if(x==-1 && y==-1) return 0;
if(x==-1 || y==-1 || v[x]!=v[y]) {
flag=1;
return 0;
}
return getN(lid[x],rid[y])+getN(rid[x],lid[y])+2;
}
int main() {
int n;
cin>>n;
for(int i=1; i<=n; i++) cin>>v[i];
for(int i=1; i<=n; i++) cin>>lid[i]>>rid[i];
for(int i=1; i<=n; i++) {
flag=false;
int sum=getN(lid[i],rid[i])+1;
if(!flag) ans=max(ans,sum);
}
cout<<ans;
return 0;
}
/*
in:
10
2 2 5 5 5 5 4 4 2 3
9 10
-1 -1
-1 -1
-1 -1
-1 -1
-1 2
3 4
5 6
-1 -1
7 8
out:
3
*/
【参考文献】
https://blog.csdn.net/qq_41431457/article/details/89339153
https://www.acwing.com/solution/content/133039/
https://www.acwing.com/solution/content/20417/
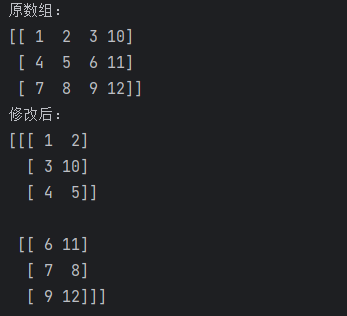






![vue路由传递对象数组,打印数据 [object Object] 解决方法](https://img-blog.csdnimg.cn/d61f48ac605e43ff957c8e0a7976a805.png#pic_center)