题目：

102. 二叉树的层序遍历

给你二叉树的根节点 root ，返回其节点值的 层序遍历 。（即逐层地，从左到右访问所有节点）。

示例 1：

输入：root = [3,9,20,null,null,15,7]
输出：[[3],[9,20],[15,7]]

示例 2：

输入：root = [1]
输出：[[1]]

示例 3：

输入：root = []
输出：[]

提示：

树中节点数目在范围 [0, 2000] 内
-1000 <= Node.val <= 1000

题解：

class Solution {
public:
    vector<vector<int>> levelOrder(TreeNode* root) {
        vector <vector <int>> ret;
        if (!root) {
            return ret;
        }

        queue <TreeNode*> q;
        q.push(root);
        while (!q.empty()) {
            int currentLevelSize = q.size();
            ret.push_back(vector <int> ());
            for (int i = 1; i <= currentLevelSize; ++i) {
                auto node = q.front(); q.pop();
                ret.back().push_back(node->val);
                if (node->left) q.push(node->left);
                if (node->right) q.push(node->right);
            }
        }
        
        return ret;
    }
};

题目：

226. 翻转二叉树

给你一棵二叉树的根节点 root ，翻转这棵二叉树，并返回其根节点。

示例 1：

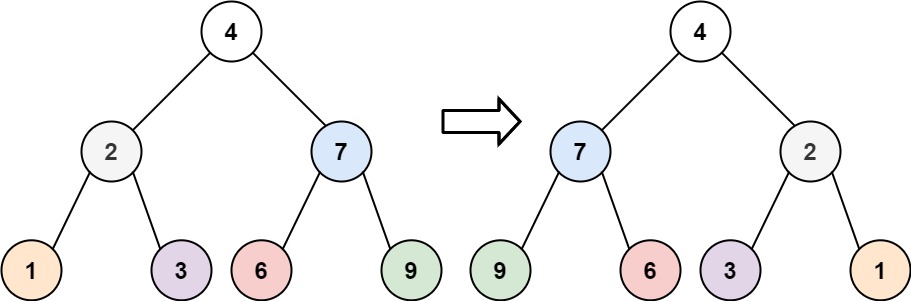
输入：root = [4,2,7,1,3,6,9]
输出：[4,7,2,9,6,3,1]

示例 2：

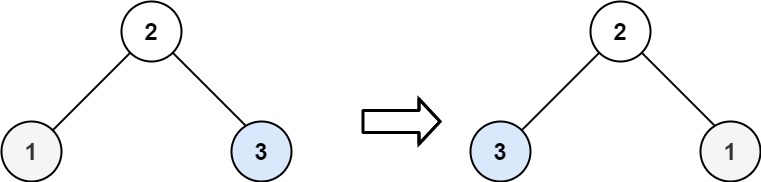
输入：root = [2,1,3]
输出：[2,3,1]

示例 3：

输入：root = []
输出：[]

提示：

树中节点数目范围在 [0, 100] 内
-100 <= Node.val <= 100

题解：

class Solution {
public:
    TreeNode* invertTree(TreeNode* root) {
        if (root == NULL) return root;
        swap(root->left, root->right);  // 中
        invertTree(root->left);         // 左
        invertTree(root->right);        // 右
        return root;
    }
};

题目：

101. 对称二叉树

给你一个二叉树的根节点 root ，检查它是否轴对称。

示例 1：

输入：root = [1,2,2,3,4,4,3]
输出：true

示例 2：

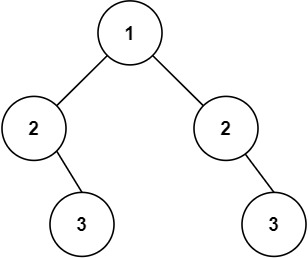
输入：root = [1,2,2,null,3,null,3]
输出：false

提示：

树中节点数目在范围 [1, 1000] 内
-100 <= Node.val <= 100

题解：

class Solution {
public:
    bool compare(TreeNode* left, TreeNode* right) {
        // 首先排除空节点的情况
        if (left == NULL && right != NULL) return false;
        else if (left != NULL && right == NULL) return false;
        else if (left == NULL && right == NULL) return true;
        // 排除了空节点，再排除数值不相同的情况
        else if (left->val != right->val) return false;

        // 此时就是：左右节点都不为空，且数值相同的情况
        // 此时才做递归，做下一层的判断
        bool outside = compare(left->left, right->right);   // 左子树：左、 右子树：右
        bool inside = compare(left->right, right->left);    // 左子树：右、 右子树：左
        bool isSame = outside && inside;                    // 左子树：中、 右子树：中 （逻辑处理）
        return isSame;

    }
    bool isSymmetric(TreeNode* root) {
        if (root == NULL) return true;
        return compare(root->left, root->right);
    }
};

欢迎点赞，收藏，评论，你的鼓励就是我创作的最大动力！(๑╹◡╹)ﾉ"""