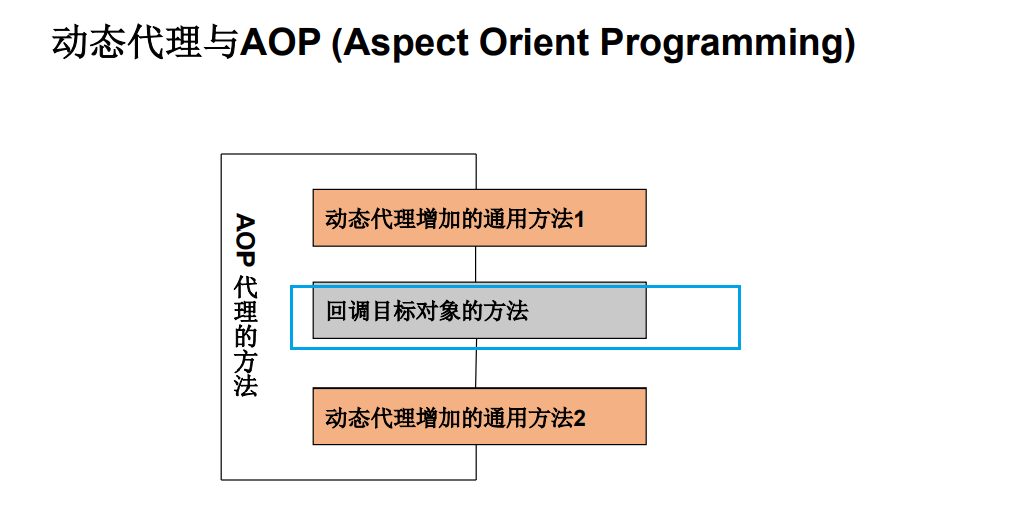
文章目录
- 1 平面图形的面积
- 1.1 直角坐标情形
- 1.2 极坐标情形
- 1.2.1 极坐标的定义
- 1.1.2 曲边扇形的面积
- 结语
1 平面图形的面积
1.1 直角坐标情形
①平面图形由 y = f ( x ) , y = 0 , x = a , y = b y=f(x),y=0,x=a,y=b y=f(x),y=0,x=a,y=b围成图像的面积,如下图1.1-1所示:

s = ∫ a b f ( x ) d x s=\int_a^bf(x)dx s=∫abf(x)dx s = ∫ a b ∣ f ( x ) ∣ d x s=\int_a^b|f(x)|dx s=∫ab∣f(x)∣dx s = ∫ a b ∣ f ( x ) ∣ d x s=\int_a^b|f(x)|dx s=∫ab∣f(x)∣dx
综上面积 s = ∫ a b ∣ f ( x ) ∣ d x s=\int_a^b|f(x)|dx s=∫ab∣f(x)∣dx
②平面图形由 y = f ( x ) , y = g ( x ) , x = a , x = b y=f(x),y=g(x),x=a,x=b y=f(x),y=g(x),x=a,x=b所围成图像的面积,如下图1.1-2所示:

s = ∫ a b [ f ( x ) − g ( x ) ] d x s=\int_a^b[f(x)-g(x)]dx s=∫ab[f(x)−g(x)]dx s = ∫ a b ∣ f ( x ) − g ( x ) ∣ d x s=\int_a^b|f(x)-g(x)|dx s=∫ab∣f(x)−g(x)∣dx s = ∫ a b ∣ f ( x ) − g ( x ) ∣ d x s=\int_a^b|f(x)-g(x)|dx s=∫ab∣f(x)−g(x)∣dx
综上面积 s = ∫ a b ∣ f ( x ) − g ( x ) ∣ d x s=\int_a^b|f(x)-g(x)|dx s=∫ab∣f(x)−g(x)∣dx
③平面图形由 x = f ( y ) , x = 0 , y = a , y = b x=f(y),x=0,y=a,y=b x=f(y),x=0,y=a,y=b围成图形面积,如下图1.1-3所示:

面积 s = ∫ a b ∣ f ( y ) ∣ d y s=\int_a^b|f(y)|dy s=∫ab∣f(y)∣dy
④平面图形由 x = f ( y ) , x = g ( y ) , y = a , y = b x=f(y),x=g(y),y=a,y=b x=f(y),x=g(y),y=a,y=b所围成图像面积,如下图1.1-4所示:

面积 s = ∫ a b ∣ f ( y ) − g ( y ) ∣ d y s=\int_a^b|f(y)-g(y)|dy s=∫ab∣f(y)−g(y)∣dy
例1 求区间 [ 1 2 , 2 ] [\frac{1}{2},2] [21,2]上连续曲线 y = ln x , x 轴及直线 x = 1 2 , x = 2 y=\ln x,x轴及直线x=\frac{1}{2},x=2 y=lnx,x轴及直线x=21,x=2所围图像的面积,如下图1-1所示:

解:
d
S
=
ln
x
d
x
,
x
∈
[
1
2
,
2
]
S
=
∫
1
2
2
d
S
=
∫
1
2
2
ln
x
d
x
=
−
∫
1
2
1
l
n
x
d
x
+
∫
1
2
ln
x
d
x
=
−
(
x
ln
x
−
x
)
∣
1
2
1
+
(
x
ln
x
−
x
)
1
2
=
3
2
ln
2
−
1
2
解:dS=\ln xdx,x\in[\frac{1}{2},2]\\ S=\int_\frac{1}{2}^2dS=\int_\frac{1}{2}^2\ln xdx=-\int_\frac{1}{2}^1ln xdx+\int_1^2\ln xdx\\ =-(x\ln x-x)|_\frac{1}{2}^1+(x\ln x-x)_1^2=\frac{3}{2}\ln2-\frac{1}{2}
解:dS=lnxdx,x∈[21,2]S=∫212dS=∫212lnxdx=−∫211lnxdx+∫12lnxdx=−(xlnx−x)∣211+(xlnx−x)12=23ln2−21
例2 求
y
2
=
x
,
y
=
x
2
y^2=x,y=x^2
y2=x,y=x2所围图像的面积,如下图2-1所示:

解:解方程组
{
y
2
=
x
y
=
x
2
,交点为
(
0
,
0
)
,
(
1
,
1
)
取
x
为积分变量,积分区间
[
0
,
1
]
S
=
∫
0
1
(
x
−
x
2
)
d
x
=
2
3
x
3
2
∣
0
1
−
1
3
x
3
∣
0
1
=
1
3
解:解方程组 \begin{cases} y^2=x\\ y=x^2\\ \end{cases},交点为(0,0),(1,1)\\ 取x为积分变量,积分区间[0,1]\\ S=\int_0^1(\sqrt{x}-x^2)dx=\frac{2}{3}x^\frac{3}{2}|_0^1-\frac{1}{3}x^3|_0^1=\frac{1}{3}
解:解方程组{y2=xy=x2,交点为(0,0),(1,1)取x为积分变量,积分区间[0,1]S=∫01(x−x2)dx=32x23∣01−31x3∣01=31
例3 求
y
2
−
2
x
,
y
=
x
0
−
4
y^2-2x,y=x0-4
y2−2x,y=x0−4围成图像的面积,如下图3-1所示:

解:方程组 { y = x − 4 , y 2 = 2 x 交点为 ( 2 , − 2 ) , ( 8 , 4 ) 取 y 为积分变量,则 S = ∫ − 2 4 ( y + 4 − y 2 2 ) d y = ( 1 2 y 2 + 4 y − 1 6 y 3 ) ∣ − 2 4 = 18 解:方程组 \begin{cases} y=x-4,\\ y^2=2x \end{cases} 交点为(2,-2),(8,4)\\ 取y为积分变量,则\\ S=\int_{-2}^4(y+4-\frac{y^2}{2})dy=(\frac{1}{2}y^2+4y-\frac{1}{6}y^3)|_{-2}^4\\ =18 解:方程组{y=x−4,y2=2x交点为(2,−2),(8,4)取y为积分变量,则S=∫−24(y+4−2y2)dy=(21y2+4y−61y3)∣−24=18
例4 求由曲线 y = x 2 , y = x 2 4 及 y = 1 y=x^2,y=\frac{x^2}{4}及y=1 y=x2,y=4x2及y=1所围成图像的面积,如下图4-1所示:

解:由上图知,所围图像左右对称 取 y 为积分变量,则 S = 2 ∫ 0 1 ( 2 y − y ) = 4 3 y 3 2 ∣ 0 1 = 4 3 解:由上图知,所围图像左右对称\\ 取y为积分变量,则\\ S=2\int_0^1(2\sqrt{y}-\sqrt{y})=\frac{4}{3}y^\frac{3}{2}|_0^1=\frac{4}{3} 解:由上图知,所围图像左右对称取y为积分变量,则S=2∫01(2y−y)=34y23∣01=34
例5 求椭圆 x 2 a 2 + y 2 b 2 = 1 \frac{x^2}{a^2}+\frac{y^2}{b^2}=1 a2x2+b2y2=1的面积,如下图5-1所示:

解: { x = a cos t , y = b sin t S = 4 ∫ 0 a y d x = 4 ∫ π 2 0 sin t d ( a cos t ) = 4 a b ∫ 0 π 2 sin 2 t d t = 4 a b ⋅ 1 2 ⋅ π 2 = π a b 解:\begin{cases} x=a\cos t,\\ y=b\sin t \end{cases}\\ S=4\int_0^aydx=4\int_\frac{\pi}{2}^0\sin td(a\cos t)=4ab\int_0^\frac{\pi}{2}\sin^2tdt\\ =4ab\cdot\frac{1}{2}\cdot\frac{\pi}{2}=\pi ab 解:{x=acost,y=bsintS=4∫0aydx=4∫2π0sintd(acost)=4ab∫02πsin2tdt=4ab⋅21⋅2π=πab
1.2 极坐标情形
1.2.1 极坐标的定义
为了表示平面上的点,在平面上取定一点 o o o,称为极点。取定一条轴 o x ox ox,称为极轴。这样在平面上建了一个坐标系,称为极坐标系。

如上图1.2.1-1所示,平面上任意一点p,设KaTeX parse error: Undefined control sequence: \ang at position 11: |op|=\rho,\̲a̲n̲g̲ ̲xop=\theta,则数对 ( ρ , θ ) (\rho,\theta) (ρ,θ)为点p的极坐标。
极坐标与平面坐标变换公式:(原点重合)
{
x
=
ρ
cos
θ
,
y
=
ρ
sin
θ
{
ρ
=
x
2
+
y
2
,
θ
=
arctan
y
x
\begin{cases} x=\rho \cos\theta,\\ y=\rho\sin\theta \end{cases}\\ \qquad \begin{cases} \rho=\sqrt{x^2+y^2},\\ \theta=\arctan\frac{y}{x} \end{cases}\\
{x=ρcosθ,y=ρsinθ{ρ=x2+y2,θ=arctanxy
注:规定
(
−
ρ
,
θ
)
=
(
ρ
,
θ
+
π
)
(-\rho,\theta)=(\rho,\theta+\pi)
(−ρ,θ)=(ρ,θ+π)
1.1.2 曲边扇形的面积

①曲边扇形:由
ρ
=
ρ
(
θ
)
,
θ
=
α
,
θ
=
β
\rho=\rho(\theta),\theta=\alpha,\theta=\beta
ρ=ρ(θ),θ=α,θ=β围成的面积
d
s
=
π
ρ
2
(
θ
)
⋅
d
θ
2
π
=
1
2
ρ
2
(
θ
)
d
θ
S
=
∫
α
β
d
s
=
1
2
∫
α
β
ρ
2
(
θ
)
d
θ
ds=\pi\rho^2(\theta)\cdot\frac{d\theta}{2\pi}=\frac{1}{2}\rho^2(\theta)d\theta\\ S=\int_\alpha^\beta ds=\frac{1}{2}\int_\alpha^\beta\rho^2(\theta)d\theta
ds=πρ2(θ)⋅2πdθ=21ρ2(θ)dθS=∫αβds=21∫αβρ2(θ)dθ
②极点在曲线
ρ
=
ρ
(
θ
)
内部
\rho=\rho(\theta)内部
ρ=ρ(θ)内部,所围成图像米面积
S = 1 2 ∫ 0 2 π ρ 2 ( θ ) d θ S=\frac{1}{2}\int_0^{2\pi}\rho^2(\theta)d\theta S=21∫02πρ2(θ)dθ
③曲边扇形由 ρ = r h o 1 ( θ ) , ρ = ρ 2 ( θ ) , θ = α , θ = β \rho=rho_1(\theta),\rho=\rho_2(\theta),\theta=\alpha,\theta=\beta ρ=rho1(θ),ρ=ρ2(θ),θ=α,θ=β所围图形,如下图1.1.2-1所示:

S
=
1
2
∫
α
β
∣
ρ
1
2
(
θ
)
−
ρ
2
2
(
θ
)
∣
d
θ
S=\frac{1}{2}\int_\alpha^\beta|\rho_1^2(\theta)-\rho_2^2(\theta)|d\theta
S=21∫αβ∣ρ12(θ)−ρ22(θ)∣dθ
例1 计算阿基米德螺线
ρ
=
a
θ
(
a
>
0
)
相应于
θ
从
0
到
2
π
\rho=a\theta(a\gt0)相应于\theta从0到2\pi
ρ=aθ(a>0)相应于θ从0到2π的弧段与极轴围成图像的面积,如下图1-1所示:

解:
S
=
1
2
∫
0
2
π
ρ
2
(
θ
)
d
θ
=
a
2
2
∫
0
2
π
θ
2
d
θ
=
4
a
2
3
π
3
解:S=\frac{1}{2}\int_0^{2\pi}\rho^2(\theta)d\theta=\frac{a^2}{2}\int_0^{2\pi}\theta^2d\theta\\ =\frac{4a^2}{3}\pi^3
解:S=21∫02πρ2(θ)dθ=2a2∫02πθ2dθ=34a2π3
例2 计算心形线
ρ
=
a
(
1
+
cos
θ
)
(
a
>
0
)
\rho=a(1+\cos\theta)(a\gt0)
ρ=a(1+cosθ)(a>0)所围成的图像的面积,如下图2-1所示:

解:图形关于极轴对称
,
积分变量
θ
,
区间
[
0
,
2
π
]
,
则
S
=
1
2
∫
0
2
π
ρ
2
(
θ
)
d
θ
=
a
2
2
∫
0
2
π
(
1
+
cos
θ
)
2
d
θ
=
a
2
2
∫
0
2
π
(
1
+
2
cos
θ
+
cos
2
θ
)
d
θ
=
π
a
2
+
0
+
2
a
2
∫
0
π
2
cos
2
θ
d
θ
=
3
a
2
2
π
解:图形关于极轴对称,积分变量\theta,区间[0,2\pi],则\\ S=\frac{1}{2}\int_0^{2\pi}\rho^2(\theta)d\theta=\frac{a^2}{2}\int_0^{2\pi}(1+\cos\theta)^2d\theta\\ =\frac{a^2}{2}\int_0^{2\pi}(1+2\cos\theta+\cos^2\theta)d\theta\\ =\pi a^2+0+2a^2\int_0^\frac{\pi}{2}\cos^2\theta d\theta=\frac{3a^2}{2}\pi
解:图形关于极轴对称,积分变量θ,区间[0,2π],则S=21∫02πρ2(θ)dθ=2a2∫02π(1+cosθ)2dθ=2a2∫02π(1+2cosθ+cos2θ)dθ=πa2+0+2a2∫02πcos2θdθ=23a2π
例3 求
ρ
=
2
a
cos
θ
(
a
>
0
)
\rho=2a\cos\theta(a\gt0)
ρ=2acosθ(a>0)围成图形的面积,如下图3-1所示:

解 : 积分变量 θ , 区间 [ 0 , 2 π ] , 则 S = 1 2 ∫ − π 2 π 2 ρ 2 ( θ ) d θ = 4 a 2 ∫ 0 π 2 cos 2 θ d θ = π a 2 解:积分变量\theta,区间[0,2\pi],则\\ S=\frac{1}{2}\int_{-\frac{\pi}{2}}^\frac{\pi}{2}\rho^2(\theta)d\theta=4a^2\int_0^\frac{\pi}{2}\cos^2\theta d\theta\\ =\pi a^2 解:积分变量θ,区间[0,2π],则S=21∫−2π2πρ2(θ)dθ=4a2∫02πcos2θdθ=πa2
注意:
- 给出曲线,但不知道夹角范围,需要自己去化简。
- 熟悉数中附录三种常见曲线。
结语
❓QQ:806797785
⭐️文档笔记地址:https://gitee.com/gaogzhen/math
参考:
[1]同济大学数学系.高等数学 第七版 上册[M].北京:高等教育出版社,2014.7.p276-286.
[2]同济七版《高等数学》全程教学视频[CP/OL].2020-04-16.p39.