669. 修剪二叉搜索树
给你二叉搜索树的根节点 root ,同时给定最小边界low 和最大边界 high。通过修剪二叉搜索树,使得所有节点的值在[low, high]中。修剪树 不应该 改变保留在树中的元素的相对结构 (即,如果没有被移除,原有的父代子代关系都应当保留)。 可以证明,存在 唯一的答案 。
所以结果应当返回修剪好的二叉搜索树的新的根节点。注意,根节点可能会根据给定的边界发生改变。
示例 1:
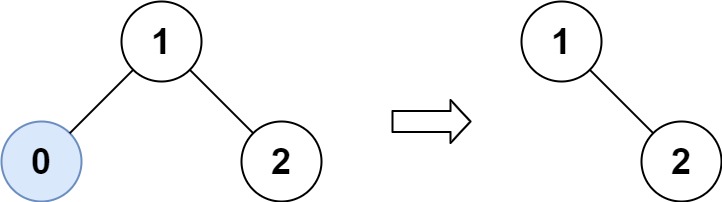
输入:root = [1,0,2], low = 1, high = 2 输出:[1,null,2]
示例 2:

输入:root = [3,0,4,null,2,null,null,1], low = 1, high = 3 输出:[3,2,null,1]
思路
个人AC代码
递归三步曲:
返回值为经过修剪的子树的根节点, 参数为待修剪子树的根节点
终止条件:修剪完成即为终止
单次递归的操作:感觉本题较之前的题目有些区别.单次递归的操作都放在了终止条件中
class Solution {
public TreeNode trimBST(TreeNode root, int low, int high) {
if(root == null) return root;
//叶子节点
if(root.left == null && root.right == null){
//应该删除
if(root.val < low || root.val > high){
return null;
}else{
//不该删除
return root;
}
}
//非叶子 不该删除
if(root.val >= low && root.val <= high){
root.left = trimBST(root.left, low, high);
root.right = trimBST(root.right, low, high);
return root;
}else{
//非叶子 该删除
//注意返回值的含义,返回当前子树修剪后的根节点
if(root.left == null){
root.right = trimBST(root.right, low, high);
return root.right;
}
if(root.right == null){
root.left = trimBST(root.left, low, high);
return root.left;
}
//左右均不空, 左子树放在右子树最左下 或 右子树 放在左子树 最右下
//记得首先先修剪
root.left = trimBST(root.left, low, high);
root.right = trimBST(root.right, low, high);
//修剪后判断
if(root.left != null && root.right != null) {
TreeNode cur = root.left;
while (cur.right != null) {
cur = cur.right;
}
cur.right = root.right;
return root.left;
}else if(root.left == null){
return root.right;
}else if(root.right == null){
return root.left;
}else{
return null;
}
}
}
}看完题解后:
递归三部曲:
返回值:经过修剪的子树的根节点, 参数为待修剪子树的根节点;
终止条件:因为修剪的操作并不是在终止条件上进行的,所以就是遇到空节点返回就可以了。
但上述代码中的修剪操作就是放在了终止条件中,根据不同的终止条件进行不同处理,这也是为什么如此繁琐.
单次递归的操作:
如果root(当前节点)的元素小于low的数值,那么应该递归右子树,并返回右子树符合条件的头结点。
如果root(当前节点)的元素大于high的,那么应该递归左子树,并返回左子树符合条件的头结点。
接下来将下一层处理完左子树的结果赋给root->left,处理完右子树的结果赋给root->right。
最后返回root节点.
题解代码
class Solution {
public TreeNode trimBST(TreeNode root, int low, int high) {
if(root == null) return root;
if(root.val < low){
root.right = trimBST(root.right, low, high);
return root.right;
}
if(root.val > high){
root.left = trimBST(root.left, low, high);
return root.left;
}
//root值在范围内
root.left = trimBST(root.left, low, high);
root.right = trimBST(root.right, low, high);
return root;
}
}总结
主要的区别在于三步曲的差别. 上述思路的代码更加简练.因为它把握了二叉搜索树的性质,
在非叶子 该删除的情况下,不需要考虑左/右子树是否为null 而应该注意到 root的值(大于high / 小于low) 对应于剩余左/右子树的节点的修剪即可. 个人代码实际是对于非搜索树适用的.
所以关键在于对二叉搜索树性质的利用.
108.将有序数组转换为二叉搜索树
给你一个整数数组 nums ,其中元素已经按 升序 排列,请你将其转换为一棵 平衡 二叉搜索树。
示例 1:

输入:nums = [-10,-3,0,5,9] 输出:[0,-3,9,-10,null,5] 解释:[0,-10,5,null,-3,null,9] 也将被视为正确答案:

示例 2:

输入:nums = [1,3] 输出:[3,1] 解释:[1,null,3] 和 [3,1] 都是高度平衡二叉搜索树。
思路
数组构造二叉树 本质就是寻找分割点,分割点作为当前节点,然后递归左区间和右区间。
分割点就是数组中间位置的节点。
那么问题来了,如果数组长度为偶数,中间节点有两个,取哪一个?
取哪一个都可以,只不过构成了不同的平衡二叉搜索树。
代码
class Solution {
public TreeNode sortedArrayToBST(int[] nums) {
TreeNode root = makeBST(nums, 0, nums.length-1);
return root;
}
public TreeNode makeBST(int [] nums, int begin, int end){
if(begin > end) return null;
int len = end - begin;
int index = len / 2 + begin;
TreeNode root = new TreeNode(nums[index]);
root.left = makeBST(nums, begin, index - 1);
root.right = makeBST(nums, index + 1, end);
return root;
}
}538.把二叉搜索树转换为累加树
给出二叉 搜索 树的根节点,该树的节点值各不相同,请你将其转换为累加树(Greater Sum Tree),使每个节点 node 的新值等于原树中大于或等于 node.val 的值之和。
提醒一下,二叉搜索树满足下列约束条件:
- 节点的左子树仅包含键 小于 节点键的节点。
- 节点的右子树仅包含键 大于 节点键的节点。
- 左右子树也必须是二叉搜索树。
注意:本题和 1038: . - 力扣(LeetCode) 相同
示例 1:
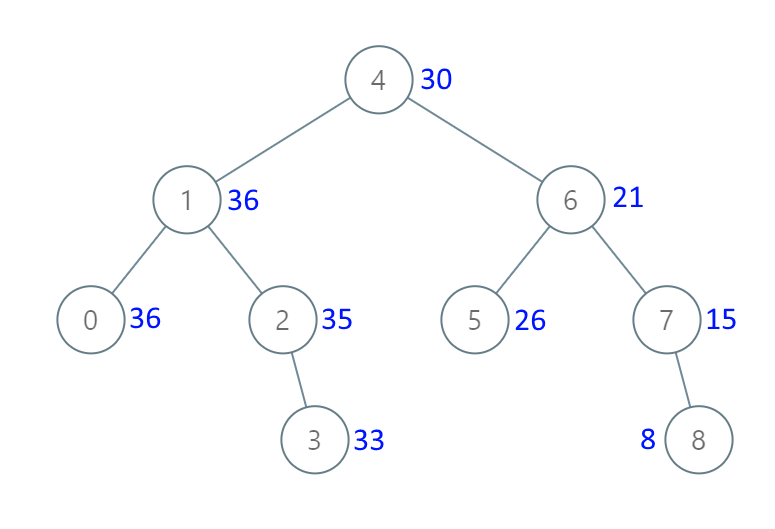
输入:[4,1,6,0,2,5,7,null,null,null,3,null,null,null,8] 输出:[30,36,21,36,35,26,15,null,null,null,33,null,null,null,8]
思路
其实将二叉搜索树 看作一个有序数组 如 [1,2,3] 本题就很好写
结果为[6,5,3] 就是从后往前累加
那么 对于二叉树而言
递归: 反中序遍历 因为 右 中 左
注意pre指针的使用, 记录前一个节点.
代码
class Solution {
TreeNode pre = null;
public TreeNode convertBST(TreeNode root) {
add(root);
return root;
}
public void add(TreeNode root){
if(root == null) return ;
add(root.right);
if(pre != null){
root.val += pre.val;
}
pre = root;
add(root.left);
}
}总结篇
代码随想录 (programmercarl.com)







![[leetcode] 46. 全排列](https://img-blog.csdnimg.cn/direct/a36c1b59f2eb48eebd27dc7ba26116ca.png)