LCS

Largest Common Subsequence 最大公共子序列

/*
Input
s1 s2//两个字符串

Output
length//长度
ans//具体字母
*/
#include<iostream>
using namespace std;
int main()
{
    string s1,s2;
    cin>>s1>>s2;
    int dp[100][100]={0};
    //dp[i][j]表示s1取前i位，s2取前j位时的最大公共长度
    int n=s1.size(),m=s2.size();
    s1=" "+s1;
    s2=" "+s2;//使范围从[0,n-1]变为[1,n]
    for(int i=1;i<=n;i++)
    {
        for(int j=1;j<=m;j++)
        {
            if(s1[i]==s2[j]){
                dp[i][j]=dp[i-1][j-1]+1;
            }else{
                dp[i][j]=max(dp[i-1][j],dp[i][j-1]);
            }
        }
    }
    cout<<dp[n][m]<<endl;//最大公共长度 完成
    
    //以下为输出具体方案 公共部分
    string ans;
    while(n>=1&&m>=1){
        if(s1[n]==s2[m]) ans=s1[n]+ans,n--,m--;//条件为两个字母相等，同时n,m也需要更新
        else if(dp[n][m-1]>dp[n-1][m]) m--;
        else n--;
    }
    cout<<ans<<endl;
}

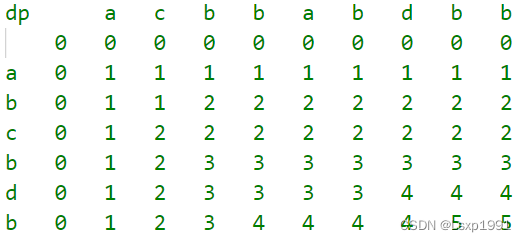
dp数组的变化如图：

LIS

Largest Increasing Sunsequence 最大递增子序列

/*
Input
n
a1 a2 a3...an//n个数

Output 
ans //长度
*/

#include<iostream>
using namespace std;
int main()
{
    int n;cin>>n;
    int a[n+2];
    int dp[n+2]={0};//dp[i]表示前i个数最大上升序列长度
    for(int i=1;i<=n;i++) cin>>a[i];

    for(int i=1;i<=n;i++)
    {
        dp[i]=1;
        for (int j = 1; j <= i; j++)//搜索1~i范围
        {
            if (a[i] > a[j])
            dp[i] = max(dp[i], dp[j] + 1);
        }
    }
    int ans=0;
    for(int i=1;i<=n;i++)
    ans=max(ans,dp[i]);
    cout<<ans<<endl;
}