121. 买卖股票的最佳时机

121. 买卖股票的最佳时机 - 力扣（LeetCode）

思路

1. 确定dp数组及其下标含义

dp[i][0] 表示第i天持有股票所得最多现金

dp[i][1] 表示第i天不持有股票所得最多现金

2. 确定递推公式

dp[i][0] = max(dp[i - 1][0], -prices[i]);
dp[i][1] = max(dp[i - 1][1], prices[i] + dp[i - 1][0]);

3. dp数组初始化

dp[0][0]表示第0天持有股票，此时的持有股票就一定是买入股票了，因为不可能有前一天推出来，所以dp[0][0] -= prices[0];

dp[0][1]表示第0天不持有股票，不持有股票那么现金就是0，所以dp[0][1] = 0;

4. 确定遍历顺序

从递推公式可以看出dp[i]都是由dp[i - 1]推导出来的，那么一定是从前向后遍历。

5. 举例推导dp数组

// 版本一
class Solution {
public:
    int maxProfit(vector<int>& prices) {
        int len = prices.size();
        if (len == 0) return 0;
        vector<vector<int>> dp(len, vector<int>(2));
        dp[0][0] -= prices[0];
        dp[0][1] = 0;
        for (int i = 1; i < len; i++) {
            dp[i][0] = max(dp[i - 1][0], -prices[i]);
            dp[i][1] = max(dp[i - 1][1], prices[i] + dp[i - 1][0]);
        }
        return dp[len - 1][1];
    }
};

时间复杂度：O(n)
空间复杂度：O(n)

// 版本二
class Solution {
public:
    int maxProfit(vector<int>& prices) {
        int len = prices.size();
        vector<vector<int>> dp(2, vector<int>(2)); // 注意这里只开辟了一个2 * 2大小的二维数组
        dp[0][0] -= prices[0];
        dp[0][1] = 0;
        for (int i = 1; i < len; i++) {
            dp[i % 2][0] = max(dp[(i - 1) % 2][0], -prices[i]);
            dp[i % 2][1] = max(dp[(i - 1) % 2][1], prices[i] + dp[(i - 1) % 2][0]);
        }
        return dp[(len - 1) % 2][1];
    }
};

时间复杂度：O(n)
空间复杂度：O(1)

122.买卖股票的最佳时机II

122. 买卖股票的最佳时机 II - 力扣（LeetCode）

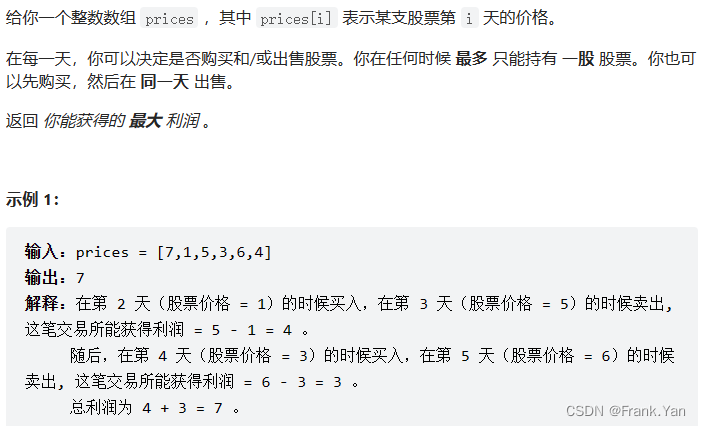
思路

本题首先要清楚两点：

只有一只股票！
当前只有买股票或者卖股票的操作

想获得利润至少要两天为一个交易单元。

// 贪心解法
class Solution {
public:
    int maxProfit(vector<int>& prices) {
        int result = 0;
        for (int i = 1; i < prices.size(); i++) {
            result += max(prices[i] - prices[i - 1], 0);
        }
        return result;
    }
};

时间复杂度：O(n)
空间复杂度：O(1)

// dp解法
class Solution {
public:
    int maxProfit(vector<int>& prices) {
        // dp[i][1]第i天持有的最多现金
        // dp[i][0]第i天持有股票后的最多现金
        int n = prices.size();
        vector<vector<int>> dp(n, vector<int>(2, 0));
        dp[0][0] -= prices[0]; // 持股票
        for (int i = 1; i < n; i++) {
            // 第i天持股票所剩最多现金 = max(第i-1天持股票所剩现金, 第i-1天持现金-买第i天的股票)
            dp[i][0] = max(dp[i - 1][0], dp[i - 1][1] - prices[i]);
            // 第i天持有最多现金 = max(第i-1天持有的最多现金，第i-1天持有股票的最多现金+第i天卖出股票)
            dp[i][1] = max(dp[i - 1][1], dp[i - 1][0] + prices[i]);
        }
        return max(dp[n - 1][0], dp[n - 1][1]);
    }
};