前言

一道好题，也就花了我一个下午而已。

本人做法比较清奇，可以当做开阔思路参考，并不太建议实操（太难调了！）。

文章较啰嗦，谅解。

思路

众所周知，我并不太喜推式子，所以我们考虑直接根据题目限定的条件硬刚。

首先，我们先假定 $1$ 为根，并求出每个点的子树的大小，记为数组 $size$，并且再维护一下每一个点的时间戳。

接着我们考虑一种特殊情况，即如果 $1$ 可以为树的重心的情况，那么他就会有接下来的两种子情况：

• 他所有的儿子的 $size$ 全部小于等于 $\frac{n}{2}$。

• 有一个儿子 $x$ 的 $size$ 大于 $\frac{n}{2}$，且这个儿子的子树上一定能找到一个点 $y$ 满足 $siz{e}_x-siz{e}_y\le \frac{n}{2},siz{e}_y\le \frac{n}{2}$。（把这个子树拆下来接到 $1$ 上就可以满足重心的条件）

第二种情况不是很理解的话可以看下面这张图：

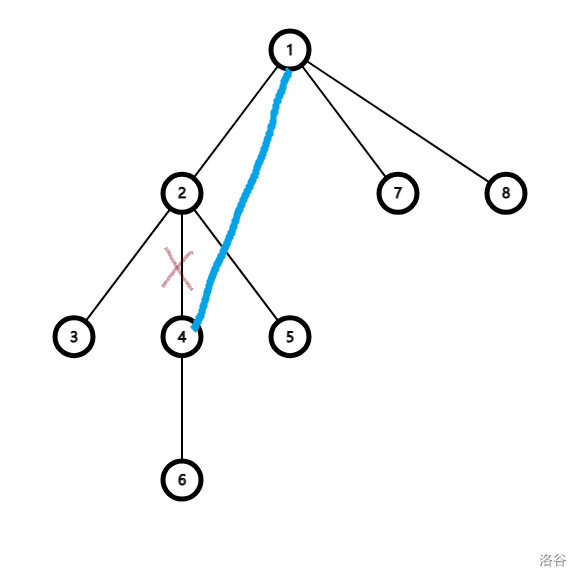
只有两种情况满足其一，那么 $1$ 就可以成为树的重心。

接下来，我们迁移到一般的情况，那么就需要考虑除了他子树以外的情况。

对于树上的任意节点 $x$，要让他成为树的重心，就会有接下来的三种情况。

• 他所有的儿子的 $size$ 全部小于等于 $\frac{n}{2}$，并且 $n-siz{e}_x\le \frac{n}{2}$。

• 同根节点的第二种情况。

• $n-siz{e}_x>\frac{n}{2}$，即删除 $x$ 后，不属于他子树的那一部分超过了重心的限制。那么在其中，我们又要分为两种情况。首先选择一个不在 $x$ 子树上的点 $y$。如果 $y$ 不在根节点到 $x$ 的那条链上，那么只需要满足 $siz{e}_y\le \frac{n}{2},n-siz{e}_x-siz{e}_y\le \frac{n}{2}$。否则，我们拆下来的，就是除了 $y$ 子树以外的部分,接到 $x$ 上面去（毕竟你不可以把 $x$ 的子树也拆下来，不然就会没有任何效果。），即满足条件 $siz{e}_y-siz{e}_z\le \frac{n}{2},n-siz{e}_y\le \frac{n}{2}$，只要找到一个符合条件的 $y$ 即可。

那么接下来，问题就转化为了 $x$ 能不能找到一个符合条件的 $y$。（毕竟第一个条件很好解决。）

接着继续转化问题，对于情况2，我们可以移一下项，将其转化为 $y$ 满足条件 $siz{e}_x-\frac{n}{2}\le siz{e}_y\le \frac{n}{2}$，且 $df{n}_x\le df{n}_y\le df{n}_x+siz{e}_x-1$。

对于情况三的第一种情况，我们也可以移一下项，转化为 $n-siz{e}_x-\frac{n}{2}\le siz{e}_y\le \frac{n}{2}$，且 $df{n}_y<df{n}_x,df{n}_y>df{n}_x+siz{e}_x-1$（对于不在链上这一限制，后面会说怎么处理）。

这两种情况都可以看做有四个常数 $p,q,l,r$，判断是否有对于 $x\in [p,q],y\in [l,r]$ 存在 $x=siz{e}_y$。

显然，我们可以对于 $size$ 分块，然后开两个 $set$ $a,b$，$a_x$ （为什么用set后面会解释。）里面放所有 $size=x$ 的所有下标，$b_x$ 放 $size$ 属于 $x$ 这个块的所有的下标。最后查询时，以 $size$ 为第一维，对于散块，在 $a$ 中二分，看有没有满足属于 $[l,r]$ 的值，对于整块，则在 $b$ 中二分。

接着，我们看向比较难处理的情况三的第二种情况。

我们可以动态维护一个数组，依次储存从根到该节点的链上的所有 $size$ 值，可以发现是单调递减。并且记得在加入数组时，删除他在 $a,b$ 中的值，在删除数组元素时，加回去。（因为必须要保证在情况三的情况1解决时要不包含这些在链上的元素）。这里的删除和加入就解释了为什么要采用 set。接着可以先在数组中二分找出满足 $n-siz{e}_y\le \frac{n}{2}$ 的 $y$ 的范围，然后在这个范围中继续二分找出是否存在满足 $siz{e}_y-siz{e}_z\le \frac{n}{2}$ 的 $y$，那么就解决了情况3的第二种情况。

时间复杂度，分块+二分，及 $n\sqrt{n}\log n$，但因为二分的区间长度不可能每次都是顶着的，所以远远达不到这个上界，但仍然很慢。

所以问题就 迎刃而解 了！

代码

#include<bits/stdc++.h>
using namespace std;
int n;
struct node
{
	int tar,nxt;
}arr[800005];
int fst[400005],cnt;
void adds(int x,int y)
{
	arr[++cnt].tar=y,arr[cnt].nxt=fst[x],fst[x]=cnt;
}
int size[400005],dfn[400005],tot,rnk[400005];
int klen,len;
set<int> a[400005],b[100005];
bool dp[400005];
void dfs(int x,int last)
{
	dfn[x]=++tot;
	rnk[dfn[x]]=x;
	for(int i=fst[x];i;i=arr[i].nxt)
	{
		int j=arr[i].tar;
		if(j==last) continue;
		dfs(j,x);
		size[dfn[x]]+=size[dfn[j]];
	} 
	size[dfn[x]]++;
}
int getk(int x)
{
	return ceil(x/(klen*1.0));
}
int lk(int x)
{
//	cout<<x<<" "<<(x-1)*klen+1<<endl;
	return (x-1)*klen+1;
}
int rk(int x)
{
	if(x==len) return n;
	else return x*klen;
}
bool ch1(int p,int x,int y)
{
//	cout<<p<<" "<<x<<" "<<y<<endl;
	set<int>::iterator q=a[p].lower_bound(x);
	if(q==a[p].end()) return false;
	else if(*q<=y) return true;
	else return false;
}
bool ch2(int p,int x,int y)
{
	set<int>::iterator q=b[p].lower_bound(x);
	if(q==b[p].end()) return false;
	else if(*q<=y) return true;
	else return false;
}
bool check(int l,int r,int x,int y)
{
//	cout<<l<<" "<<r<<" "<<x<<" "<<y<<endl;
	if(l>r) return false;
	int p=getk(x)+1,q=getk(y)-1;
	int pp=rk(getk(x)),qq=lk(getk(y));
	if(p>q)
	{
		for(int i=x;i<=y;++i) if(ch1(i,l,r)) return true;
	}
	else
	{
		for(int i=x;i<=pp;++i) if(ch1(i,l,r)) return true;
		for(int i=qq;i<=y;++i) if(ch1(i,l,r)) return true;
		for(int i=p;i<=q;++i) if(ch2(i,l,r)) return true;
	}
	return false;
}
int p[400005],tail=400001;
void add(int x)
{
	p[--tail]=size[dfn[x]];
	x=dfn[x];
	set<int>::iterator q=a[size[x]].lower_bound(x);
	a[size[x]].erase(q);
	q=b[getk(size[x])].lower_bound(x);
	b[getk(size[x])].erase(q);
}
void del(int x)
{
	tail++;
	x=dfn[x];
	a[size[x]].insert(x);
	b[getk(size[x])].insert(x);
}
void get_ans(int x,int last)
{
	if(x!=1)
	add(x);
	int flg=0,num=0,l,r;
	bool bj=0;
	for(int i=fst[x];i;i=arr[i].nxt)
	{
		int j=arr[i].tar;
		if(j==last) continue;
		if(size[dfn[j]]>n/2) flg++,num=size[dfn[j]],l=dfn[j],r=dfn[j]+size[dfn[j]]-1;
		get_ans(j,x);
	}
	del(x);
	if(n-size[dfn[x]]>n/2) flg++,num=n-size[dfn[x]],bj=1;
	if(flg>=2) return;
	if(flg==0)
	{
		dp[x]=1;
		return;
	}
	if(!bj)
	{
		if(check(l,r,num-n/2,n/2))
		dp[x]=1;
	}
	else
	{	
		//x,x+size[x]-1
		if(check(1,dfn[x]-1,num-n/2,n/2)||check(dfn[x]+size[dfn[x]],n,num-n/2,n/2))
		dp[x]=1;
		if(dp[x])
		{
			return;
		}
//		cout<<x<<" "<<1<<endl;
		int ll=lower_bound(p+tail,p+400000+1,n-n/2)-p,rr=400000,mid,ans;
		while(ll<=rr)
		{
			mid=(ll+rr)>>1;
			if(p[mid]-size[dfn[x]]<=n/2)
			{
				dp[x]=1;
				break;
			}
			else
			{
				rr=mid-1;
			}
		}
	}
}
int main()
{
	scanf("%d",&n);
	for(int i=1;i<n;++i)
	{
		int x,y;
		scanf("%d%d",&x,&y);
		adds(x,y);
		adds(y,x);
	}
	klen=sqrt(n),len;
	if(klen*klen==n) len=klen;
	else len=klen+1;
	dfs(1,0);
	for(int i=1;i<=n;++i) a[size[i]].insert(i),b[getk(size[i])].insert(i);
	get_ans(1,0);
	for(int i=1;i<=n;++i) printf("%d ",dp[i]);
}

暴力出奇迹！！！！！

$$\mathcal{T}he\mathcal{E}nd$$