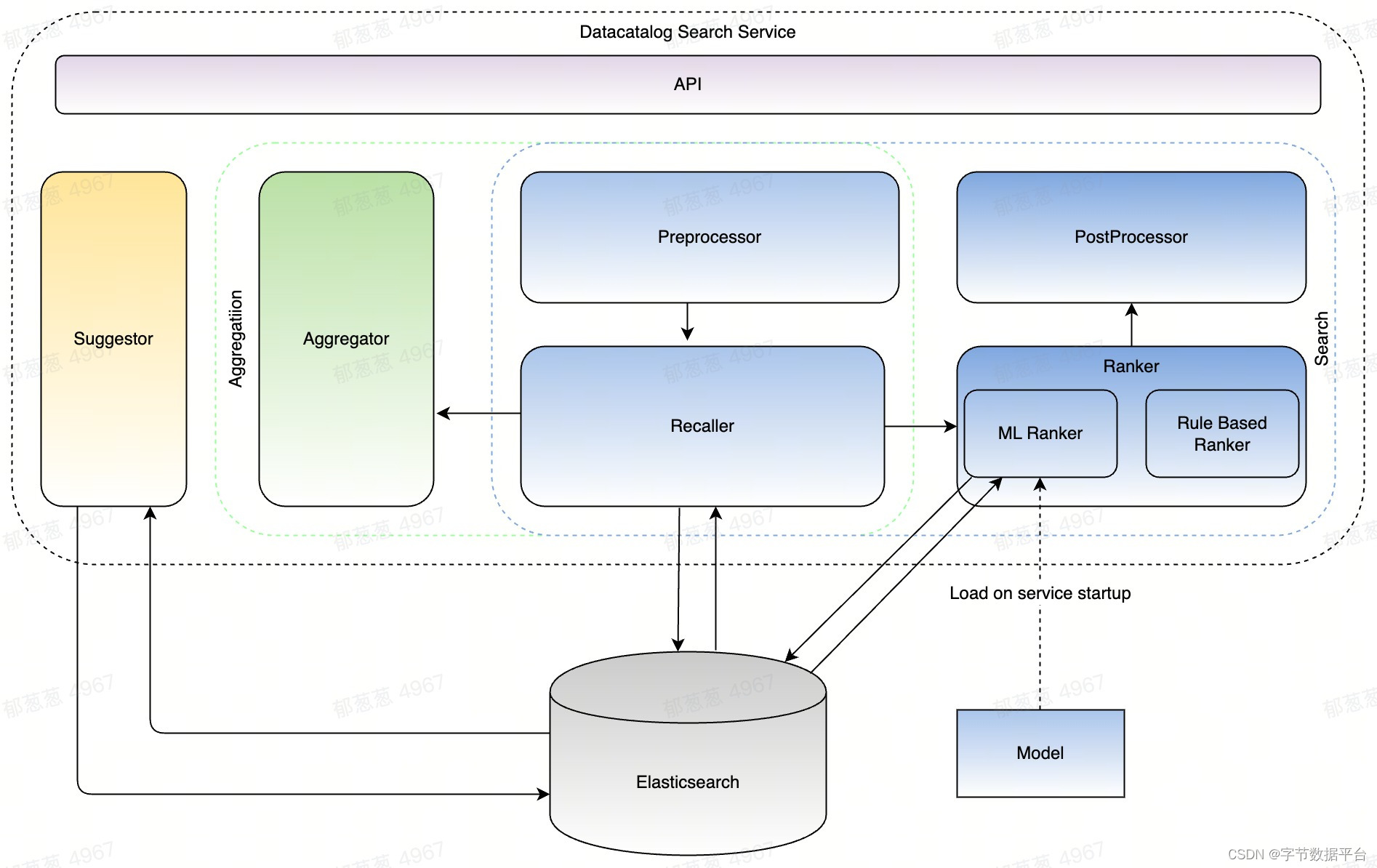
分数 30

全屏浏览题目

切换布局

作者 陈越

单位 浙江大学

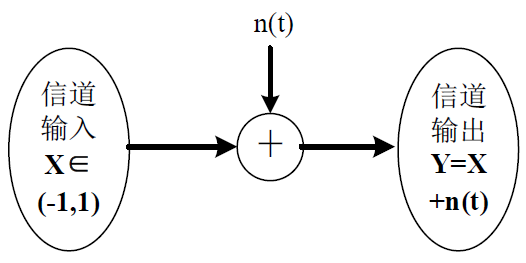
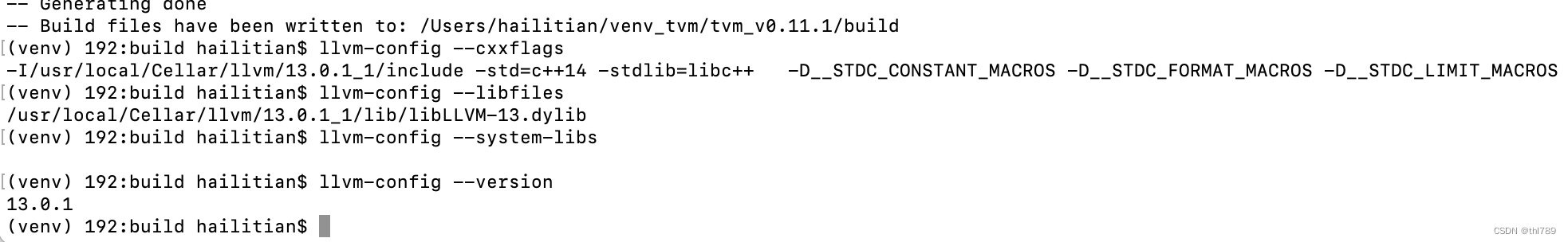
A Cartesian tree is a binary tree constructed from a sequence of distinct numbers. The tree is heap-ordered, and an inorder traversal returns the original sequence. For example, given the sequence { 8, 15, 3, 4, 1, 5, 12, 10, 18, 6 }, the min-heap Cartesian tree is shown by the figure.

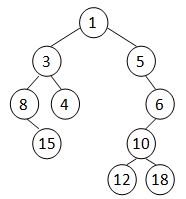
Your job is to output the level-order traversal sequence of the min-heap Cartesian tree.

Input Specification:

Each input file contains one test case. Each case starts from giving a positive integer N (≤30), and then N distinct numbers in the next line, separated by a space. All the numbers are in the range of int.

Output Specification:

For each test case, print in a line the level-order traversal sequence of the min-heap Cartesian tree. All the numbers in a line must be separated by exactly one space, and there must be no extra space at the beginning or the end of the line.

Sample Input:

10
8 15 3 4 1 5 12 10 18 6

Sample Output:

1 3 5 8 4 6 15 10 12 18

代码长度限制

16 KB

时间限制

400 ms

内存限制

64 MB

#include<bits/stdc++.h>
using namespace std;
int n,in[40];
map<int,int>l,r,pos;
int findmin(int il,int ir){//找到在il和ir之间最小的元素 
    int min=0x3f3f3f3f;
    for(int i=il;i<=ir;i++){
        if(min>in[i])min=in[i];
    }
    return min;
}
int build(int il,int ir){
    int root=findmin(il,ir);//找到根结点 
    int k=pos[root];//根结点在中序遍历的下标 
    if(il<k)l[root]=build(il,k-1);//若有左子树，递归 
    if(ir>k)r[root]=build(k+1,ir);//若有右子树，递归 
    return root;//返回根结点 
}
int main(){
    cin>>n;
    for(int i=0;i<n;i++){//输入中序遍历并记录各元素的位置 
        cin>>in[i];
        pos[in[i]]=i;
    }
    int root=build(0,n-1);//建树 
    queue<int>q;
    q.push(root);//插入根结点 
    while(q.size()){//层序遍历 
        int t=q.front();
        q.pop();
        if(l.count(t))q.push(l[t]);
        if(r.count(t))q.push(r[t]);
        cout<<t;
        if(q.size())cout<<' ';
    }
    return 0;
}