【蓝桥日记⑤】2014第五届省赛(软件类)JavaA组☃答案解析
文章目录
- 【蓝桥日记⑤】2014第五届省赛(软件类)JavaA组☃答案解析
- 1、猜年龄
- 2、李白打酒
- 3、神奇算式
- 4、写日志
- 5、锦标赛
- 6、六角填数
- 7、绳圈
- 8、兰顿蚂蚁
- 9、斐波那契
- 10、波动数列
1、猜年龄
解法:暴力枚举
package fiveSession;
/*** 2014第五届 1、猜年龄 ***/
public class test1 {
public static void main(String[] args) {
int age1 = 0, age2 = 0;
boolean find = false;
for (int i = 1; i < 50; i++) {
for (int j = i + 1; j < i + 9; j++) {
if (i * j == 6 * (i + j)) {
age1 = i;
age2 = j;
find = true;
break;
}
}
if (find) break;
}
System.out.println(age1);
}
}
答案:
10
2、李白打酒
解法:递归
package fiveSession;
/*** 2014第五届 2、李白打酒 ***/
public class test2 {
public static void main(String[] args) {
int wine = 2;
int shop = 5, flower = 10;
int res = f(wine, shop, flower);
System.out.println(res);
}
private static int f(int wine, int shop, int flower) {
if (shop < 0 || flower < 0) return 0;
if (wine == 0 && (shop != 0 || flower != 0)) return 0;
if (wine == 0 && shop == 0 && flower == 0) return 1;
int res = f(wine * 2, shop - 1, flower);
res += f(wine - 1, shop, flower - 1);
return res;
}
}
递归二
package fiveSession;
/*** 2014第五届 2、李白打酒 ***/
public class test2 {
static int res = 0;
public static void main(String[] args) {
int wine = 2;
int shop = 5, flower = 10 - 1;
f(wine, shop, flower);
System.out.println(res);
}
private static void f(int wine, int shop, int flower) {
if (wine == 1 && shop == 0 && flower == 0) {
res++;
return;
}
if (shop > 0) f(wine * 2, shop - 1, flower);
if (flower > 0) f(wine - 1, shop, flower - 1);
}
}
答案:
14
3、神奇算式
解法:暴力枚举
package fiveSession;
import java.util.Arrays;
/*** 2014第五届 3、神奇算式 ***/
public class test3 {
public static void main(String[] args) {
int res = 0;
for (int i = 1; i < 10; i++) {
for (int j = 0; j < 10; j++) {
if (i == j) continue;
for (int k = 0; k < 10; k++) {
if (i == k || j == k) continue;
for (int p = 0; p < 10; p++) {
if (i == p || j == p || k == p) continue;
int src = i * 1000 + j * 100 + k * 10 + p;
if (j != 0) {
res += check(src, i * (j * 100 + k * 10 + p));
}
if (k != 0 && (i * 10 + j) < (k * 10 + p)) {
res += check(src, (i * 10 + j) * (k * 10 + p));
}
}
}
}
}
System.out.println(res);
}
public static int check(int src, int r2) {
String s1 = String.valueOf(src);
String s2 = String.valueOf(r2);
char[] cs1 = s1.toCharArray();
char[] cs2 = s2.toCharArray();
Arrays.sort(cs1);
Arrays.sort(cs2);
if (new String(cs1).equals(new String(cs2))) return 1;
return 0;
}
}
答案:
12
4、写日志
解法:找规律
package fiveSession;
/*** 2014第五届 4、写日志 ***/
public class test4 {
private static int n = 1;
public static void write(String msg) {
String filename = "t" + n + ".log";
n = (n % 3) + 1;
System.out.println(filename + "," + msg);
}
public static void main(String[] args) {
for (int i = 0; i < 15; i++) write("a");
}
}
答案:
(n % 3) + 1
5、锦标赛
package fiveSession;
/*** 2014第五届 5、锦标赛 ***/
public class test5 {
static void f(int[] a) {
int n = 1;
while (n < a.length) n *= 2;
int[] b = new int[2 * n - 1];
for (int i = 0; i < n; i++) {
if (i < a.length)
b[n - 1 + i] = i;
else
b[n - 1 + i] = -1;
}
//从最后一个向前处理
for (int i = b.length - 1; i > 0; i -= 2) {
if (b[i] < 0) {
if (b[i - 1] >= 0)
b[(i - 1) / 2] = b[i - 1];
else
b[(i - 1) / 2] = -1;
} else {
if (a[b[i]] > a[b[i - 1]])
b[(i - 1) / 2] = b[i];
else
b[(i - 1) / 2] = b[i - 1];
}
}
//输出树根
System.out.println(b[0] + ":" + a[b[0]]);
//值等于根元素的需要重新pk
pk(a, b, 0, b[0]);
//再次输出树根
System.out.println(b[0] + ":" + a[b[0]]);
}
static void pk(int[] a, int[] b, int k, int v) {
//if(k>b.length) return;
int k1 = k * 2 + 1;
int k2 = k1 + 1;
if (k1 >= b.length || k2 >= b.length) {
// 此处将 叶子结点为最大值的 下标b[k]抹去,置为-1
b[k] = -1; //(要是我我会该行代码设为考点~(@^_^@)~)
return;
}
if (b[k1] == v)
pk(a, b, k1, v);
else
pk(a, b, k2, v);
//重新比较
if (b[k1] < 0) {
if (b[k2] >= 0)
b[k] = b[k2];
else
b[k] = -1;
return;
}
if (b[k2] < 0) {
if (b[k1] >= 0)
b[k] = b[k1];
else
b[k] = -1;
return;
}
if (a[b[k1]]>a[b[k2]])
b[k] = b[k1];
else
b[k] = b[k2];
}
public static void main(String[] args) {
int[] a = {54, 55, 18, 16, 122, 255, 30, 9, 58, 66};
f(a);
}
}
答案:
a[b[k1]]>a[b[k2]]
6、六角填数
解法:全排列+剪枝
package fiveSession;
/*** 2014第五届 6、六角填数 ***/
public class test6 {
static int[] a = {2, 4, 5, 6, 7, 9, 10, 11, 12};
public static void main(String[] args) {
backtrack(0, a.length);
}
private static void backtrack(int begin, int end) {
if (begin == 6 && 8 + a[0] + a[1] + a[2] != 1 + a[0] + a[3] + a[5]) return;
if (begin == 7 && 8 + a[0] + a[1] + a[2] != 8 + a[3] + a[6] + 3) return;
if (begin == end) {
if (8 + a[0] + a[1] + a[2] != a[5] + a[6] + a[7] + a[8]) return;
if (8 + a[0] + a[1] + a[2] != a[2] + a[4] + a[7] + 3) return;
if (8 + a[0] + a[1] + a[2] != 1 + a[1] + a[4] + a[8]) return;
System.out.println(a[3]);
}
for (int i = begin; i < end; i++) {
int t = a[begin]; a[begin] = a[i]; a[i] = t;
backtrack(begin + 1, end);
t = a[begin]; a[begin] = a[i]; a[i] = t;
}
}
}
答案:
10
7、绳圈
解法:动态规划
绳圈组合数:自成一圈+加入前一组合(2个头+i -1个分割点)
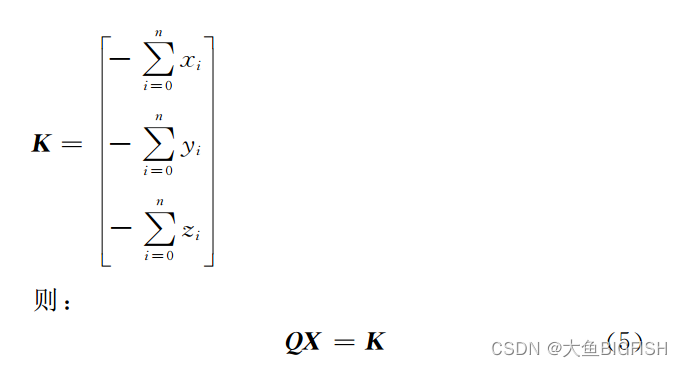
令C[i]表示i个绳的组合数,则有
C
[
i
]
=
c
[
i
−
1
]
+
C
[
i
−
1
]
∗
(
i
−
1
)
∗
2
=
C
[
i
−
1
]
∗
(
2
i
−
1
)
C[i] = c[i -1] + C[i - 1] *(i - 1) * 2 = C[i-1]*(2i-1)
C[i]=c[i−1]+C[i−1]∗(i−1)∗2=C[i−1]∗(2i−1)
令F[i][j]表示i个绳组j个圈的概率,则有
F
[
i
]
[
j
]
=
F
[
i
−
1
]
[
j
−
1
]
∗
C
[
i
−
1
]
+
F
[
i
−
1
]
[
j
]
∗
C
[
i
−
1
]
∗
(
i
−
1
)
∗
2
C
[
i
]
F[i][j]=\frac{F[i-1][j-1]*C[i-1]+F[i-1][j]*C[i-1]*(i-1)*2}{C[i]}
F[i][j]=C[i]F[i−1][j−1]∗C[i−1]+F[i−1][j]∗C[i−1]∗(i−1)∗2
联立两式约分有
F
[
i
]
[
j
]
=
F
[
i
−
1
]
[
j
−
1
]
+
F
[
i
−
1
]
[
j
]
∗
(
i
−
1
)
∗
2
2
i
−
1
F[i][j]=\frac{F[i-1][j-1]+F[i-1][j]*(i-1)*2}{2i-1}
F[i][j]=2i−1F[i−1][j−1]+F[i−1][j]∗(i−1)∗2
package fiveSession;
/*** 2014第五届 7、绳圈 ***/
public class test7 {
public static void main(String[] args) {
double[][] f = new double[101][101];
f[1][1] = 1;
for (int rope = 2; rope <= 100; rope++) {
f[rope][1] = f[rope - 1][1] * (rope - 1) * 2 / (2 * rope - 1);
for (int circle = 2; circle <= rope; circle++) {
f[rope][circle] = (f[rope - 1][circle - 1] + f[rope - 1][circle] * (rope - 1) * 2) / (2 * rope - 1);
}
}
int ans = 0;
double maxVal = 0.0;
for (int circle = 1; circle <= 100; circle++) {
if (f[100][circle] > maxVal) {
maxVal = f[100][circle];
ans = circle;
}
}
System.out.println(ans);
}
}
答案:
3
8、兰顿蚂蚁
解法:方向模拟
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 8、兰顿蚂蚁 ***/
public class test8 {
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
int m = sc.nextInt();
int n = sc.nextInt();
int[][] g = new int[m][n];
for (int i = 0; i < m; i++) {
for (int j = 0; j < n; j++) {
g[i][j] = sc.nextInt();
}
}
int x = sc.nextInt();
int y = sc.nextInt();
String s = sc.next();
int d = getD(s);
int k = sc.nextInt();
// 方向: 上右下左
int[][] dirs = {{-1, 0}, {0, 1}, {1, 0}, {0, -1}};
for (int i = 1; i <= k; i++) {
if (g[x][y] == 0) {
d = (d + 3) % 4;
g[x][y] = 1;
} else {
d = (d + 1) % 4;
g[x][y] = 0;
}
x = x + dirs[d][0];
y = y + dirs[d][1];
}
System.out.println(x + " " + y);
}
private static int getD(String s) {
if (s.equals("U")) return 0;
if (s.equals("R")) return 1;
if (s.equals("D")) return 2;
return 3;
}
}
9、斐波那契
解法一:暴力法(4/7)
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 9、斐波那契,暴力法1 ***/
public class test9 {
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
long n = sc.nextLong();
long m = sc.nextLong();
long p = sc.nextLong();
long sum = 1;
long t = 0, f1 = 0, f2 = 1, mMod = 0;
for (long i = 2; i <= n; i++) {
t = f2 + f1;
f1 = f2;
f2 = t;
sum += t;
if (i == m) mMod = t;
}
if (m > n) {
for (long i = n + 1; i <= m; i++) {
t = f2 + f1;
f1 = f2;
f2 = t;
}
mMod = f2;
}
System.out.println(sum % mMod % p);
}
}
解法二:公式定理+矩阵运算
我这个水平,能暴力就暴力,现在记公式定理性价比不高,推理构造的到时可以深究一下。
有兴趣的可以看一下参考:从蓝桥杯来谈Fibonacci数列
10、波动数列
解法一:枚举首项+dfs(2/8)
x x+a x+2a x+3a ··· x+(n-1)a s = nx + n(n-1)a/2
x x-b x-2b x-3b ··· x-(n-1)b s = nx - n(n-1)b/2
通过上式可以求出首项x的范围
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 10、波动数列 ***/
public class test10 {
static int n, s, a, b;
static long ans;
static final int MOD = (int)1e8 + 7;
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
n = sc.nextInt();
s = sc.nextInt();
a = sc.nextInt();
b = sc.nextInt();
sc.close();
// x x+a x+2a x+3a ··· x+(n-1)a s = nx + n(n-1)a/2
//x x-b x-2b x-3b ··· x-(n-1)b s = nx - n(n-1)b/2
int minX = (s - (n - 1) * n * a / 2) / n;
int maxX = (s + (n - 1) * n * b / 2) / n;
for (int x = minX; x <= maxX; x++) {
dfs(x, 1, x);
}
System.out.println(ans);
}
private static void dfs(int x, int cnt, int sum) {
if (cnt == n) {
if (sum == s) {
ans++;
if (ans > MOD) ans %= MOD;
}
return;
}
dfs(x + a, cnt + 1, sum + x + a);
dfs(x - b, cnt + 1, sum + x - b);
}
}
优化:枚举a,b的数目+dfs(2/8)
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 10、波动数列, 优化枚举 ***/
public class test10_2 {
static int n, s, a, b;
static long ans;
static final int MOD = (int)1e8 + 7;
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
n = sc.nextInt();
s = sc.nextInt();
a = sc.nextInt();
b = sc.nextInt();
sc.close();
// x x+p x+2p x+3p ··· x+(n-1)p s = nx + n(n-1)p/2
// t = n(n-1)/2
// 若a的数目为ta, 则b数目为tb
int t = n * (n - 1) / 2;
long minX = (s - a * t) / n;
long maxX = (s + b * t) / n;
for (long x = minX; x <= maxX; x++) {
for (long ta = 0; ta <= t; ta++) {
// 减少对x的枚举
long curSum = x * n + ta * a - (t - ta) * b;
if (curSum == s) dfs(x, 1, x);
}
}
System.out.println(ans);
}
private static void dfs(long x, int cnt, long sum) {
if (cnt == n) {
if (sum == s) {
ans++;
if (ans > MOD) ans %= MOD;
}
return;
}
dfs(x + a, cnt + 1, sum + x + a);
dfs(x - b, cnt + 1, sum + x - b);
}
}
解法二:动态规划,求a和b的组合数(7/10)
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 10、波动数列, 动态规划 ***/
public class test10_3{
static int n, s, a, b;
static long ans;
static final int MOD = (int)1e8 + 7;
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
n = sc.nextInt();
s = sc.nextInt();
a = sc.nextInt();
b = sc.nextInt();
sc.close();
dpMethod();
System.out.println(ans);
}
private static void dpMethod() {
int t = n * (n - 1) / 2;
// dp[i][j]表示用前i个数组成值j的组合数方法
int[][] dp = new int[n][t + 1];
dp[0][0] = 1;
for (int i = 0; i < n; i++) dp[i][0] = 1;
for (int i = 1; i < n; i++) {
for (int j = 1; j <= t; j++) {
if (i > j) {
dp[i][j] = dp[i - 1][j];
} else {
dp[i][j] = dp[i - 1][j] + dp[i - 1][j - i];
}
dp[i][j] %= MOD;
}
}
for (int ta = 0; ta <= t; ta++) {
if ((s - ta * a + (t - ta) * b) % n == 0) {
ans = (ans + dp[n - 1][ta]) % MOD;
}
}
}
}
优化:动态规划+滚动数组将状态压缩为二维(7/10)
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 10、波动数列, 动态规划 ***/
public class test10_3{
static int n, s, a, b;
static long ans;
static final int MOD = (int)1e8 + 7;
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
n = sc.nextInt();
s = sc.nextInt();
a = sc.nextInt();
b = sc.nextInt();
sc.close();
dpMethod2();
System.out.println(ans);
}
private static void dpMethod2() {
int t = n * (n - 1) / 2;
// dp[i][j]表示用前i个数组成值j的组合数方法
int[][] dp = new int[2][t + 1];
dp[0][0] = dp[1][0] = 1;
int row = 0;
for (int i = 1; i < n; i++) {
row = 1 - row;
for (int j = 1; j <= t; j++) {
if (i > j) {
dp[row][j] = dp[1 - row][j];
} else {
dp[row][j] = dp[1 - row][j] + dp[1 - row][j - i];
}
dp[row][j] %= MOD;
}
}
for (long ta = 0; ta <= t; ta++) {
if ((s - ta * a + (t - ta) * b) % n == 0) {
ans = (ans + dp[row][(int)ta]) % MOD;
}
}
}
}
优化:动态规划+状态压缩为一维(10/10)
package fiveSession;
import java.util.Scanner;
/*** 2014第五届 10、波动数列, 动态规划 ***/
public class test10_3{
static int n, s, a, b;
static long ans;
static final int MOD = (int)1e8 + 7;
public static void main(String[] args) {
Scanner sc = new Scanner(System.in);
n = sc.nextInt();
s = sc.nextInt();
a = sc.nextInt();
b = sc.nextInt();
sc.close();
dpMethod3();
System.out.println(ans);
}
private static void dpMethod3() {
int t = n * (n - 1) / 2;
// dp[i][j]表示用前i个数组成值j的组合数方法
int[] dp = new int[t + 1];
dp[0] = 1;
for (int i = 1; i < n; i++) {
// 减少j的枚举
for (int j = i * (i + 1) / 2; j >= i; j--) {
dp[j] = (dp[j] + dp[j - i]) % MOD;
}
}
for (long ta = 0; ta <= t; ta++) {
if ((s - ta * a + (t - ta) * b) % n == 0) {
ans = (ans + dp[(int)ta]) % MOD;
}
}
}
}
这道题目的两种方法的一步步优化真的惊艳到我了!佩服佩服!
参考资料:【蓝桥杯JavaA组】2013-2018年试题讲解(附含C语言版)
总的来说排列组合和动态规划涉及的很多,动态规划永远的伤啊~