数据结构与算法

1. 概述

二叉树是这么一种树状结构：每个节点最多有两个孩子，左孩子和右孩子

完全二叉树：是一种二叉树结构，除了最后一层以外，每一层都必须填满，填充时要遵循从左到右

平衡二叉树：是一种二叉树结构，其中每个节点的左右子树高度相差不超过1

2. 存储

存储方式分为两种:

①定义树结点与左、右孩子引用（TreeNode）

②使用数组，若用0作为树的根节点，索引可以通过以下方式计算

父 = floor((子 - 1) / 2)
左孩子 = 父 * 2 + 1
右孩子 = 父 * 2 + 2

3. 遍历

遍历方式也分为两种：

①广度优先遍历：尽可能先访问距离根节点最近的节点，也称为层序遍历

②深度优先遍历：对于二叉树，可以进一步划分为三种（要深入到叶子节点）

pre-order前序遍历：对于每一棵子树，先访问该节点，然后是左子树，最后是右子树
in-order中序遍历：对于每一棵子树，先访问左子树，然后是该节点，最后是右子树
post-order后续遍历：对于每一棵子树，先访问左子树，然后是右子树，最后是该节点

3.1 广度优先遍历

本轮开始时队列	本轮访问节点
[1]	1
[2, 3]	2
[3, 4]	3
[4, 5, 6]	4
[5, 6]	5
[6, 7, 8]	6
[7, 8]	7
[8]	8
[]

1. 初始化，将根节点加入队列

2. 循环处理队列中每个节点，直至队列为空

3. 每次循环内处理节点后，将它的孩子节点（即下一层节点）加入队列

注意：

以上用队列来实现层序遍历是针对TreeNode这种方式表示的二叉树
对于数组实现的二叉树，则直接遍历数组即可，自然为层序遍历的顺序

3.2 深度优先遍历

栈暂存	已处理	前序遍历	中序遍历
[1]	1 ✔️ 左💤 右💤	1
[1, 2]	2✔️ 左💤 右💤 1✔️ 左💤 右💤	2
[1, 2, 4]	4✔️ 左✔️ 右✔️ 2✔️ 左💤 右💤 1✔️ 左💤 右💤	4	4
[1, 2]	2✔️ 左✔️ 右✔️ 1✔️ 左💤 右💤		2
[1]	1✔️ 左✔️ 右💤		1
[1, 3]	3✔️ 左💤 右💤 1✔️ 左✔️ 右💤	3
[1, 3, 5]	5✔️ 左✔️ 右✔️ 3✔️ 左💤 右💤 1✔️ 左✔️ 右💤	5	5
[1, 3]	3✔️ 左✔️ 右💤 1✔️ 左✔️ 右💤		3
[1, 3, 6]	6✔️ 左✔️ 右✔️ 3✔️ 左✔️ 右💤 1✔️ 左✔️ 右💤	6	6
[1, 3]	3✔️ 左✔️ 右✔️ 1✔️ 左✔️ 右💤
[1]	1✔️ 左✔️ 右✔️
[]

3.2.1 递归实现

/**
 * <h3>前序遍历</h3>
 * @param node 节点
 */
static void preOrder(TreeNode node) {
    if (node == null) {
        return;
    }
    System.out.print(node.val + "\t"); // 值
    preOrder(node.left); // 左
    preOrder(node.right); // 右
}

/**
 * <h3>中序遍历</h3>
 * @param node 节点
 */
static void inOrder(TreeNode node) {
    if (node == null) {
        return;
    }
    inOrder(node.left); // 左
    System.out.print(node.val + "\t"); // 值
    inOrder(node.right); // 右
}

/**
 * <h3>后序遍历</h3>
 * @param node 节点
 */
static void postOrder(TreeNode node) {
    if (node == null) {
        return;
    }
    postOrder(node.left); // 左
    postOrder(node.right); // 右
    System.out.print(node.val + "\t"); // 值
}

3.2.2 非递归实现

前序遍历

LinkedListStack<TreeNode> stack = new LinkedListStack<>();  // 此处的LinkedListStack为自己实现的
TreeNode curr = root;

while (!stack.isEmpty() || curr != null) {
    if (curr != null) {
        stack.push(curr);
        System.out.println(curr);
        curr = curr.left;
    } else {
        TreeNode pop = stack.pop();
        curr = pop.right;
    }

}

中序遍历

LinkedListStack<TreeNode> stack = new LinkedListStack<>();
TreeNode curr = root;

while (!stack.isEmpty() || curr != null) {
    if (curr != null) {
        stack.push(curr);
        curr = curr.left;
    } else {
        TreeNode pop = stack.pop();
        System.out.println(pop);
        curr = pop.right;
    }
}

后序遍历

LinkedListStack<TreeNode> stack = new LinkedListStack<>();
TreeNode curr = root;
TreeNode pop = null;

while (!stack.isEmpty() || curr != null) {
    if (curr != null) {
        stack.push(curr);
        curr = curr.left;
    } else {
        TreeNode peek = stack.peek();
        if (peek.right == null || peek.right == pop) {
            pop = stack.pop();
            System.out.println(pop);
        } else {
            curr = peek.right;
        }
    }
}

对于后序遍历，向后走时，需要处理完右子树才能pop出栈。如何直到右子树处理完成呢？

①如果栈顶元素的 right == null ，表示没啥可处理的，可以出栈

②如果栈顶元素的 right != null

那么使用lastPop记录最近出栈的节点，即表示从这个节点向回走
如果栈顶元素 right == lastPop，此时应当出栈

对于前、中两种遍历，实际以上代码从右子树向回走时，并未走完全程（stack提前出栈了），而后序遍历以上代码是走完全程了。

统一写法（依据后序遍历修改）

LinkedList<TreeNode> stack = new LinkedList<>();

TreeNode curr = root; // 代表当前节点
TreeNode pop = null; // 最近一次弹栈的元素
while (curr != null || !stack.isEmpty()) {
    if (curr != null) {
        colorPrintln("前: " + curr.val, 31);
        stack.push(curr); // 压入栈，为了记住回来的路
        curr = curr.left;
    } else {
        TreeNode peek = stack.peek();
        // 右子树可以不处理, 对中序来说, 要在右子树处理之前打印
        if (peek.right == null) {
            colorPrintln("中: " + peek.val, 36);
            pop = stack.pop();
            colorPrintln("后: " + pop.val, 34);
        }
        // 右子树处理完成, 对中序来说, 无需打印
        else if (peek.right == pop) {
            pop = stack.pop();
            colorPrintln("后: " + pop.val, 34);
        }
        // 右子树待处理, 对中序来说, 要在右子树处理之前打印
        else {
            colorPrintln("中: " + peek.val, 36);
            curr = peek.right;
        }
    }
}

public static void colorPrintln(String origin, int color) {
    System.out.printf("\033[%dm%s\033[0m%n", color, origin);
}

一张图演示三种遍历

红色：前序遍历
绿色：中序遍历
蓝色：后序遍历

4. 习题

4.1 前序遍历二叉树

给你二叉树的根节点 root ，返回它节点值的前序遍历。

示例 1：

输入：root = [1,null,2,3]
输出：[1,2,3]

示例 2：

输入：root = []
输出：[]

示例 3：

输入：root = [1]
输出：[1]

示例 4：

输入：root = [1,2]
输出：[1,2]

示例 5：

输入：root = [1,null,2]
输出：[1,2]

提示：

树中节点数目在范围 [0, 100] 内
-100 <= Node.val <= 100

进阶：递归算法很简单，你可以通过迭代算法完成吗？

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<>();
        preorderHelper(root, result);
        return result;
    }

    private void preorderHelper(TreeNode root, List<Integer> result) {
        if (root == null) {
            return;
        }
        result.add(root.val);
        preorderHelper(root.left, result);
        preorderHelper(root.right, result);
    }
}

解法二：迭代

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        LinkedList<TreeNode> stack = new LinkedList<>();
        List<Integer> result = new ArrayList<>();
        TreeNode curr = root;

        while (!stack.isEmpty() || curr != null) {
            if (curr != null) {
                stack.push(curr);
                result.add(curr.val);
                curr = curr.left;
            } else {
                TreeNode pop = stack.pop();
                curr = pop.right;
            }
        }

        return result;
    }
}

解法三：莫里斯遍历（Morris Traversal）

①莫里斯遍历的核心思想是通过利用树的空指针链接来避免使用栈

②对于每个节点，如果它的左子树为空，则访问当前节点并移动到右子树

③如果左子树不为空，找到当前节点的前驱节点（即左子树中最右的节点），检查它的右指针

如果它的右指针为空，则将其指向当前节点，并返回当前节点
如果它的右指针已经指向当前节点，说明左子树已经遍历结束，将右指针恢复为null，并移动到右子树。

时间复杂度：O(n)；空间复杂度：O(1)

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {  
    public List<Integer> preorderTraversal(TreeNode root) {  
        List<Integer> result = new ArrayList<>();  
        TreeNode curr = root;  

        while (curr != null) {  
            if (curr.left == null) {  
                // 访问当前节点  
                result.add(curr.val);  
                curr = curr.right; // 移动到右子树  
            } else {  
                // 找到当前节点的前驱节点  
                TreeNode pred = curr.left;  
                while (pred.right != null && pred.right != curr) {  
                    pred = pred.right;  
                }  
                
                // 建立链接  
                if (pred.right == null) {  
                    pred.right = curr; // 建立临时连接  
                    result.add(curr.val); // 访问当前节点  
                    curr = curr.left; // 移动到左子树  
                } else {  
                    // 恢复树结构  
                    pred.right = null;  
                    curr = curr.right; // 移动到右子树  
                }  
            }  
        }  

        return result;  
    }  
}

4.2 中序遍历二叉树

给定一个二叉树的根节点 root ，返回 它的中序遍历 。

示例 1：

输入：root = [1,null,2,3]
输出：[1,3,2]

示例 2：

输入：root = []
输出：[]

示例 3：

输入：root = [1]
输出：[1]

提示：

树中节点数目在范围 [0, 100] 内
-100 <= Node.val <= 100

进阶: 递归算法很简单，你可以通过迭代算法完成吗？

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<>();
        inorderHelper(root, result);
        return result;
    }

    private void inorderHelper(TreeNode root, List<Integer> result) {
        if (root == null) {
            return;
        }
        inorderHelper(root.left, result);
        result.add(root.val);
        inorderHelper(root.right, result);
    }
}

解法二：迭代

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        LinkedList<TreeNode> stack = new LinkedList<>();
        List<Integer> result = new ArrayList<>();
        TreeNode curr = root;

        while (!stack.isEmpty() || curr != null) {
            if (curr != null) {
                stack.push(curr);
                curr = curr.left;
            } else {
                TreeNode pop = stack.pop();
                result.add(pop.val);
                curr = pop.right;
            }
        }

        return result;
    }
}

解法三：莫里斯算法

①莫里斯遍历的核心思想是通过利用树的空指针链接来避免使用栈

②对于每个节点，如果它的左子树为空，则访问当前节点并移动到右子树

③如果左子树不为空，找到当前节点的前驱节点（即左子树中最右的节点），检查它的右指针

如果它的右指针为空，则将其指向当前节点，并返回当前节点
如果它的右指针已经指向当前节点，说明左子树已经遍历结束，将右指针恢复为null，并移动到右子树。

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<>();
        TreeNode curr = root;

        while (curr != null) {
            if (curr.left == null) { // 左子树为空
                // 访问当前节点
                result.add(curr.val);
                // 移动到右子树
                curr = curr.right;
            } else {
                // 找到当前节点的前驱节点
                TreeNode pred = curr.left;
                while (pred.right != null && pred.right != curr) {
                    pred = pred.right;
                }

                // 建立链接
                if (pred.right == null) {
                    // 建立临时链接
                    pred.right = curr;
                    // 移动到左子树
                    curr = curr.left;
                } else {
                    // 恢复树结构
                    pred.right = null;
                    // 访问当前节点
                    result.add(curr.val);
                    // 移动到右子树
                    curr = curr.right;
                }
            }
        }
        return result;
    }
}

4.3 后序遍历二叉树

给你一棵二叉树的根节点 root ，返回其节点值的 后序遍历 。

示例 1：

输入：root = [1,null,2,3]
输出：[3,2,1]

示例 2：

输入：root = []
输出：[]

示例 3：

输入：root = [1]
输出：[1]

提示：

树中节点的数目在范围 [0, 100] 内
-100 <= Node.val <= 100

进阶：递归算法很简单，你可以通过迭代算法完成吗？

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<>();
        postOrderHelper(root, result);
        return result;
    }

    private void postOrderHelper(TreeNode root, List<Integer> result) {
        if (root == null) {
            return;
        }
        postOrderHelper(root.left, result);
        postOrderHelper(root.right, result);
        result.add(root.val);
    }
}

解法二：迭代

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        LinkedList<TreeNode> stack = new LinkedList<>();
        List<Integer> result = new ArrayList<>();
        TreeNode curr = root;
        TreeNode pop = null;

        while (!stack.isEmpty() || curr != null) {
            if (curr != null) {
                stack.push(curr);
                curr = curr.left;
            } else {
                TreeNode peek = stack.peek();
                if (peek.right == null || peek.right == pop) {
                    pop = stack.pop();
                    result.add(pop.val);
                } else {
                    curr = peek.right;
                }
            }
        }

        return result;
    }
}

4.4 对称二叉树

给你一个二叉树的根节点 root ，检查它是否轴对称。

示例 1：

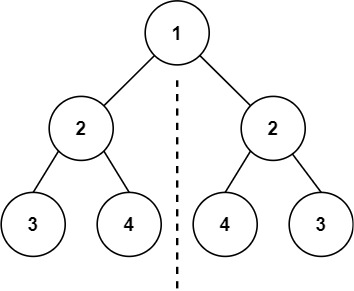
输入：root = [1,2,2,3,4,4,3]
输出：true

示例 2：

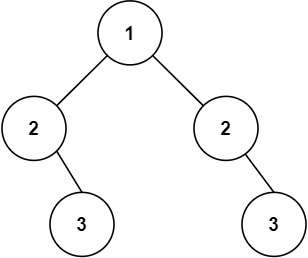
输入：root = [1,2,2,null,3,null,3]
输出：false

提示：

树中节点数目在范围 [1, 1000] 内
-100 <= Node.val <= 100

进阶：你可以运用递归和迭代两种方法解决这个问题吗？

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    public boolean isSymmetric(TreeNode root) {
        if(root == null) {
            return true;
        }
        return dfs(root.left, root.right);
    }

    private boolean dfs(TreeNode left, TreeNode right) {
        if(left == null && right == null) {
            return true;
        } 
        if(left == null || right == null) {
            return false;
        }

        return (left.val == right.val) && dfs(left.left, right.right) && dfs(left.right, right.left);
    }
}

解法二：迭代

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public boolean isSymmetric(TreeNode root) {
        if (root == null) {
            return true;
        }

        Queue<TreeNode> queue = new LinkedList<>();
        queue.offer(root.left);
        queue.offer(root.right);

        while (!queue.isEmpty()) {
            TreeNode leftNode = queue.poll();
            TreeNode rightNode = queue.poll();

            if (leftNode == null && rightNode == null) {  // 左右两个子树为空
                continue;
            }

            if (leftNode == null || rightNode == null) {  // 两边只有一个子树为空
                return false;
            }

            if (leftNode.val != rightNode.val) {
                return false;
            }

            queue.offer(leftNode.left);
            queue.offer(rightNode.right);
            queue.offer(leftNode.right);
            queue.offer(rightNode.left);
        }

        return true;
    }
}

4.5 二叉树最大深度

给定一个二叉树 root ，返回其最大深度。

二叉树的 最大深度 是指从根节点到最远叶子节点的最长路径上的节点数。

示例 1：

输入：root = [3,9,20,null,null,15,7]
输出：3

示例 2：

输入：root = [1,null,2]
输出：2

提示：

树中节点的数量在 [0, 10^4] 区间内。
-100 <= Node.val <= 100

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public int maxDepth(TreeNode root) {
        if (root == null) {
            return 0;
        }
        return Math.max(maxDepth(root.left), maxDepth(root.right)) + 1;
    }
}

解法二：

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public int maxDepth(TreeNode root) {
        if (root == null) {
            return 0;
        }
        Stack<Pair<TreeNode, Integer>> stack = new Stack<>();
        stack.push(new Pair<>(root, 1));
        int maxDepth = 0;

        while (!stack.isEmpty()) {
            Pair<TreeNode, Integer> current = stack.pop();
            TreeNode node = current.getKey();
            int depth = current.getValue();
            maxDepth = Math.max(depth, maxDepth);

            if (node.left != null) {
                stack.push(new Pair<>(node.left, depth + 1));
            }
            if (node.right != null) {
                stack.push(new Pair<>(node.right, depth + 1));
            }
        }

        return maxDepth;
    }
}

解法三：使用二叉树的非递归后序遍历，栈的最大高度即为最大深度

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    // 使用非递归后序遍历，栈的最大高度即为最大深度
    public int maxDepth(TreeNode root) {
        TreeNode curr = root;
        TreeNode pop = null;
        LinkedList<TreeNode> stack = new LinkedList<>();
        int max = 0; // 栈的最大高度

        while(curr != null || !stack.isEmpty()) {
            if(curr != null) {
                stack.push(curr);
                max = Integer.max(stack.size(), max);
                curr = curr.left;
            } else {
                TreeNode peek = stack.peek();
                if(peek.right == null || peek.right == pop) {
                    pop = stack.pop();
                } else {
                    curr = peek.right;
                }
            }
        }
        return max;
    }
}

解法四：二叉树的层序遍历，层数即最大深度

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public int maxDepth(TreeNode root) {
        if (root == null) {
            return 0;
        }
        Queue<TreeNode> queue = new LinkedList<>();
        queue.offer(root);
        int depth = 0;

        while (!queue.isEmpty()) {
            int size = queue.size();
            for (int i = 0; i < size; i++) {
                TreeNode poll = queue.poll();
                if (poll.left != null) {
                    queue.offer(poll.left);
                }
                if (poll.right != null) {
                    queue.offer(poll.right);
                }
            }
            depth++;
        }

        return depth;
    }
}

4.6 二叉树最小深度

给定一个二叉树，找出其最小深度。

最小深度是从根节点到最近叶子节点的最短路径上的节点数量。

说明：叶子节点是指没有子节点的节点。

示例 1：

输入：root = [3,9,20,null,null,15,7]
输出：2

示例 2：

输入：root = [2,null,3,null,4,null,5,null,6]
输出：5

提示：

树中节点数的范围在 [0, 10^5] 内
-1000 <= Node.val <= 1000

解法一：层序遍历。遇到第一个叶子节点所在层数即为最小深度

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public int minDepth(TreeNode root) {
        if (root == null) {
            return 0;
        }

        Queue<TreeNode> queue = new LinkedList<>();
        queue.offer(root);
        int depth = 1;

        while (!queue.isEmpty()) {
            int size = queue.size();
            for (int i = 0; i < size; i++) {
                TreeNode node = queue.poll();
                if (node.left == null && node.right == null) {
                    return depth;
                }
                if (node.left != null) {
                    queue.offer(node.left);
                }
                if (node.right != null) {
                    queue.offer(node.right);
                }
            }
            depth++;
        }
        return depth;
    }
}

解法二：后序遍历

相较于求最大深度，应当考虑：

当右子树为null，应当返回左子树深度加一
当左子树为null，应当返回右子树深度加一

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public int minDepth(TreeNode node) {
        if (node == null) {
            return 0;
        }
        int d1 = minDepth(node.left);
        int d2 = minDepth(node.right);
        if (d1 == 0 || d2 == 0) {
            return d1 + d2 + 1;
        }
        return Integer.min(d1, d2) + 1;
    }
}

4.7 翻转二叉树

给你一棵二叉树的根节点 root ，翻转这棵二叉树，并返回其根节点。

示例 1：

输入：root = [4,2,7,1,3,6,9]
输出：[4,7,2,9,6,3,1]

示例 2：

输入：root = [2,1,3]
输出：[2,3,1]

示例 3：

输入：root = []
输出：[]

提示：

树中节点数目范围在 [0, 100] 内
-100 <= Node.val <= 100

解法一：递归

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    public TreeNode invertTree(TreeNode root) {
        reverse(root);
        return root;
    }

    private void reverse(TreeNode node) {
        if(node == null) {
            return;
        }

        TreeNode t = node.left;
        node.left = node.right;
        node.right = t;

        reverse(node.left);
        reverse(node.right);
    }
}

或

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 *     int val;
 *     TreeNode left;
 *     TreeNode right;
 *     TreeNode() {}
 *     TreeNode(int val) { this.val = val; }
 *     TreeNode(int val, TreeNode left, TreeNode right) {
 *         this.val = val;
 *         this.left = left;
 *         this.right = right;
 *     }
 * }
 */
class Solution {
    public TreeNode invertTree(TreeNode root) {
        if(root == null) {
            return null;
        }

        // 递归翻转左右子树
        TreeNode left = invertTree(root.left);
        TreeNode right = invertTree(root.right);

        // 交换左右子树
        // TreeNode t = root.left;
        root.left = root.right;
        root.right = left;

        return root;
    }
}

4.8 后缀表达式转二叉树

遇到运算符，则出栈两次，将出栈元素与当前节点建立父子关系，当前节点入栈
遇到数字则入栈

    public TreeNode constructExpressionTree(String[] tokens) {
        LinkedList<TreeNode> stack = new LinkedList<>();
        for (String token : tokens) {
            switch (token) {
                // 遇到运算符，出栈两次，与当前节点建立父子关系，将当前节点入栈
                case "+", "-", "*", "/" -> {
                    TreeNode right = stack.pop();
                    TreeNode left = stack.pop();
                    TreeNode parent = new TreeNode(Integer.parseInt(token));
                    parent.left = left;
                    parent.right = right;
                    stack.push(parent);
                }
                default -> {  // 遇到数字入栈
                    stack.push(new TreeNode(Integer.parseInt(token)));
                }
            }
        }
        return stack.peek();
    }

4.9 根据前序与中序遍历结果构造二叉树

给定两个整数数组 preorder 和 inorder ，其中 preorder 是二叉树的先序遍历， inorder 是同一棵树的中序遍历，请构造二叉树并返回其根节点。

示例 1:

输入: preorder = [3,9,20,15,7], inorder = [9,3,15,20,7]
输出: [3,9,20,null,null,15,7]

示例 2:

输入: preorder = [-1], inorder = [-1]
输出: [-1]

提示:

1 <= preorder.length <= 3000
inorder.length == preorder.length
-3000 <= preorder[i], inorder[i] <= 3000
preorder 和 inorder 均 无重复 元素
inorder 均出现在 preorder
preorder 保证为二叉树的前序遍历序列
inorder 保证为二叉树的中序遍历序列

解法一：

先通过前序遍历结果定位根节点
再结合中序遍历结果切分左右子树

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public TreeNode buildTree(int[] preorder, int[] inorder) {
        Map<Integer, Integer> indexMap = new HashMap<>();
        for (int i = 0; i < inorder.length; i++) {
            indexMap.put(inorder[i], i);
        }

        return buildTreeHelper(preorder, inorder, 0, preorder.length - 1, 0, inorder.length - 1, indexMap);
    }

    private TreeNode buildTreeHelper(int[] preorder, int[] inorder, int preStart, int preEnd, int inStart, int inEnd,
            Map<Integer, Integer> indexMap) {
        if (preStart > preEnd || inStart > inEnd) {
            return null;
        }

        int rootVal = preorder[preStart]; // 根节点的位置
        TreeNode root = new TreeNode(rootVal);

        int inIndex = indexMap.get(rootVal);
        int leftSubtreeSize = inIndex - inStart; // 左子树

        root.left = buildTreeHelper(preorder, inorder, preStart + 1, preStart + leftSubtreeSize, inStart, inIndex - 1,
                indexMap);
        root.right = buildTreeHelper(preorder, inorder, preStart + leftSubtreeSize + 1, preEnd, inIndex + 1, inEnd,
                indexMap);

        return root;
    }
}

4.10 根据中序与后序遍历结果构造二叉树

解法一：

先通过后序遍历结果定位根节点
再结合中序遍历结果划分左右子树

/**
 * Definition for a binary tree node.
 * public class TreeNode {
 * int val;
 * TreeNode left;
 * TreeNode right;
 * TreeNode() {}
 * TreeNode(int val) { this.val = val; }
 * TreeNode(int val, TreeNode left, TreeNode right) {
 * this.val = val;
 * this.left = left;
 * this.right = right;
 * }
 * }
 */
class Solution {
    public TreeNode buildTree(int[] inorder, int[] postorder) {
        Map<Integer, Integer> indexMap = new HashMap<>();
        for (int i = 0; i < inorder.length; i++) {
            indexMap.put(inorder[i], i);
        }

        return buildTreeHelper(inorder, postorder, 0, inorder.length - 1, 0, postorder.length - 1, indexMap);
    }

    private TreeNode buildTreeHelper(int[] inorder, int[] postorder, int inStart, int inEnd, int postStart, int postEnd,
            Map<Integer, Integer> indexMap) {
        if (inStart > inEnd || postStart > postEnd) {
            return null;
        }

        int rootVal = postorder[postEnd]; // 根节点的位置
        TreeNode root = new TreeNode(rootVal);

        int inIndex = indexMap.get(rootVal);
        int rightSubtreeSize = inEnd - inIndex; // 右子树

        root.left = buildTreeHelper(inorder, postorder, inStart, inIndex - 1, postStart, postEnd - rightSubtreeSize - 1,
                indexMap);
        root.right = buildTreeHelper(inorder, postorder, inIndex + 1, inEnd, postEnd - rightSubtreeSize, postEnd - 1,
                indexMap);

        return root;
    }
}