day15|各种遍历的应用

相关题目：
层次遍历会一打十
反转二叉树
对称二叉树

层次遍历会一打十

自底向上的层序遍历

在这里插入图片描述
实现思路：层次遍历二叉树，将遍历后的结果revers即可

public List<List<Integer>> levelOrderBottom(TreeNode root) {
        List<List<Integer>> res = new ArrayList<>();
        Queue<TreeNode> que = new ArrayDeque<>();
        if (root == null) {
            return res;
        }
        que.offer(root);
        int size = 0;
        while (!que.isEmpty()) {
            size = que.size();
            List<Integer> list = new ArrayList<>();
            while (size > 0) {
                TreeNode cur = que.poll();
                list.add(cur.val);
                if (cur.left != null) {
                    que.offer(cur.left);
                }
                if (cur.right != null) {
                    que.offer(cur.right);
                }

                size--;
            }
            res.add(list);
        }
        Collections.reverse(res);
        return res;
    }

输出二叉树的右视图节点

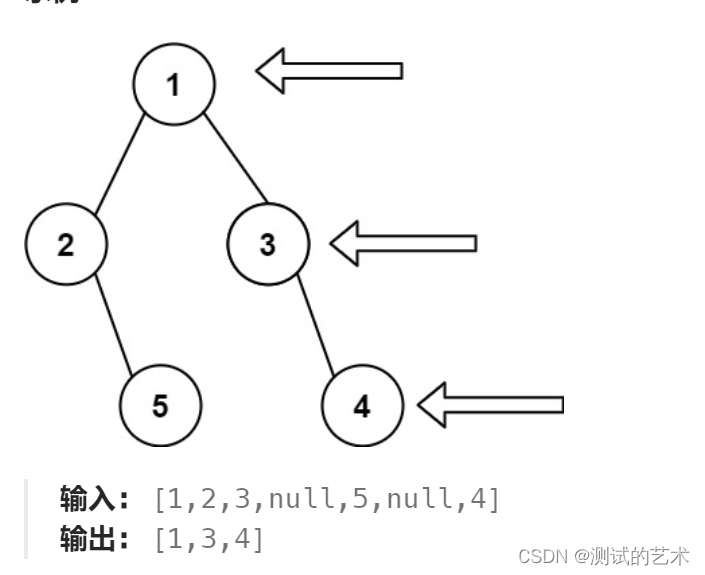
在这里插入图片描述
实现思路：层次遍历二叉树，记录二叉树每一层的节点数，到达该层最后一个节点时，将其加入到结果集即可

public List<Integer> rightSideView(TreeNode root) {
        List<Integer> res = new ArrayList<>();
        if(root == null){
            return res;
        }
        Queue<TreeNode> que = new ArrayDeque<>();
        que.offer(root);
        int size = 0;
        while(!que.isEmpty()){
            size = que.size();
            while(size>0){
                TreeNode cur = que.poll();
                //遍历到该层最后一个节点
                if(size == 1){
                    res.add(cur.val);
                }
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
                size--;
            }
        }
        return res;
    }

二叉树每层的平均值

在这里插入图片描述
实现思路：层次遍历二叉树，计算每一层的平均值，注意：每层数之和及平均值的数据类型

public List<Double> averageOfLevels(TreeNode root) {
        List<Double> res = new ArrayList<>();
        Queue<TreeNode> que = new ArrayDeque<>();
        if(root == null){
            return res;
        }
        int size = 0;
        que.offer(root);
        while(!que.isEmpty()){
            size = que.size();
            double sum = 0;
            for (int i = 0; i < size; i++) {
                TreeNode cur = que.poll();
                sum += cur.val;
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
            }
            res.add(sum/size);
        }
        return res;
    }

N叉树的·层次遍历

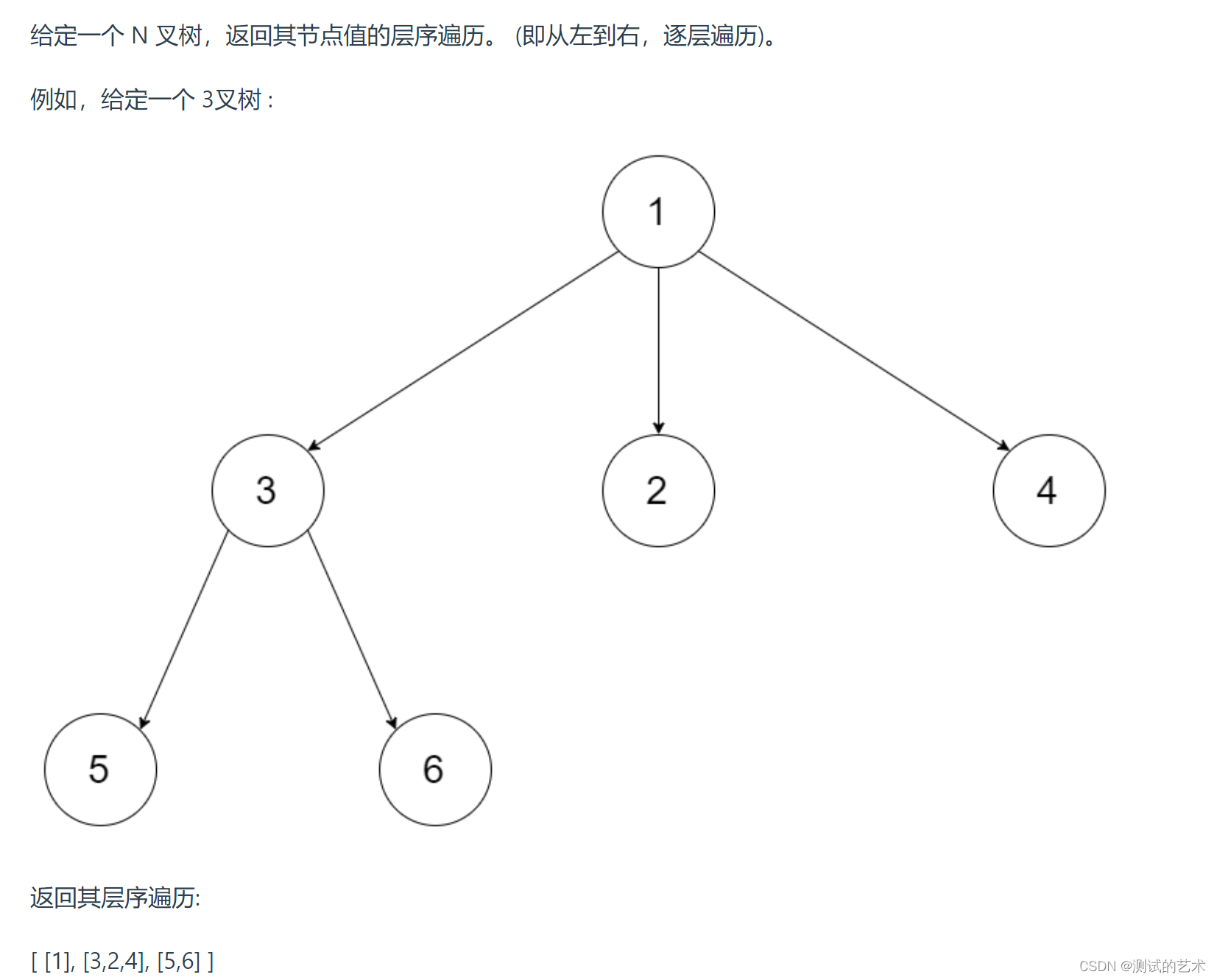
在这里插入图片描述

class Node {
    public int val;
    public List<Node> children;

    public Node() {
    }

    public Node(int _val) {
        val = _val;
    }

    public Node(int _val, List<Node> _children) {
        val = _val;
        children = _children;
    }
};

public class LevelOrderNode {
    public List<List<Integer>> levelOrder(Node root) {
        List<List<Integer>> res = new ArrayList<>();
        if (root == null) {
            return res;
        }
        Queue<Node> que = new ArrayDeque<>();
        que.offer(root);
        while (!que.isEmpty()) {
            int size = que.size();
            List<Integer> list = new ArrayList<>();
            for (int i = 0; i < size; i++) {
                Node cur = que.poll();
                list.add(cur.val);
                List<Node> children = cur.children;
                if (children == null || children.size() == 0) {
                    continue;
                }
                for (Node child : children) {
                    if (child != null) {
                        que.offer(child);
                    }
                }
            }
            res.add(list);
        }
        return res;
    }

}

在二叉树的每一行中找最大值

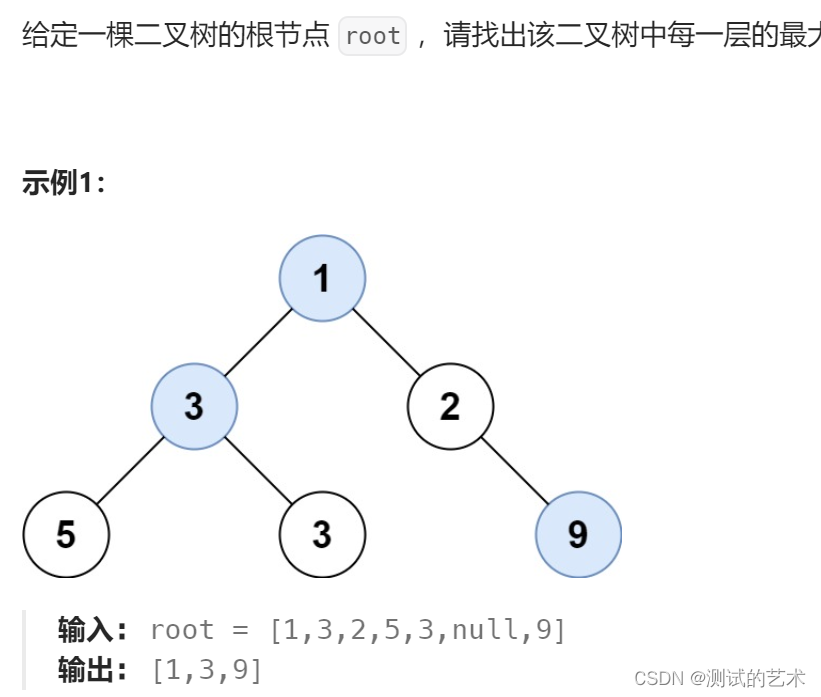
在这里插入图片描述
实现思路：层次遍历二叉树，记录每一层的最大值

public List<Integer> largestValues(TreeNode root) {
        List<Integer> res = new ArrayList<>();
        if(root == null){
            return res;
        }
        Queue<TreeNode> que = new ArrayDeque<>();
        int size = 0;
        que.offer(root);
        while(!que.isEmpty()){
            size = que.size();
            int max = Integer.MIN_VALUE;
            while(size>0) {
                TreeNode cur = que.poll();
                max = Math.max(max,cur.val);
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
                size--;
            }
            res.add(max);
        }
        return res;
    }

填充每个节点的下一个右侧节点指针

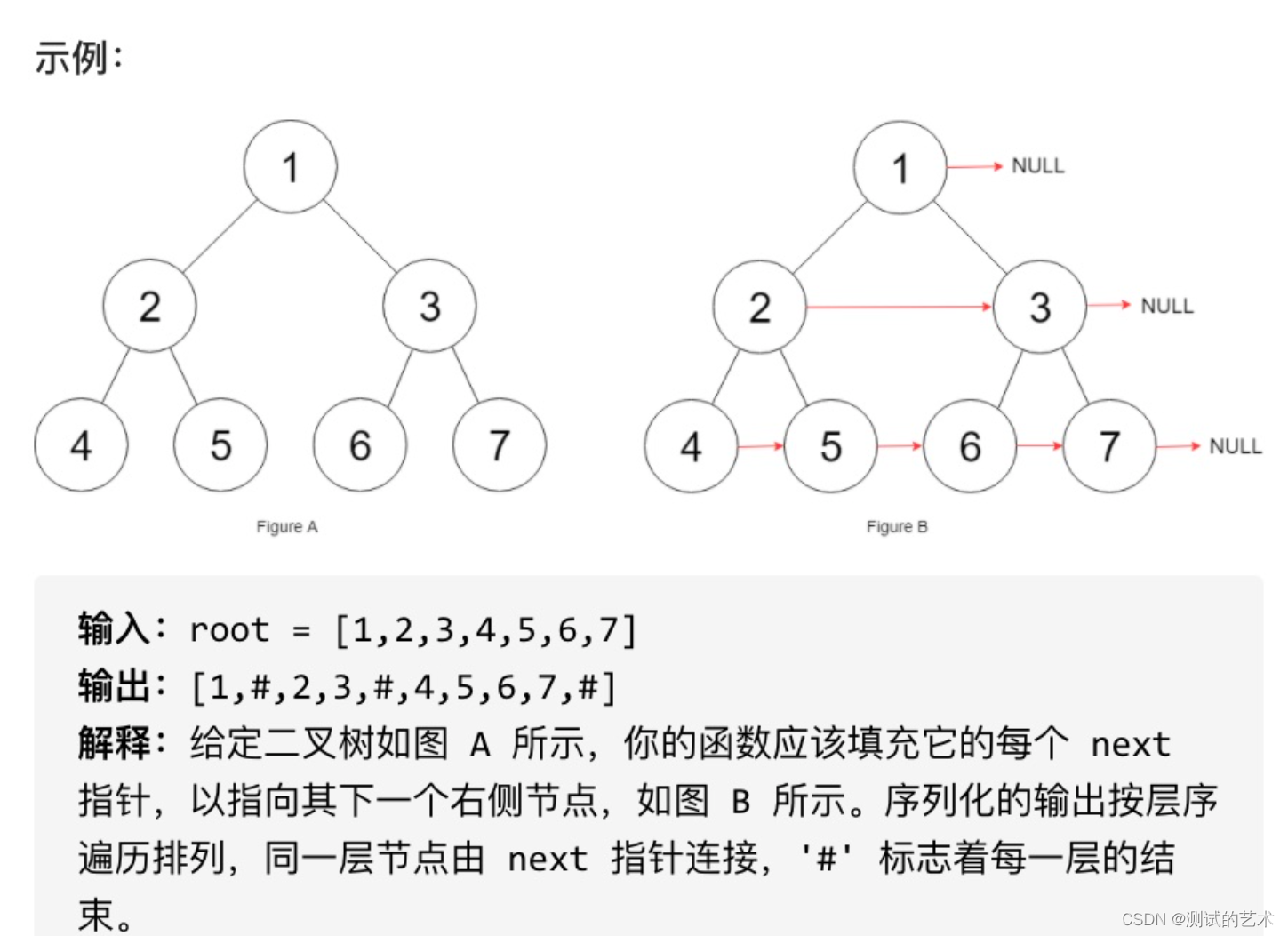
在这里插入图片描述
实现思路：层次遍历二叉树，记录每层元素个数，遍历每层元素，元素个数大于1时，使其next指针指向队头元素；元素个数为1时，使其next指针指向null即可

class NodeText {
    public int val;
    public NodeText left;
    public NodeText right;
    public NodeText next;

    public NodeText() {}

    public NodeText(int _val) {
        val = _val;
    }

    public NodeText(int _val, NodeText _left, NodeText _right, NodeText _next) {
        val = _val;
        left = _left;
        right = _right;
        next = _next;
    }
};
public class Connect {
    public NodeText connect(NodeText root) {
        Queue<NodeText> que = new ArrayDeque<>();
        if(root == null){
            return root;
        }
        que.offer(root);
        while(!que.isEmpty()){
            int size = que.size();
            while(size>0){
                NodeText cur = que.poll();
                if(size>1){
                    NodeText temp = que.peek();
                    cur.next = temp;
                }
                else{
                    cur.next =null;
                }
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
                size--;
            }
        }
        return root;
    }
}

求二叉树的最大深度

在这里插入图片描述
实现思路：层次遍历二叉树，记录树的层数

public int maxDepth(TreeNode root) {
        int maxDepth = 0;
        int size = 0;
        if(root == null){
            return 0;
        }
        Queue<TreeNode> que = new ArrayDeque<>();
        que.offer(root);
        while(!que.isEmpty()){
            maxDepth++;
            size = que.size();
            while(size>0){
                TreeNode cur = que.poll();
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
                size--;
            }
        }
        return maxDepth;
    }

求二叉树的最小深度

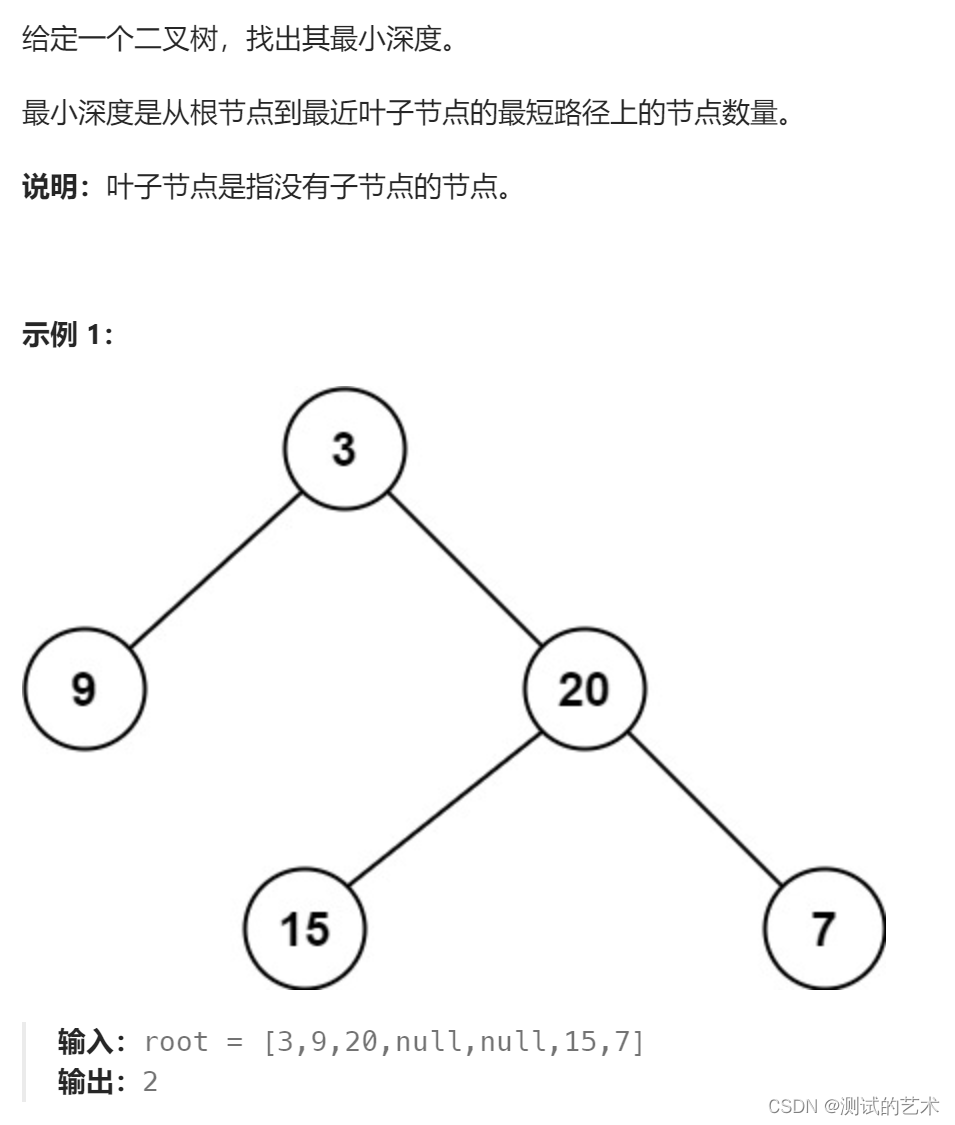
在这里插入图片描述

实现思路：层次遍历二叉树，当某个节点的左右孩子均为null时，输出该节点所在层数，及就是树的最小深度

public int minDepth(TreeNode root) {
        if (root == null) {
            return 0;
        }
        int deep = 0;
        int size = 0;
        Queue<TreeNode> que = new ArrayDeque<>();
        que.offer(root);
        while (!que.isEmpty()) {
            deep++;
            size = que.size();
            while (size > 0) {
                TreeNode cur = que.poll();
                if (cur.left == null && cur.right == null) {
                    return deep;
                } else {
                    if (cur.left != null) {
                        que.offer(cur.left);
                    }
                    if (cur.right != null) {
                        que.offer(cur.right);
                    }
                }
                size--;
            }
        }
        return deep;
    }

反转二叉树（掌握递归遍历）

实现方法：使用前序遍历、后序遍历及其层次遍历均可实现
前序遍历实现方法：遍历中间节点，中间节点的左右孩子交换位置即可
后序遍历实现方法：遍历的左子树和右子树，交换中间节点左右孩子交换位置即可
层次遍历：遍历每一层的各个节点，反转各个节点的左右孩子即可

public class InvertTree extends TreeNode {
    public TreeNode invertTree(TreeNode root) {
        if(root == null){
            return null;
        }
//        //前序
//        swap(root);
//        invertTree(root.left);
//        invertTree(root.right);

//        //后序
//        invertTree(root.left);
//        invertTree(root.right);
//        swap(root);
        //层次遍历--迭代
        Queue<TreeNode> que = new ArrayDeque<>();
        int size = 0;
        que.offer(root);
        while(!que.isEmpty()){
            size = que.size();
            while(size>0){
                TreeNode cur = que.poll();
                swap(cur);
                if(cur.left!=null){
                    que.offer(cur.left);
                }
                if(cur.right!=null){
                    que.offer(cur.right);
                }
                size--;
            }
        }

        return root;
    }
    public void swap(TreeNode root){
        TreeNode temp = root.left;
        root.left = root.right;
        root.right = temp;
    }
}

对称二叉树

递归实现：
递归三部曲

确定递归函数的参数和返回值
因为我们要比较的是根节点的两个子树是否是相互翻转的，进而判断这个树是不是对称树，所以要比较的是两个树，参数自然也是左子树节点和右子树节点。
返回值自然是bool类型。
代码如下：
bool compare(TreeNode* left, TreeNode* right)
确定终止条件
要比较两个节点数值相不相同，首先要把两个节点为空的情况弄清楚！否则后面比较数值的时候就会操作空指针了。
节点为空的情况有：（注意我们比较的其实不是左孩子和右孩子，所以如下我称之为左节点右节点）

左节点为空，右节点不为空，不对称，return false
左不为空，右为空，不对称 return false
左右都为空，对称，返回true
左右都不为空，比较节点数值，不相同就return false
代码如下：

if (left == NULL && right != NULL) return false;
else if (left != NULL && right == NULL) return false;
else if (left == NULL && right == NULL) return true;
else if (left->val != right->val) return false; // 注意这里我没有使用else

注意上面最后一种情况，我没有使用else，而是else if，因为我们把以上情况都排除之后，剩下的就是左右节点都不为空，且数值相同的情况。
3. 确定单层递归的逻辑
此时才进入单层递归的逻辑，单层递归的逻辑就是处理左右节点都不为空，且数值相同的情况。

比较二叉树外侧是否对称：传入的是左节点的左孩子，右节点的右孩子。
比较内侧是否对称：传入左节点的右孩子，右节点的左孩子。

如果左右都对称就返回true ，有一侧不对称就返回false 。
实现过程：

class Solution {
    public boolean isSymmetric(TreeNode root) {
        if (root == null) {
            return true;
        }
        return compare(root.left,root.right);
    }

    //递归实现
    public boolean compare(TreeNode left, TreeNode right) {
        if (left == null && right != null) return false;
        else if (left != null && right == null) return false;
        else if (left != null && right != null && left.val != right.val) return false;
        else if (left == null && right == null) return true;
        boolean inside = compare(left.left, right.right);
        boolean outside = compare(left.right, right.left);
        return inside && outside;
    }
}