10.2 矩形覆盖

题目链接

牛客网

题目描述

我们可以用 2*1 的小矩形横着或者竖着去覆盖更大的矩形。请问用 n 个 2*1 的小矩形无重叠地覆盖一个 2*n 的大矩形，总共有多少种方法？

解题思路

当 n 为 1 时，只有一种覆盖方法：

当 n 为 2 时，有两种覆盖方法：

要覆盖 2*n 的大矩形，可以先覆盖 2*1 的矩形，再覆盖 2*(n-1) 的矩形；或者先覆盖 2*2 的矩形，再覆盖 2*(n-2) 的矩形。而覆盖 2*(n-1) 和 2*(n-2) 的矩形可以看成子问题。该问题的递推公式如下：

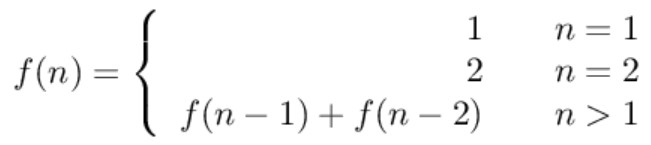
public int rectCover(int n) {
    if (n <= 2)
        return n;
    int pre2 = 1, pre1 = 2;
    int result = 0;
    for (int i = 3; i <= n; i++) {
        result = pre2 + pre1;
        pre2 = pre1;
        pre1 = result;
    }
    return result;
}

原文链接