目录
一、概念
二、实现
2.1 - BST.h
2.2 - test.cpp
三、应用
四、性能分析
一、概念
二叉搜索树(BST,Binary Search Tree),又称二叉排序树或二叉查找树。
二叉搜索树是一棵二叉树,可以为空;如果不为空,满足以下性质:
-
非空左子树的所有键值小于其根节点的键值
-
非空右子树的所有键值大于其根节点的键值
-
左、右子树本身也都是二叉搜索树。

二、实现
2.1 - BST.h
#pragma once
#include <stack>
#include <iostream>
template<class K>
struct BSTNode
{
BSTNode<K>* _left;
BSTNode<K>* _right;
K _key;
BSTNode(const K& key = K())
: _left(nullptr), _right(nullptr), _key(key)
{ }
};
template<class K>
class BST
{
typedef BSTNode<K> BSTNode;
public:
/*---------- 构造函数和析构函数 ----------*/
// 默认构造函数
BST() : _root(nullptr)
{ }
// 拷贝构造函数(实现深拷贝)
BST(const BST<K>& t)
{
_root = Copy(t._root);
}
// 析构函数
~BST()
{
Destroy(_root);
}
/*---------- 赋值运算符重载 ----------*/
// 利用上面写好的拷贝构造函数实现深拷贝
BST<K>& operator=(BST<K> tmp)
{
std::swap(_root, tmp._root);
return *this;
}
/*---------- 插入 ----------*/
// 非递归写法
bool Insert(const K& key)
{
if (_root == nullptr)
{
_root = new BSTNode(key);
return true;
}
BSTNode* parent = nullptr;
BSTNode* cur = _root;
while (cur)
{
parent = cur;
if (key < cur->_key)
cur = cur->_left;
else if (key > cur->_key)
cur = cur->_right;
else
return false;
}
cur = new BSTNode(key);
if (key < parent->_key)
parent->_left = cur;
else
parent->_right = cur;
return true;
}
// 递归(Recursion)写法
bool InsertRecursion(const K& key)
{
return _InsertRecursion(_root, key);
}
/*---------- 删除 ----------*/
// 非递归写法
bool Erase(const K& key)
{
BSTNode* parent = nullptr;
BSTNode* cur = _root;
while (cur)
{
if (key < cur->_key)
{
parent = cur;
cur = cur->_left;
}
else if (key > cur->_key)
{
parent = cur;
cur = cur->_right;
}
else
{
if (cur->_left == nullptr) // 左子树为空
{
if (parent == nullptr) // 或者 cur == _root
{
_root = cur->_right;
}
else
{
if (parent->_left == cur)
parent->_left = cur->_right;
else
parent->_right = cur->_right;
}
}
else if (cur->_right == nullptr) // 右子树为空
{
if (parent == nullptr) // 或者 cur == _root
{
_root = cur->_left;
}
else
{
if (parent->_left == cur)
parent->_left = cur->_left;
else
parent->_right = cur->_left;
}
}
else // 左、右子树非空
{
// 找到左子树中关键字最大的节点(或者找到右子树中关键字最小的节点)
BSTNode* parentLeftMax = cur;
BSTNode* leftMax = cur->_left;
while (leftMax->_right)
{
parentLeftMax = leftMax;
leftMax = leftMax->_right;
}
// 让 leftMax 指向的节点代替 cur 指向的节点,然后删除 leftMax 指向的节点,
// 这样就转换成了上面的情况
std::swap(cur->_key, leftMax->_key);
if (parentLeftMax->_left == leftMax) // 或者 parentLeftMax == cur
parentLeftMax->_left = leftMax->_left;
else
parentLeftMax->_right = leftMax->_left;
cur = leftMax;
}
delete cur;
return true;
}
}
return false;
}
// 递归写法
bool EraseRecursion(const K& key)
{
return _EraseRecursion(_root, key);
}
/*---------- 查找 ----------*/
// 非递归写法
bool Search(const K& key) const
{
BSTNode* cur = _root;
while (cur)
{
if (key < cur->_key)
cur = cur->_left;
else if (key > cur->_key)
cur = cur->_right;
else
return true;
}
return false;
}
// 递归写法
bool SearchRecursion(const K& key) const
{
return _SearchRecursion(_root, key);
}
/*---------- 中序遍历 ----------*/
// 非递归写法
void InOrder() const
{
std::stack<BSTNode*> st;
BSTNode* cur = _root;
while (cur || !st.empty())
{
while (cur)
{
st.push(cur);
cur = cur->_left;
}
BSTNode* top = st.top();
st.pop();
std::cout << top->_key << " ";
cur = top->_right;
}
std::cout << std::endl;
}
// 递归写法
void InOrderRecursion() const
{
_InOrderRecursion(_root);
std::cout << std::endl;
}
private:
BSTNode* Copy(BSTNode* root)
{
if (root == nullptr)
return nullptr;
BSTNode* copyRoot = new BSTNode(root->_key);
copyRoot->_left = Copy(root->_left);
copyRoot->_right = Copy(root->_right);
return copyRoot;
}
void Destroy(BSTNode*& root)
{
// 【注意:root 为 _root 或者某个节点的左或右指针的引用】
if (root == nullptr)
return;
Destroy(root->_left);
Destroy(root->_right);
delete root;
root = nullptr;
}
bool _InsertRecursion(BSTNode*& root, const K& key)
{
// 【注意:root 为 _root 或者某个节点的左或右指针的引用】
if (root == nullptr)
{
root = new BSTNode(key);
return true;
}
if (key < root->_key)
_InsertRecursion(root->_left, key);
else if (key > root->_key)
_InsertRecursion(root->_right, key);
else
return false;
}
bool _EraseRecursion(BSTNode*& root, const K& key)
{
// 【注意:root 为 _root 或者某个节点的左或右指针的引用】
if (root == nullptr)
return false;
if (key < root->_key)
_EraseRecursion(root->_left, key);
else if (key > root->_key)
_EraseRecursion(root->_right, key);
else
{
BSTNode* tmp = root;
if (root->_left == nullptr)
root = root->_right;
else if (root->_right == nullptr)
root = root->_left;
else
{
BSTNode* leftMax = root->_left;
while (leftMax->_right)
{
leftMax = leftMax->_right;
}
std::swap(leftMax->_key, root->_key);
return _EraseRecursion(root->_left, key);
}
delete tmp;
return true;
}
}
bool _SearchRecursion(BSTNode* root, const K& key) const
{
if (root == nullptr)
return false;
if (key < root->_key)
_SearchRecursion(root->_left, key);
else if (key > root->_key)
_SearchRecursion(root->_right, key);
else
return true;
}
void _InOrderRecursion(BSTNode* root) const
{
if (root == nullptr)
return;
_InOrderRecursion(root->_left);
std::cout << root->_key << " ";
_InOrderRecursion(root->_right);
}
private:
BSTNode* _root;
};2.2 - test.cpp
#include "BST.h"
using namespace std;
void TestBST1()
{
int arr[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };
BST<int> t1;
for (auto e : arr)
{
t1.Insert(e);
}
t1.InOrder(); // 1 3 4 6 7 8 10 13 14
BST<int> t2(t1);
t2.InOrder(); // 1 3 4 6 7 8 10 13 14
BST<int> t3;
t3 = t1;
t1.InOrder(); // 1 3 4 6 7 8 10 13 14
// 左子树为空
t1.Erase(4);
t1.InOrder(); // 1 3 6 7 8 10 13 14
t1.Erase(6);
t1.InOrder(); // 1 3 7 8 10 13 14
// 右子树为空
t1.Erase(14);
t1.InOrder(); // 1 3 7 8 10 13
// 左、右子树非空
t1.Erase(8);
t1.InOrder(); // 1 3 7 10 13
cout << t1.Search(8) << endl; // 0
cout << t1.Search(7) << endl; // 1
}
void TestBST2()
{
int arr[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };
BST<int> t;
for (auto e : arr)
{
t.InsertRecursion(e);
}
t.InOrderRecursion(); // 1 3 4 6 7 8 10 13 14
// 左子树为空
t.EraseRecursion(4);
t.InOrderRecursion(); // 1 3 6 7 8 10 13 14
t.EraseRecursion(6);
t.InOrderRecursion(); // 1 3 7 8 10 13 14
// 右子树为空
t.EraseRecursion(14);
t.InOrderRecursion(); // 1 3 7 8 10 13
// 左、右子树非空
t.EraseRecursion(8);
t.InOrderRecursion(); // 1 3 7 10 13
cout << t.SearchRecursion(8) << endl; // 0
cout << t.SearchRecursion(7) << endl; // 1
}
int main()
{
TestBST1();
TestBST2();
return 0;
}
三、应用
-
K 模型:结构体中只需要存储关键码 key,关键码即为需要搜索到的值。
例如,要判断一个单词是否拼写正确,我们首先把词库中的每个单词作为 key,构建一棵二叉搜索树,然后在这棵二叉搜索树中检索单词是否存在,若存在则表明拼写正确,不存在则表明拼写错误。
-
KV 模型:每个关键码 key,都有与之对应的值 value,即 <key, value> 的键值对。
这种模型在现实生活中也很常见:
-
比如英汉词典就是英文与中文的对应关系,通过英文可以快速找到与其对应的中文,英文单词与其对应的中文 <word, Chinese> 就构成一种键值对。
-
再比如统计单词次数,统计成功后,给定单词就可以快速找到其出现的次数,单词与其出现的次数 <word, count> 就构成一种键值对。
BST.h:#pragma once #include <iostream> template<class K, class V> struct BSTNode { BSTNode<K, V>* _left; BSTNode<K, V>* _right; K _key; V _value; BSTNode(const K& key = K(), const V& value = V()) : _left(nullptr), _right(nullptr), _key(key), _value(value) { } }; template<class K, class V> class BST { typedef BSTNode<K, V> BSTNode; public: BST() : _root(nullptr) { } BST(const BST<K, V>& t) { _root = Copy(t._root); } ~BST() { Destroy(_root); } BST<K, V>& operator=(BST<K, V> tmp) { std::swap(_root, tmp._root); return *this; } bool Insert(const K& key, const V& value) { return _Insert(_root, key, value); } bool Erase(const K& key) { return _Erase(_root, key); } BSTNode* Search(const K& key) const { return _Search(_root, key); } void InOrder() const { _InOrder(_root); std::cout << std::endl; } private: BSTNode* Copy(BSTNode* root) { if (root == nullptr) return nullptr; BSTNode* copyRoot = new BSTNode(root->_key, root->_value); copyRoot->_left = Copy(root->_left); copyRoot->_right = Copy(root->_right); return copyRoot; } void Destroy(BSTNode*& root) { // 【注意:root 为 _root 或者某个节点的左或右指针的引用】 if (root == nullptr) return; Destroy(root->_left); Destroy(root->_right); delete root; root = nullptr; } bool _Insert(BSTNode*& root, const K& key, const V& value) { // 【注意:root 为 _root 或者某个节点的左或右指针的引用】 if (root == nullptr) { root = new BSTNode(key, value); return true; } if (key < root->_key) _Insert(root->_left, key, value); else if (key > root->_key) _Insert(root->_right, key, value); else return false; } bool _Erase(BSTNode*& root, const K& key) { // 【注意:root 为 _root 或者某个节点的左或右指针的引用】 if (root == nullptr) return false; if (key < root->_key) _Erase(root->_left, key); else if (key > root->_key) _Erase(root->_right, key); else { BSTNode* tmp = root; if (root->_left == nullptr) root = root->_right; else if (root->_right == nullptr) root = root->_left; else { BSTNode* leftMax = root->_left; while (leftMax->_right) { leftMax = leftMax->_right; } std::swap(leftMax->_key, root->_key); return _Erase(root->_left, key); } delete tmp; return true; } } BSTNode* _Search(BSTNode* root, const K& key) const { if (root == nullptr) return nullptr; if (key < root->_key) _Search(root->_left, key); else if (key > root->_key) _Search(root->_right, key); else return root; } void _InOrder(BSTNode* root) const { if (root == nullptr) return; _InOrder(root->_left); std::cout << root->_key << " : " << root->_value << std::endl; _InOrder(root->_right); } private: BSTNode* _root; };test.cpp:#include "BST_KV.h" using namespace std; void TestBST1() { BST<string, string> t; t.Insert("insert", "插入"); t.Insert("erase", "删除"); t.Insert("search", "查找"); t.Insert("left", "左边"); t.Insert("right", "右边"); // 输入英文单词,找到对应的中文 string str; while (cin >> str) { BSTNode<string, string>* ret = t.Search(str); if (ret) cout << str << "对应的中文是:" << ret->_value << endl; else cout << "单词拼写错误,词库中没有此单词!" << endl; } } void TestBST2() { string arr[] = { "苹果", "西瓜", "苹果", "西瓜", "苹果", "苹果", "西瓜", "苹果", "香蕉", "苹果", "香蕉" }; BST<string, int> t; // 统计每种水果出现的次数 for (const auto& str : arr) { BSTNode<string, int>* ret = t.Search(str); if (ret == nullptr) t.Insert(str, 1); else ret->_value += 1; } t.InOrder(); // 苹果 : 6 // 西瓜: 3 // 香蕉 : 2 } int main() { // TestBST1(); TestBST2(); return 0; } -
四、性能分析
在二叉搜索树的插入和删除操作中,都必须先进行查找操作,所以查找的效率就代表了各个操作的性能。
对含 n 个节点的二叉搜索树,若每个节点查找的概率相等,则二叉搜树的平均查找长度是节点在二叉搜树树的深度的函数,即节点越深,比较次数越多。
但对于同一个关键码集合,如果各关键码插入的次序不同,可能得到不同结构的二叉搜索树,例如:
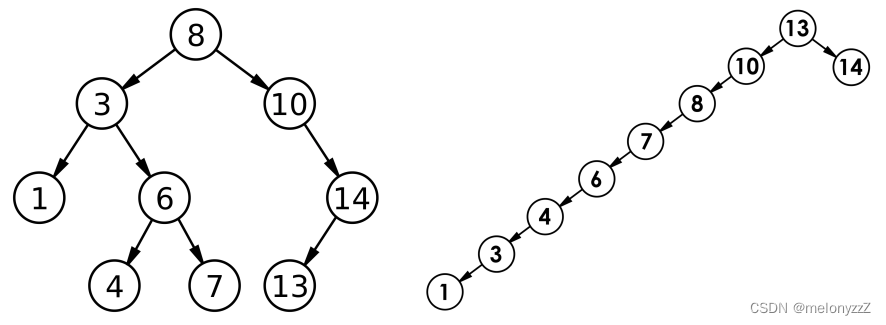
最好情况下,二叉搜索树为完全二叉树(或者接近完全二叉树),其平均比较次数为 。
最坏情况下,二叉搜索树退化为单支树(或者类似单支树),其平均比较次数为 。
如果退化成单支树,二叉搜树的性能就丢失了,那么能否改进,不论按照什么次序插入关键码,二叉搜索树的性能都能达到最优?
后续所要学习的 AVL 树和红黑树就可以解决上述问题。