文章目录
- 前言
- 一.非线性反步法
- 1.原系统对应的s函数脚本文件(仅修改模板的初始化函数、导数函数和输出函数三个部分)
- 2.控制器对应的s函数脚本文件(仅修改模板的初始化函数和输出函数两个部分)
- 3.其他参数脚本文件
- 4.输入
- 5.输出(x1和x1d对比)
- 二.滑模控制
- 1.不需要显示相轨迹就下面这个
- 跟踪效果
- 2.需要显示相轨迹
- 3.如果不想抖振这么严重,可以将符号函数改成饱和函数
前言
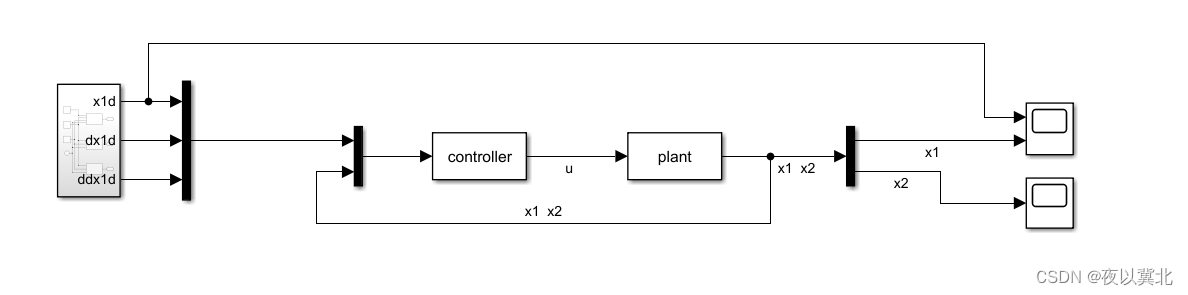
其实用s函数搭建系统就是将各个公式组表示出来,如状态变量和其导数为一组,即在属于“原系统”的s函数中修改初始化函数、导数函数和输出函数,而中间那些误差和控制器u为一组,即在属于“控制器”的s函数中修改初始化函数和输出函数;
一.非线性反步法
1.原系统对应的s函数脚本文件(仅修改模板的初始化函数、导数函数和输出函数三个部分)
function [sys,x0,str,ts,simStateCompliance] = plant(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u,pa);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 2; %系统里的连续状态变量x1,x2
sizes.NumDiscStates = 0; %系统里的离散状态变量
sizes.NumOutputs = 2; %系统里的输出变量x1,x2
sizes.NumInputs = 1; %系统里的输入变量u
sizes.DirFeedthrough = 0; %一般系统的输出不会和u有关,控制器才是和u有关的
sizes.NumSampleTimes = 1; % 至少一个,如果0个下面的ts=[]
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [0 0]; %状态变量的初始化,也可以写成其他形式[0,0]、[0;0]
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0]; %第一个零表示采样时间,第二个零表示初始量
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u,pa)
% 导数
%先从x向量中取出每个状态
x1 = x(1);
x2 = x(2);
x1dot = x2; %x1的导数
x2dot = -pa.k / pa.m *x1.^3 + 1 / pa.m * u; %x2的导数,注意要从结构体里取k.m
sys = [x1dot x2dot];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
% 更新模块的离散状态,这里是连续系统,不用填
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u)
sys = [x(1) x(2)]; %注意这里要从x取出
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
2.控制器对应的s函数脚本文件(仅修改模板的初始化函数和输出函数两个部分)
【提醒:一般只有被控制的那个原系统才有状态变量,控制器没有状态变量】
function [sys,x0,str,ts,simStateCompliance] = controller(t,x,u,flag, pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u,pa);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 0; %控制器中一般没有状态变量,而状态变量一般都在被控对象中。这也就导致了没有连续状态变量的定义,也就没有了连续状态更新的函数定义(状态方程)
sizes.NumDiscStates = 0;
sizes.NumOutputs = 1; %输出的就是u,注意是控制器的u,不是输入的u,这里可以换个符号uc
sizes.NumInputs = 5; %输入有x1、x2、x1d、dx1d、ddx1d
sizes.DirFeedthrough = 1; %有u就有直接馈入
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = []; %x0不需要定义值,因为本来就没有状态变量
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u)
% 没有状态变量不用导
sys = [];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u, pa)
% 从u里取出来先
x1d = u(1);
dx1d = u(2);
ddx1d = u(3);
x1 = u(4);
x2 = u(5);
% 从pa里取出m.k.k1.k2,一直都加pa很繁琐
k = pa.k;
m = pa.m;
k1 = pa.k1;
k2 = pa.k2;
% 用上述变量表示e
e1 = x1d - x1;
e2 = dx1d + k1 * e1 - x2;
uc = m * e1 + m * ddx1d + m * k1 * (dx1d - x2) + k * x1.^3 + m * k2 * e2;
sys = uc;
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
3.其他参数脚本文件
%把系统的外部参数设置一下
pa.k = 8;
pa.m = 1;
pa.k1 = 1;
pa.k2 = 1;
4.输入
先把x1d、dx1d、ddx1d这些画出来,然后全选中右键,选择形成系统,就可以装成一个小盒子
x1d脚本文件
function x1d = inputFcn(B,A,T,t)
x1d = B + A * sin(2 * pi / T * t);
dx1d脚本文件
function dx1d = inputFcn(A,T,t)
dx1d = 2 *pi / T * A * cos(2 * pi / T * t);
ddx1d脚本文件
function ddx1d = inputFcn(A,T,t)
ddx1d = - 2 * pi / T * 2 * pi / T * A * sin(2 * pi / T * t);

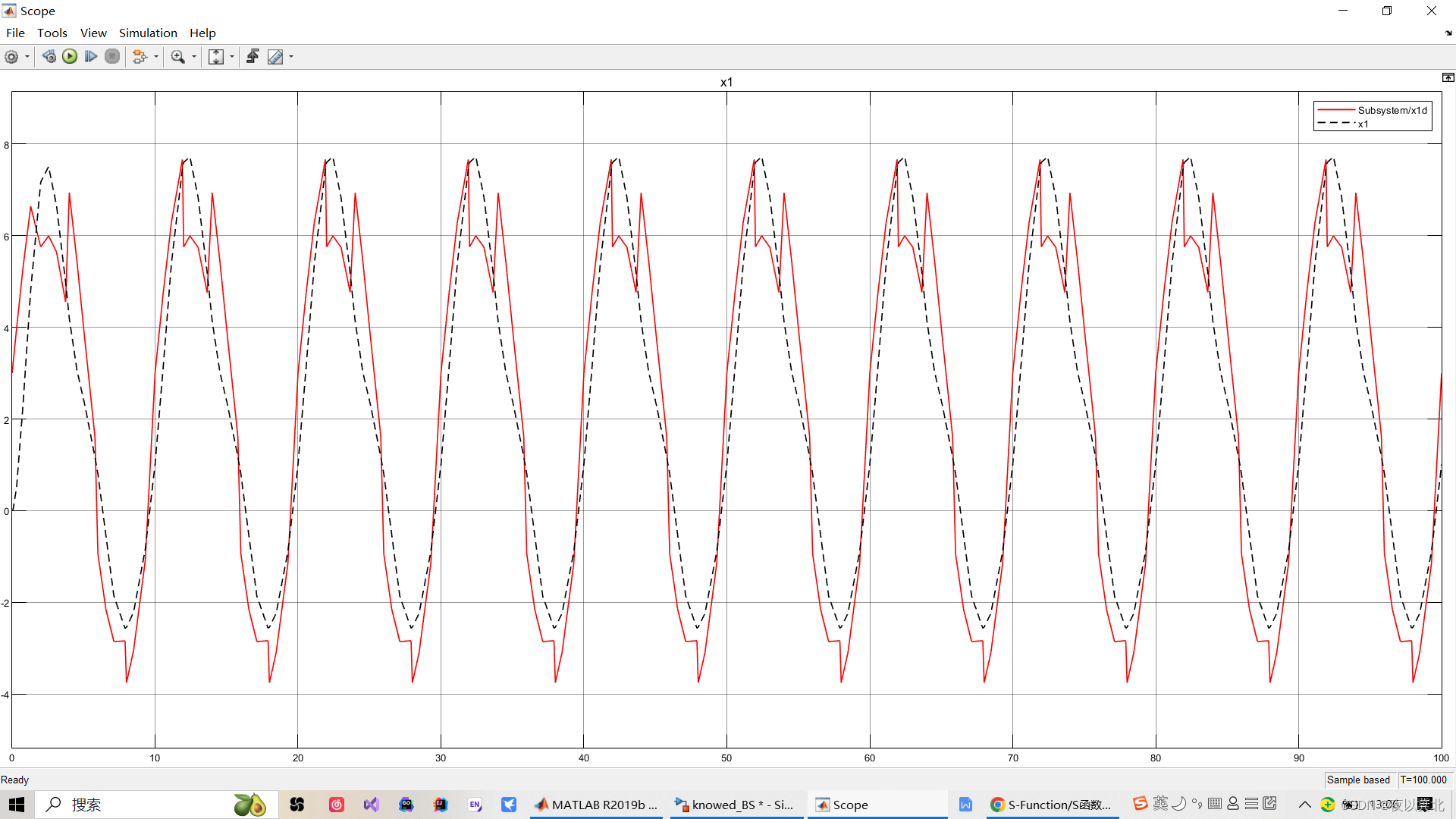
5.输出(x1和x1d对比)
二.滑模控制
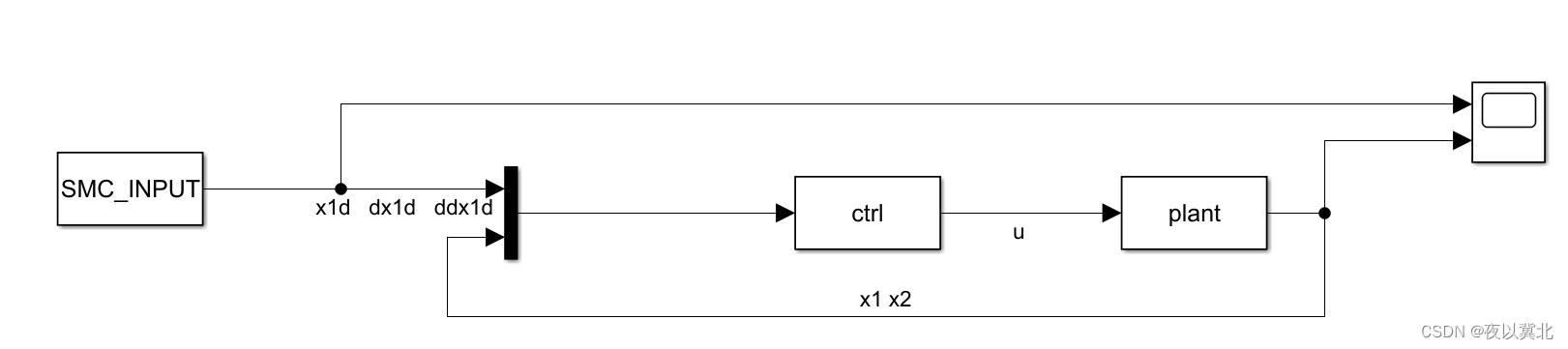
1.不需要显示相轨迹就下面这个
function [sys,x0,str,ts,simStateCompliance] = SMC_INPUT(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u,pa);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 0;
sizes.NumDiscStates = 0;
sizes.NumOutputs = 3;
sizes.NumInputs = 0;
sizes.DirFeedthrough = 0;
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [];
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u)
sys = [];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u,pa)
A=pa.A;
T=pa.T;
x1d=A*sin(2*pi/T*t);
dx1d=2*pi/T*A*cos(2*pi/T*t);
ddx1d=-2*pi/T*2*pi/T*A*sin(2*pi/T*t);
sys = [x1d dx1d ddx1d];
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
function [sys,x0,str,ts,simStateCompliance] = plant(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u,pa);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 2;
sizes.NumDiscStates = 0;
sizes.NumOutputs = 2;
sizes.NumInputs = 1;
sizes.DirFeedthrough = 0;
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [0.5 1]; %这里给一点扰动
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u,pa)
x1=x(1);
x2=x(2);
k=pa.k;
m=pa.m;
dx1=x2;
dx2=-k/m*x1.^3+1/m*u;
sys = [dx1 dx2];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u)
sys = [x(1) x(2)];
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
function [sys,x0,str,ts,simStateCompliance] = ctrl(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u,pa);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 0;
sizes.NumDiscStates = 0;
sizes.NumOutputs = 1; %u
sizes.NumInputs = 5; %x1d dx1d ddx1d x1 x2
sizes.DirFeedthrough = 1;
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [];
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u)
sys = [];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u,pa)
x1d = u(1);
dx1d = u(2);
ddx1d = u(3);
x1 = u(4);
x2 = u(5);
m = pa.m;
epsilon = pa.epsilon;
p=pa.p;
c=pa.c;
k=pa.k;
e=x1d-x1;
de=dx1d-x2;
s=c*e+de;
uc=m*(epsilon*sign(s)+p*s+c*(dx1d-x2)+ddx1d+k/m*x1.^3);
sys = uc;
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
pa.A=5;
pa.T=20;
pa.k=8;
pa.m=1;
pa.epsilon=5;
pa.p=10;
pa.c=15;
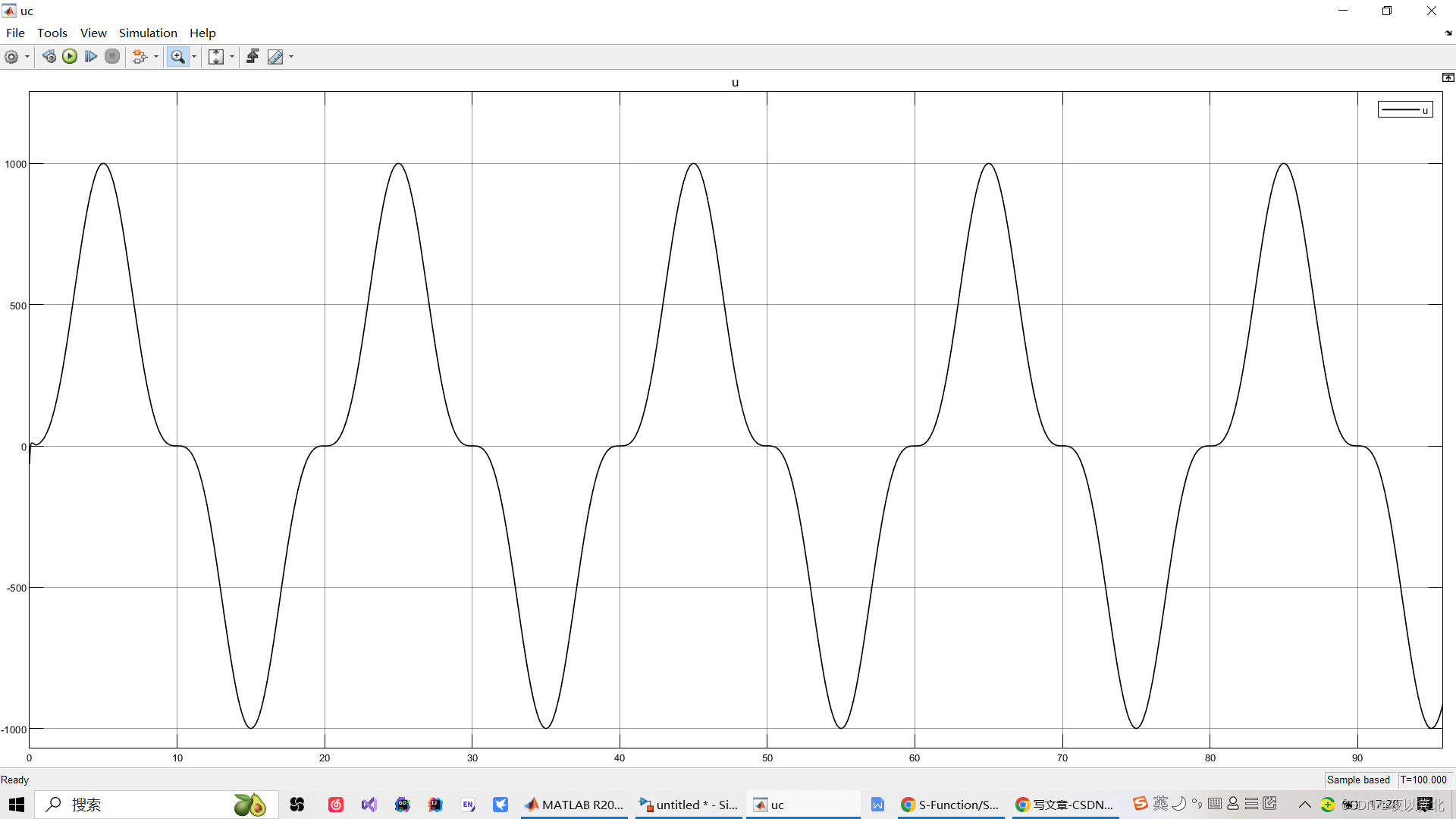
跟踪效果
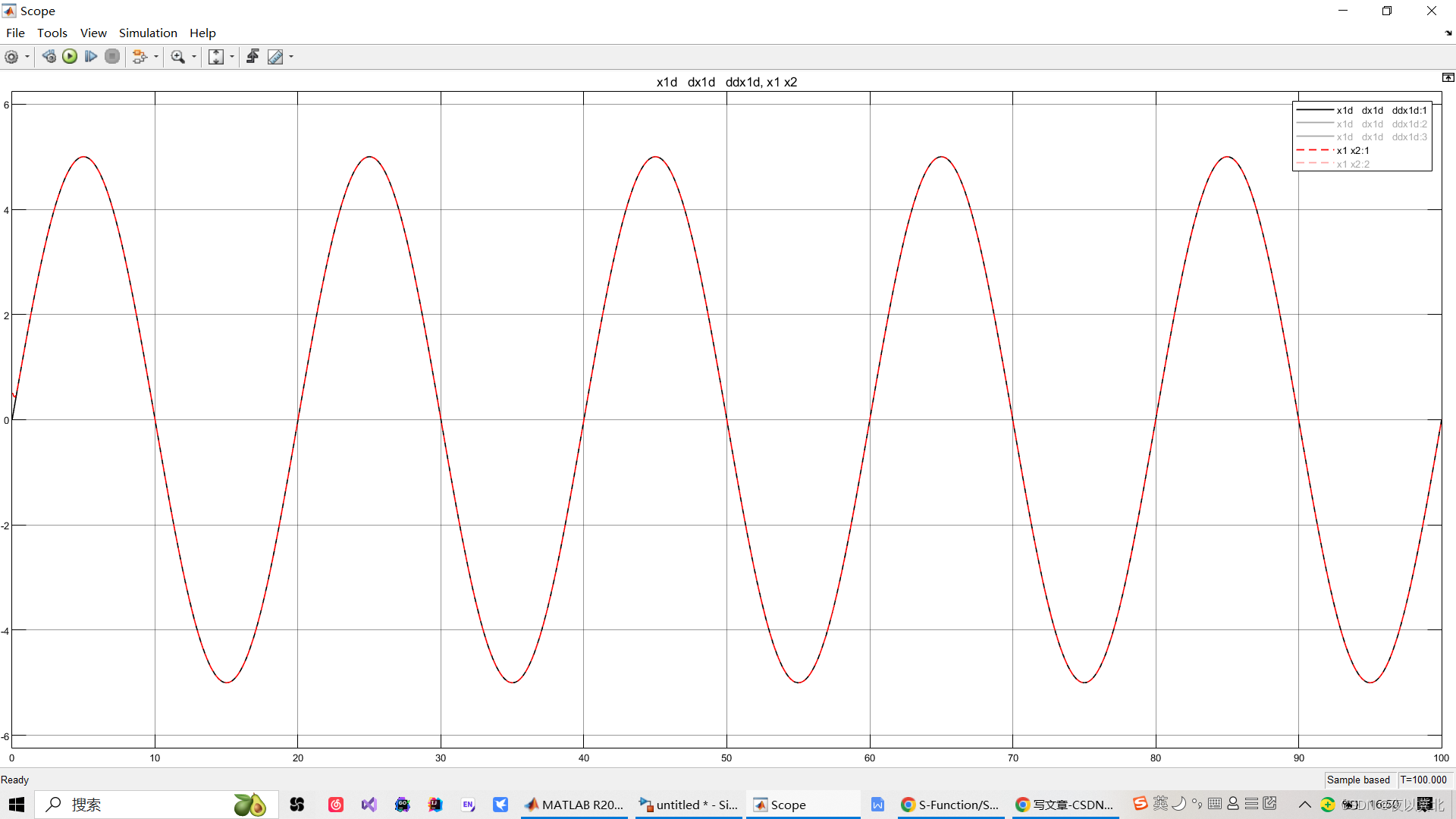
2.需要显示相轨迹

那就要显示e和de的关系,那控制器的s函数就要修改
function [sys,x0,str,ts,simStateCompliance] = ctrl(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u,pa);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 0;
sizes.NumDiscStates = 0;
sizes.NumOutputs = 3; %u e de
sizes.NumInputs = 5; %x1d dx1d ddx1d x1 x2
sizes.DirFeedthrough = 1;
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [];
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u)
sys = [];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u,pa)
x1d = u(1);
dx1d = u(2);
ddx1d = u(3);
x1 = u(4);
x2 = u(5);
m = pa.m;
epsilon = pa.epsilon;
p=pa.p;
c=pa.c;
k=pa.k;
e=x1d-x1;
de=dx1d-x2;
s=c*e+de;
uc=m*(epsilon*sign(s)+p*s+c*(dx1d-x2)+ddx1d+k/m*x1.^3);
sys = [uc e de];
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
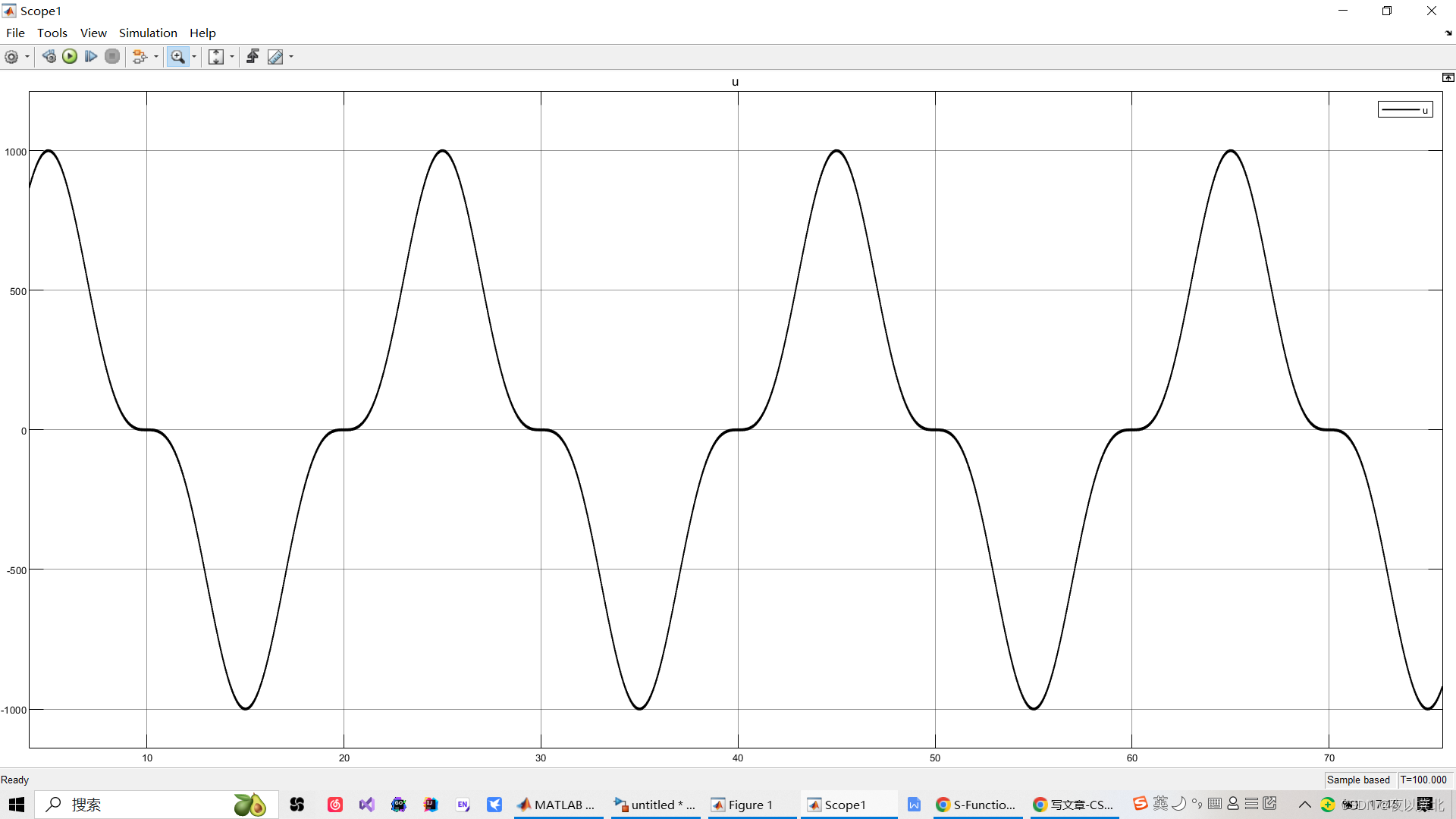
下面是画出滑模面和实际相图的关系
close all;
e=out.e;
de=out.de;
c=pa.c;
%根据s=ce+de,s=0画出理想滑模面,再画出e和de的关系画出相图(即现实的滑动轨迹)
plot(e, -c*e, 'k', e, de, 'r:','linewidth',2);
legend('s=0','s change');
xlabel('e');ylabel('de');
title('Phase portrait');

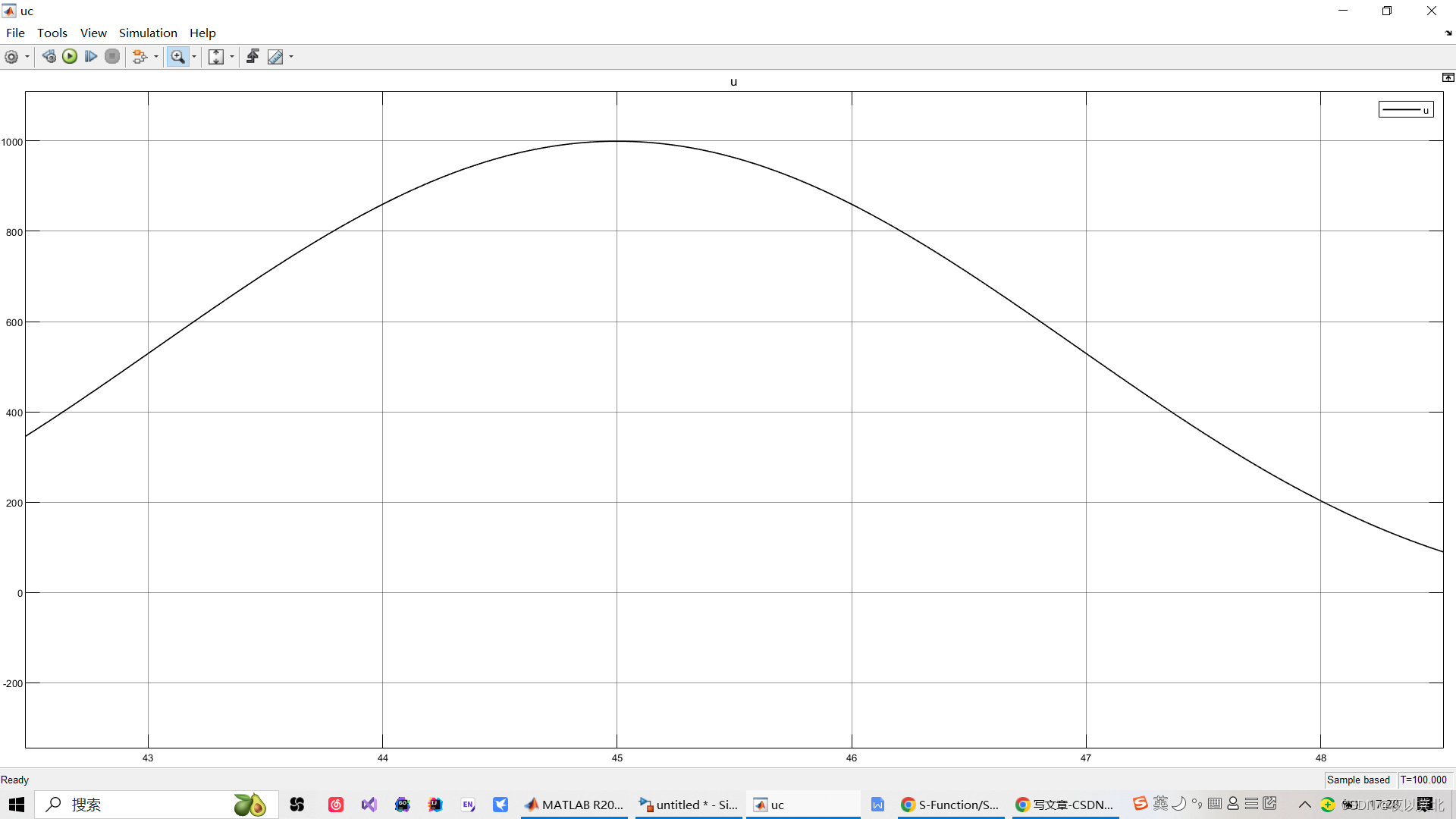
这里用的是符号函数对应的控制信号
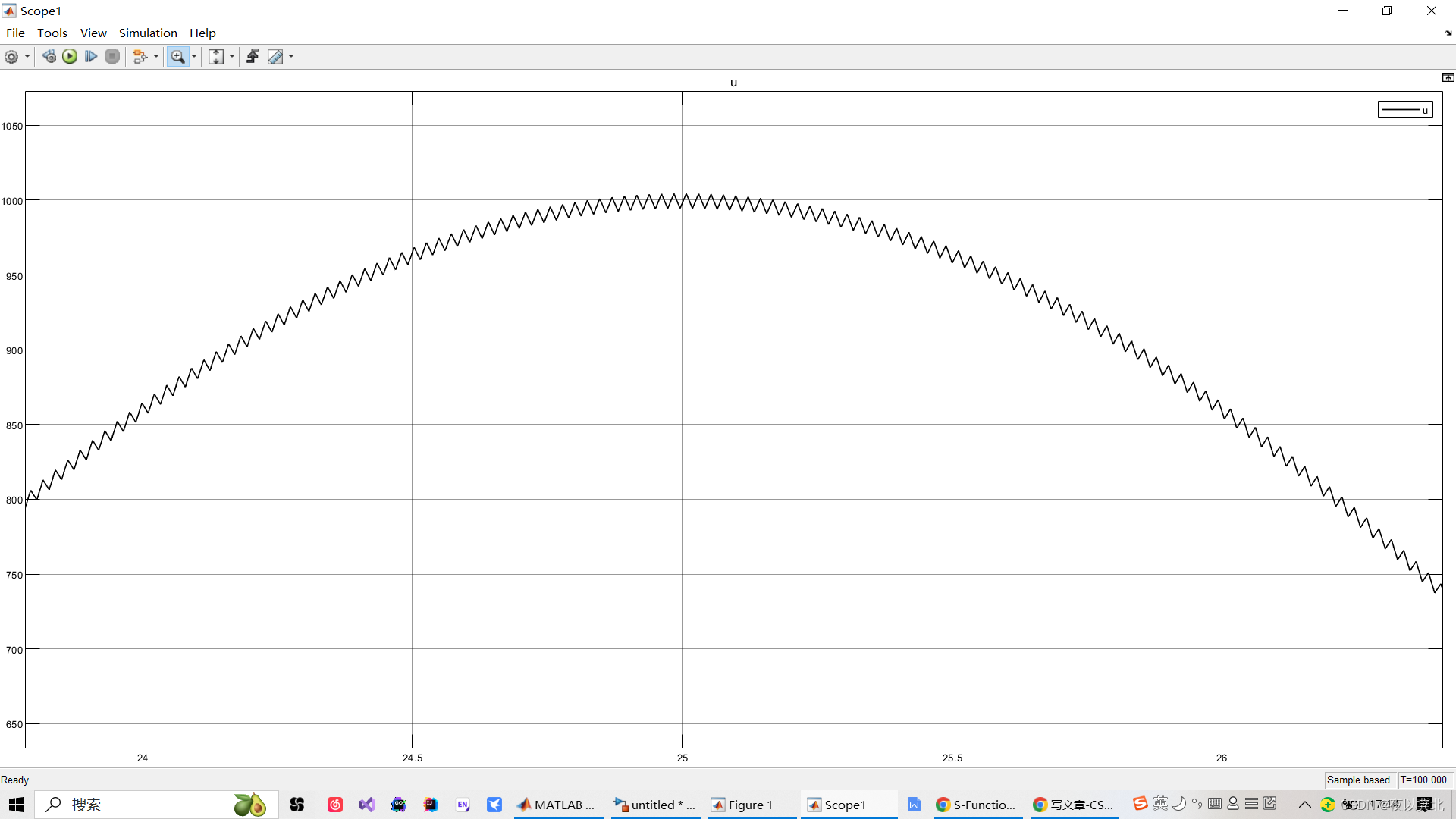
上图放大后的样子:
3.如果不想抖振这么严重,可以将符号函数改成饱和函数
那就从控制器的s函数那里改一下输出函数,这里就用一个变量M弄成if语句保留之前的符号函数了
function [sys,x0,str,ts,simStateCompliance] = ctrl(t,x,u,flag,pa)
%SFUNTMPL General MATLAB S-Function Template
% With MATLAB S-functions, you can define you own ordinary differential
% equations (ODEs), discrete system equations, and/or just about
% any type of algorithm to be used within a Simulink block diagram.
%
% The general form of an MATLAB S-function syntax is:
% [SYS,X0,STR,TS,SIMSTATECOMPLIANCE] = SFUNC(T,X,U,FLAG,P1,...,Pn)
%
% What is returned by SFUNC at a given point in time, T, depends on the
% value of the FLAG, the current state vector, X, and the current
% input vector, U.
%
% FLAG RESULT DESCRIPTION
% ----- ------ --------------------------------------------
% 0 [SIZES,X0,STR,TS] Initialization, return system sizes in SYS,
% initial state in X0, state ordering strings
% in STR, and sample times in TS.
% 1 DX Return continuous state derivatives in SYS.
% 2 DS Update discrete states SYS = X(n+1)
% 3 Y Return outputs in SYS.
% 4 TNEXT Return next time hit for variable step sample
% time in SYS.
% 5 Reserved for future (root finding).
% 9 [] Termination, perform any cleanup SYS=[].
%
%
% The state vectors, X and X0 consists of continuous states followed
% by discrete states.
%
% Optional parameters, P1,...,Pn can be provided to the S-function and
% used during any FLAG operation.
%
% When SFUNC is called with FLAG = 0, the following information
% should be returned:
%
% SYS(1) = Number of continuous states.
% SYS(2) = Number of discrete states.
% SYS(3) = Number of outputs.
% SYS(4) = Number of inputs.
% Any of the first four elements in SYS can be specified
% as -1 indicating that they are dynamically sized. The
% actual length for all other flags will be equal to the
% length of the input, U.
% SYS(5) = Reserved for root finding. Must be zero.
% SYS(6) = Direct feedthrough flag (1=yes, 0=no). The s-function
% has direct feedthrough if U is used during the FLAG=3
% call. Setting this to 0 is akin to making a promise that
% U will not be used during FLAG=3. If you break the promise
% then unpredictable results will occur.
% SYS(7) = Number of sample times. This is the number of rows in TS.
%
%
% X0 = Initial state conditions or [] if no states.
%
% STR = State ordering strings which is generally specified as [].
%
% TS = An m-by-2 matrix containing the sample time
% (period, offset) information. Where m = number of sample
% times. The ordering of the sample times must be:
%
% TS = [0 0, : Continuous sample time.
% 0 1, : Continuous, but fixed in minor step
% sample time.
% PERIOD OFFSET, : Discrete sample time where
% PERIOD > 0 & OFFSET < PERIOD.
% -2 0]; : Variable step discrete sample time
% where FLAG=4 is used to get time of
% next hit.
%
% There can be more than one sample time providing
% they are ordered such that they are monotonically
% increasing. Only the needed sample times should be
% specified in TS. When specifying more than one
% sample time, you must check for sample hits explicitly by
% seeing if
% abs(round((T-OFFSET)/PERIOD) - (T-OFFSET)/PERIOD)
% is within a specified tolerance, generally 1e-8. This
% tolerance is dependent upon your model's sampling times
% and simulation time.
%
% You can also specify that the sample time of the S-function
% is inherited from the driving block. For functions which
% change during minor steps, this is done by
% specifying SYS(7) = 1 and TS = [-1 0]. For functions which
% are held during minor steps, this is done by specifying
% SYS(7) = 1 and TS = [-1 1].
%
% SIMSTATECOMPLIANCE = Specifices how to handle this block when saving and
% restoring the complete simulation state of the
% model. The allowed values are: 'DefaultSimState',
% 'HasNoSimState' or 'DisallowSimState'. If this value
% is not speficified, then the block's compliance with
% simState feature is set to 'UknownSimState'.
% Copyright 1990-2010 The MathWorks, Inc.
%
% The following outlines the general structure of an S-function.
%
switch flag,
%%%%%%%%%%%%%%%%%%
% Initialization %
%%%%%%%%%%%%%%%%%%
case 0,
[sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes;
%%%%%%%%%%%%%%%
% Derivatives %
%%%%%%%%%%%%%%%
case 1,
sys=mdlDerivatives(t,x,u);
%%%%%%%%%%
% Update %
%%%%%%%%%%
case 2,
sys=mdlUpdate(t,x,u);
%%%%%%%%%%%
% Outputs %
%%%%%%%%%%%
case 3,
sys=mdlOutputs(t,x,u,pa);
%%%%%%%%%%%%%%%%%%%%%%%
% GetTimeOfNextVarHit %
%%%%%%%%%%%%%%%%%%%%%%%
case 4,
sys=mdlGetTimeOfNextVarHit(t,x,u);
%%%%%%%%%%%%%
% Terminate %
%%%%%%%%%%%%%
case 9,
sys=mdlTerminate(t,x,u);
%%%%%%%%%%%%%%%%%%%%
% Unexpected flags %
%%%%%%%%%%%%%%%%%%%%
otherwise
DAStudio.error('Simulink:blocks:unhandledFlag', num2str(flag));
end
% end sfuntmpl
%
%=============================================================================
% mdlInitializeSizes
% Return the sizes, initial conditions, and sample times for the S-function.
%=============================================================================
%
function [sys,x0,str,ts,simStateCompliance]=mdlInitializeSizes
%
% call simsizes for a sizes structure, fill it in and convert it to a
% sizes array.
%
% Note that in this example, the values are hard coded. This is not a
% recommended practice as the characteristics of the block are typically
% defined by the S-function parameters.
%
sizes = simsizes;
sizes.NumContStates = 0;
sizes.NumDiscStates = 0;
sizes.NumOutputs = 3; %u e de
sizes.NumInputs = 5; %x1d dx1d ddx1d x1 x2
sizes.DirFeedthrough = 1;
sizes.NumSampleTimes = 1; % at least one sample time is needed
sys = simsizes(sizes);
%
% initialize the initial conditions
%
x0 = [];
%
% str is always an empty matrix
%
str = [];
%
% initialize the array of sample times
%
ts = [0 0];
% Specify the block simStateCompliance. The allowed values are:
% 'UnknownSimState', < The default setting; warn and assume DefaultSimState
% 'DefaultSimState', < Same sim state as a built-in block
% 'HasNoSimState', < No sim state
% 'DisallowSimState' < Error out when saving or restoring the model sim state
simStateCompliance = 'UnknownSimState';
% end mdlInitializeSizes
%
%=============================================================================
% mdlDerivatives
% Return the derivatives for the continuous states.
%=============================================================================
%
function sys=mdlDerivatives(t,x,u)
sys = [];
% end mdlDerivatives
%
%=============================================================================
% mdlUpdate
% Handle discrete state updates, sample time hits, and major time step
% requirements.
%=============================================================================
%
function sys=mdlUpdate(t,x,u)
sys = [];
% end mdlUpdate
%
%=============================================================================
% mdlOutputs
% Return the block outputs.
%=============================================================================
%
function sys=mdlOutputs(t,x,u,pa)
x1d = u(1);
dx1d = u(2);
ddx1d = u(3);
x1 = u(4);
x2 = u(5);
m = pa.m;
epsilon = pa.epsilon;
p=pa.p;
c=pa.c;
k=pa.k;
e=x1d-x1;
de=dx1d-x2;
s=c*e+de;
M=pa.M;
Delta=pa.Delta;
if M==1
uc=m*(epsilon*sign(s)+p*s+c*(dx1d-x2)+ddx1d+k/m*x1.^3);
elseif M==2
if abs(s) > Delta
sat=sign(s);
else
sat=s/Delta;
end
uc=m*(epsilon*sat+p*s+c*(dx1d-x2)+ddx1d+k/m*x1.^3);
end
sys = [uc e de];
% end mdlOutputs
%
%=============================================================================
% mdlGetTimeOfNextVarHit
% Return the time of the next hit for this block. Note that the result is
% absolute time. Note that this function is only used when you specify a
% variable discrete-time sample time [-2 0] in the sample time array in
% mdlInitializeSizes.
%=============================================================================
%
function sys=mdlGetTimeOfNextVarHit(t,x,u)
sampleTime = 1; % Example, set the next hit to be one second later.
sys = t + sampleTime;
% end mdlGetTimeOfNextVarHit
%
%=============================================================================
% mdlTerminate
% Perform any end of simulation tasks.
%=============================================================================
%
function sys=mdlTerminate(t,x,u)
sys = [];
% end mdlTerminate
跟踪效果还可以
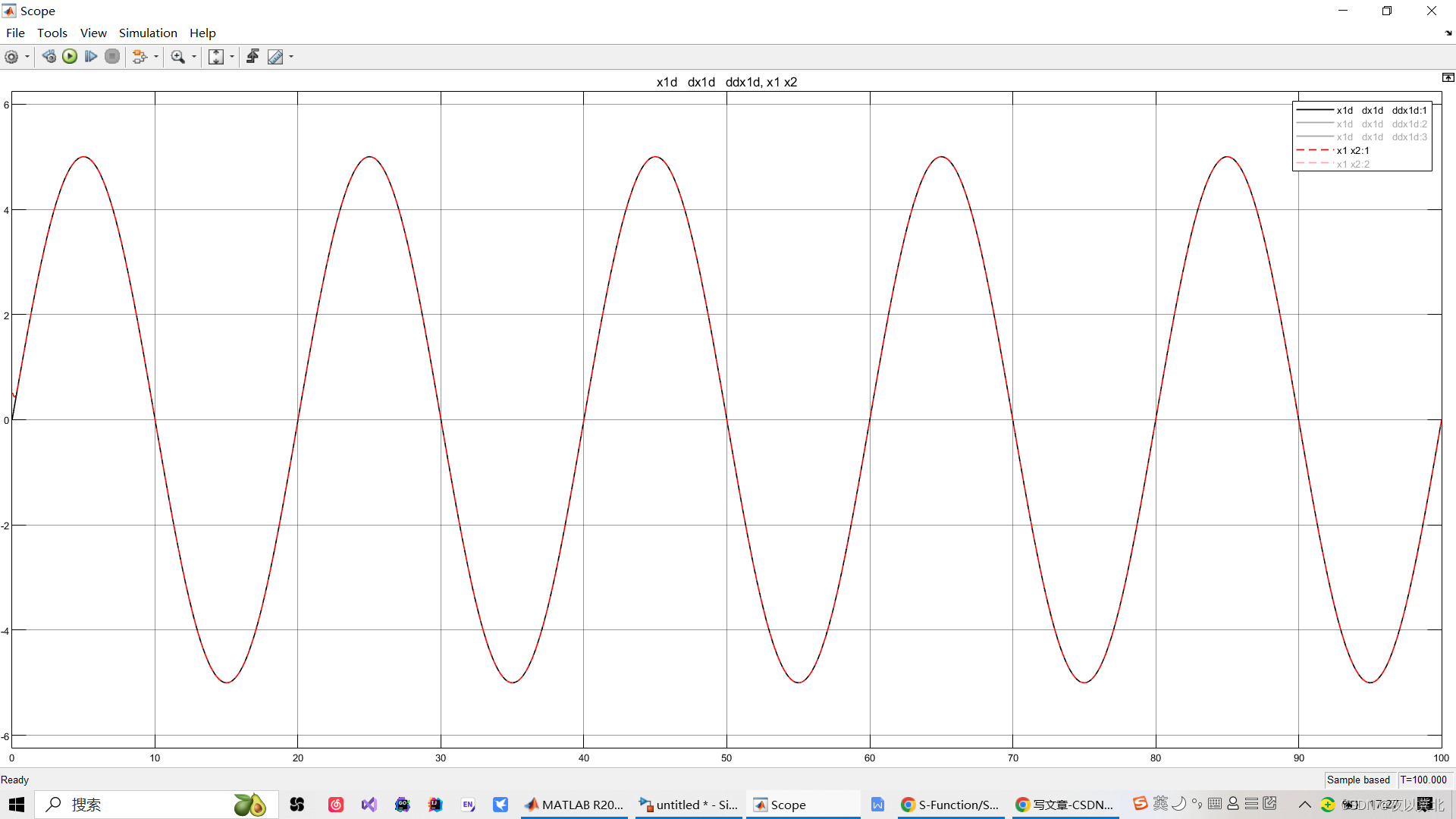
控制信号大体上不变,不过抖振现象消除得不错