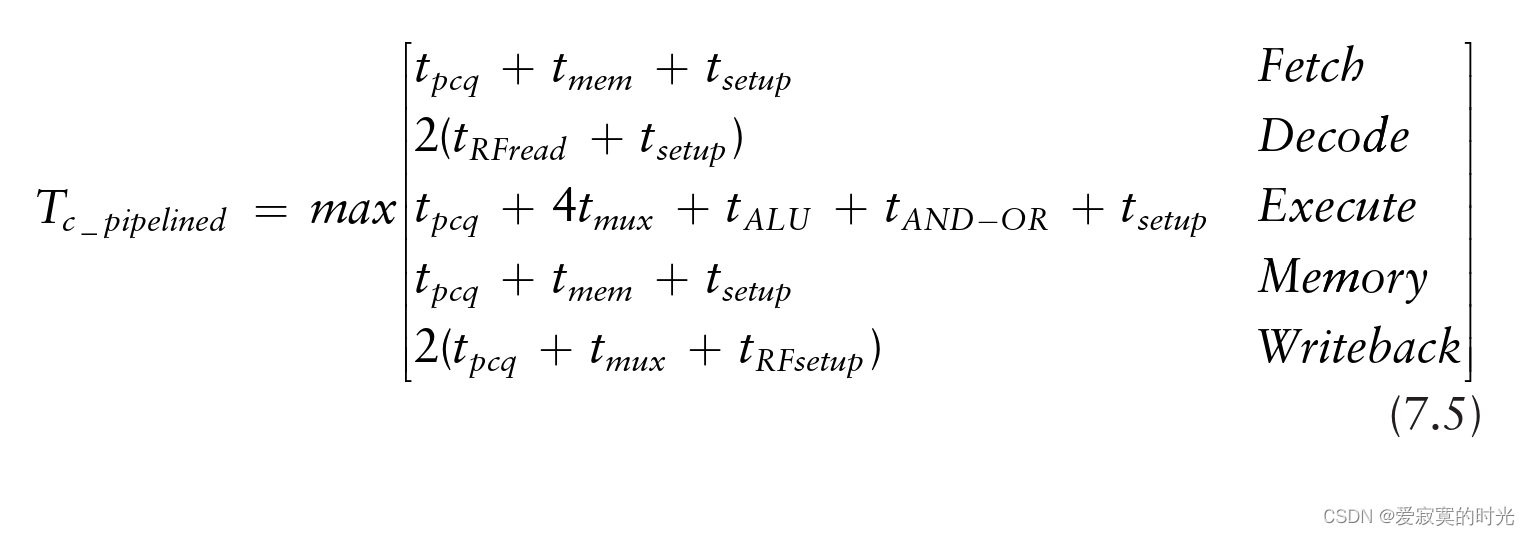hist+scatter

如果想描述二维数据的分布特征，那么一个直方图显然是不够用的，为此可使用两个直方图分别代表x和y方向上的分布情况，同时透过散点图查看其整体的分布特征。

下面创建一组二元高斯分布的数据，用于直方图测试。多元高斯分布的主要参数仍为期望和方差，但所谓多元分布，在坐标层面的表现就是坐标轴的个数，也就是向量维度。所以N个元素对应N维向量，也就有N个期望；而方差则进化为了协方差矩阵

import numpy as np
import matplotlib.pyplot as plt

mean = [0, 0]
cov = [[0, 1], [10, 0]]
x, y = np.random.multivariate_normal(mean, cov, 5000).T

其中，x,y就是待统计的数据。

fig = plt.figure()
gs = fig.add_gridspec(2, 2,  
    width_ratios=(4, 1),  
    height_ratios=(1, 4))

ax = fig.add_subplot(gs[1, 0])
ax.scatter(x, y, marker='x')        # 散点图绘制

xHist = fig.add_subplot(gs[0, 0], sharex=ax)
xHist.tick_params(axis="x", labelbottom=False)

yHist = fig.add_subplot(gs[1, 1], sharey=ax)
yHist.tick_params(axis="y", labelleft=False)

binwidth = 0.25
lim = (int(np.max(np.abs([x,y]))/0.25) + 1) * 0.25
bins = np.arange(-lim, lim + binwidth, binwidth)
xHist.hist(x, bins=bins)
yHist.hist(y, bins=bins, orientation='horizontal')

plt.show()

其中，tick_params用于取消直方图左侧和下面的坐标刻度，效果如下

hist2d

相比之下，hist2d可以更加便捷地绘制直方图，并以图像的形式反馈回来

plt.hist2d(x,y,bins=40)
plt.show()

其中，x,y即x和y轴分别要统计的样本值，bins和hist中的参数相同，表示数据条个数，只不过对应到图像中，数据条变成了数据块，效果如图所示

当然，也可以把hist+scatter图中的散点图代之以hist2d

fig = plt.figure()
gs = fig.add_gridspec(2, 2,  
    width_ratios=(4, 1),  
    height_ratios=(1, 4))

ax = fig.add_subplot(gs[1, 0])
ax.hist2d(x, y, bins=40)        # 散点图绘制

xHist = fig.add_subplot(gs[0, 0], sharex=ax)
xHist.tick_params(axis="x", labelbottom=False)

yHist = fig.add_subplot(gs[1, 1], sharey=ax)
yHist.tick_params(axis="y", labelleft=False)

binwidth = 0.25
lim = (int(np.max(np.abs([x,y]))/0.25) + 1) * 0.25
bins = np.arange(-lim, lim + binwidth, binwidth)
xHist.hist(x, bins=bins)
yHist.hist(y, bins=bins, orientation='horizontal')

plt.show()