【LeetCode热题100】打卡第19天：最大数组和&跳跃游戏

⛅前言

大家好，我是知识汲取者，欢迎来到我的LeetCode热题100刷题专栏！

精选 100 道力扣（LeetCode）上最热门的题目，适合初识算法与数据结构的新手和想要在短时间内高效提升的人，熟练掌握这 100 道题，你就已经具备了在代码世界通行的基本能力。在此专栏中，我们将会涵盖各种类型的算法题目，包括但不限于数组、链表、树、字典树、图、排序、搜索、动态规划等等，并会提供详细的解题思路以及Java代码实现。如果你也想刷题，不断提升自己，就请加入我们吧！QQ群号：827302436。我们共同监督打卡，一起学习，一起进步。

博客主页💖：知识汲取者的博客

LeetCode热题100专栏🚀：LeetCode热题100

Gitee地址📁：知识汲取者 (aghp) - Gitee.com

Github地址📁：Chinafrfq · GitHub

题目来源📢：LeetCode 热题 100 - 学习计划 - 力扣（LeetCode）全球极客挚爱的技术成长平台

PS：作者水平有限，如有错误或描述不当的地方，恳请及时告诉作者，作者将不胜感激

最大数组和

🔒题目

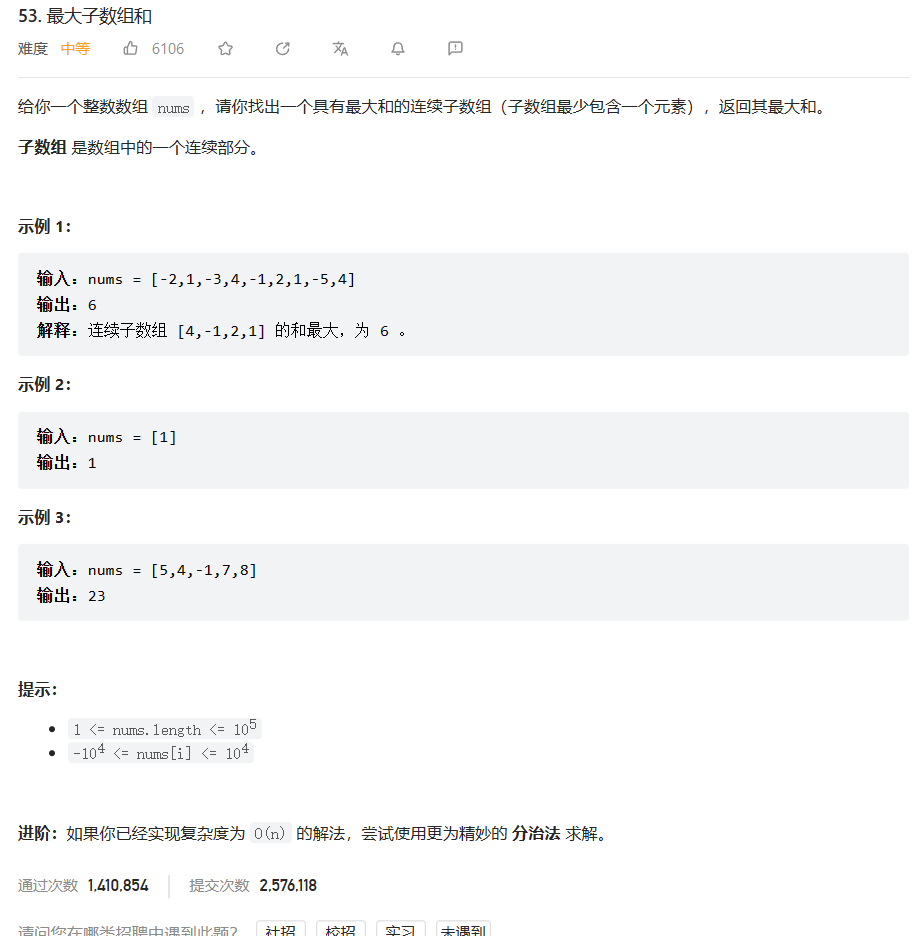
原题链接：53. 最大子数组和

🔑题解

• 解法一：暴力（内存超限，210个数据通过200个）

  import java.util.Arrays;
  import java.util.Collections;
  import java.util.List;
  import java.util.stream.Collectors;
  
  /**
   * @author ghp
   * @title 最大数组和
   */
  class Solution {
      public int maxSubArray(int[] nums) {
          List<Integer> list = Arrays.stream(nums).boxed().collect(Collectors.toList());
          for (int i = 0; i < nums.length; i++) {
              int sum = nums[i];
              for (int j = i + 1; j < nums.length; j++) {
                  sum += nums[j];
                  list.add(sum);
              }
          }
          return Collections.max(list);
      }
  }
  

  复杂度分析：

  • 时间复杂度：$O(n^2)$
  • 空间复杂度：$O(2^n)$

  其中 $n$ 为数组中元素的个数

  不出所料，对于元素个数较少的情况，是可以通过的，但是遇到长度较大的时候会出现内存超限！

  

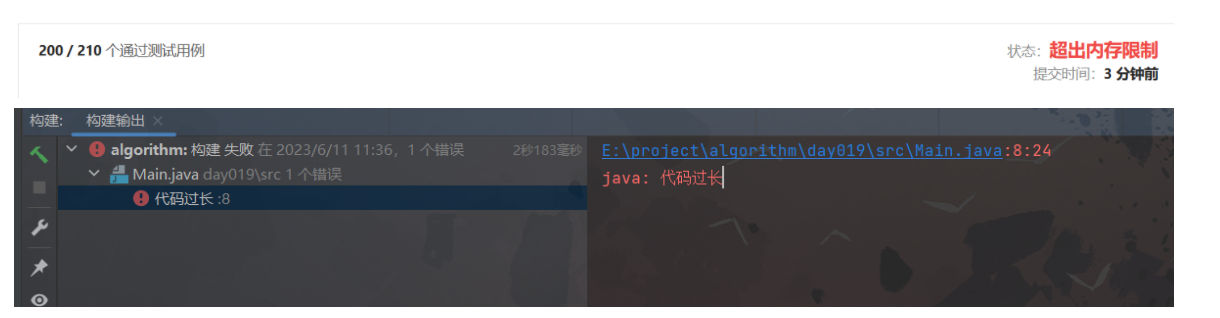
  备注：Java规定一个方法编译后的字节码大小不能超过 65535 字节，这个长度在一般情况下都是够用的，但碰到极端情况就不行了

  /**
   * @author ghp
   * @title 最大数组和
   */
  class Solution {
      public int maxSubArray(int[] nums) {
          int max = Integer.MIN_VALUE;
          for (int i = 0; i < nums.length; i++) {
              if (nums[i] > max){
                  max = nums[i];
              }
          }
          for (int i = 0; i < nums.length; i++) {
              int sum = nums[i];
              for (int j = i + 1; j < nums.length; j++) {
                  sum += nums[j];
                  if (sum>max){
                      max = sum;
                  }
              }
          }
          return max;
      }
  }
  

  复杂度分析：

  • 时间复杂度：$O(n^2)$
  • 空间复杂度：$O(1)$

  其中 $n$ 为数组中元素的个数

  这样写内存不超限了，但是时间超限！

• 解法二：动态规划

  主要思路：因为我们是要求数组连续元素之和的最大值，所以每次我们在遍历数组时，有两种选择的可能性，要么是选择当前元素，要么是选择之前求的和加上当前元素。这样我们就可以得到一个递推式：$Math.max(sum+nums[i],nums[i])$，这里需要注意，我们要需要有一个变量来记录这个过程中出现的最大值，所以还应当有：$Math.max(max,sum)$

  /**
   * @author ghp
   * @title 最大数组和
   */
  class Solution {
      public int maxSubArray(int[] nums) {
          int max = Integer.MIN_VALUE;
          int sum = 0;
          for (int i = 0; i < nums.length; i++) {
              sum = Math.max(sum+nums[i], nums[i]);
              max = Math.max(max, sum);
          }
          return sum;
      }
  }
  

  复杂度分析：

  • 时间复杂度：$O(n)$
  • 空间复杂度：$O(1)$

  其中 $n$ 为数组中元素的个数

• 解法三：

跳跃游戏

🔒题目

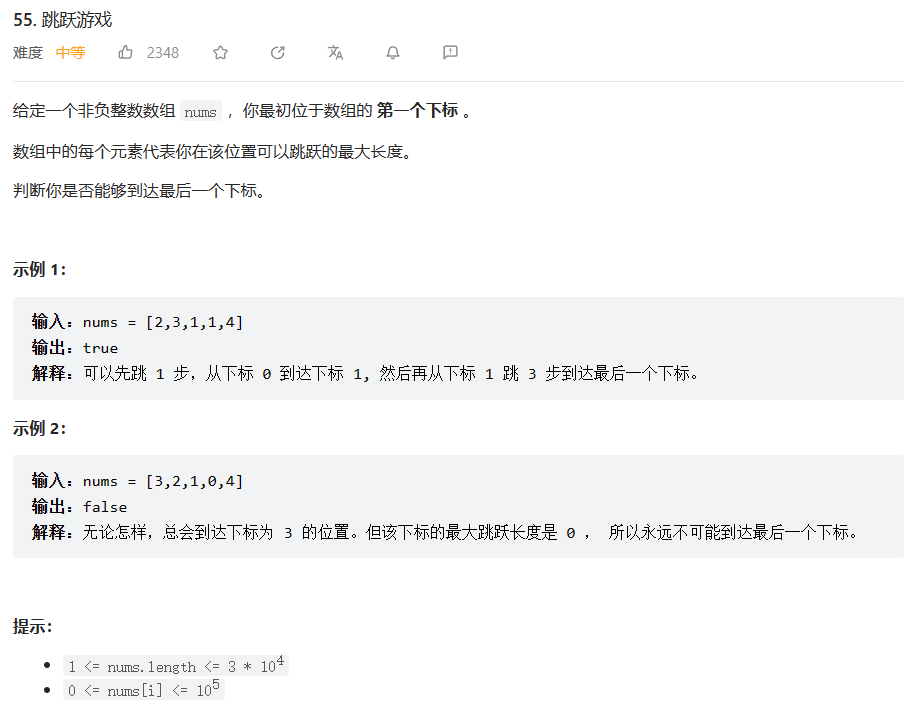
原题链接：55.跳跃游戏

🔑题解

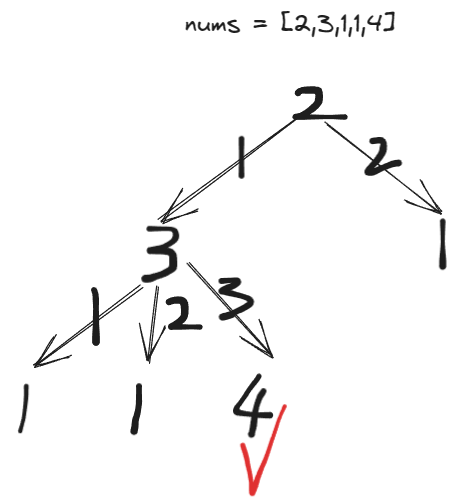
• 解法一：BFS（时间超限）

  主要不断进行搜索遍历，然后因为这相当于是一个带权的树，只要树的权值之和等于数组长度-1，就说明数组的最后一个元素可达。否则就不可达。

/**
 * @author ghp
 * @title 跳跃游戏
 */
class Solution {

    static boolean f = false;

    public boolean canJump(int[] nums) {
        int path = 0;
        bfs(nums, path);
        return f;
    }

    private void bfs(int[] nums, int path) {
        if (path >= nums.length - 1) {
            // 当前可达距离>=数组最大长度，说明可到达最后一个元素，结束递归
            f = true;
            return;
        }
        for (int i = 1; i <= nums[path]; i++) {
            // 遍历下一层
            bfs(nums, path + i);
        }
    }
}

复杂度分析：

• 时间复杂度：$O(n!)$
• 空间复杂度：$O(1)$

其中 $n$ 为数组中元素的个数

• 解法二：贪心

  通过不断迭代，计算出当前可达的最大长度，只要最大长度超过数组的长度，就可以达到数组中的最后一个元素

  /**
   * @author ghp
   * @title 跳跃游戏
   */
  class Solution {
      public boolean canJump(int[] nums) {
          int max = Integer.MIN_VALUE;
          for (int i = 0; i < nums.length; i++) {
              if (i <= max){
                  // 当前距离是能够到达的
                  // 更新最大距离
                  max = Math.max(max, i + nums[i]);
                  if (max >= nums.length-1){
                      // 最大距离已经超过了数组的长度，所以可以到达最后一个元素
                      return true;
                  }
              }
          }
          return false;
      }
  }
  

  复杂度分析：

  • 时间复杂度：$O(n)$
  • 空间复杂度：$O(1)$

  其中 $n$ 为数组中元素的个数