👉️ 力扣原文

题目

给你一个 无重复元素 的整数数组 candidates 和一个目标整数 target ，找出 candidates 中可以使数字和为目标数 target 的 所有 不同组合 ，并以列表形式返回。你可以按 任意顺序 返回这些组合。

candidates 中的 同一个 数字可以 无限制重复被选取 。如果至少一个数字的被选数量不同，则两种组合是不同的。

对于给定的输入，保证和为 target 的不同组合数少于 150 个。

示例

输入：candidates = [2,3,6,7], target = 7
输出：[[2,2,3],[7]]
解释：
2 和 3 可以形成一组候选，2 + 2 + 3 = 7 。注意 2 可以使用多次。
7 也是一个候选， 7 = 7 。
仅有这两种组合。

输入: candidates = [2,3,5], target = 8
输出: [[2,2,2,2],[2,3,3],[3,5]]

输入: candidates = [2], target = 1
输出: []

分析思路1

使用回溯算法:
使用了一个 start 变量来避免重复搜索。每次迭代从 start 开始，而不是从0开始。这是因为我们不需要在同一层次上搜索相同的元素，这会导致重复组合的出现。

此外，我们还进行了剪枝操作，即当候选数大于目标数时，我们停止搜索。这可以大大减少搜索空间，提高代码效率。

最后，我们使用了一个 result 列表来存储所有找到的组合。每当找到一个组合时，我们将其添加到结果列表中。

题解1

class Solution {
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        List<List<Integer>> result = new ArrayList<>();
        List<Integer> temp = new ArrayList<>();
        Arrays.sort(candidates); // 排序，便于剪枝
        backtrack(candidates, target, 0, temp, result);
        return result;
    }

    private void backtrack(int[] candidates, int target, int start, List<Integer> temp, List<List<Integer>> result) {
        if (target < 0) {
            return;
        }
        if (target == 0) {
            result.add(new ArrayList<>(temp));
            return;
        }
        for (int i = start; i < candidates.length; i++) {
            if (candidates[i] > target) { // 剪枝
                break;
            }
            temp.add(candidates[i]);
            backtrack(candidates, target - candidates[i], i, temp, result); // 注意这里传入的参数
            temp.remove(temp.size() - 1);
        }
    }
}

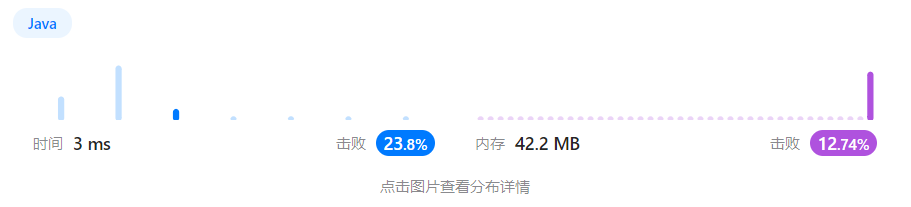
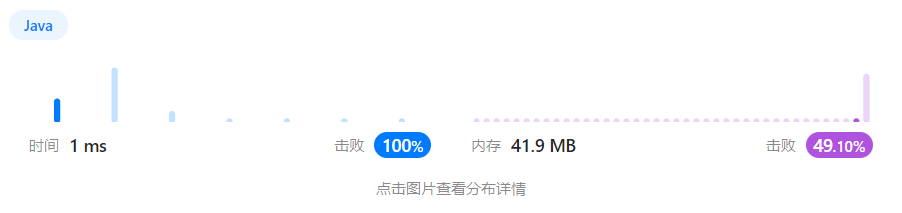
执行结果

分析思路2

动态规划：
使用了一个二维数组 dp 来存储中间结果，其中 dp[i] 表示数字和为 i 时的所有组合。初始时，dp[0] 存储一个空列表，表示数字和为 0 时只有一种组合，即不选任何数字。
接下来，对于每个数字 candidates[i]，从 candidates[i] 开始枚举数字和 j，如果 dp[j - candidates[i]] 不为空，则将 dp[j - candidates[i]] 中的每个组合都加上 candidates[i]，得到一个新的组合，将其加入到 dp[j] 中。这样，当枚举到 target 时，dp[target] 中存储的就是所有符合要求的组合。
最后，如果 dp[target] 为空，则返回一个空列表，否则返回 dp[target]。

题解2

class Solution {
    public List<List<Integer>> combinationSum(int[] candidates, int target) {
        List<List<Integer>>[] dp = new List[target + 1];
        dp[0] = new ArrayList<>();
        dp[0].add(new ArrayList<>());
        for (int i = 0; i < candidates.length; i++) {
            for (int j = candidates[i]; j <= target; j++) {
                if (dp[j - candidates[i]] != null) {
                    if (dp[j] == null) {
                        dp[j] = new ArrayList<>();
                    }
                    for (List<Integer> list : dp[j - candidates[i]]) {
                        List<Integer> temp = new ArrayList<>(list);
                        temp.add(candidates[i]);
                        dp[j].add(temp);
                    }
                }
            }
        }
        return dp[target] == null ? new ArrayList<>() : dp[target];
    }
}

执行结果