1.定义
图的定义:图是由一组顶点和一组能够将两个顶点相连的边组成
边:edge
顶点:vertex
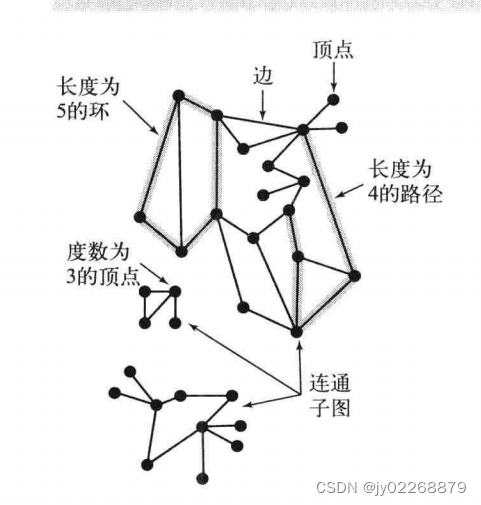
连通图:如果从任意一个顶点都存在一条路径到达另外一个任意顶点,我们称这幅图是连通图。
非连通图:由若干连通的部分组成,他们都是其极大连通子图。
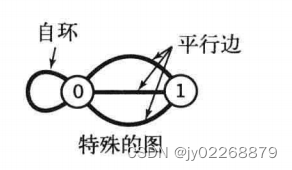
自环:即一条连接一个顶点和其自身的边
平行边:连接同一顶点的两条边称为平行边
图的密度:是指已经连接的顶点对占所有可能被连接的顶点对的比例。在稀疏图中,被连接的顶点对很少;而在稠密图中,只有少部分顶点对之间没有边连接。

二分图:是一种能够将所有结点分为两部分的图,其中图的每条边所连接的两个顶点都分别属于不同的部分。
2.图的不同表示方法
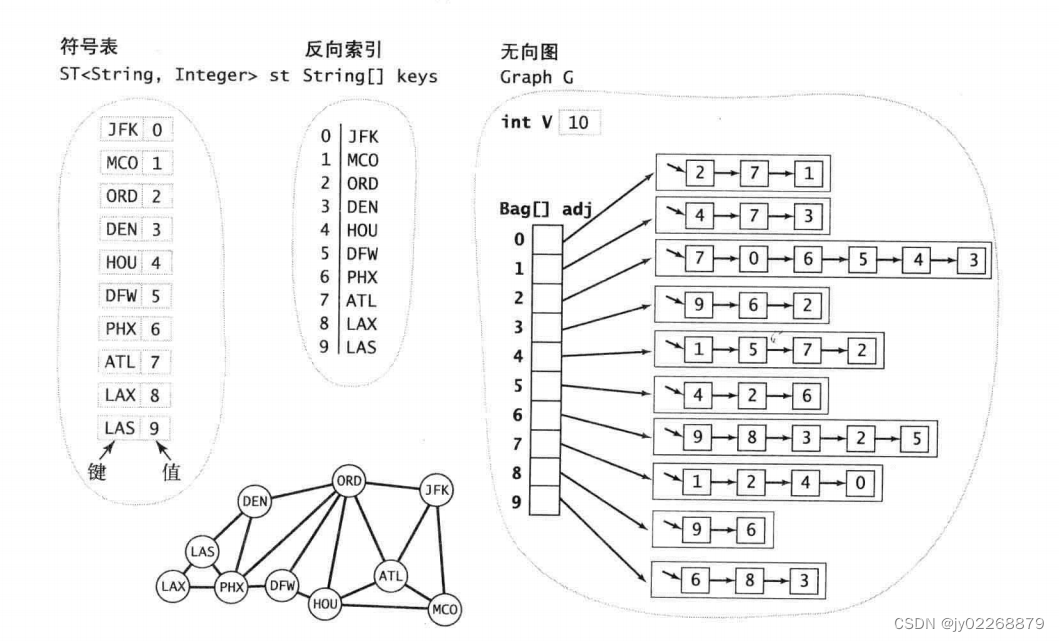
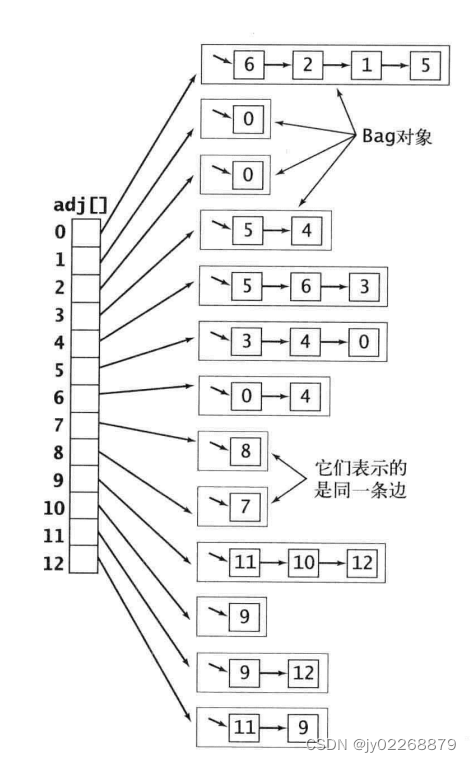
2.1邻接表数组表示
用一个以顶点为索引的列表数组,其中的每个元素都是和该顶点相邻的定点列表
2.2邻接矩阵 表示
用一个V乘以V的布尔矩阵。当顶点V和顶点W之间有相连的边时,定义V行W列的元素值为true,否则为false。如果含有上百万个顶点,V的平方个布尔值所需要的空间会非常大。
3.相关API及代码


package com.sid.graph;
public class Vertex {
String value;//顶点的值
Edge firstEdge;//第一条边
int index;//在外层数组的下标
public Vertex(String value, Edge firstEdge,int index) {
super();
this.value = value;
this.firstEdge = firstEdge;
this.index = index;
}
}
package com.sid.graph;
public class Edge {
Vertex vertex;//该边对应的顶点
int weight;//权重,无向图,有向图权重为0
Edge next;//下一个边
/**
* 构建一条边 weight未0表示无向图或者有向图 不为0表示网
* @param vertex
* @param weight
* @param next
*/
public Edge(Vertex vertex, int weight, Edge next) {
super();
this.vertex = vertex;
this.weight = weight;
this.next = next;
}
}
package com.sid.graph;
import java.util.ArrayList;
import java.util.LinkedList;
import java.util.List;
public class Graph {
/**
* 顶点数
*/
private final int V;
/**
* 边数
*/
private int E;
/**
* 邻接表
*/
private List<Vertex> adj;
public Graph(int V) {
this.V = V;
this.E = 0;
adj = new ArrayList<Vertex>(V); /** 创建邻接表 */
}
public int V() {
return V;
}
public int E() {
return E;
}
/**
* 插入顶点
*/
public Vertex addVertex(String value) {
int size = adj.size();
Vertex vertex = new Vertex(value, null,size);
adj.add(vertex);
return vertex;
}
/**
* 获取顶点
*/
public Vertex getVertexByValue(String value) {
for (int i = 0; i < V; i++) {
if (adj.get(i).value.equals(value)) {
return adj.get(i);
}
}
return null;
}
/**
* 获取顶点
*/
public Vertex getVertexByIndex(int index) {
return adj.get(index);
}
/**
* 添加无向图的边
* @param vertex1 第一个顶点
* @param vertex2 第二个顶点
*/
public void addUndigraphEdge(Vertex vertex1,Vertex vertex2) {
//因为是无向图,所以就直接加入
addEdgeByVertex(vertex1,vertex2,0);
addEdgeByVertex(vertex2,vertex1,0);
}
/**
* 添加有向图的边
* @param start 开始节点
* @param end 结束节点
*/
public void addDigraphEdge(Vertex start,Vertex end) {
//因为是有向图,所以只有一条边
addEdgeByVertex(start,end,0);
}
/**
* 添加一条由start-->end的边
*
* @param start
* @param end
* @param weight 权重未0表示无向图或者有向图,部位0表示网
*/
private void addEdgeByVertex(Vertex start, Vertex end, int weight) {
//1、找到第一个顶点
Vertex v1 = this.getVertexByValue(start.value);
if(v1 == null){
v1 = addVertex(start.value);
}
//2、检查这条边是否已经存在,已经存在就直接报错
for (Vertex w : adj(v1)) {
if (end.value.equals(w.value)) {
System.out.println("边" + start.value + "-->" + end.value + "已经加入,不可以再加入");
return;
}
}
//3.添加
Edge firstEdge = v1.firstEdge;
if (firstEdge == null) {
firstEdge = new Edge(end, weight, null);
v1.firstEdge = firstEdge;
} else {
//当前节点变为第一个节点
Edge nowEdge = new Edge(end, weight, null);
v1.firstEdge = nowEdge;
nowEdge.next = firstEdge;
}
E++;
}
/**
* 计算V节点的度数
*/
public static int degree(Graph g, int v) {
int degree = 0;
Edge firstEdge = g.getVertexByIndex(v).firstEdge;
while (firstEdge != null) {
degree++;
firstEdge = firstEdge.next;
}
return degree;
}
/**
* 计算所有顶点的最大度数
*/
public static int maxDegree(Graph G) {
int max = 0;
for (int v = 0; v < G.V(); v++) {
if (degree(G, v) > max) {
max = degree(G, v);
}
}
return max;
}
/**
* 计算所有顶点的平均度数
*/
public static int avgDegree(Graph G) {
return 2 * G.E() / G.V();
}
/**
* 计算自环的的个数
*/
public static int numberOfSelfLoops(Graph g) {
int count = 0;
for (int v = 0; v < g.V(); v++) {
for(int w : g.adj(v)){
if(v == w){
count++;
}
}
}
return count / 2; //每条边都被记过两次
}
/**
* 得到跟V节点相邻的所有节点
*/
public static List<Vertex> adj(Vertex vertex) {
List result = new LinkedList();
Edge firstEdge = vertex.firstEdge;
while (firstEdge != null) {
result.add(firstEdge.vertex);
firstEdge = firstEdge.next;
}
return result;
}
/**
* 得到跟V节点相邻的所有节点下标 入参是节点的数组下标
*/
public List<Integer> adj(int index) {
List result = new ArrayList();
Edge firstEdge = this.getVertexByIndex(index).firstEdge;
while (firstEdge != null) {
result.add(firstEdge.vertex.index);
firstEdge = firstEdge.next;
}
return result;
}
/**
* 图的邻接表的字符串表示
*/
public String toString() {
String s = V + " vertices, " + E + " edges\n";
for (int v = 0; v < V; v++) {
Vertex vertex = this.getVertexByIndex(v);
s += vertex.value + ": ";
for (Vertex w : adj(vertex)) {
s += w.value + "";
}
s += "\n";
}
return s;
}
}
4.深度优先搜索
4.1 找到以起点s连通的所有顶点,和个数
实现:
用一个递归方法来遍历所有顶点,在访问一个顶点时:
将它标记为已访问
递归地访问它的所有没有被标记过的邻居顶点

package com.sid.graph;
public class DepthFirstSearch {
private boolean[] marked;
private int count;
public DepthFirstSearch(Graph G,int s){
marked = new boolean[G.V()];
dfs(G,s);
}
private void dfs(Graph G, int index) {
marked[index] = true;
count++;
for(int w : G.adj(index)){
if(!marked[w]){
dfs(G,w);
}
}
}
public boolean marked(int w ){
return marked[w];
}
public int count(){
return count;
}
}
4.2深度优先搜索查找图中路径

注意这不一定是最短路径,比如说下面这个例子,查出来的0到1的路径,不是0----1,而是0----2----1

package com.sid.graph;
import java.util.Stack;
public class DepthFirstPaths {
private boolean[] marked; //这个顶点上调用过dfs()了吗
private int[] edgeTo; //从起点到一个顶点的已知路径上的最后一个顶点 比如S---A---C 则 edgeTo[C]=A edgeTo[C]其实表示的是谁直接指向C点
private final int s; //起点
public DepthFirstPaths(Graph G, int s) {
marked = new boolean[G.V()];
edgeTo = new int[G.V()];
this.s = s;
dfs(G, s);
}
private void dfs(Graph G, int index) {
marked[index] = true;
for (int w : G.adj(index)) {
if (!marked[w]) {
edgeTo[w] = index;
dfs(G, w);
}
}
}
public boolean hasPathTo(int v){ //起点S是否有路径到V
return marked[v];
}
public Iterable<Integer> pathTo(int v){
if(!hasPathTo(v)){
return null;
}
Stack<Integer> path = new Stack<Integer>();
for(int x = v ; x != s ; x = edgeTo[x]){
path.push(x);
}
path.push(s);
return path;
}
}
5.广度优先搜索
深度优先搜索得到的路径不仅取决于图的结构,还取决于图的表示和递归调用的性质。
“从S到给定目标的顶点V是否存在一条路径?如果有,找出其中最短的那条”,则需要用广度优先搜索。
实现:
1.使用一个队列来保存所有已经被标记过,但其邻接表还未被检查过的顶点
2.先将起点加入队列,然后重复以下步骤直到队列为空
取队列中的下一个顶点v并标记它
将与v相邻的所有未被标记过的顶点加入队列

package com.sid.graph;
import java.util.*;
public class BreadthFirstPaths {
private boolean[] marked; //这个顶点上调用过dfs()了吗
private int[] edgeTo; //从起点到一个顶点的已知路径上的最后一个顶点 比如S---A---C 则 edgeTo[C]=A edgeTo[C]其实表示的是谁直接指向C点
private final int s; //起点
public BreadthFirstPaths(Graph G, int s) {
marked = new boolean[G.V()];
edgeTo = new int[G.V()];
this.s = s;
bfs(G, s);
}
private void bfs(Graph G, int s) {
Queue<Integer> queue = new PriorityQueue<>();
marked[s] = true;
queue.add(s);
while (!queue.isEmpty()){
int v = queue.poll();
for(int w : G.adj(v)){
if(!marked[w]){
edgeTo[w] = v;
marked[w] = true;
queue.add(w);
}
}
}
}
public boolean hasPathTo(int v){
return marked[v];
}
public Iterable<Integer> pathTo(int v){
if(!hasPathTo(v)){
return null;
}
Stack<Integer> path = new Stack<Integer>();
for(int x = v ; x != s ; x = edgeTo[x]){
path.push(x);
}
path.push(s);
return path;
}
}
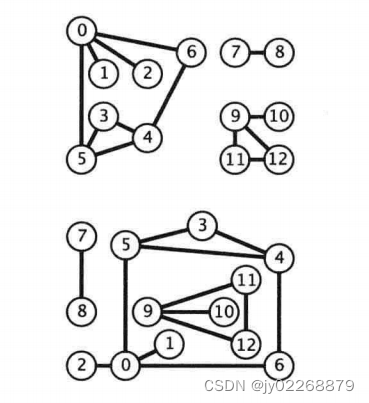
6.连通分量
无向图G的极大连通子图称为G的连通分量( Connected Component)。
任何连通图的连通分量只有一个,即是其自身,非连通的无向图有多个连通分量。

实现
使用marked[]数组来寻找一个顶点作为每个连通分量中深度优先搜索的起点。
递归的深度优先搜索第一次调用的参数是顶点0,它会标记所有与0连通的顶点。
然后构造函数中for循环会查找每个没有被标记的顶点,并且递归调用dfs()来标记和它相邻的所有顶点。
使用一个以顶点作为索引的数组id[],将同一个连通分量的顶点和连通分量的标识符关联起来(int 值)。这个数组是的connected()方法的实现变得十分简单。
标识符0会被赋予第一个连通分量中的所有顶点,1会被赋予第二个连通分量中的所有顶点,以此类推。这样所有的标识符都会如API中指定的那样在0到count()-1之间。这个约定使得以子图作为索引的数组成为可能。
例子

最后
id[0]= 0
id[1]= 0
id[2]= 0
id[3]= 0
id[4]= 0
id[5]= 0
id[6]= 0
id[7]= 1
id[8]= 1
id[9]= 2
id[10]= 2
id[11]= 2
id[12]= 2
也就是说count[]的值相同的是一个连通分量,count[]的值不同的节点之间是走不通的。
代码
package com.sid.graph;
public class CC {
private boolean[] marked;
private int[] id;
private int count;
public CC(Graph G){
marked = new boolean[G.V()];
id = new int[G.V()];
for(int s = 0 ; s < G.V(); s++){
if(!marked[s]){
dfs(G,s);
count++;
}
}
}
private void dfs(Graph G,int v){
marked[v] = true;
id[v] = count;
for(int w : G.adj(v)){
if(!marked[w]){
dfs(G,w);
}
}
}
public boolean connected(int v, int w){
return id[v] == id[w];
}
public int id(int v){
return id[v];
}
public int count(){
return count;
}
}
7.G是无环图吗
使用深度优先处理
如果不存在自环和平行边,就是无环图
package com.sid.graph;
public class Cycle {
private boolean[] marked;
private boolean hasCycle;
public Cycle(Graph G){
marked = new boolean[G.V()];
for(int s = 0 ; s < G.V(); s++){
if(!marked[s]){
dfs(G,s,s);
}
}
}
private void dfs(Graph G, int v , int u){
marked[v] = true;
for(int w : G.adj(v)){
if(!marked[w]){
dfs(G,w,v);
}
else if(w != u){ //A---B 与B相邻的节点肯定有A,我理解的是无向图中他们属于同一条边,不是环。
hasCycle = true;
}
}
}
public boolean hasCycle(){
return hasCycle;
}
}
8.G是二分图吗(双色问题)
package com.sid.graph;
public class TwoColor {
private boolean[] marked;
private boolean[] color;
private boolean isTowColorable = true;
public TwoColor(Graph G){
marked = new boolean[G.V()];
color = new boolean[G.V()];
for(int s = 0 ; s < G.V(); s++){
if(!marked[s]){
dfs(G,s);
}
}
}
private void dfs(Graph G, int v) {
marked[v] = true;
for (int w : G.adj(v)){
if(!marked[w]){
color[w] = !color[v];
}else if(color[w] == color[v]){
isTowColorable = false;
return;
}
}
}
public boolean isBipartite(){
return isTowColorable;
}
}
9.符号图
节点里面装的不是数字,而是其他的,比如字符串