C++哈希(个人笔记)

news2025/1/20 15:52:43

C++哈希

    • 1.unordered_mapd
      • 1.1unordered_map的构造函数
      • 1.2unorder_map的容量
      • 1.3unordered_map的迭代器
      • 1.4unordered_map的元素访问
      • 1.5unorder_map的查找
      • 1.6unordered_map的修改操作
      • 1.7unordered_map的桶操作
    • 2.unordered_set
    • 3.unordered_set和unordered_set的笔试题
    • 4.哈希
      • 4.1哈希概念
      • 4.2哈希冲突
      • 4.3哈希函数
      • 4.4哈希冲突解决
        • 4.4.1闭散列
          • 4.4.1.1线性探测的实现
        • 4.4.2开散列
          • 4.4.2.1开散列的实现
    • 4.unordered_map和unordered_set模拟实现
      • 4.1哈希表的改造
      • 4.2unordered_set模拟实现
      • 4.3unordered_map模拟实现
    • 5.位图
      • 5.1位图的实现
      • 5.2布隆过滤器
        • 5.2.1布隆过滤器的实现

1.unordered_mapd

C++unorder_map官方文档

1.1unordered_map的构造函数

函数声明功能介绍
unordered_map构造不同格式的unordered_map对象

1.2unorder_map的容量

函数声明功能介绍
bool empty() const检测unordered_map是否为空
size_t size() const获取unordered_map的有效元素个数

1.3unordered_map的迭代器

函数声明功能介绍
begin返回unordered_map第一个元素的迭代器
end返回unordered_map最后一个元素下一个位置的迭代器
cbegin返回unordered_map第一个元素的const迭代器
cend返回unordered_map最后一个元素下一个位置的const迭代器

1.4unordered_map的元素访问

函数声明功能介绍
operator[]返回与key对应的value，没有则返回一个默认值

注意：
该函数中实际调用哈希桶的插入操作，用参数key与V()构造一个默认值往底层哈希桶中插入，如果key不在哈希桶中，插入成功，返回V()，插入失败，说明key已经在哈希桶中，将key对应的value返回。

1.5unorder_map的查找

函数声明功能介绍
iterator find(const K& key)返回key在哈希桶中的位置
size_t count(const K& key)返回哈希桶中关键码为key的键值对的个数

注意：unordered_map中key是不能重复的，因此count函数的返回值最大为1

1.6unordered_map的修改操作

函数声明功能介绍
insert向容器中插入键值对
erase删除容器中的键值对
void clear()清空容器中有效元素个数
void swap(unorder map&)交换两个容器中的元素

1.7unordered_map的桶操作

函数声明功能介绍
size_t bucket count()const返回哈希桶中桶的总个数
size_t bucket size(size_t n)const返回n号桶中有效元素的总个数
size_t bucket(const K& key)返回元素key所在的桶号

2.unordered_set

C++unordered_set官方文档
这里不在一 一列举

3.unordered_set和unordered_set的笔试题

在长度 2N 的数组中找出重复 N 次的元素
在这里插入图片描述

class Solution {
public:
    int repeatedNTimes(vector<int>& nums)
    {
        unordered_map<int, int> found;
        for (int num : nums)
        {
            found[num]++;
        }
        for (auto it = found.begin(); it != found.end(); ++it)
        {
            if (it->second == nums.size() / 2)
            {
                return it->first;
            }
        }
        return -1;
    }
};

两个数组的交集
在这里插入图片描述

class Solution {
public:
    vector<int> intersection(vector<int>& nums1, vector<int>& nums2)
    {
        set<int> s1(nums1.begin(),nums1.end());
        set<int> s2(nums2.begin(),nums2.end());
        auto it1=s1.begin();
        auto it2=s2.begin();
        vector<int> v;
        while(it1!=s1.end()&&it2!=s2.end())
        {
            if(*it1<*it2)
            {
                ++it1;
            }
            else if(*it1>*it2)
            {
                ++it2;
            }
            else
            {
                v.push_back(*it1);
                ++it1;
                ++it2;
            }
        }
        return v;
    }
};

两个数组的交集 II
在这里插入图片描述

class Solution {
public:
    vector<int> intersect(vector<int>& nums1, vector<int>& nums2)
    {
        multiset<int> s1(nums1.begin(),nums1.end());
        multiset<int> s2(nums2.begin(),nums2.end());
        auto it1=s1.begin();
        auto it2=s2.begin();
        vector<int> v;
        while(it1!=s1.end()&&it2!=s2.end())
        {
            if(*it1<*it2)
            {
                it1++;
            }
            else if(*it1>*it2)
            {
                it2++;
            }
            else
            {
                v.push_back(*it1);
                it1++;
                it2++;
            }
        }
        return v;
    }
};

存在重复元素
在这里插入图片描述

class Solution {
public:
    bool containsDuplicate(vector<int>& nums)
    {
        unordered_map<int,int> mp;
        for(int num:nums)
        {
            mp[num]++;
        }
        auto it=mp.begin();
        while(it!=mp.end())
        {
            if(it->second>=2)
            {
                return true;
            }
            ++it;
        }
        return false;
    }
};

两句话中的不常见单词
在这里插入图片描述

class Solution {
public:
    vector<string> uncommonFromSentences(string s1, string s2)
    {
        vector<string> v;
        unordered_map<string,int> mp;
        stringstream ss1(s1);
        string word;
        while(ss1>>word)
        {
            mp[word]++;
        }

        stringstream ss2(s2);
        while(ss2>>word)
        {
            mp[word]++;
        }
        for(auto& w:mp)
        {
            if(w.second==1)
            {
                v.push_back(w.first);
            }
        }
        return v;
    }
};

4.哈希

4.1哈希概念

通过某种函数(hashFunc)使元素的存储位置与它的关键码之间能够建立一 一映射的关系，在查找时通过该函数可以很快找到该元素。

1.插入元素
根据待插入元素的关键码，以此函数计算出该元素的存储位置并按此位置进行存放

2.搜索元素
对元素的关键码进行同样的计算，把求得的函数值当做元素的存储位置，在结构中按此位置取元素比较，若关键码相等，则找到了

哈希方法中使用的转换函数称为哈希(散列)函数，构造出来的结构称为哈希表(Hash Table)(或者称散列表)

4.2哈希冲突

不同关键字通过相同哈希哈数计算出相同的哈希地址，该种现象称为哈希冲突或哈希碰撞。

4.3哈希函数

哈希函数设计原则：

  1. 1.哈希函数的定义域必须包括需要存储的全部关键码，而如果散列表允许有m个地址时，其值域必须在0到m-1之间

  2. 哈希函数计算出来的地址能均匀分布在整个空间中

  3. 哈希函数应该比较简单
    常见的哈希函数

  4. 直接定址法–(常用)
    取关键字的某个线性函数为散列地址：Hash（Key）= A*Key + B
    优点：简单、均匀
    缺点：需要事先知道关键字的分布情况
    使用场景：适合查找比较小且连续的情况

  5. 除留余数法–(常用)
    设散列表中允许的地址数为m，取一个不大于m，但最接近或者等于m的质数p作为除数，
    按照哈希函数：Hash(key) = key% p(p<=m),将关键码转换成哈希地址

4.4哈希冲突解决

解决哈希冲突两种常见的方法是：闭散列和开散列

4.4.1闭散列

也叫开放定址法，当发生哈希冲突时，如果哈希表未被装满，说明在哈希表中必然还有空位置，那么可以把key存放到冲突位置中的“下一个” 空位置中去。

线性探测：从发生冲突的位置开始，依次向后探测，直到寻找到下一个空位置为止。

1.插入

  1. 通过哈希函数获取待插入元素在哈希表中的位置
  2. 如果该位置中没有元素则直接插入新元素，如果该位置中有元素发生哈希冲突， 使用线性探测找到下一个空位置，插入新元素
    在这里插入图片描述
    2.删除
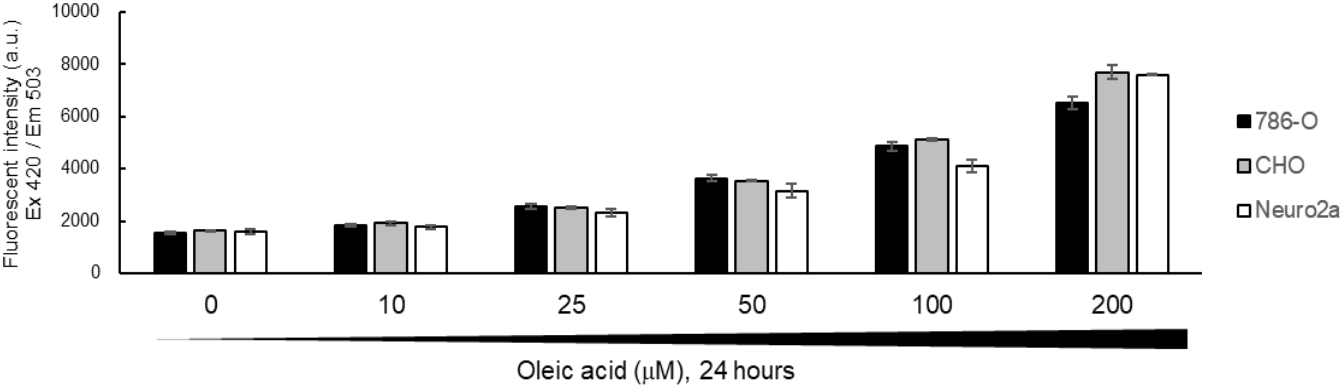
    采用闭散列处理哈希冲突时，不能物理删除哈希表中已有的元素，若直接删除元素会影响其他元素的搜索。比如删除元素4，如果直接删除掉，44查找起来可能会受影响。因此线性探测采用标记的伪删除法来删除一个元素。（也就是给位置标记状态）
// 哈希表每个空间给个标记
// EMPTY此位置空， EXIST此位置已经有元素， DELETE元素已经删除
enum State{EMPTY, EXIST, DELETE};
4.4.1.1线性探测的实现
enum Status
{
	EMPTY,
	EXIST,
	DELETE
};

template<class K,class V>
struct HashData
{
	pair<K, V> _kv;
	Status _s;          //状态
};

//HashFunc<int>
template<class K>
struct HashFunc
{
	size_t operator()(const K& Key)
	{
		return (size_t)Key;
	}
};

//HashFunc<string>
template<>
struct HashFunc<string>
{
	size_t operator()(const string& key)
	{
		size_t hash = 0;
		for (auto e : key)
		{
			hash *= 31;
			hash += e;
		}
		cout << key << ":" << hash << endl;
		return hash;
	}
};

template<class K,class V,class Hash=HashFunc<K>>
class HashTable
{
public:
	HashTable()
	{
		_tables.resize(10);
	}

	bool Insert(const pair<K, V>& kv)
	{
		if (Find(kv.first))
		{
			return false;
		}
		//负载因子0.7就扩容
		if (_n * 10 / _tables.size() == 7)
		{
			size_t newSize = _tables.size() * 2;
			HashTable<K, V> newHT;
			newHT._tables.resize(newSize);
			for (size_t i = 0;i < _tables.size();i++)
			{
				if (_tables[i]._s == EXIST)
				{
					newHT.Insert(_tables[i]._kv);
				}
			}
			_tables.swap(newHT._tables);
		}

		Hash hf;
		size_t hashi = hf(kv.first) % _tables.size();
		while (_tables[hashi]._s == EXIST)
		{
			hashi++;
			hashi %= _tables.size();
		}
		_tables[hashi]._kv = kv;
		_tables[hashi]._s = EXIST;
		++_n;
		return true;
	}

	HashData<K, V>* Find(const K& key)
	{
		Hash hf;
		size_t hashi = hf(key) % _tables.size();
		while (_tables[hashi]._s != EMPTY)
		{
			if (_tables[hashi]._s == EXIST && _tables[hashi]._kv.first == key)
			{
				return &_tables[hashi];
			}
			hashi++;
			hashi %= _tables.size();
		}
		return nullptr;
	}

	bool Erase(const K& key)
	{
		HashData<K, V>* ret = Find(key);
		if (ret)
		{
			ret->_s = DELETE;
			--_n;
			return true;
		}
		else
		{
			return false;
		}
	}

	void Print()
	{
		for (size_t i = 0;i < _tables.size();i++)
		{
			if (_tables[i]._s == EXIST)
			{
				cout << "[" << i << "]->" << _tables[i]._kv.first << ":" << _tables[i]._kv.second << endl;
			}
			else if (_tables[i]._s == EMPTY)
			{
				printf("[%d]->\n", i);
			}
			else
			{
				printf("[%d]->D\n", i);
			}
		}
		cout << endl;
	}

private:
	vector<HashData<K,V>> _tables;
	size_t _n = 0;//存储的关键字的个数
};

线性探测优点：实现非常简单

线性探测缺点：一旦发生哈希冲突，所有的冲突连在一起，容易产生数据“堆积”，即：不同
关键码占据了可利用的空位置，使得寻找某关键码的位置需要许多次比较，导致搜索效率降
低。

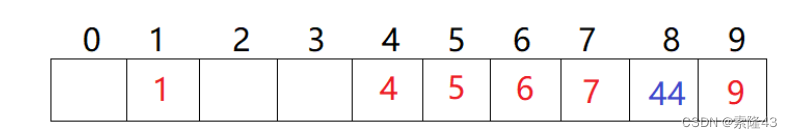
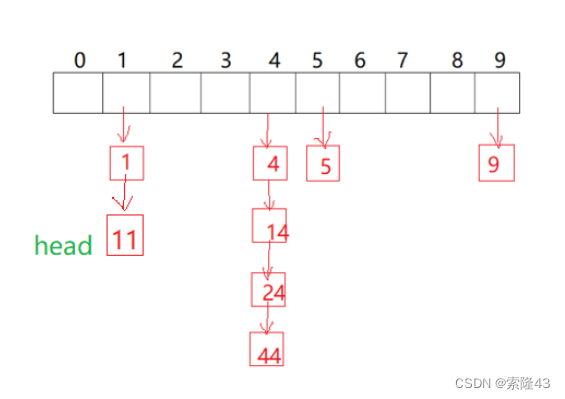
4.4.2开散列

开散列法又叫链地址法(开链法)，首先对关键码集合用散列函数计算散列地址，具有相同地
址的关键码归于同一子集合，每一个子集合称为一个桶，各个桶中的元素通过一个单链表链
接起来，各链表的头结点存储在哈希表中。
在这里插入图片描述

4.4.2.1开散列的实现
//HashFunc<int>
template<class K>
struct HashFunc
{
	size_t operator()(const K& Key)
	{
		return (size_t)Key;
	}
};

//HashFunc<string>
template<>
struct HashFunc<string>
{
	size_t operator()(const string& key)
	{
		size_t hash = 0;
		for (auto e : key)
		{
			hash *= 31;
			hash += e;
		}
		cout << key << ":" << hash << endl;
		return hash;
	}
};

template<class K, class V>
struct HashNode
{
	HashNode* _next;
	pair<K, V> _kv;

	HashNode(const pair<K, V>& kv)
		:_kv(kv)
		, _next(nullptr)
	{}
};

template<class K, class V,class Hash=HashFunc<K>>
class HashTable
{
	typedef HashNode<K, V> Node;
public:
	HashTable()
	{
		_tables.resize(10);
	}

	~HashTable()
	{
		for (size_t i = 0; i < _tables.size(); i++)
		{
			Node* cur = _tables[i];
			while (cur)
			{
				Node* next = cur->_next;
				delete cur;
				cur = next;
			}
			_tables[i] = nullptr;
		}
	}

	bool Insert(const pair<K, V>& kv)
	{
		if (Find(kv.first))
			return false;

		Hash hf;

		// 负载因子最大到1
		if (_n == _tables.size())
		{
			vector<Node*> newTables;
			newTables.resize(_tables.size() * 2, nullptr);
			// 遍历旧表
			for (size_t i = 0; i < _tables.size(); i++)
			{
				Node* cur = _tables[i];
				while (cur)
				{
					Node* next = cur->_next;

					//挪动到映射的新表
					size_t hashi = hf(cur->_kv.first) % newTables.size();

					cur->_next = newTables[hashi];//标记
					newTables[hashi] = cur;
					cur = next;
				}
				_tables[i] = nullptr;
			}

			_tables.swap(newTables);
		}

		size_t hashi = hf(kv.first) % _tables.size();
		Node* newnode = new Node(kv);

		// 头插
		newnode->_next = _tables[hashi];
		_tables[hashi] = newnode;
		++_n;

		return true;
	}

	Node* Find(const K& key)
	{
		Hash hf;
		size_t hashi = hf(key) % _tables.size();
		Node* cur = _tables[hashi];
		while (cur)
		{
			if (cur->_kv.first == key)
			{
				return cur;
			}
			cur = cur->_next;
		}
		return nullptr;
	}

	bool Erase(const K& key)
	{
		Hash hf;
		size_t hashi = hf(key) % _tables.size();
		Node* prev = nullptr;
		Node* cur = _tables[hashi];
		while (cur)
		{
			if (cur->_kv.first == key)
			{
				if (prev == nullptr)
				{
					_tables[hashi] = cur->_next;
				}
				else
				{
					prev->_next = cur->_next;
				}
				delete cur;
				return true;
			}
			prev = cur;
			cur = cur->_next;
		}
		return false;
	}

	void Some()
	{
		size_t bucketSize = 0;
		size_t maxBucketLen = 0;
		size_t sum = 0;
		double averageBucketLen = 0;

		for (size_t i = 0;i < _tables.size();i++)
		{
			Node* cur = _tables[i];
			if (cur)
			{
				++bucketSize;
			}
			size_t bucketLen = 0;
			while (cur)
			{
				++bucketLen;
				cur = cur->_next;
			}

			sum += bucketLen;
			if (bucketLen > maxBucketLen)
			{
				maxBucketLen = bucketLen;
			}
		}
		printf("all bucketSize:%d\n", _tables.size());
		printf("bucketSize:%d\n", bucketSize);
		printf("maxBucketLen:%d\n", maxBucketLen);
		printf("averageBucketLen:%lf\n\n", averageBucketLen);
	}

private:
	vector<Node*> _tables;
	size_t _n = 0;
};

4.unordered_map和unordered_set模拟实现

4.1哈希表的改造

//HashFunc<int>
template<class K>
struct HashFunc
{
	size_t operator()(const K& Key)
	{
		return (size_t)Key;
	}
};

//HashFunc<string>
template<>
struct HashFunc<string>
{
	size_t operator()(const string& key)
	{
		size_t hash = 0;
		for (auto e : key)
		{
			hash *= 31;
			hash += e;
		}
		cout << key << ":" << hash << endl;
		return hash;
	}
};
namespace hash_bucket
{
	template<class T>
	struct HashNode
	{
		HashNode* _next;
		T _data;

		HashNode(const T& data)
			:_data(data)
			, _next(nullptr)
		{}
	};

	// 前置声明
	template<class K, class T, class KeyOfT, class Hash>
	class HashTable;

	template<class K,class T,class Ref,class Ptr,class KeyOfT,class Hash>
	struct __HTIterator
	{
		typedef HashNode<T> Node;
		typedef __HTIterator<K, T, Ref, Ptr, KeyOfT, Hash> Self;
		Node* _node;
		const HashTable<K, T, KeyOfT, Hash>* _pht;
		size_t _hashi;

		__HTIterator(Node* node,HashTable<K,T,KeyOfT,Hash>* pht,size_t hashi)
			:_node(node)
			,_pht(pht)
			,_hashi(hashi)
		{}

		__HTIterator(Node* node, const HashTable<K, T, KeyOfT, Hash>* pht, size_t hashi)
			:_node(node)
			, _pht(pht)
			, _hashi(hashi)
		{}

		Self& operator++()
		{
			if (_node->_next)
			{
				_node = _node->_next;
			}
			else
			{
				++_hashi;
				while (_hashi < _pht->_tables.size())
				{
					if (_pht->_tables[_hashi])
					{
						_node = _pht->_tables[_hashi];
						break;
					}
					++_hashi;
				}
				if (_hashi == _pht->_tables.size())
				{
					_node = nullptr;
				}
			}
			return *this;
		}

		Ref operator*()
		{
			return _node->_data;
		}

		Ptr operator->()
		{
			return &(_node->_data);
		}

		bool operator!=(const Self& s)
		{
			return _node != s._node;
		}
	};

	//unordered_set->HashTable<K,K>
	//unordered_map->HashTable<K,pair<K,V>>
	template<class K, class T,class KeyOfT,class Hash>
	class HashTable
	{
		typedef HashNode<T> Node;

		template<class K, class T, class Ref, class Ptr, class KeyOfT, class Hash>
		friend struct __HTIterator;

	public:
		typedef __HTIterator<K, T, T&, T*, KeyOfT, Hash> iterator;
		typedef __HTIterator<K, T, const T&, const T*, KeyOfT, Hash> const_iterator;

		iterator begin()
		{
			for (size_t i = 0;i < _tables.size();i++)
			{
				if (_tables[i])
				{
					return iterator(_tables[i], this, i);
				}
			}
			return end();
		}

		iterator end()
		{
			return iterator(nullptr, this, -1);
		}

		const_iterator begin() const
		{
			for (size_t i = 0;i < _tables.size();i++)
			{
				if (_tables[i])
				{
					return const_iterator(_tables[i], this, i);
				}
			}
			return end();
		}

		const_iterator end() const
		{
			return const_iterator(nullptr, this, -1);
		}

		HashTable()
		{
			_tables.resize(10);
		}

		~HashTable()
		{
			for (size_t i = 0; i < _tables.size(); i++)
			{
				Node* cur = _tables[i];
				while (cur)
				{
					Node* next = cur->_next;
					delete cur;
					cur = next;
				}
				_tables[i] = nullptr;
			}
		}

		pair<iterator,bool> Insert(const T& data)
		{
			Hash hf;
			KeyOfT kot;
			iterator it = Find(kot(data));
			if (it != end())
			{
				return make_pair(it, false);
			}

			// 负载因子最大到1
			if (_n == _tables.size())
			{
				vector<Node*> newTables;
				newTables.resize(_tables.size() * 2, nullptr);
				// 遍历旧表
				for (size_t i = 0; i < _tables.size(); i++)
				{
					Node* cur = _tables[i];
					while (cur)
					{
						Node* next = cur->_next;

						//挪动到映射的新表
						size_t hashi = hf(kot(data)) % newTables.size();

						cur->_next = newTables[hashi];//标记
						newTables[hashi] = cur;
						cur = next;
					}
					_tables[i] = nullptr;
				}

				_tables.swap(newTables);
			}

			size_t hashi = hf(kot(data)) % _tables.size();
			Node* newnode = new Node(data);

			// 头插
			newnode->_next = _tables[hashi];
			_tables[hashi] = newnode;
			++_n;

			return make_pair(iterator(newnode,this,hashi),true);
		}

		iterator Find(const K& key)
		{
			Hash hf;
			KeyOfT kot;
			size_t hashi = hf(key) % _tables.size();
			Node* cur = _tables[hashi];
			while (cur)
			{
				if (kot(cur->_data) == key)
				{
					return iterator(cur,this,hashi);
				}
				cur = cur->_next;
			}
			return end();
		}

		bool Erase(const K& key)
		{
			Hash hf;
			KeyOfT kot;
			size_t hashi = hf(key) % _tables.size();
			Node* prev = nullptr;
			Node* cur = _tables[hashi];
			while (cur)
			{
				if (kot(cur->_data) == key)
				{
					if (prev == nullptr)
					{
						_tables[hashi] = cur->_next;
					}
					else
					{
						prev->_next = cur->_next;
					}
					delete cur;
					return true;
				}
				prev = cur;
				cur = cur->_next;
			}
			return false;
		}

		void Some()
		{
			size_t bucketSize = 0;
			size_t maxBucketLen = 0;
			size_t sum = 0;
			double averageBucketLen = 0;

			for (size_t i = 0;i < _tables.size();i++)
			{
				Node* cur = _tables[i];
				if (cur)
				{
					++bucketSize;
				}
				size_t bucketLen = 0;
				while (cur)
				{
					++bucketLen;
					cur = cur->_next;
				}

				sum += bucketLen;
				if (bucketLen > maxBucketLen)
				{
					maxBucketLen = bucketLen;
				}
			}
			averageBucketLen = (double)sum / (double)bucketSize;
			printf("all bucketSize:%d\n", _tables.size());
			printf("bucketSize:%d\n", bucketSize);
			printf("maxBucketLen:%d\n", maxBucketLen);
			printf("averageBucketLen:%lf\n\n", averageBucketLen);
		}

	private:
		vector<Node*> _tables;
		size_t _n = 0;
	};
}

4.2unordered_set模拟实现

#pragma once
#include"HashTable.h"

namespace ljh
{
	template<class K, class Hash = HashFunc<K>>
	class unordered_set
	{
		struct SetKeyOfT
		{
			const K& operator()(const K& key)
			{
				return key;
			}
		};
	public:
		typedef typename hash_bucket::HashTable<K, K, SetKeyOfT, Hash>::const_iterator iterator;
		typedef typename hash_bucket::HashTable<K, K, SetKeyOfT, Hash>::const_iterator const_iterator;

		/*iterator begin()
		{
			return _ht.begin();
		}

		iterator end()
		{
			return _ht.end();
		}*/

		const_iterator begin() const
		{
			return _ht.begin();
		}

		const_iterator end() const
		{
			return _ht.end();
		}

		pair<const_iterator, bool> insert(const K& key)
		{
			auto ret = _ht.Insert(key);
			return pair<const_iterator, bool>(const_iterator(ret.first._node, ret.first._pht, ret.first._hashi), ret.second);
		}

		iterator find(const K& key)
		{
			return _ht.Find(key);
		}

		bool erase(const K& key)
		{
			return _ht.Erase(key);
		}
	private:
		hash_bucket::HashTable<K, K, SetKeyOfT, Hash> _ht;
	};
}

4.3unordered_map模拟实现

#pragma once
#include"HashTable.h"
namespace ljh
{
	template<class K,class V,class Hash=HashFunc<K>>
	class unordered_map
	{
		struct MapKeyOfT
		{
			const K& operator()(const pair<K, V>& kv)
			{
				return kv.first;
			}
		};
	public:
		typedef typename hash_bucket::HashTable<K, pair<const K, V>, MapKeyOfT, Hash>::iterator iterator;
		iterator begin()
		{
			return _ht.begin();
		}

		iterator end()
		{
			return _ht.end();
		}

		pair<iterator, bool> insert(const pair<K, V>& kv)
		{
			return _ht.Insert(kv);
		}

		V& operator[](const K& key)
		{
			pair<iterator, bool> ret = _ht.Insert(make_pair(key, V()));
			return ret.first->second;
		}

		const V& operator[](const K& key) const
		{
			pair<iterator, bool> ret = _ht.Insert(make_pair(key, V()));
			return ret.first->second;
		}

		iterator find(const K& key)
		{
			return _ht.Find(key);
		}

		bool erase(const K& key)
		{
			return _ht.Erase(key);
		}

	private:
		hash_bucket::HashTable<K, pair<const K, V>, MapKeyOfT, Hash> _ht;
	};
}

5.位图

给40亿个不重复的无符号整数，没排过序。给一个无符号整数，如何快速判断一个数是否在这40亿个数中。
解决方案：
1：暴力遍历：时间复杂度O（N）
2.快排（O（NlogN）)+二分查找（logN）
3.位图
数据是否在给定的整形数据中，结果是在或者不在，刚好是两种状态，可以使用一个二进制比特位来代表数据是否存在，如果二进制比特位为1，代表存在，为0代表不存在。

5.1位图的实现

//N为需要多少比特位
template<size_t N>
class bitset
{
public:
	bitset()
	{
		_bits.resize(N / 32 + 1);
	}

	void set(size_t x)
	{
		size_t i = x / 32;
		size_t j = x % 32;
		_bits[i] |= (1 << j);
	}

	void reset(size_t x)
	{
		size_t i = x / 32;
		size_t j = x % 32;
		_bits[i] &= ~(1 << j);
	}

	bool test(size_t x)
	{
		size_t i = x / 32;
		size_t j = x % 32;
		return _bits[i] & (1 << j);
	}

private:
	vector<int> _bits;
};

template<size_t N>
class twobitset
{
public:
	void set(size_t x)
	{
		//00->01
		//01->10
		//10->11
		//11->不变
		if (_bs1.test(x) == false && _bs2.test(x) == false)
		{
			_bs2.set(x);
		}
		else if (_bs1.test(x) == false && _bs2.test(x) == true)
		{
			_bs1.set(x);
			_bs2.reset(x);
		}
		else if (_bs1.test(x) == true && _bs2.test(x) == false)
		{
			_bs2.set(x);
		}
	}

	void Print()
	{
		for (size_t i = 0;i < N;i++)
		{
			if (_bs1.test(i) == false && _bs2.test(i) == true)
			{
				cout << "1->" << i << endl;
			}
			else if (_bs1.test(i) == true && _bs2.test(i) == false)
			{
				cout << "2->" << i << endl;
			}
		}
		cout << endl;
	}

private:
	bitset<N> _bs1;
	bitset<N> _bs2;
};

5.2布隆过滤器

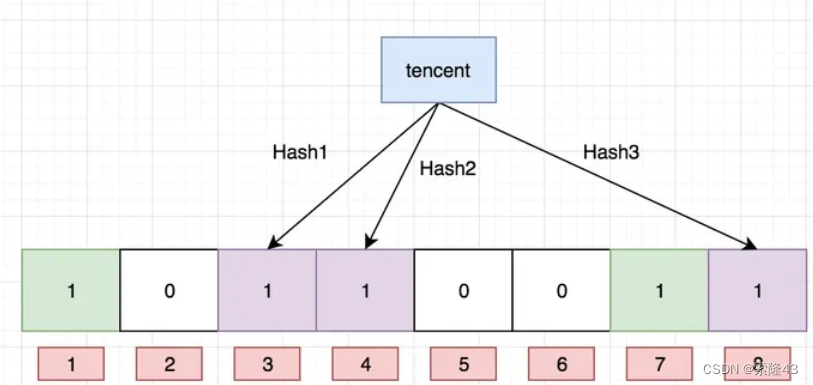
具体实现思想：用多个哈希函数，将一个数据映射到位图结构中
作用：某样东西一定不存在或者可能存在
在这里插入图片描述
在这里插入图片描述

5.2.1布隆过滤器的实现
#include<string>
#include<iostream>
#include<vector>
using namespace std;
#include"bitset.h"
struct BKDRHash
{
	size_t operator()(const string& key)
	{
		// BKDR
		size_t hash = 0;
		for (auto e : key)
		{
			hash *= 31;
			hash += e;
		}

		return hash;
	}
};

struct APHash
{
	size_t operator()(const string& key)
	{
		size_t hash = 0;
		for (size_t i = 0; i < key.size(); i++)
		{
			char ch = key[i];
			if ((i & 1) == 0)
			{
				hash ^= ((hash << 7) ^ ch ^ (hash >> 3));
			}
			else
			{
				hash ^= (~((hash << 11) ^ ch ^ (hash >> 5)));
			}
		}
		return hash;
	}
};

struct DJBHash
{
	size_t operator()(const string& key)
	{
		size_t hash = 5381;
		for (auto ch : key)
		{
			hash += (hash << 5) + ch;
		}
		return hash;
	}
};

template<size_t N,class K = string,class HashFunc1 = BKDRHash,class HashFunc2 = APHash,class HashFunc3 = DJBHash>
class BloomFilter
{
public:
	void Set(const K& key)
	{
		size_t hash1 = HashFunc1()(key) % N;
		size_t hash2 = HashFunc2()(key) % N;
		size_t hash3 = HashFunc3()(key) % N;
		_bs.set(hash1);
		_bs.set(hash2);
		_bs.set(hash3);
	}

	//void Reset(const K& key);一般不支持删除

	bool Test(const K& key)
	{
		//判断不存在是准确的，其他的都是存在偏差的
		size_t hash1 = HashFunc1()(key) % N;
		if (_bs.test(hash1) == false)
		{
			return false;
		}

		size_t hash2 = HashFunc2()(key) % N;
		if (_bs.test(hash2) == false)
		{
			return false;
		}

		size_t hash3 = HashFunc3()(key) % N;
		if (_bs.test(hash3) == false)
		{
			return false;
		}
		// 存在误判的
		return true;
	}

private:
	ljh::bitset<N> _bs;
};

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.coloradmin.cn/o/1674061.html

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈，一经查实，立即删除！

相关文章

window.localStorage.setItem() 报错

window.localStorage.setItem() 报错

目录标题 一、原因二、快速解决三、彻底解决 一、原因 在使用sessionStorage做缓存处理时&#xff0c;报了如上的错误提示&#xff0c;经查是sessionStorage对大小是有限制的&#xff0c;所以要进行try catch&#xff0c;500KB左右的东西保存起来就会令到Resources变卡&#x…
阅读更多...
240W 宽电压输入 AC/DC 导轨式开关电源——TPR/NDR-U240-XS 系列

240W 宽电压输入 AC/DC 导轨式开关电源——TPR/NDR-U240-XS 系列

TPR/NDR-U240-XS 系列导轨式开关电源&#xff0c;额定输出功率为240W&#xff0c;产品输入范围&#xff1a;90-264VAC。提供24V、48V输出&#xff0c;具有短路保护&#xff0c;过载保护等功能&#xff0c;并具备高效率&#xff0c;高可靠性、高寿命、更安全、更稳定等特点&…
阅读更多...
YOLOv8_seg训练流程-原理解析[实例分割理论篇]

YOLOv8_seg训练流程-原理解析[实例分割理论篇]

本篇将介绍一下YOLOv8实例分割网络的训练流程，同样在看此篇文章之前先去看一下预测流程YOLOv8_seg预测流程-原理解析[实例分割理论篇]-CSDN博客 ，还有目标检测任务的训练流程YOLOv8训练流程-原理解析[目标检测理论篇]-CSDN博客 ，这两篇都是前置课程，下图是YOLOv8实例分割的…
阅读更多...
【Linux取经路】进程通信之匿名管道

【Linux取经路】进程通信之匿名管道

文章目录 一、进程间通信介绍1.1 进程间通信是什么&#xff1f;1.2 进程间通信的目的1.3 进程通信该如何实现 二、管道2.1 匿名管道2.1.1 站在文件描述符角度深入理解管道2.1.2 接口使用2.1.3 PIPE_BUFFER 和 Pipe capacity2.1.4 管道中的四种情况2.1.5 管道特征总结 2.2 匿名管…
阅读更多...
八字排盘软件-​无敌八字排盘软件

八字排盘软件-​无敌八字排盘软件

功能介绍 1.完全免费使用&#xff0c;即使用不需要付费且无任何限制。 2.同时推出手机版电脑版&#xff0c;两版本数据互通互用&#xff0c;即电脑版的数据可以备份到手机版上导入&#xff0c;手机版的数据也可以备份到电脑版上恢复导入&#xff0c;方便手机和电脑共用的朋友。…
阅读更多...
前端崽的java study笔记

前端崽的java study笔记

文章目录 basic1、sprint boot概述2、sprint boot入门3、yml 配置信息书写和获取 持续更新ing~ basic 1、sprint boot概述 sprint boot特性&#xff1a; 起步依赖&#xff08;maven坐标&#xff09;&#xff1a;解决配置繁琐的问题&#xff0c;只需要引入sprint boot起步依赖的…
阅读更多...
周围很多朋友都不做java开发了而转换学网络安全，这个值得吗？

周围很多朋友都不做java开发了而转换学网络安全，这个值得吗？

在开始前我有一些资料&#xff0c;是我根据网友给的问题精心整理了一份「java的资料从专业入门到高级教程」&#xff0c; 点个关注在评论区回复“888”之后私信回复“888”&#xff0c;全部无偿共享给大家&#xff01;&#xff01;&#xff01; 其实说白了&#xff0c;值不值…
阅读更多...
在线音乐系统

在线音乐系统

文章目录 在线音乐系统一、项目演示二、项目介绍三、部分功能截图四、部分代码展示五、底部获取项目&#xff08;9.9&#xffe5;带走&#xff09; 在线音乐系统 一、项目演示 音乐网站 二、项目介绍 基于springbootvue的前后端分离在线音乐系统 登录角色 : 用户、管理员 用…
阅读更多...
超详细Redis下载安装图文教程（Win和Linux版）

超详细Redis下载安装图文教程（Win和Linux版）

超级详细 的 Redis 安装教程 说明 Windows 版本的 Redis 是 Microsoft 的开源部门提供的 Redis. 这个版本的 Redis 适合开发人员学习使用&#xff0c;生产环境中使用 Linux 系统上的 Redis, 这里讲解了这两种的安装和下载。按照你们需要的liunx 或window步骤来 就可以了&#x…
阅读更多...
Linux sudo 指令

Linux sudo 指令

sudo命令 概念&#xff1a; sudo是linux下常用的允许普通用户使用超级用户权限的工具&#xff0c;允许系统管理员让普通用户执行一些或者全部的root命令&#xff0c;如halt&#xff0c;reboot&#xff0c;su等。这样不仅减少了root用户的登录和管理时间&#xff0c;同样也提高…
阅读更多...
案例分享 I 千视协助Lentia City 购物中心实现轻量化、数字化转型

案例分享 I 千视协助Lentia City 购物中心实现轻量化、数字化转型

随着文娱活动的日益复苏&#xff0c;Lentia City作为奥地利最受欢迎的社交和文化聚集地之一&#xff0c;正逐渐成为人们追逐乐趣和交流的热门去处。这里丰富多彩的音乐表演和活动吸引着大量人群&#xff0c;为城市注入了生机和活力。 这些活动不仅仅是简单的娱乐&#xff0c;它…
阅读更多...
社区新零售：家门口的便利与温暖

社区新零售：家门口的便利与温暖

社区新零售&#xff1a;家门口的便利与温暖 随着都市生活节奏的加快&#xff0c;人们对于便捷、高效的生活方式有了更高的追求。社区新零售&#xff0c;作为零售业的一股新兴力量&#xff0c;正以其独特的魅力&#xff0c;悄然改变着我们的日常生活。 家门口的便利 社区新零…
阅读更多...
Python以docker形式部署，flask简易服务器。

Python以docker形式部署，flask简易服务器。

公司大部分都是springboot 服务器&#xff0c;有时候用到python写的一些模型&#xff0c;部署在linux上进行处理 首先项目这样&#xff1a; flask就不说了&#xff0c;快捷服务器&#xff0c; # -*- coding: utf-8 -*-from flask import Flask, request# 实例化Flask对象 app…
阅读更多...
个人投资黄金td该怎样操作？

个人投资黄金td该怎样操作？

黄金TD&#xff0c;即黄金延期交易&#xff0c;是投资者在金融市场上常见的一种操作方式。它允许投资者通过交易所进行黄金买卖&#xff0c;利用黄金价格的波动来赚取差价。对于个人投资者而言&#xff0c;正确理解和操作黄金TD是实现资产增值的关键。本文将详细介绍如何操作黄…
阅读更多...
一键追爆款，GPT一键改文‌‍‬⁣⁡​⁤⁢​⁢⁡⁣‬‍‌​​‬ ​‍⁤‬ ‬⁡⁡⁡‍‌‬⁡⁡⁢‬⁤⁢⁢⁤​‍‌​​‬ ​⁣‌，绘唐3，绘唐工具

一键追爆款，GPT一键改文‌‍‬⁣⁡​⁤⁢​⁢⁡⁣‬‍‌​​‬ ​‍⁤‬ ‬⁡⁡⁡‍‌‬⁡⁡⁢‬⁤⁢⁢⁤​‍‌​​‬ ​⁣‌，绘唐3，绘唐工具

ai画影满足你的制作要求 一键追爆款&#xff0c;GPT一键改文 入口工具 AI推文小说&漫画解说&解压混剪 人物定义&#xff0c;角色定义&#xff0c;lora转换&#xff0c;模型转换&#xff0c;可视化参考满足 一键追爆款 一键挂机生成&#xff0c;效果更精彩&#xff…
阅读更多...
专题模块项目功能说明和运行方法-01

专题模块项目功能说明和运行方法-01

项目集介绍 SpringbootSeries父工程 此模块中只有一个pom.xml文件&#xff0c;是后面所有模块的父模块&#xff0c;主要功能有两个&#xff1a;子模块管理和依赖管理。 类别必选可选基础框架jdk 17 spring-boot-starter 3.2.4spring-boot-starter-web 3.2.4spring-cloud 2023…
阅读更多...
【java】代理

【java】代理

什么是代理 假设有一个核心方法叫转账&#xff0c;为了安全性考虑&#xff0c;不能让用户直接访问接口。此时涉及到了代理&#xff0c;这使得用户只能访问代理类&#xff0c;增加了访问限制。 代理的定义&#xff1a;给目标对象提供一个代理对象&#xff0c;并且由代理对象控…
阅读更多...
[XYCTF]-PWN:Intermittent解析（pop栈内数据构造shellcode，自己编写shellcode）

[XYCTF]-PWN:Intermittent解析（pop栈内数据构造shellcode，自己编写shellcode）

查看ida 这里程序只会把输入的前12字节内容移到虚拟地址里&#xff0c;然后执行&#xff0c;大小不足以让执行shellcode&#xff0c;只能用pop寄存器调用read&#xff0c;再把gets hell的shellcode输入进去 完整exp&#xff1a; from pwn import* context(log_leveldebug,arc…
阅读更多...
一个完美的回到顶部按钮

一个完美的回到顶部按钮

大家好，我是 Just，这里是「设计师工作日常」，今天给大家写了一个丝滑回到顶部的按钮，原生js实现的，兼容性所有主流浏览器，可在vue中使用，适用于网页、h5等。 最新实例通过公众号「设计师工作日常」发布。 目录 整体效果核心代码html 代码css 部分代码js 部分代码完整代…
阅读更多...
Funakoshi — LipiDye Ⅱ脂滴活细胞成像试剂

Funakoshi — LipiDye Ⅱ脂滴活细胞成像试剂

Funakoshi LipiDye II是一款适用于长时间活细胞成像以观察动态脂滴&#xff08;LDS&#xff09;合成、移动或降解的绿色荧光染料&#xff1b;是LipiDye&#xff08;货号&#xff1a;FDV-0010&#xff09;的升级版&#xff0c;同时具备超强的光稳定性和高灵敏度等特点。 ➧ 产品…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023