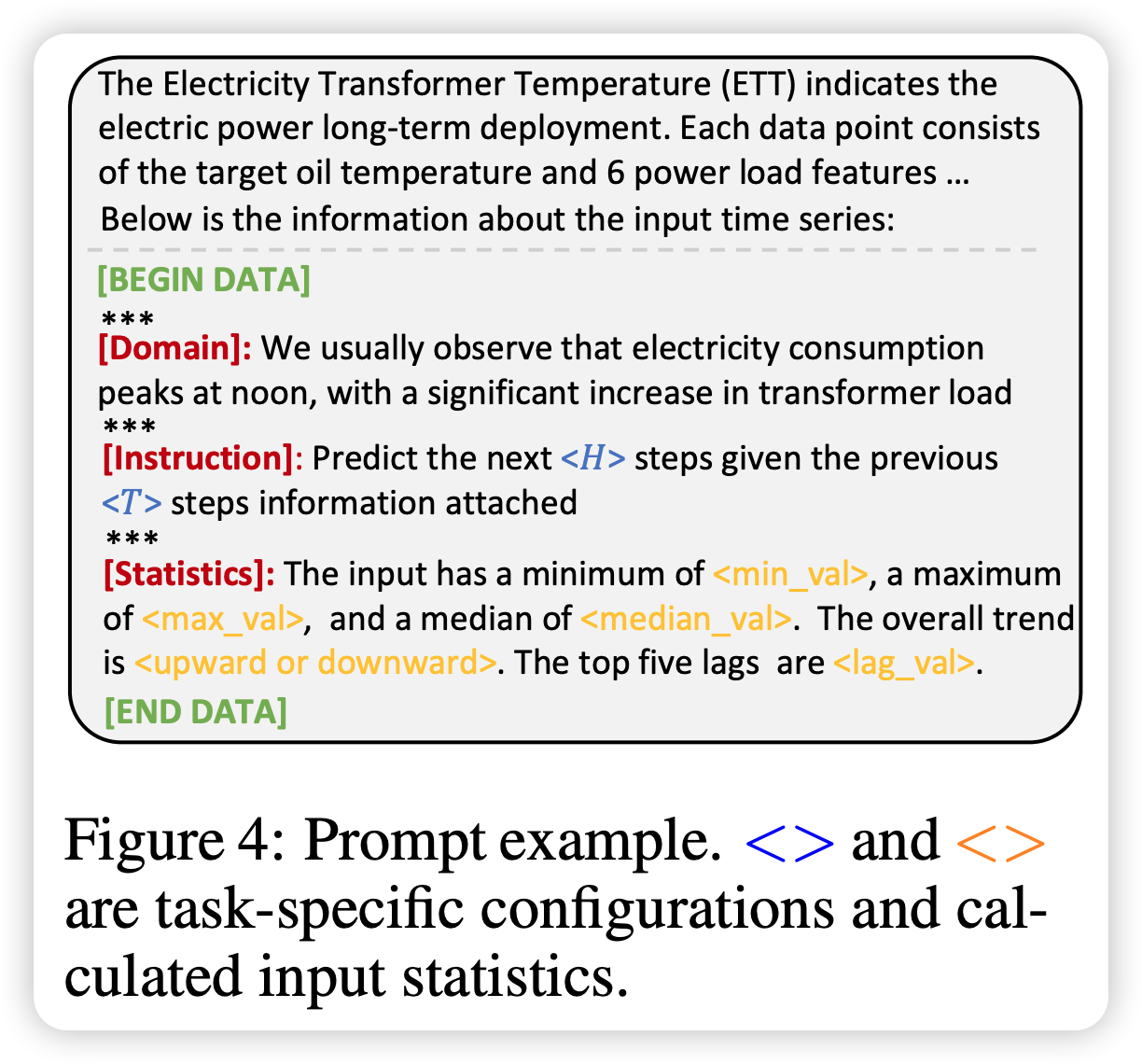
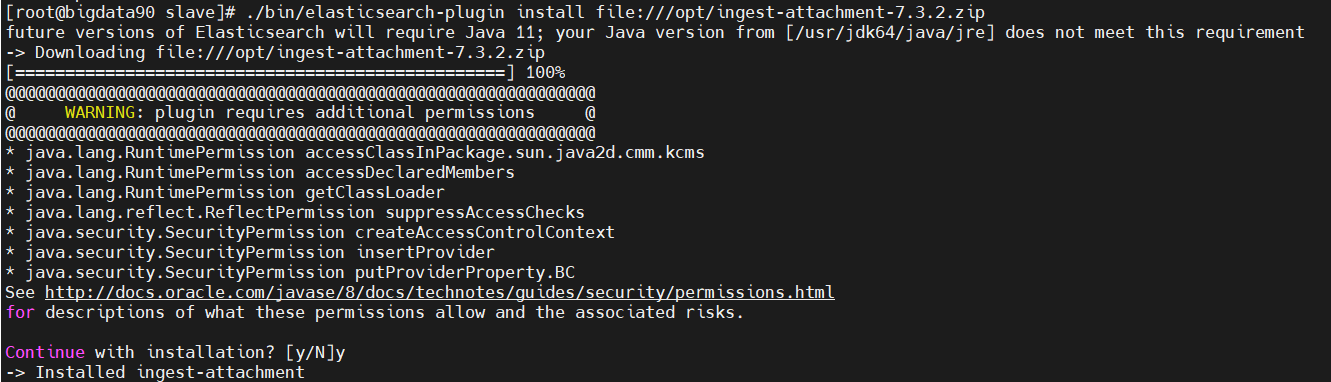
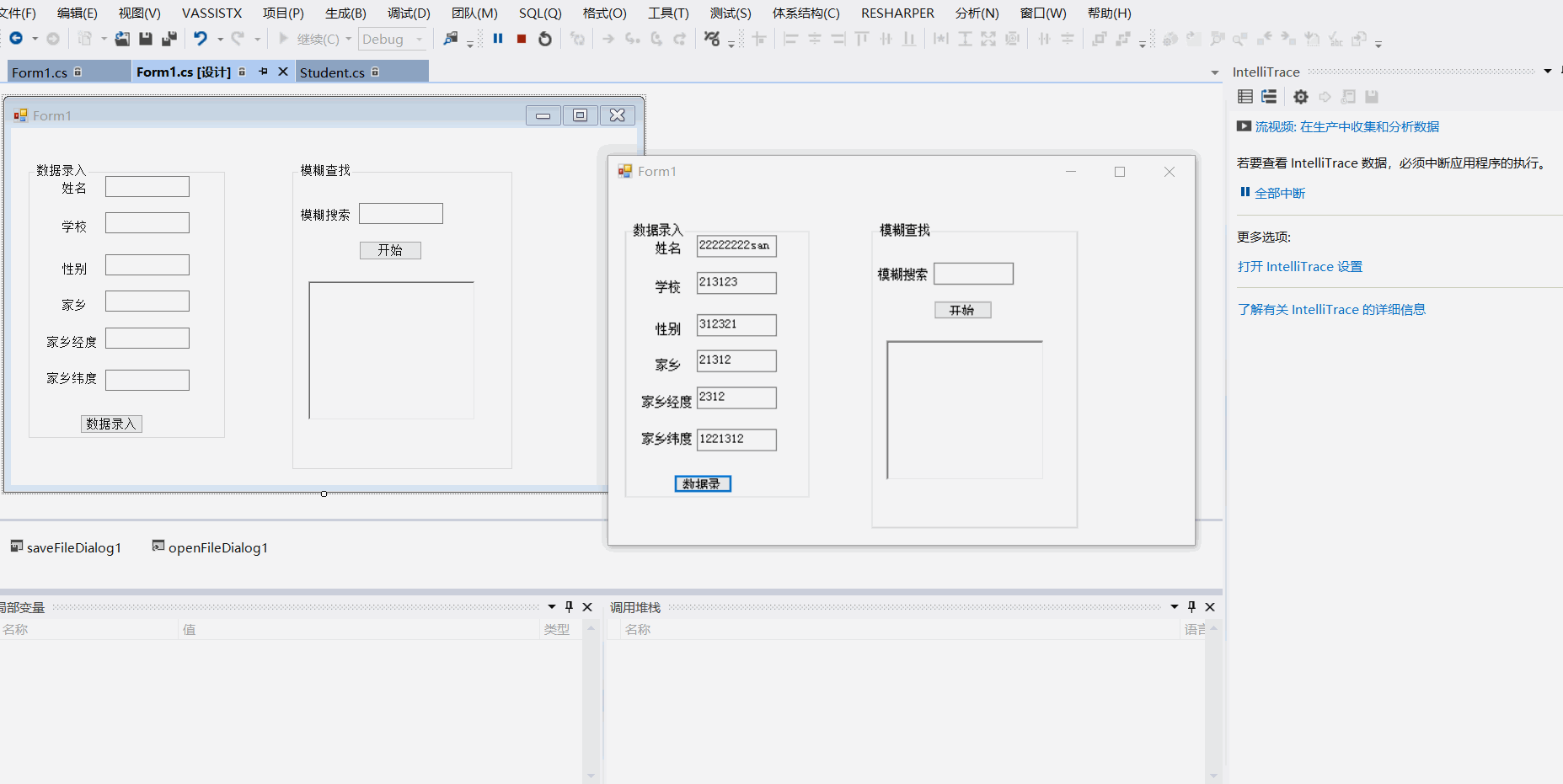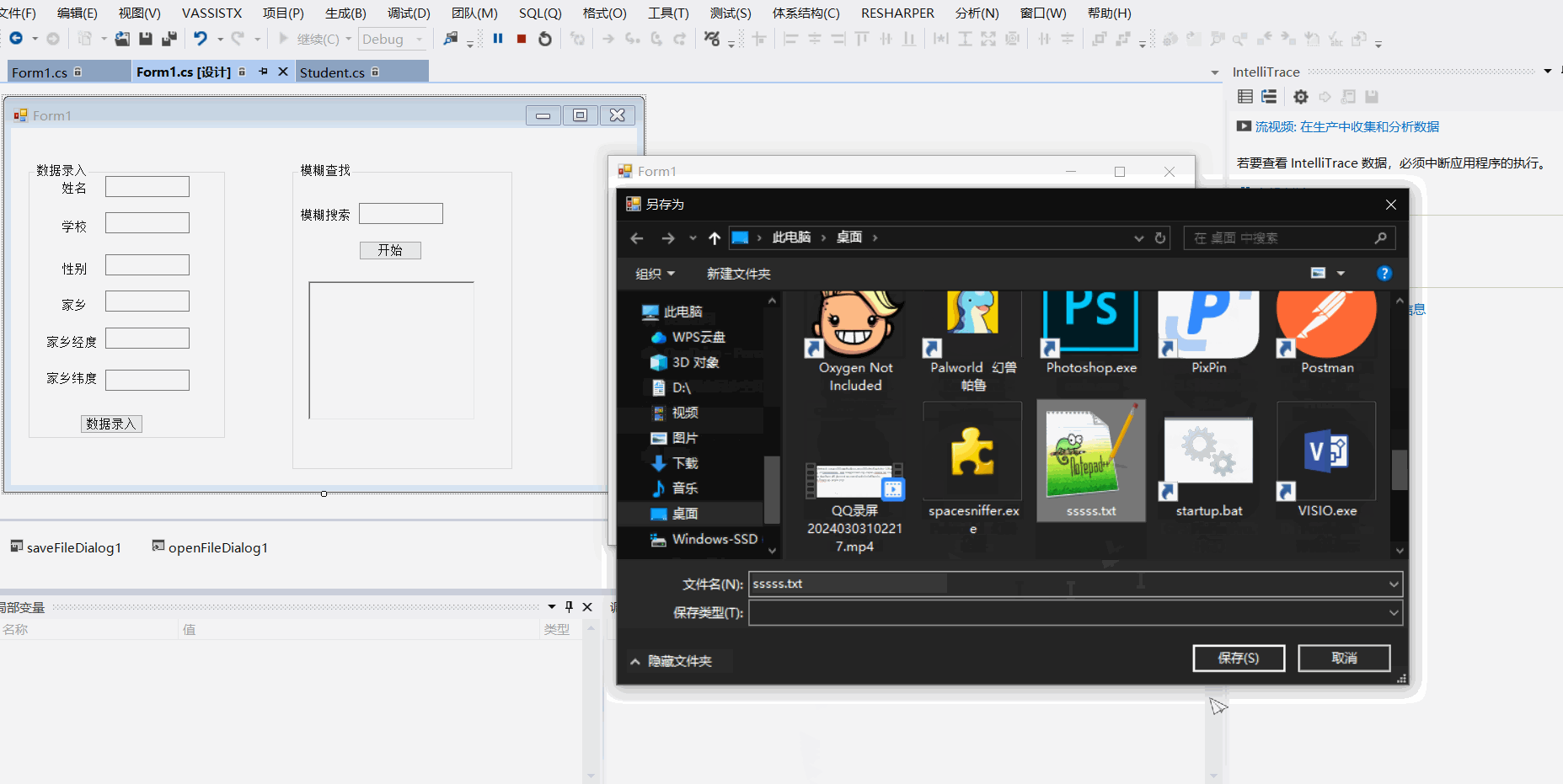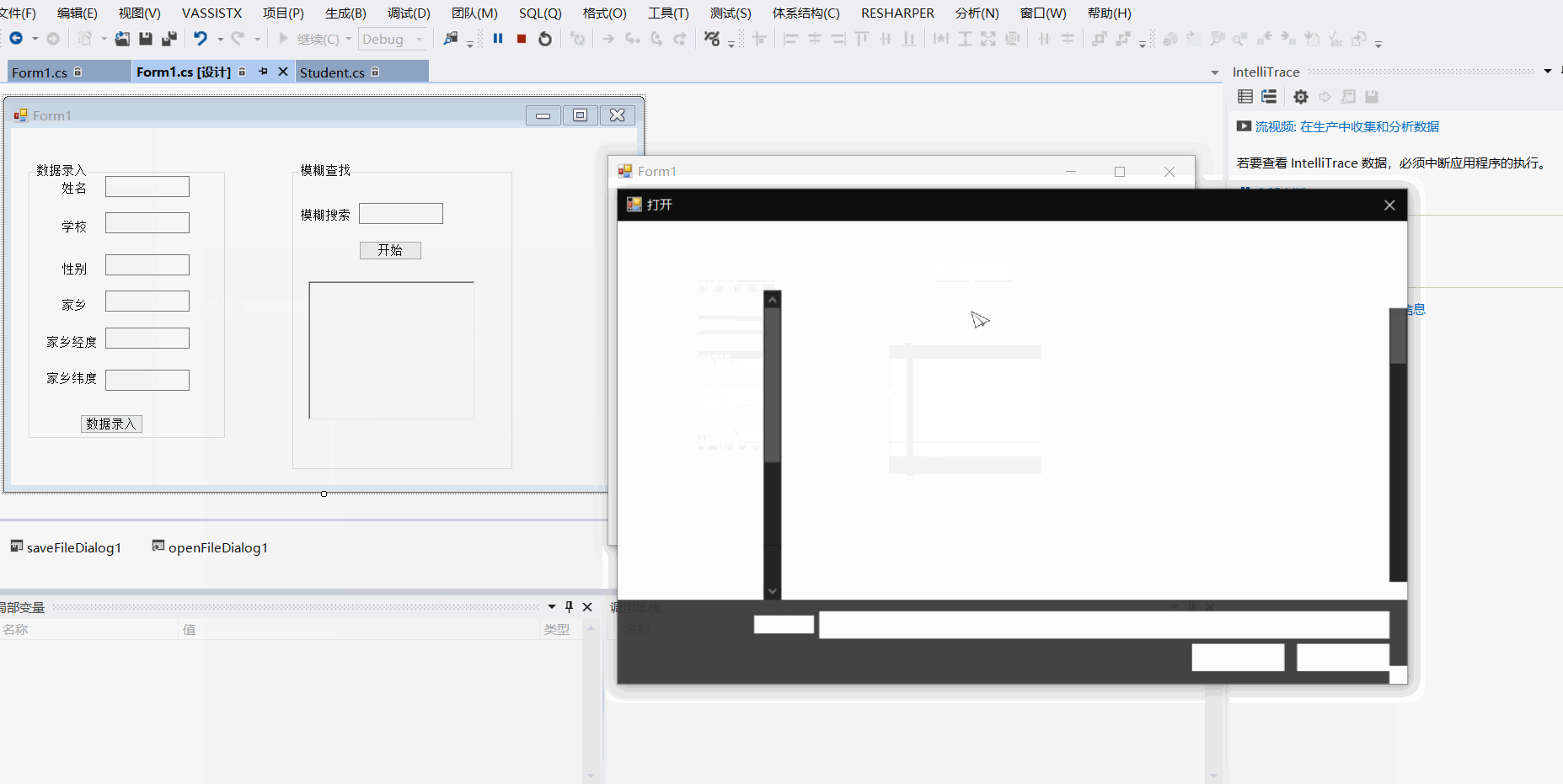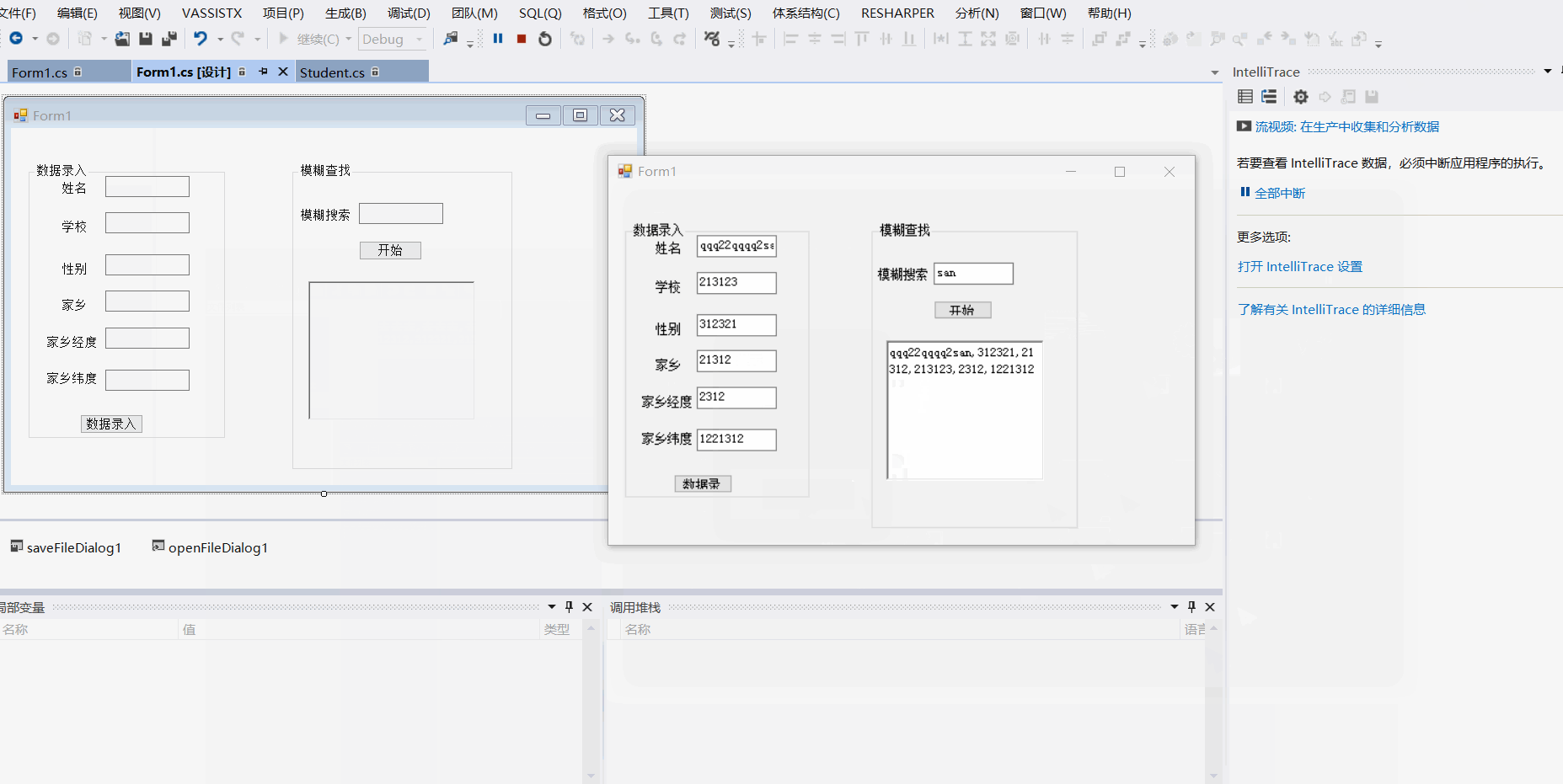
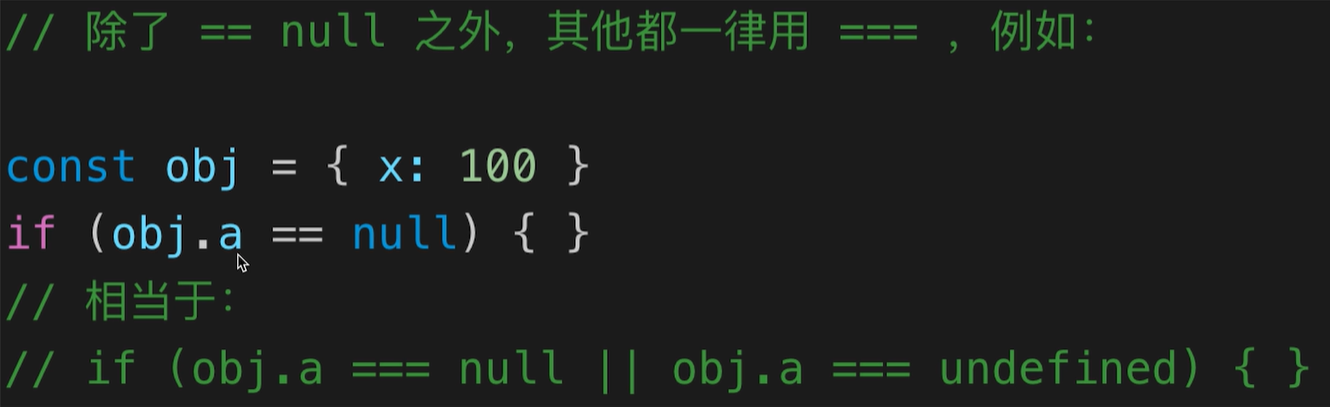
括号。设计一种算法，打印n对括号的所有合法的（例如，开闭一一对应）组合。

说明：解集不能包含重复的子集。

例如，给出 n = 3，生成结果为：

[
“((()))”,
“(()())”,
“(())()”,
“()(())”,
“()()()”
]

法一：回溯法：

class Solution {
public:
    vector<string> generateParenthesis(int n) {
        vector<string> ans;
        string oneAns(n * 2, '\0');
        generate(ans, 0, 0, oneAns, n);

        return ans;
    }

private:
    void generate(vector<string> &ans, int leftNum, int rightNum, string &oneAns, int n)
    {
        if (rightNum == n)
        {
            ans.push_back(oneAns);
            return;
        }

        int curIndex = leftNum + rightNum;

        if (leftNum < n)
        {
            oneAns[curIndex] = '(';
            generate(ans, leftNum + 1, rightNum, oneAns, n);
        }

        if (leftNum > rightNum)
        {
            oneAns[curIndex] = ')';
            generate(ans, leftNum, rightNum + 1, oneAns, n);
        }
    }
};

法二：我们可以把n个括号组成的结果看成(a)b，其中a和b分别是合法的括号，因此我们只需要遍历所有长度的a和b即可：

class Solution {
public:
    vector<string> generateParenthesis(int n) {
        temp.resize(n + 1);
        temp[0] = shared_ptr<vector<string>>(new vector<string>({""}));
        return *generate(n);
    }

private:
    shared_ptr<vector<string>> generate(int length)
    {
        if (length == 0 || temp[length] != nullptr)
        {
            return temp[length];
        }

        temp[length] = shared_ptr<vector<string>>(new vector<string>());
        for (int i = 1; i <= length; ++i)
        {
            shared_ptr<vector<string>> lv = generate(i - 1);
            shared_ptr<vector<string>> rv = generate(length - i);

            for (string &ls : *lv)
            {
                for (string &rs : *rv)
                {
                    temp[length]->push_back("(" + ls + ")" + rs);
                }
            }
        }

        return temp[length];
    }

    vector<shared_ptr<vector<string>>> temp;
};

复杂度同法一。