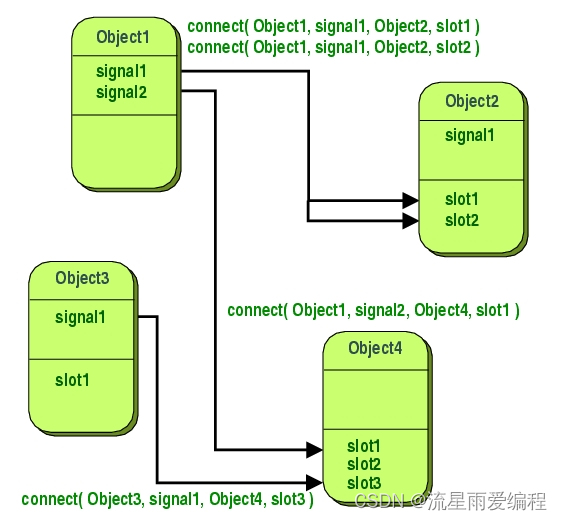
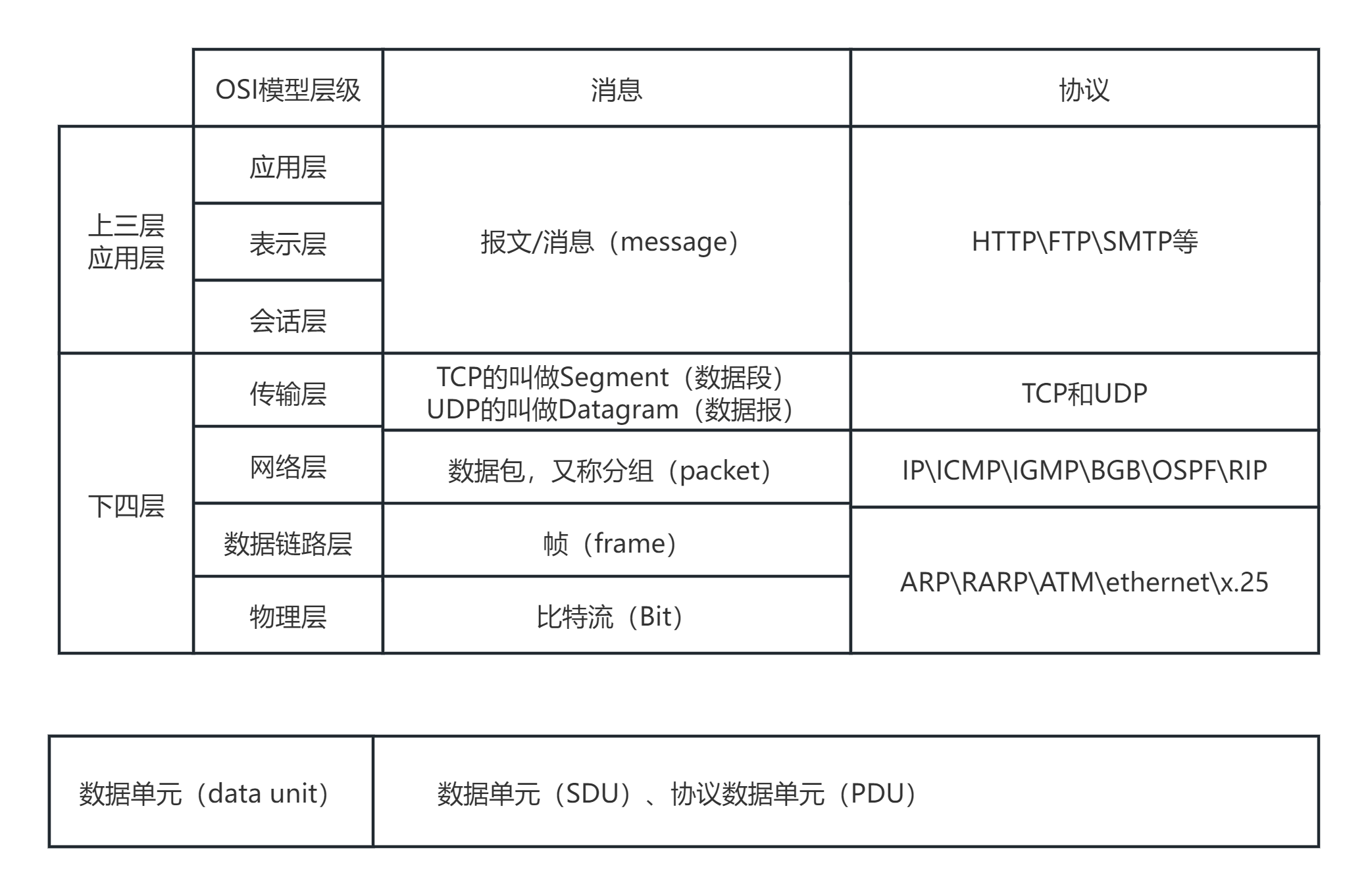
定理6.3.2
如果n是对于基b的强伪素数，则n是对于基b得到欧拉伪素数

n=int(input("给定一奇合数n："))
b=int(input("给定一个整数b："))
def solution(a,b):
   #若b>a，则交换两个数的值
    if(b>a):
        t=a
        a=b
        b=t
    r = b #初始化r
    while(r!=0):
        r = a%b #r为a/b的余数
        a = b
        b = r
    return a  


while(solution(n,b)!=1):
    n=int(input("重新给定一奇合数n"))
    b=int(input("重新给定一个整数b"))

flag=False
s=1
t=(n-1)/pow(2,s)
if pow(b,t)%n==1:
        print('{0}是对于基{1}的强伪素数'.format(n,b))
        flag=True
else:
    while(t%2==0 or t%1!=0 ):#t必须是奇数还必须是整数
        t=(n-1)/pow(2,s)
        s+=1
        if pow(b,t)%n==1:
            print('{0}是对于基{1}的强伪素数'.format(n,b))
            flag=True

##判断欧拉伪素数
if flag==True:
    q=(n-1)/2
    f=((n**2)-1)/8
    if pow(b,q)%n==1 and pow(-1,f)==1:
         print('{0}是对于基{1}的欧拉伪素数'.format(n,b))

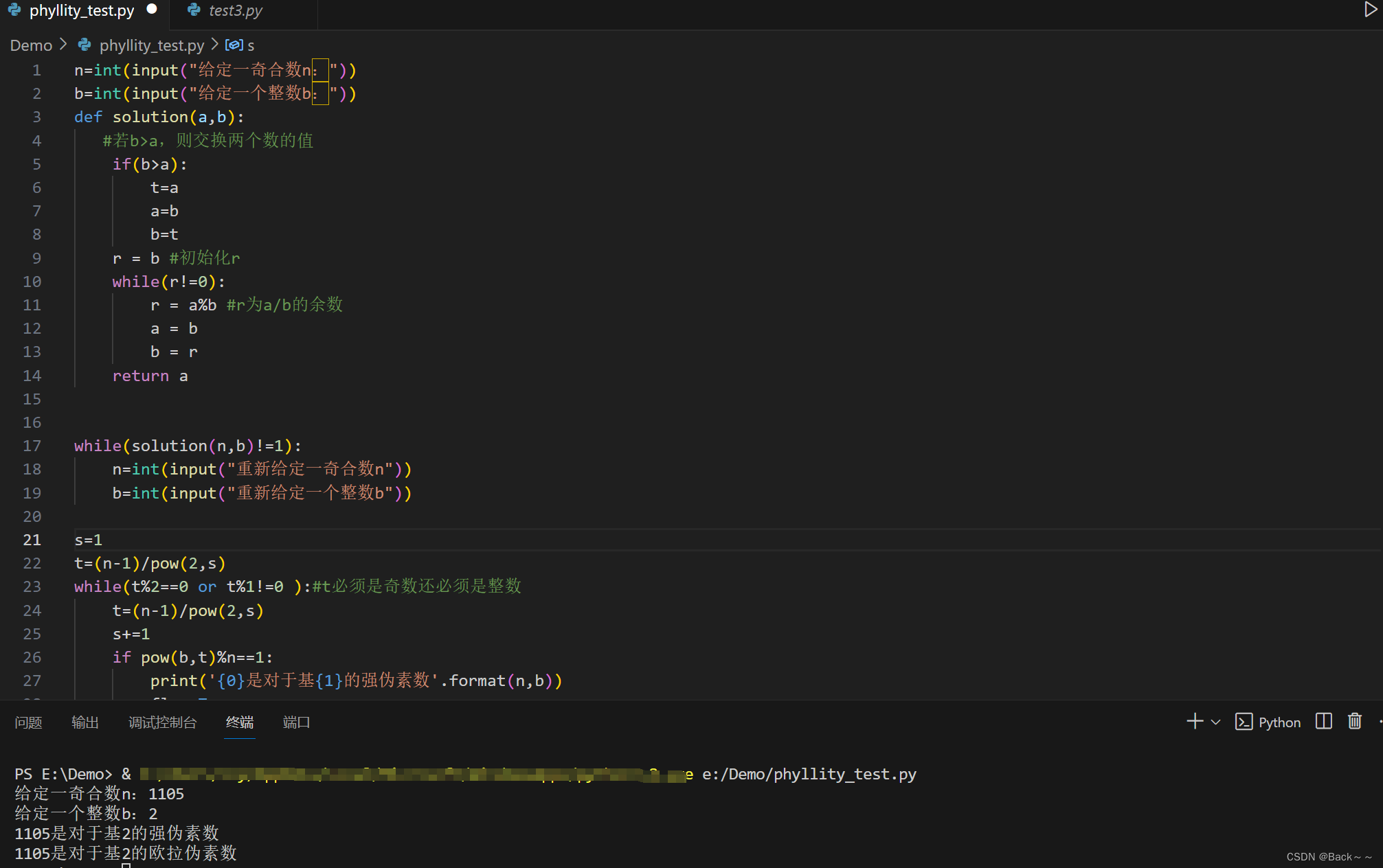
定理6.3.2
设一个奇合数，则n是对于基b的强伪素数的可能性至多为25%

n=int(input("给定一奇合数n："))
def solution(a,b):
   #若b>a，则交换两个数的值
    if(b>a):
        t=a
        a=b
        b=t
    r = b #初始化r
    while(r!=0):
        r = a%b #r为a/b的余数
        a = b
        b = r
    return a  

##我们需要找的是b
b=1
num=0
while(1<=b and b<=(n-1)):
    if solution(n,b)==1:
        ##在这找到t的值
        s=1
        t=(n-1)/pow(2,s)
        if pow(b,t)%n==1:
            num+=1
        while(t%2==0 or t%1!=0 ):#t必须是奇数还必须是整数
            t=(n-1)/pow(2,s)
            s+=1
            if pow(b,t)%n==1:
                num+=1
    b+=1

propertion=(num/(n-1))*100
if propertion<=25:
    print('可能性为{:.2f}%，且少于%25'.format(propertion))