代码随想录算法训练营|二叉树总结

二叉树的定义：

struct TreeNode
{
	int val;
	TreeNode* left;
	TreeNode* right;
	TreeNode():val(0),left(nullptr),right(nullptr){}
	TreeNode(int val):val(val),left(nullptr),right(nullptr){}
	TreeNode(int val,TreeNode* left,TreeNode* right):val(val),left(left),right(right){}
}

其实二叉树就是一种类似链表结构（故打好链表基础十分重要！）。

各种遍历方法

在这里插入图片描述

前序遍历

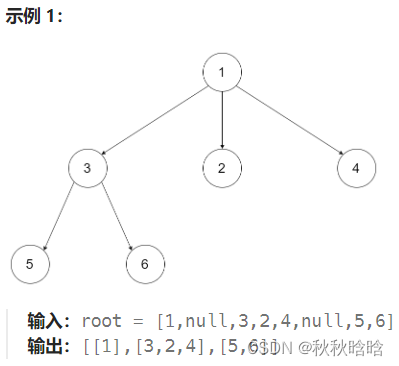
前序遍历的顺序是中左右，用一个图来感受这个过程，前序遍历：5412678

递归法

class Solution {
public:
    vector<int> result;
    void preorder(TreeNode* node)
    {
        if(node==nullptr)return;
        result.push_back(node->val);
        preorder(node->left);
        preorder(node->right);
    }
    vector<int> preorderTraversal(TreeNode* root) {
        result.clear();
        preorder(root);
        return result;
    }
};

迭代法

上面我们采用递归能做出来，递归的本质就是栈，故迭代法可用栈这种数据结构！

class Solution {
public:
    vector<int> preorderTraversal(TreeNode* root) {
        vector<int> result;
        stack<TreeNode*> stk;
        //先判断是否需要push进去，首先需要push一个root进去，不然下面的while循环都进不去
        if(root==nullptr) return result;
        stk.push(root);
        while(!stk.empty())
        {
            TreeNode* node=stk.top();
            stk.pop();
            result.push_back(node->val);
            //一个注意点，应该先push右子树，因为栈的特点是先入后出
            if(node->right)stk.push(node->right);
            if(node->left)stk.push(node->left);
        }
        return result;
    }
};

中序遍历

递归法

中序遍历的顺序为左中右，参考上面的图，应该是1425768

class Solution {
public:
    vector<int> result;
    void inorder(TreeNode* node)
    {
        if(node==nullptr)return;
        preorder(node->left);
        result.push_back(node->val);
        preorder(node->right);
    }
    vector<int> inorderTraversal(TreeNode* root) {
        result.clear();
        inorder(root);
        return result;
    }
};

迭代法

class Solution {
public:
    vector<int> inorderTraversal(TreeNode* root) {
        vector<int> result;
        stack<TreeNode*>stk;
        if(root==nullptr)return result;
        TreeNode* cur=root;
        while(!stk.empty()||cur!=nullptr)
        {
            if(cur)
            {
                stk.push(cur);
                cur=cur->left;
            }
            else
            {
                cur=stk.top();
                stk.pop();
                result.push_back(cur->val);
                cur=cur->right;
            }
        }
        return result;
    }
};

后序遍历

后序遍历：左右中，参考上面的图：1247865

递归法

class Solution {
public:
    vector<int> result;
    void postorder(TreeNode* node)
    {
        if(node==nullptr)return;
        preorder(node->left);
        preorder(node->right);
        result.push_back(node->val);
    }
    vector<int> postorderTraversal(TreeNode* root) {
        result.clear();
        postorder(root);
        return result;
    }
};

迭代法

对比前序遍历顺序：中左右，后序遍历顺序为：左右中，如果我们将顺序变为：中右左，然后对于结果反转一下，是不是就是正确的呢？

class Solution {
public:
    vector<int> postorderTraversal(TreeNode* root) {
        vector<int> result;
        stack<TreeNode*> stk;
        if(root==nullptr)return result;
        stk.push(root);
        while(!stk.empty())
        {
            TreeNode* node=stk.top();
            stk.pop();
            result.push_back(node->val);
            if(node->left)stk.push(node->left);
            if(node->right)stk.push(node->right);
        }
        reverse(result.begin(),result.end());
        return result;
    }
};

层序遍历

其实上文的前中后序遍历，在图论中就是DFS（深度优先搜索），而对应还有一种方法，那就是宽度优先搜索（BFS），其实对于BFS而言，用处还是蛮大的，很多需要遍历整个树的问题，用BFS做起来蛮方便的！当然要想实现BFS，也要借用一种数据结构，其用于存储每一层的元素！这种数据结构就是queue

class Solution {
public:
    vector<vector<int>> levelOrder(TreeNode* root) {
        vector<vector<int>>result;
        queue<TreeNode*> que;
        if(root==nullptr)return result;
        que.push(root);
        while(!que.empty())
        {
            int size=que.size();
            vector<int>vec;
            //在遍历上一层的同时，也将下一层的节点加入queue，这个过程
            while(size--)
            {
                TreeNode* node=que.front();
                que.pop();
                vec.push_back(node->val);
                if(node->left)que.push(node->left);
                if(node->right)que.push(node->right);
            }
            result.push_back(vec);
        }
        return result;
    }
};

二叉树遍历（带有回溯的）

236.二叉树的最近公共祖先

class Solution {
public:
    TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
        if(root==nullptr||root==p||root==q)return root;
        TreeNode* left=lowestCommonAncestor(root->left,p,q);
        TreeNode* right=lowestCommonAncestor(root->right,p,q);
        if(left&&right)return root;
        else if(left==nullptr&&right)return right;
        else if(left&&right==nullptr)return left;
        else return nullptr;
    }
};

做完这道题，明白了公共祖先的问题，都是自顶向上的遍历（采用后序遍历）以及搜索整个树采用：

        TreeNode* left=lowestCommonAncestor(root->left,p,q);
        TreeNode* right=lowestCommonAncestor(root->right,p,q);

同样的一道题：255.二叉搜索树的最近公共祖先，但是这道题还有一个特点，每次要遍历左子树还是右子树的时候可以先进行判断，相当于进行剪枝操作了！

分解问题

将一个二叉树分解为左子树和右子树的问题！
其实要求遍历整个树的问题，很多时候都是分解问题，比较左子树和右子树的问题！
222.完全二叉树的节点个数
这是一道最基本的分解问题，求整个树的个数，分解为左子树加右子树再加根节点！（当然这里还有要遍历整个树要怎么写的问题！）

class Solution {
public:
    int traversal(TreeNode* node)
    {
        if(node==nullptr)return 0;
        int left=traversal(node->left);
        int right=traversal(node->right);
        //为什么这里是1+left+right呢？相当于左子树的个数加上右子树的个数再加上根节点(分解问题)
        return 1+left+right;
    }
    int countNodes(TreeNode* root) {
        return traversal(root);
    }
};

构造二叉树

关键点：在于不断确定中间节点，左子树以及右子树！
105.从前序与中序遍历序列构造二叉树

class Solution {
public:
    TreeNode* traversal(vector<int>& preorder,vector<int>& inorder)
    {
        if(preorder.size()==0)return nullptr;
        int rootvalue=preorder[0];
        TreeNode* root=new TreeNode(rootvalue);
        int index=0;
        for(;index<inorder.size();index++)
        {
            if(inorder[index]==rootvalue)break;
        }
        vector<int> leftinorder(inorder.begin(),inorder.begin()+index);
        vector<int> rightinorder(inorder.begin()+index+1,inorder.end());
        vector<int> leftpreorder(preorder.begin()+1,preorder.begin()+1+leftinorder.size());
        vector<int> rightpreorder(preorder.begin()+1+leftinorder.size(),preorder.end());
        root->left=traversal(leftpreorder,leftinorder);
        root->right=traversal(rightpreorder,rightinorder);
        return root;
    }
    TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {
        if(preorder.size()==0||inorder.size()==0) return nullptr;
        return traversal(preorder,inorder);
    }   
};

做过这道题，可以试试看
106.从中序与后序遍历序列构造二叉树

二叉搜索树

1.有序的问题！（联系中序遍历），求二叉搜索树中第k个最小值！一般做法：采用中序遍历，将二叉树变为有序数组，一定要采用中序遍历！
230.二叉搜索树中第K小的元素

class Solution {
public:
    vector<int> result;
    void inorder(TreeNode* root)
    {
        if(root==nullptr)return;
        inorder(root->left);
        result.push_back(root->val);
        inorder(root->right);
    }
    int kthSmallest(TreeNode* root, int k) {
        inorder(root);
        return result[k-1];
    }
};

98.验证二叉搜索树
这两道题都是将二叉搜索树的问题转换成数组问题来解决的！故对于二叉搜索树的很多问题一定要学会联系中序遍历！

路径问题

插入节点

701.二叉搜索树中的插入操作

class Solution {
public:
    TreeNode* insertIntoBST(TreeNode* root, int val) {
        if(root==nullptr)
        {
            TreeNode* node=new TreeNode(val);
            return node;
        }
        if(root->val<val)
        {
            root->right=insertIntoBST(root->right,val);
        }
        if(root->val>val)
        {
            root->left=insertIntoBST(root->left,val);
        }
        return root;
    }
};

删除节点

450.删除二叉搜索树的节点

class Solution {
public:
    TreeNode* deleteNode(TreeNode* root, int key) {
        if(root==nullptr)return nullptr;
        if(root->val==key)
        {
            if(root->left==nullptr)return root->right;
            else if(root->right==nullptr)return root->left;
            else if(root->left!=nullptr && root->right!=nullptr)
            {
                TreeNode* cur=root->right;
                while(cur->left!=nullptr)
                {
                    cur=cur->left;
                }
                cur->left=root->left;
                TreeNode* temp=root;
                root=root->right;
                delete temp;
                return root;
            }
        }
        if(root->val<key)root->right=deleteNode(root->right,key);
        if(root->val>key)root->left=deleteNode(root->left,key);
        return root;
    }
};