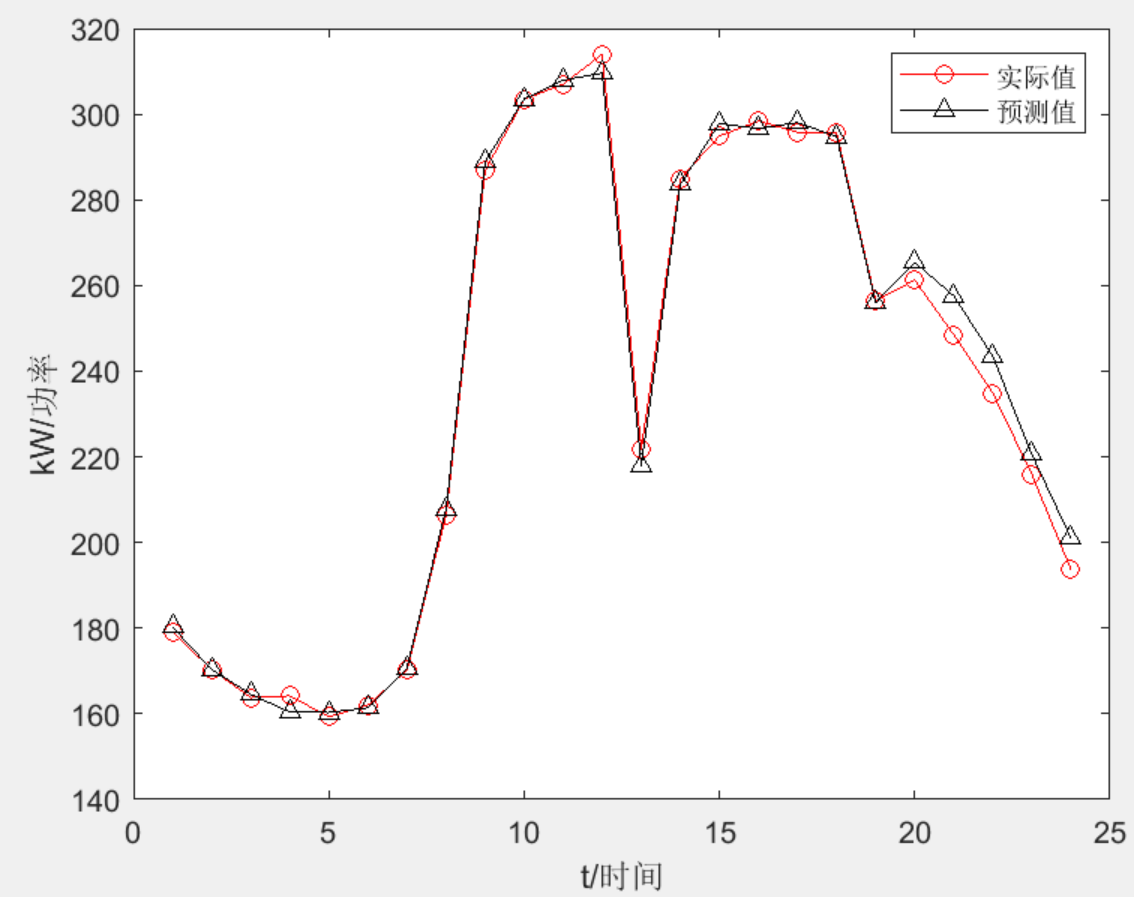
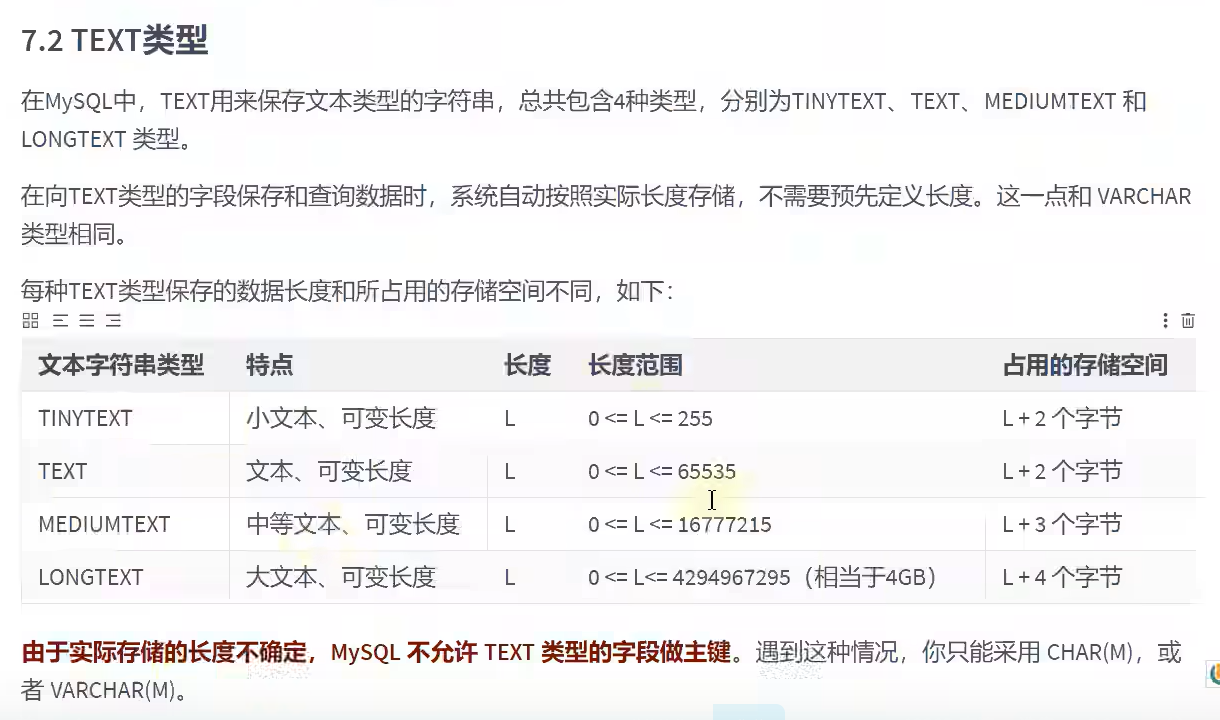
文章目录
- 3. 初始屈服条件
- 3.1 两个假设以及屈服条件基本形式
- 3.2 π \pi π平面、Lode参数
- 3.3 屈服曲线的一般特征
3. 初始屈服条件
3.1 两个假设以及屈服条件基本形式
在简单拉伸时,材料的屈服很明确,即
σ
>
σ
s
(1)
\sigma\gt\sigma_s\tag{1}
σ>σs(1)
那么当一般应力状态下,材料的屈服条件是怎样的形式呢?参考简单拉伸,我们可以写下通用形式的屈服条件
f
(
σ
i
j
)
≥
0
,
屈服
f
(
σ
i
j
)
<
0
,
弹性
(2)
f(\sigma_{ij}) \ge0 \quad, \quad屈服\\ f(\sigma_{ij})\lt 0 \quad, \quad弹性\tag{2}
f(σij)≥0,屈服f(σij)<0,弹性(2)
即当 f ( σ i j ) = 0 f(\sigma_{ij}) =0 f(σij)=0时就是屈服时刻。
我们在讨论一般的金属材料的塑性时,为了简化讨论,先提出两个假设:
1。 材料在初始屈服前,是各项同性的。
2。 静水应力不影响材料的塑性。
因此,屈服只与应力偏量有关,那么式(2)就能转化成如下的形式
f
(
J
1
,
J
2
,
J
3
)
=
0
(3)
f(J_1,J_2,J_3)=0\tag{3}
f(J1,J2,J3)=0(3)
进一步,有
J
1
=
0
J_1=0
J1=0,那么
f
(
J
2
,
J
3
)
=
0
(4)
f(J_2,J_3)=0\tag{4}
f(J2,J3)=0(4)
3.2 π \pi π平面、Lode参数
在主应力空间中,一点的应力状态
(
σ
1
,
σ
2
,
σ
3
)
(\sigma_1,\sigma_2,\sigma_3)
(σ1,σ2,σ3)为P点坐标,那么
O
P
→
=
σ
1
i
1
→
+
σ
2
i
2
→
+
σ
3
i
3
→
(5)
\overrightarrow{OP}=\sigma_1\overrightarrow{i_1}+\sigma_2\overrightarrow{i_2}+\sigma_3\overrightarrow{i_3}\tag{5}
OP=σ1i1+σ2i2+σ3i3(5)
将上式分解
O
P
→
=
σ
1
i
1
→
+
σ
2
i
2
→
+
σ
3
i
3
→
=
σ
m
i
1
→
+
σ
m
i
2
→
+
σ
m
i
3
→
+
s
1
i
1
→
+
s
2
i
2
→
+
s
3
i
3
→
=
O
N
→
+
O
Q
→
(6)
\begin{aligned} \overrightarrow{OP}&=\sigma_1\overrightarrow{i_1}+\sigma_2\overrightarrow{i_2}+\sigma_3\overrightarrow{i_3}\\ &=\sigma_m\overrightarrow{i_1}+\sigma_m\overrightarrow{i_2}+\sigma_m\overrightarrow{i_3}+s_1\overrightarrow{i_1}+s_2\overrightarrow{i_2}+s_3\overrightarrow{i_3}\\ &=\overrightarrow{ON}+\overrightarrow{OQ} \end{aligned}\tag{6}
OP=σ1i1+σ2i2+σ3i3=σmi1+σmi2+σmi3+s1i1+s2i2+s3i3=ON+OQ(6)
上式中,
O
N
→
\overrightarrow{ON}
ON、
O
Q
→
\overrightarrow{OQ}
OQ如下图1所示。
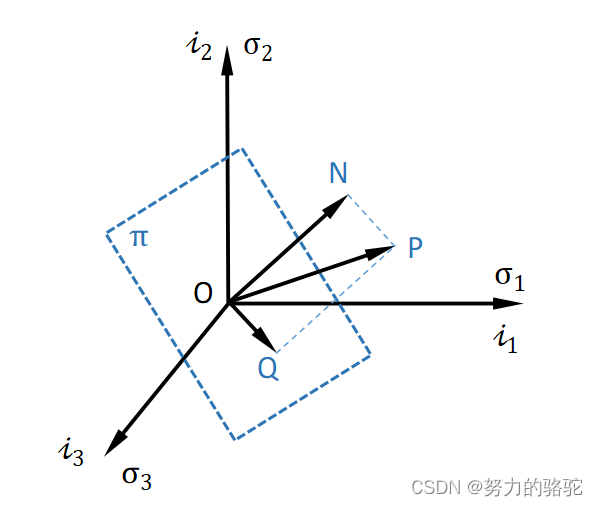
图
1
应力分解图
图1\quad 应力分解图
图1应力分解图
且
O
N
→
⋅
O
Q
→
=
(
σ
m
,
σ
m
,
σ
m
)
⋅
(
s
1
,
s
2
,
s
3
)
=
σ
m
⋅
(
s
1
+
s
2
+
s
3
)
=
0
(7)
\overrightarrow{ON}\cdot\overrightarrow{OQ}=(\sigma_m,\sigma_m,\sigma_m)\cdot (s_1,s_2,s_3)=\sigma_m\cdot(s_1+s_2+s_3)=0\tag{7}
ON⋅OQ=(σm,σm,σm)⋅(s1,s2,s3)=σm⋅(s1+s2+s3)=0(7)
因此
O
N
→
\overrightarrow{ON}
ON、
O
Q
→
\overrightarrow{OQ}
OQ互相垂直,其中
O
N
→
\overrightarrow{ON}
ON的单位向量为
(
1
3
,
1
3
,
1
3
)
(\frac{1}{\sqrt 3},\frac{1}{\sqrt 3},\frac{1}{\sqrt 3})
(31,31,31),以此为法向量,并过原点O的平面方程为
σ
1
+
σ
2
+
σ
3
=
0
(8)
\sigma_1+\sigma_2+\sigma_3=0\tag{8}
σ1+σ2+σ3=0(8)
不难发现,任意的应力状态点
(
σ
1
,
σ
2
,
σ
3
)
(\sigma_1,\sigma_2,\sigma_3)
(σ1,σ2,σ3)在这个平面上的投影点Q在主应力坐标系中的坐标为
(
s
1
,
s
2
,
s
3
)
(s_1,s_2,s_3)
(s1,s2,s3),这代表该应力状态的静水应力部分经过投影变为零。由3.1的第二个假设,静水应力不影响材料的塑性行为,因此只需要在此平面上讨论屈服条件即可,我们把这个平面称为
π
\pi
π平面。
材料屈服条件形式如式(3),因此在 π \pi π平面内讨论式(3)的形式。为了方便讨论,需在 π \pi π平面内建立相应的坐标系。
将主应力空间的基向量 ( i 1 → , i 2 → , i 3 → ) (\overrightarrow{i_1},\overrightarrow{i_2},\overrightarrow{i_3}) (i1,i2,i3)在 π \pi π平面上的投影记为 ( i 1 ′ → , i 2 ′ → , i 3 ′ → ) (\overrightarrow{i_1^{'}},\overrightarrow{i_2^{'}},\overrightarrow{i_3^{'}}) (i1′,i2′,i3′),讨论 i i → \overrightarrow{i_i} ii和 i i ′ → \overrightarrow{i_i^{'}} ii′的关系。以 i 1 → \overrightarrow{i_1} i1为例, i 1 ′ → \overrightarrow{i_1^{'}} i1′为其在 π \pi π平面上的投影,如图2, π \pi π平面法向量 O N → \overrightarrow{ON} ON与 i 1 → \overrightarrow{i_1} i1夹角为 α \alpha α, i 1 → \overrightarrow{i_1} i1和 i 1 ′ → \overrightarrow{i_1^{'}} i1′夹角为 β \beta β,并且 α + β = 9 0 。 \alpha+\beta=90^。 α+β=90。,由 cos α = 1 3 \cos\alpha=\frac{1}{\sqrt 3} cosα=31可知, cos β = 2 3 \cos\beta=\sqrt\frac{2}{ 3} cosβ=32。那么有 ∣ i 1 ′ → ∣ = 2 3 |\overrightarrow{i_1^{'}}|=\sqrt\frac{2}{ 3} ∣i1′∣=32, i 2 ′ → 、 i 3 ′ → \overrightarrow{i_2^{'}}、\overrightarrow{i_3^{'}} i2′、i3′也同理可得,并且不难得出 i 1 ′ → \overrightarrow{i_1^{'}} i1′、 i 2 ′ → \overrightarrow{i_2^{'}} i2′、 i 3 ′ → \overrightarrow{i_3^{'}} i3′在 π \pi π平面上夹角为 12 0 。 120^。 120。。
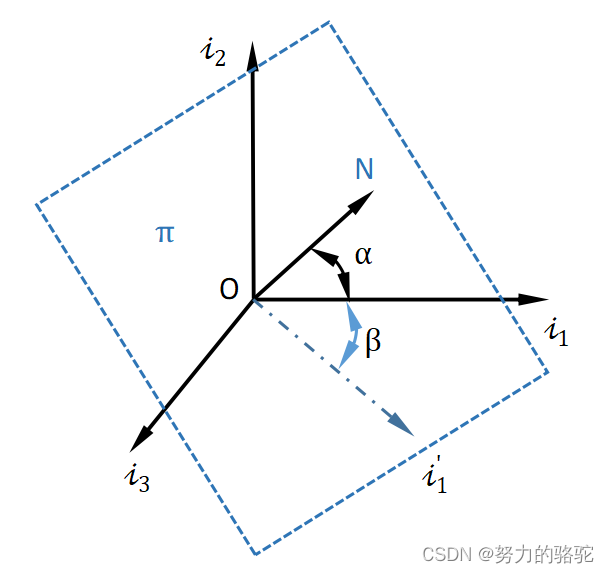
图
2
基向量投影
图2\quad 基向量投影
图2基向量投影
i
1
′
→
\overrightarrow{i_1^{'}}
i1′、
i
2
′
→
\overrightarrow{i_2^{'}}
i2′、
i
3
′
→
\overrightarrow{i_3^{'}}
i3′在
π
\pi
π平面上的投影如图3,其中
i
i
′
→
\overrightarrow{i_i^{'}}
ii′的模为
cos
β
\cos\beta
cosβ。以
i
2
′
→
\overrightarrow{i_2^{'}}
i2′为
y
y
y轴,右手定则确定
x
x
x轴。
O
Q
→
\overrightarrow{OQ}
OQ在主应力空间中分量为
s
1
i
1
→
s_1\overrightarrow{i_1}
s1i1、
s
2
i
2
→
s_2\overrightarrow{i_2}
s2i2、
s
3
i
3
→
s_3\overrightarrow{i_3}
s3i3,那么投影到
π
\pi
π平面上,就是
s
1
i
1
′
→
s_1\overrightarrow{i_1^{'}}
s1i1′、
s
2
i
2
′
→
s_2\overrightarrow{i_2^{'}}
s2i2′、
s
3
i
3
′
→
s_3\overrightarrow{i_3^{'}}
s3i3′,那么在
x
−
y
x-y
x−y坐标系中,将
s
1
i
1
′
→
s_1\overrightarrow{i_1^{'}}
s1i1′、
s
2
i
2
′
→
s_2\overrightarrow{i_2^{'}}
s2i2′、
s
3
i
3
′
→
s_3\overrightarrow{i_3^{'}}
s3i3′写成
(
x
,
y
)
(x,y)
(x,y)分量的形式,如下所示。
s
1
i
1
′
→
=
(
s
1
cos
3
0
。
,
−
s
1
cos
6
0
。
)
⋅
cos
β
s
2
i
2
′
→
=
(
0
,
s
2
)
⋅
cos
β
s
1
i
1
′
→
=
(
−
s
3
cos
6
0
。
,
−
s
3
cos
3
0
。
)
⋅
cos
β
(9)
s_1\overrightarrow{i_1^{'}}=(s_1\cos30^。,-s_1\cos60^。)\cdot\cos\beta\\ s_2\overrightarrow{i_2^{'}}=(0,s_2)\cdot\cos\beta\\ s_1\overrightarrow{i_1^{'}}=(-s_3\cos60^。,-s_3\cos30^。)\cdot\cos\beta\tag{9}
s1i1′=(s1cos30。,−s1cos60。)⋅cosβs2i2′=(0,s2)⋅cosβs1i1′=(−s3cos60。,−s3cos30。)⋅cosβ(9)
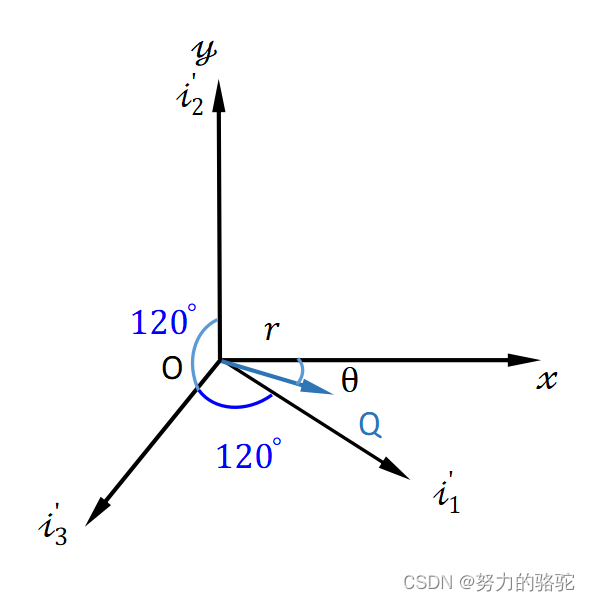
图 3 π 平面中基向量投影 图3\quad \pi平面中基向量投影 图3π平面中基向量投影
因此,
O
Q
→
\overrightarrow{OQ}
OQ在
π
\pi
π平面上的坐标为
x
=
(
s
1
cos
3
0
。
+
0
−
s
3
cos
6
0
。
)
cos
β
=
2
2
(
s
1
−
s
3
)
=
2
2
(
σ
1
−
σ
3
)
y
=
(
−
s
1
cos
6
0
。
+
s
2
−
s
3
cos
3
0
。
)
cos
β
=
1
6
(
2
s
2
−
s
1
−
s
3
)
=
1
6
(
2
σ
2
−
σ
1
−
σ
3
)
(10)
\begin{aligned} &x=(s_1\cos30^。+0-s_3\cos60^。)\cos\beta=\frac{\sqrt2}{2}(s_1-s_3)=\frac{\sqrt2}{2}(\sigma_1-\sigma_3)\\ &y=(-s_1\cos60^。+s_2-s_3\cos30^。)\cos\beta=\frac{1}{\sqrt6}(2s_2-s_1-s_3)=\frac{1}{\sqrt6}(2\sigma_2-\sigma_1-\sigma_3)\end{aligned}\tag{10}
x=(s1cos30。+0−s3cos60。)cosβ=22(s1−s3)=22(σ1−σ3)y=(−s1cos60。+s2−s3cos30。)cosβ=61(2s2−s1−s3)=61(2σ2−σ1−σ3)(10)
如果用极坐标的形式表示,则有
r
=
x
2
+
y
2
=
1
2
(
σ
1
−
σ
3
)
2
+
1
6
(
2
σ
2
−
σ
1
−
σ
3
)
2
tan
θ
=
y
x
=
1
6
(
2
σ
2
−
σ
1
−
σ
3
)
2
2
(
σ
1
−
σ
3
)
=
1
3
⋅
2
σ
2
−
σ
1
−
σ
3
σ
1
−
σ
3
=
1
3
⋅
μ
σ
(11)
\begin{aligned} &r=\sqrt{x^2+y^2}=\sqrt{\frac{1}{2}(\sigma_1-\sigma_3)^2+\frac{1}{6}(2\sigma_2-\sigma_1-\sigma_3)^2}\\ &\tan\theta=\frac{y}{x}=\frac{ \frac{1}{\sqrt6}(2\sigma_2-\sigma_1-\sigma_3) }{ \frac{\sqrt2}{2}(\sigma_1-\sigma_3) }=\frac{1}{\sqrt3}\cdot\frac{2\sigma_2-\sigma_1-\sigma_3}{\sigma_1-\sigma_3}=\frac{1}{\sqrt3}\cdot\mu_\sigma \end{aligned}\tag{11}
r=x2+y2=21(σ1−σ3)2+61(2σ2−σ1−σ3)2tanθ=xy=22(σ1−σ3)61(2σ2−σ1−σ3)=31⋅σ1−σ32σ2−σ1−σ3=31⋅μσ(11)
其中 μ σ = 2 σ 2 − σ 1 − σ 3 σ 1 − σ 3 \mu_\sigma=\frac{2\sigma_2-\sigma_1-\sigma_3}{\sigma_1-\sigma_3} μσ=σ1−σ32σ2−σ1−σ3称为Lode参数,它表示了主应力之间的相对比值。
3.3 屈服曲线的一般特征
现在可以讨论屈服条件,在主应力空间中屈服条件 f ( σ i j ) = f ( J 2 , J 3 ) = 0 f(\sigma_{ij})=f(J_2,J_3)=0 f(σij)=f(J2,J3)=0形成一个以 O N → \overrightarrow{ON} ON为母线的柱面(静水压力不影响塑性)。柱面和 π \pi π平面形成的截面线称为屈服曲线。
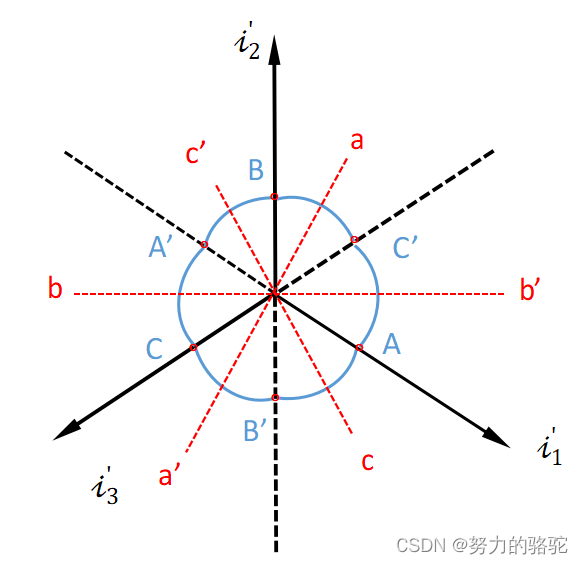
根据3.1的第一个假设:材料在初始屈服前,是各项同性的,那么屈服曲线肯定式关于三个坐标轴对称的,三个坐标轴正方向在 π \pi π平面上是夹角是一样的为 12 0 。 120^。 120。,三个坐标轴负方向在 π \pi π平面上是夹角是一样为 12 0 。 120^。 120。,那么一个坐标轴的负方向和其他两个坐标轴的正方向夹 6 0 。 60^。 60。,如图4。
屈服曲线关于坐标轴正方向对称,那么屈服曲线对称角度为 12 0 。 120^。 120。,同时屈服曲线关于坐标轴负方向对称,那么屈服曲线对称角度为 6 0 。 60^。 60。。即在图中 A C ′ ⌢ \overset{\LARGE{\frown}}{AC'} AC′⌢、 A B ′ ⌢ \overset{\LARGE{\frown}}{AB'} AB′⌢对称,同时 A C ′ ⌢ \overset{\LARGE{\frown}}{AC'} AC′⌢、 B C ′ ⌢ \overset{\LARGE{\frown}}{BC'} BC′⌢对称,因此只需要知道 6 0 。 60^。 60。的曲线,就能完整确定整条曲线。
同时,在各项同性的同时,假设压缩和拉伸性能相等,则
A
C
′
⌢
\overset{\LARGE{\frown}}{AC'}
AC′⌢自己是关于bb’对称的,那么只需要知道
3
0
。
30^。
30。的曲线就能完整确定整条曲线。
图
4
π
平面中坐标轴投影
图4\quad \pi平面中坐标轴投影
图4π平面中坐标轴投影