本文仅供学习使用,总结很多本现有讲述运动学或动力学书籍后的总结,从矢量的角度进行分析,方法比较传统,但更易理解,并且现有的看似抽象方法,两者本质上并无不同。
2024年底本人学位论文发表后方可摘抄
若有帮助请引用
本文参考:
食用方法
坐标系的组成与表达方式
点的运动在不同三维坐标系中的表达
广义坐标系的推广
点的表达与向量表达,及其不同点
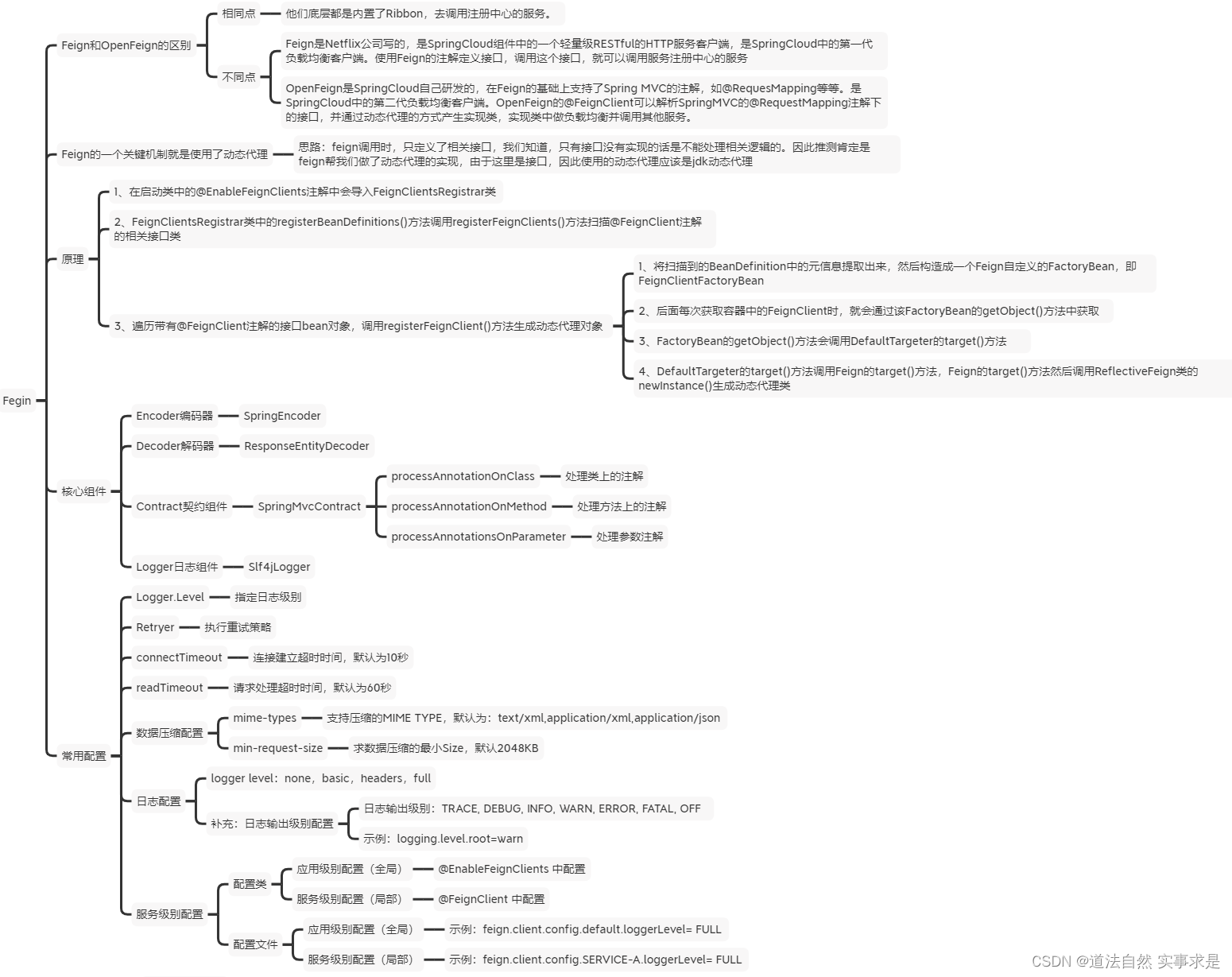
机构运动学与动力学分析与建模 Ch00-1 坐标系与概念基准
- 1. 空间坐标系
- 1.1 笛卡尔坐标系 Cartesian coordinate system
- 1.2 点Partical的运动与表达
- 1.2.1 笛卡尔直角坐标系
- 1.2.2 笛卡尔柱坐标系
- 1.2.3 笛卡尔球坐标系
- 1.2.4 曲线坐标系:Frenet标架(详见微分几何-曲线论内容)
- 1.2.5 广义坐标系 Generalized coordinates system
- 1.3 矢量Vector在坐标系下的表示与关系转换
1. 空间坐标系
坐标系Coordinates是一个用于描述
n
n
n维系统中某一状态参数的坐标表示系统:对于同一
n
n
n维系统的状态参数可用不同的坐标系进行表示,即具有不同的 基底Basis(基矢量) ;而对于不同的坐标系而言,表示同一状态参数存在对应关系,因此坐标系之间也存在着 变化关系,这种变化关系的本质是不同坐标系的基底之间的转换。
-
坐标系的表达: { F } \left\{ F \right\} {F}, { M } \left\{ M \right\} {M},其中“ { } \{\} {}”符号特指坐标系,一般用 { F } \left\{ F \right\} {F}表示
固定坐标系 Fixed, { M } \left\{ M \right\} {M}表示运动坐标系 Moving;对于部分坐标系也可以称为标架Frame。 -
基矢量的表达: i ^ , j ^ , k ^ \hat{i},\hat{j},\hat{k} i^,j^,k^,其中 “ ^ \hat{ } ^ ” 符号特指基矢量;对于固定坐标系 { F } \left\{ F \right\} {F},其基矢量特定为: I ^ , J ^ , K ^ \hat{I},\hat{J},\hat{K} I^,J^,K^,对于其他运动坐标系而言,以坐标系 { M } \left\{ M \right\} {M}为例,其基矢量可写成: i ^ M , j ^ M , k ^ M \hat{i}^M,\hat{j}^M,\hat{k}^M i^M,j^M,k^M
-
标架的表达:以 { M } \left\{ M \right\} {M}为例,其运动标架可表示为: { M : ( i ^ M , j ^ M , k ^ M ) } \left\{ M:\left( \hat{i}^M,\hat{j}^M,\hat{k}^M \right) \right\} {M:(i^M,j^M,k^M)}
当坐标系的维数 n = 3 n=3 n=3 时,便称为空间坐标系。
1.1 笛卡尔坐标系 Cartesian coordinate system
笛卡尔坐标系是我们在三维空间中常用的表示空间运动的坐标系,基于观测位置与对象的不同,还有GPS坐标系 等其他表达方式。对于任一笛卡尔坐标系,视其基矢量为 X ^ 1 , X ^ 2 , X ^ 3 \hat{X}_1,\hat{X}_2,\hat{X}_3 X^1,X^2,X^3,对于该空间内任一点 P P P 都可以这组基矢量进行表达。
1.2 点Partical的运动与表达
对于空间中任意一点
P
P
P而言,其位置Position表述该系统空间的一种状态参数,因此其在各基矢量上的标量分量即为对应的坐标参数。因此该点
P
P
P在笛卡尔坐标系中的表达为:
R
⃗
P
X
=
P
⃗
=
P
1
X
^
1
+
P
2
X
^
2
+
P
3
X
^
3
\vec{R}_{\mathrm{P}}^{X}=\vec{P}=P_1\hat{X}_1+P_2\hat{X}_2+P_3\hat{X}_3
RPX=P=P1X^1+P2X^2+P3X^3
1.2.1 笛卡尔直角坐标系
{
F
:
(
X
^
1
,
X
^
2
,
X
^
3
)
}
=
{
F
:
(
I
^
,
J
^
,
K
^
)
}
\left\{ F:\left( \hat{X}_1,\hat{X}_2,\hat{X}_3 \right) \right\} =\left\{ F:\left( \hat{I},\hat{J},\hat{K} \right) \right\}
{F:(X^1,X^2,X^3)}={F:(I^,J^,K^)}

对于状态空间中一点
P
P
P,其在固定坐标系标架
{
F
:
(
I
^
,
J
^
,
K
^
)
}
\left\{ F:\left( \hat{I},\hat{J},\hat{K} \right) \right\}
{F:(I^,J^,K^)}上基矢量的投影参数为
(
P
1
,
P
2
,
P
3
)
\left( P_1,P_2,P_3 \right)
(P1,P2,P3),因此可将点
P
P
P 在笛卡尔直角坐标系中进行表述
R
⃗
P
F
=
P
1
I
^
+
P
2
J
^
+
P
3
K
^
=
[
I
^
J
^
K
^
]
T
[
P
1
P
2
P
3
]
\vec{R}_{\mathrm{P}}^{F}=P_1\hat{I}+P_2\hat{J}+P_3\hat{K}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} P_1\\ P_2\\ P_3\\ \end{array} \right]
RPF=P1I^+P2J^+P3K^=
I^J^K^
T
P1P2P3
进而可以求解其速度Velocity参数
V
⃗
P
F
\vec{V}_{\mathrm{P}}^{F}
VPF 为:
V
⃗
P
F
=
R
⃗
˙
P
F
=
d
R
⃗
P
F
d
t
=
[
I
^
˙
↗
0
J
^
˙
↗
0
K
^
˙
↗
0
]
T
[
P
1
P
2
P
3
]
+
[
I
^
J
^
K
^
]
T
[
P
˙
1
P
˙
2
P
˙
3
]
\vec{V}_{\mathrm{P}}^{F}=\dot{\vec{R}}_{\mathrm{P}}^{F}=\frac{\mathrm{d}\vec{R}_{\mathrm{P}}^{F}}{\mathrm{dt}}=\left[ \begin{array}{c} \dot{\hat{I}}_{\nearrow 0}\\ \dot{\hat{J}}_{\nearrow 0}\\ \dot{\hat{K}}_{\nearrow 0}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} P_1\\ P_2\\ P_3\\ \end{array} \right] +\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \dot{P}_1\\ \dot{P}_2\\ \dot{P}_3\\ \end{array} \right]
VPF=R˙PF=dtdRPF=
I^˙↗0J^˙↗0K^˙↗0
T
P1P2P3
+
I^J^K^
T
P˙1P˙2P˙3
其加速度acceleration参数
a
⃗
P
F
\vec{a}_{\mathrm{P}}^{F}
aPF 为:
a
⃗
P
F
=
V
⃗
˙
P
F
=
R
⃗
¨
P
F
=
d
V
⃗
P
F
d
t
=
[
I
^
˙
↗
0
J
^
˙
↗
0
K
^
˙
↗
0
]
T
[
P
˙
1
P
˙
2
P
˙
3
]
+
[
I
^
J
^
K
^
]
T
[
P
¨
1
P
¨
2
P
¨
3
]
\vec{a}_{\mathrm{P}}^{F}=\dot{\vec{V}}_{\mathrm{P}}^{F}=\ddot{\vec{R}}_{\mathrm{P}}^{F}=\frac{\mathrm{d}\vec{V}_{\mathrm{P}}^{F}}{\mathrm{dt}}=\left[ \begin{array}{c} \dot{\hat{I}}_{\nearrow 0}\\ \dot{\hat{J}}_{\nearrow 0}\\ \dot{\hat{K}}_{\nearrow 0}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \dot{P}_1\\ \dot{P}_2\\ \dot{P}_3\\ \end{array} \right] +\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \ddot{P}_1\\ \ddot{P}_2\\ \ddot{P}_3\\ \end{array} \right]
aPF=V˙PF=R¨PF=dtdVPF=
I^˙↗0J^˙↗0K^˙↗0
T
P˙1P˙2P˙3
+
I^J^K^
T
P¨1P¨2P¨3
1.2.2 笛卡尔柱坐标系
{
F
:
(
X
^
1
,
X
^
2
,
X
^
3
)
}
=
{
F
:
(
X
^
r
,
X
^
θ
,
K
^
)
}
\left\{ F:\left( \hat{X}_1,\hat{X}_2,\hat{X}_3 \right) \right\} =\left\{ F:\left( \hat{X}_{\mathrm{r}},\hat{X}_{\mathrm{\theta}},\hat{K} \right) \right\}
{F:(X^1,X^2,X^3)}={F:(X^r,X^θ,K^)}

对于不同的坐标系,点
P
P
P 在状态空间中并没有发生变化,而由于基矢量的变化导致其投影参数发生改变。在柱坐标系中,点
P
P
P 表述为:
R
⃗
P
C
=
P
⃗
=
P
1
′
X
^
r
+
P
2
′
X
^
θ
+
P
3
′
K
^
\vec{R}_{\mathrm{P}}^{\mathrm{C}}=\vec{P}=P_1\mathrm{'}\hat{X}_{\mathrm{r}}+P_2\mathrm{'}\hat{X}_{\theta}+P_3\mathrm{'}\hat{K}
RPC=P=P1′X^r+P2′X^θ+P3′K^
对于投影参数而言,
P
1
′
P_1\mathrm{'}
P1′表示
X
^
r
\hat{X}_{\mathrm{r}}
X^r方向上的长度参数,而
P
2
′
P_2\mathrm{'}
P2′表示
X
^
θ
\hat{X}_{\mathrm{\theta}}
X^θ方向上的角度参数,而单纯的角度参数在实际的矢量运算过程中是比较难于理解的,因此对柱坐标系而言,实际上是将该方向上的已知投影参数转换到直角坐标系下进行表示
若已知柱坐标系下点
P
P
P 的投影参数
P
=
(
r
,
θ
,
k
)
P=\left( r,\theta ,k \right)
P=(r,θ,k),其位置方程在直角坐标系下的表示为:
R
⃗
P
C
=
P
⃗
=
r
cos
θ
I
^
+
r
sin
θ
J
^
+
k
K
^
\vec{R}_{\mathrm{P}}^{\mathrm{C}}=\vec{P}=r\cos \theta \hat{I}+r\sin \theta \hat{J}+k\hat{K}
RPC=P=rcosθI^+rsinθJ^+kK^
可视为:
[
P
1
P
2
P
3
]
=
[
r
cos
θ
r
sin
θ
k
]
\left[ \begin{array}{c} P_1\\ P_2\\ P_3\\ \end{array} \right] =\left[ \begin{array}{c} r\cos \theta\\ r\sin \theta\\ k\\ \end{array} \right]
P1P2P3
=
rcosθrsinθk
,对速度参数
V
⃗
P
C
\vec{V}_{\mathrm{P}}^{\mathrm{C}}
VPC进行求解:
V
⃗
P
C
=
R
⃗
˙
P
C
=
d
R
⃗
P
C
d
t
=
[
I
^
J
^
K
^
]
T
[
P
˙
1
P
˙
2
P
˙
3
]
=
[
I
^
J
^
K
^
]
T
[
d
r
d
t
cos
θ
−
r
d
θ
d
t
sin
θ
d
r
d
t
sin
θ
+
r
d
θ
d
t
cos
θ
d
k
d
t
]
\vec{V}_{\mathrm{P}}^{\mathrm{C}}=\dot{\vec{R}}_{\mathrm{P}}^{\mathrm{C}}=\frac{\mathrm{d}\vec{R}_{\mathrm{P}}^{\mathrm{C}}}{\mathrm{dt}}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \dot{P}_1\\ \dot{P}_2\\ \dot{P}_3\\ \end{array} \right] =\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \frac{\mathrm{d}r}{\mathrm{dt}}\cos \theta -r\frac{\mathrm{d}\theta}{\mathrm{dt}}\sin \theta\\ \frac{\mathrm{d}r}{\mathrm{dt}}\sin \theta +r\frac{\mathrm{d}\theta}{\mathrm{dt}}\cos \theta\\ \frac{\mathrm{d}k}{\mathrm{dt}}\\ \end{array} \right]
VPC=R˙PC=dtdRPC=
I^J^K^
T
P˙1P˙2P˙3
=
I^J^K^
T
dtdrcosθ−rdtdθsinθdtdrsinθ+rdtdθcosθdtdk
当
d
r
d
t
=
0
\frac{\mathrm{d}r}{\mathrm{dt}}=0
dtdr=0,
d
k
d
t
=
0
\frac{\mathrm{d}k}{\mathrm{dt}}=0
dtdk=0 时,即点
P
P
P 不在矢径方向上运动,仅绕
K
^
\hat{K}
K^ 进行平面上的纯回转,可将上式简化为:
V
⃗
P
C
∣
d
r
d
t
=
0
,
d
k
d
t
=
0
=
[
I
^
J
^
K
^
]
T
[
−
r
d
θ
d
t
sin
θ
r
d
θ
d
t
cos
θ
0
]
=
r
θ
˙
[
I
^
J
^
K
^
]
T
[
−
sin
θ
cos
θ
0
]
\left. \vec{V}_{\mathrm{P}}^{\mathrm{C}} \right|_{\frac{\mathrm{d}r}{\mathrm{dt}}=0,\frac{\mathrm{d}k}{\mathrm{dt}}=0}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} -r\frac{\mathrm{d}\theta}{\mathrm{dt}}\sin \theta\\ r\frac{\mathrm{d}\theta}{\mathrm{dt}}\cos \theta\\ 0\\ \end{array} \right] =r\dot{\theta}\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} -\sin \theta\\ \cos \theta\\ 0\\ \end{array} \right]
VPC
dtdr=0,dtdk=0=
I^J^K^
T
−rdtdθsinθrdtdθcosθ0
=rθ˙
I^J^K^
T
−sinθcosθ0
对上式进一步求解其加速度参数
a
⃗
P
C
∣
d
r
d
t
=
0
,
d
k
d
t
=
0
\left. \vec{a}_{\mathrm{P}}^{\mathrm{C}} \right|_{\frac{\mathrm{d}r}{\mathrm{dt}}=0,\frac{\mathrm{d}k}{\mathrm{dt}}=0}
aPC
dtdr=0,dtdk=0:
a
⃗
P
C
∣
d
r
d
t
=
0
,
d
k
d
t
=
0
=
r
θ
¨
[
I
^
J
^
K
^
]
T
[
−
sin
θ
cos
θ
0
]
+
r
θ
˙
2
[
I
^
J
^
K
^
]
T
[
−
cos
θ
−
sin
θ
0
]
=
α
⃗
P
C
×
R
⃗
P
C
+
ω
⃗
P
C
×
V
⃗
P
C
\left. \vec{a}_{\mathrm{P}}^{\mathrm{C}} \right|_{\frac{\mathrm{d}r}{\mathrm{dt}}=0,\frac{\mathrm{d}k}{\mathrm{dt}}=0}=r\ddot{\theta}\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} -\sin \theta\\ \cos \theta\\ 0\\ \end{array} \right] +r\dot{\theta}^2\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} -\cos \theta\\ -\sin \theta\\ 0\\ \end{array} \right] =\vec{\alpha}_{\mathrm{P}}^{\mathrm{C}}\times \vec{R}_{\mathrm{P}}^{\mathrm{C}}+\vec{\omega}_{\mathrm{P}}^{\mathrm{C}}\times \vec{V}_{\mathrm{P}}^{\mathrm{C}}
aPC
dtdr=0,dtdk=0=rθ¨
I^J^K^
T
−sinθcosθ0
+rθ˙2
I^J^K^
T
−cosθ−sinθ0
=αPC×RPC+ωPC×VPC
若考虑真实的向量表达,则柱坐标系中,点
P
P
P 还可以表述为:
R
⃗
P
C
=
r
(
θ
)
X
^
r
(
θ
)
+
k
K
^
\vec{R}_{\mathrm{P}}^{\mathrm{C}}=r\left( \theta \right) \hat{X}_{\mathrm{r}\left( \theta \right)}+k\hat{K}
RPC=r(θ)X^r(θ)+kK^
其中:
[
X
^
r
X
^
θ
]
=
[
cos
θ
sin
θ
−
sin
θ
cos
θ
]
[
I
^
J
^
]
\left[ \begin{array}{c} \hat{X}_{\mathrm{r}}\\ \hat{X}_{\mathrm{\theta}}\\ \end{array} \right] =\left[ \begin{matrix} \cos \theta& \sin \theta\\ -\sin \theta& \cos \theta\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \end{array} \right]
[X^rX^θ]=[cosθ−sinθsinθcosθ][I^J^]
进而可得:
[
X
^
˙
r
X
^
˙
θ
]
=
[
cos
θ
−
θ
˙
sin
θ
sin
θ
+
θ
˙
cos
θ
−
sin
θ
−
θ
˙
cos
θ
cos
θ
−
θ
˙
sin
θ
]
[
I
^
J
^
]
=
[
0
θ
˙
−
θ
˙
0
]
[
X
^
r
X
^
θ
]
\left[ \begin{array}{c} \dot{\hat{X}}_{\mathrm{r}}\\ \dot{\hat{X}}_{\mathrm{\theta}}\\ \end{array} \right] =\left[ \begin{matrix} \cos \theta -\dot{\theta}\sin \theta& \sin \theta +\dot{\theta}\cos \theta\\ -\sin \theta -\dot{\theta}\cos \theta& \cos \theta -\dot{\theta}\sin \theta\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \end{array} \right] =\left[ \begin{matrix} 0& \dot{\theta}\\ -\dot{\theta}& 0\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{X}_{\mathrm{r}}\\ \hat{X}_{\mathrm{\theta}}\\ \end{array} \right]
[X^˙rX^˙θ]=[cosθ−θ˙sinθ−sinθ−θ˙cosθsinθ+θ˙cosθcosθ−θ˙sinθ][I^J^]=[0−θ˙θ˙0][X^rX^θ]
对于
X
^
r
\hat{X}_{\mathrm{r}}
X^r与
X
^
θ
\hat{X}_{\theta}
X^θ而言有:
X
^
˙
r
=
θ
˙
X
^
θ
,
X
^
θ
=
−
θ
˙
X
^
˙
r
\dot{\hat{X}}_{\mathrm{r}}=\dot{\theta}\hat{X}_{\theta},\hat{X}_{\theta}=-\dot{\theta}\dot{\hat{X}}_{\mathrm{r}}
X^˙r=θ˙X^θ,X^θ=−θ˙X^˙r,此处的
X
^
θ
\hat{X}_{\theta}
X^θ表示的是垂直于基矢量
X
^
r
\hat{X}_{\mathrm{r}}
X^r的切矢量,与
X
^
θ
\hat{X}_{\theta}
X^θ不同。
虽然在三维系统中正常应该具有三个基矢量,而在上式中只有两个基矢量,但其投影参数与矢径上的基矢量为另一个参数
θ
\theta
θ的函数,因此该式为真实表达形式,同样可得其速度与加速度参数为:
{
V
⃗
P
C
=
R
⃗
˙
P
C
=
r
˙
X
^
r
+
r
X
^
˙
r
+
k
˙
K
^
=
r
˙
X
^
r
+
r
θ
˙
X
^
θ
+
k
˙
K
^
a
⃗
P
C
=
V
⃗
˙
P
C
=
r
¨
X
^
r
+
r
˙
X
^
˙
r
+
r
˙
θ
˙
X
^
θ
+
r
θ
¨
X
^
θ
+
r
θ
˙
X
^
˙
θ
+
k
¨
K
^
=
r
¨
X
^
r
+
r
˙
θ
˙
X
^
θ
+
r
˙
θ
˙
X
^
θ
+
r
θ
¨
X
^
θ
−
r
θ
˙
2
X
^
r
+
k
¨
K
^
\left\{ \begin{array}{c} \vec{V}_{\mathrm{P}}^{\mathrm{C}}=\dot{\vec{R}}_{\mathrm{P}}^{\mathrm{C}}=\dot{r}\hat{X}_{\mathrm{r}}+r\dot{\hat{X}}_{\mathrm{r}}+\dot{k}\hat{K}=\dot{r}\hat{X}_{\mathrm{r}}+r\dot{\theta}\hat{X}_{\mathrm{\theta}}+\dot{k}\hat{K}\\ \vec{a}_{\mathrm{P}}^{\mathrm{C}}=\dot{\vec{V}}_{\mathrm{P}}^{\mathrm{C}}=\ddot{r}\hat{X}_{\mathrm{r}}+\dot{r}\dot{\hat{X}}_{\mathrm{r}}+\dot{r}\dot{\theta}\hat{X}_{\mathrm{\theta}}+r\ddot{\theta}\hat{X}_{\mathrm{\theta}}+r\dot{\theta}\dot{\hat{X}}_{\mathrm{\theta}}+\ddot{k}\hat{K}=\ddot{r}\hat{X}_{\mathrm{r}}+\dot{r}\dot{\theta}\hat{X}_{\mathrm{\theta}}+\dot{r}\dot{\theta}\hat{X}_{\mathrm{\theta}}+r\ddot{\theta}\hat{X}_{\mathrm{\theta}}-r\dot{\theta}^2\hat{X}_{\mathrm{r}}+\ddot{k}\hat{K}\\ \end{array} \right.
⎩
⎨
⎧VPC=R˙PC=r˙X^r+rX^˙r+k˙K^=r˙X^r+rθ˙X^θ+k˙K^aPC=V˙PC=r¨X^r+r˙X^˙r+r˙θ˙X^θ+rθ¨X^θ+rθ˙X^˙θ+k¨K^=r¨X^r+r˙θ˙X^θ+r˙θ˙X^θ+rθ¨X^θ−rθ˙2X^r+k¨K^
对上式)进行化简,可得:
{
V
⃗
P
C
=
r
˙
X
^
r
+
r
θ
˙
X
^
θ
+
k
˙
K
^
a
⃗
P
C
=
(
r
¨
−
r
θ
˙
2
)
X
^
r
+
(
2
r
˙
θ
˙
+
r
θ
¨
)
X
^
θ
+
k
¨
K
^
\left\{ \begin{array}{c} \vec{V}_{\mathrm{P}}^{\mathrm{C}}=\dot{r}\hat{X}_{\mathrm{r}}+r\dot{\theta}\hat{X}_{\theta}+\dot{k}\hat{K}\\ \vec{a}_{\mathrm{P}}^{\mathrm{C}}=\left( \ddot{r}-r\dot{\theta}^2 \right) \hat{X}_{\mathrm{r}}+\left( 2\dot{r}\dot{\theta}+r\ddot{\theta} \right) \hat{X}_{\theta}+\ddot{k}\hat{K}\\ \end{array} \right.
{VPC=r˙X^r+rθ˙X^θ+k˙K^aPC=(r¨−rθ˙2)X^r+(2r˙θ˙+rθ¨)X^θ+k¨K^
其中:
r
θ
¨
r\ddot{\theta}
rθ¨ 称为欧拉项Eulerian term,
2
r
˙
θ
˙
2\dot{r}\dot{\theta}
2r˙θ˙ 称为科里奥利项Coriolis term。
1.2.3 笛卡尔球坐标系
{
F
:
(
X
^
1
,
X
^
2
,
X
^
3
)
}
=
{
F
:
(
X
^
r
,
X
^
θ
,
X
^
ϕ
)
}
\left\{ F:\left( \hat{X}_1,\hat{X}_2,\hat{X}_3 \right) \right\} =\left\{ F:\left( \hat{X}_{\mathrm{r}},\hat{X}_{\mathrm{\theta}},\hat{X}_{\mathrm{\phi}} \right) \right\}
{F:(X^1,X^2,X^3)}={F:(X^r,X^θ,X^ϕ)}

笛卡尔球坐标系也可以基于投影参数
P
=
(
r
,
θ
,
ϕ
)
P=\left( r,\theta ,\mathrm{\phi} \right)
P=(r,θ,ϕ) 在直角坐标系中进行表达,则点
P
P
P 的运动参数为:
R
⃗
P
S
=
[
I
^
J
^
K
^
]
T
[
r
sin
ϕ
cos
θ
r
sin
ϕ
sin
θ
r
cos
ϕ
]
\vec{R}_{\mathrm{P}}^{S}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} r\sin \phi \cos \theta\\ r\sin \phi \sin \theta\\ r\cos \phi\\ \end{array} \right]
RPS=
I^J^K^
T
rsinϕcosθrsinϕsinθrcosϕ
V
⃗
P
S
=
[
I
^
J
^
K
^
]
T
[
r
˙
sin
ϕ
cos
θ
+
r
ϕ
˙
cos
ϕ
cos
θ
−
r
θ
˙
sin
ϕ
sin
θ
r
˙
sin
ϕ
sin
θ
+
r
ϕ
˙
cos
ϕ
sin
θ
+
r
θ
˙
sin
ϕ
cos
θ
r
˙
cos
ϕ
−
r
ϕ
˙
sin
ϕ
]
\vec{V}_{\mathrm{P}}^{S}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \dot{r}\sin \phi \cos \theta +r\dot{\phi}\cos \phi \cos \theta -r\dot{\theta}\sin \phi \sin \theta\\ \dot{r}\sin \phi \sin \theta +r\dot{\phi}\cos \phi \sin \theta +r\dot{\theta}\sin \phi \cos \theta\\ \dot{r}\cos \phi -r\dot{\phi}\sin \phi\\ \end{array} \right]
VPS=
I^J^K^
T
r˙sinϕcosθ+rϕ˙cosϕcosθ−rθ˙sinϕsinθr˙sinϕsinθ+rϕ˙cosϕsinθ+rθ˙sinϕcosθr˙cosϕ−rϕ˙sinϕ
a
⃗
P
S
=
[
I
^
J
^
K
^
]
T
[
(
r
¨
−
r
ϕ
˙
2
−
r
θ
˙
2
)
sin
ϕ
cos
θ
+
(
2
r
˙
ϕ
˙
+
r
ϕ
¨
)
cos
ϕ
cos
θ
−
(
2
r
˙
θ
˙
+
r
θ
¨
)
sin
ϕ
sin
θ
−
(
2
r
θ
˙
ϕ
˙
)
cos
ϕ
sin
θ
(
r
¨
−
r
ϕ
˙
2
−
r
θ
˙
2
)
sin
ϕ
sin
θ
+
(
2
r
˙
ϕ
˙
+
r
ϕ
¨
)
cos
ϕ
sin
θ
+
(
2
r
˙
θ
˙
+
r
θ
¨
)
sin
ϕ
cos
θ
+
(
2
r
θ
˙
ϕ
˙
)
cos
ϕ
cos
θ
(
r
¨
−
r
ϕ
˙
2
)
cos
ϕ
−
(
2
r
˙
ϕ
˙
+
r
ϕ
¨
)
sin
ϕ
]
\vec{a}_{\mathrm{P}}^{S}=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \left( \ddot{r}-r\dot{\phi}^2-r\dot{\theta}^2 \right) \sin \phi \cos \theta +\left( 2\dot{r}\dot{\phi}+r\ddot{\phi} \right) \cos \phi \cos \theta -\left( 2\dot{r}\dot{\theta}+r\ddot{\theta} \right) \sin \phi \sin \theta -\left( 2r\dot{\theta}\dot{\phi} \right) \cos \phi \sin \theta\\ \left( \ddot{r}-r\dot{\phi}^2-r\dot{\theta}^2 \right) \sin \phi \sin \theta +\left( 2\dot{r}\dot{\phi}+r\ddot{\phi} \right) \cos \phi \sin \theta +\left( 2\dot{r}\dot{\theta}+r\ddot{\theta} \right) \sin \phi \cos \theta +\left( 2r\dot{\theta}\dot{\phi} \right) \cos \phi \cos \theta\\ \left( \ddot{r}-r\dot{\phi}^2 \right) \cos \phi -\left( 2\dot{r}\dot{\phi}+r\ddot{\phi} \right) \sin \phi\\ \end{array} \right]
aPS=
I^J^K^
T
(r¨−rϕ˙2−rθ˙2)sinϕcosθ+(2r˙ϕ˙+rϕ¨)cosϕcosθ−(2r˙θ˙+rθ¨)sinϕsinθ−(2rθ˙ϕ˙)cosϕsinθ(r¨−rϕ˙2−rθ˙2)sinϕsinθ+(2r˙ϕ˙+rϕ¨)cosϕsinθ+(2r˙θ˙+rθ¨)sinϕcosθ+(2rθ˙ϕ˙)cosϕcosθ(r¨−rϕ˙2)cosϕ−(2r˙ϕ˙+rϕ¨)sinϕ
在笛卡尔球坐标中,如图所示,可将点
P
P
P 的位置表述为:
R
⃗
p
s
=
r
x
^
r
\vec{R}_{\mathrm{p}}^{s}=r\hat{x}_{\mathrm{r}}
Rps=rx^r
其中:
[
X
^
ϕ
X
^
θ
X
^
r
]
=
[
cos
ϕ
cos
θ
cos
ϕ
sin
θ
−
sin
ϕ
−
sin
θ
cos
θ
0
sin
ϕ
cos
θ
sin
ϕ
sin
θ
cos
ϕ
]
[
I
^
J
^
K
^
]
\left[ \begin{array}{c} \hat{X}_{\mathrm{\phi}}\\ \hat{X}_{\mathrm{\theta}}\\ \hat{X}_{\mathrm{r}}\\ \end{array} \right] =\left[ \begin{matrix} \cos \phi \cos \theta& \cos \phi \sin \theta& -\sin \phi\\ -\sin \theta& \cos \theta& 0\\ \sin \phi \cos \theta& \sin \phi \sin \theta& \cos \phi\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right]
X^ϕX^θX^r
=
cosϕcosθ−sinθsinϕcosθcosϕsinθcosθsinϕsinθ−sinϕ0cosϕ
I^J^K^
进而求得:
[
X
^
˙
ϕ
X
^
˙
θ
X
^
˙
r
]
=
[
−
ϕ
˙
sin
ϕ
cos
θ
−
θ
˙
cos
ϕ
sin
θ
−
ϕ
˙
sin
ϕ
sin
θ
+
θ
˙
cos
ϕ
cos
θ
−
ϕ
˙
cos
ϕ
−
θ
˙
cos
θ
−
θ
˙
sin
θ
0
ϕ
˙
cos
ϕ
cos
θ
−
θ
˙
sin
ϕ
sin
θ
ϕ
˙
cos
ϕ
sin
θ
+
θ
˙
sin
ϕ
cos
θ
−
ϕ
˙
sin
ϕ
]
[
I
^
J
^
K
^
]
=
[
0
θ
˙
cos
ϕ
−
ϕ
˙
−
θ
˙
cos
ϕ
0
−
θ
˙
sin
ϕ
ϕ
˙
θ
˙
sin
ϕ
0
]
[
X
^
ϕ
X
^
θ
X
^
r
]
\begin{split} \left[ \begin{array}{c} \dot{\hat{X}}_{\mathrm{\phi}}\\ \dot{\hat{X}}_{\mathrm{\theta}}\\ \dot{\hat{X}}_{\mathrm{r}}\\ \end{array} \right] &=\left[ \begin{matrix} -\dot{\phi}\sin \phi \cos \theta -\dot{\theta}\cos \phi \sin \theta& -\dot{\phi}\sin \phi \sin \theta +\dot{\theta}\cos \phi \cos \theta& -\dot{\phi}\cos \phi\\ -\dot{\theta}\cos \theta& -\dot{\theta}\sin \theta& 0\\ \dot{\phi}\cos \phi \cos \theta -\dot{\theta}\sin \phi \sin \theta& \dot{\phi}\cos \phi \sin \theta +\dot{\theta}\sin \phi \cos \theta& -\dot{\phi}\sin \phi\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] \\ &=\left[ \begin{matrix} 0& \dot{\theta}\cos \phi& -\dot{\phi}\\ -\dot{\theta}\cos \phi& 0& -\dot{\theta}\sin \phi\\ \dot{\phi}& \dot{\theta}\sin \phi& 0\\ \end{matrix} \right] \left[ \begin{array}{c} \hat{X}_{\mathrm{\phi}}\\ \hat{X}_{\mathrm{\theta}}\\ \hat{X}_{\mathrm{r}}\\ \end{array} \right] \end{split}
X^˙ϕX^˙θX^˙r
=
−ϕ˙sinϕcosθ−θ˙cosϕsinθ−θ˙cosθϕ˙cosϕcosθ−θ˙sinϕsinθ−ϕ˙sinϕsinθ+θ˙cosϕcosθ−θ˙sinθϕ˙cosϕsinθ+θ˙sinϕcosθ−ϕ˙cosϕ0−ϕ˙sinϕ
I^J^K^
=
0−θ˙cosϕϕ˙θ˙cosϕ0θ˙sinϕ−ϕ˙−θ˙sinϕ0
X^ϕX^θX^r
进而求得其速度参数为:
V
⃗
P
S
=
R
⃗
˙
P
S
=
r
˙
X
^
r
+
r
X
^
˙
r
=
r
˙
X
^
r
+
r
ϕ
˙
X
^
ϕ
+
r
θ
˙
sin
ϕ
X
^
θ
\vec{V}_{\mathrm{P}}^{S}=\dot{\vec{R}}_{\mathrm{P}}^{S}=\dot{r}\hat{X}_{\mathrm{r}}+r\dot{\hat{X}}_{\mathrm{r}}=\dot{r}\hat{X}_{\mathrm{r}}+r\dot{\phi}\hat{X}_{\mathrm{\phi}}+r\dot{\theta}\sin \phi \hat{X}_{\mathrm{\theta}}
VPS=R˙PS=r˙X^r+rX^˙r=r˙X^r+rϕ˙X^ϕ+rθ˙sinϕX^θ
角速度参数为:
ω
⃗
=
θ
˙
sin
ϕ
X
^
ϕ
−
θ
˙
cos
ϕ
X
^
r
+
ϕ
˙
X
^
θ
\vec{\omega}=\dot{\theta}\sin \phi \hat{X}_{\mathrm{\phi}}-\dot{\theta}\cos \phi \hat{X}_{\mathrm{r}}+\dot{\phi}\hat{X}_{\mathrm{\theta}}
ω=θ˙sinϕX^ϕ−θ˙cosϕX^r+ϕ˙X^θ
加速度参数为:
a
⃗
P
S
=
V
⃗
˙
P
S
=
{
r
¨
X
^
r
+
r
˙
X
^
˙
r
+
r
˙
ϕ
˙
X
^
ϕ
+
r
ϕ
¨
X
^
ϕ
+
r
ϕ
X
^
˙
ϕ
+
r
˙
θ
˙
sin
ϕ
X
^
θ
+
r
θ
¨
sin
ϕ
X
^
θ
+
r
θ
˙
ϕ
˙
cos
ϕ
X
^
θ
+
r
θ
˙
sin
ϕ
X
^
˙
θ
=
{
r
¨
X
^
r
+
r
˙
(
ϕ
˙
X
^
ϕ
+
θ
˙
sin
ϕ
X
^
θ
)
+
(
r
˙
ϕ
˙
+
r
ϕ
¨
)
X
^
ϕ
+
r
ϕ
(
θ
˙
cos
ϕ
X
^
θ
−
ϕ
˙
X
^
r
)
+
(
r
˙
θ
˙
sin
ϕ
+
r
θ
¨
sin
ϕ
+
r
θ
˙
ϕ
˙
cos
ϕ
)
X
^
θ
+
r
θ
˙
sin
ϕ
(
−
θ
˙
cos
ϕ
X
^
ϕ
−
θ
˙
sin
ϕ
X
^
r
)
=
(
r
¨
−
r
ϕ
ϕ
˙
−
r
θ
˙
2
sin
ϕ
2
)
X
^
r
+
(
2
r
˙
ϕ
˙
+
r
ϕ
¨
−
r
θ
˙
2
sin
ϕ
cos
ϕ
)
X
^
ϕ
+
[
(
2
r
˙
θ
˙
+
r
θ
¨
)
sin
ϕ
+
(
r
θ
˙
ϕ
˙
+
r
ϕ
θ
˙
)
cos
ϕ
]
X
^
θ
\begin{split} \vec{a}_{\mathrm{P}}^{S}&=\dot{\vec{V}}_{\mathrm{P}}^{S}=\begin{cases} \ddot{r}\hat{X}_{\mathrm{r}}+\dot{r}\dot{\hat{X}}_{\mathrm{r}}+\dot{r}\dot{\phi}\hat{X}_{\mathrm{\phi}}+r\ddot{\phi}\hat{X}_{\mathrm{\phi}}+r\phi \dot{\hat{X}}_{\mathrm{\phi}}\\ +\dot{r}\dot{\theta}\sin \phi \hat{X}_{\mathrm{\theta}}+r\ddot{\theta}\sin \phi \hat{X}_{\mathrm{\theta}}+r\dot{\theta}\dot{\phi}\cos \phi \hat{X}_{\mathrm{\theta}}+r\dot{\theta}\sin \phi \dot{\hat{X}}_{\mathrm{\theta}}\\ \end{cases} \\ &=\begin{cases} \ddot{r}\hat{X}_{\mathrm{r}}+\dot{r}\left( \dot{\phi}\hat{X}_{\mathrm{\phi}}+\dot{\theta}\sin \phi \hat{X}_{\mathrm{\theta}} \right) +\left( \dot{r}\dot{\phi}+r\ddot{\phi} \right) \hat{X}_{\mathrm{\phi}}+r\phi \left( \dot{\theta}\cos \phi \hat{X}_{\mathrm{\theta}}-\dot{\phi}\hat{X}_{\mathrm{r}} \right)\\ +\left( \dot{r}\dot{\theta}\sin \phi +r\ddot{\theta}\sin \phi +r\dot{\theta}\dot{\phi}\cos \phi \right) \hat{X}_{\mathrm{\theta}}+r\dot{\theta}\sin \phi \left( -\dot{\theta}\cos \phi \hat{X}_{\mathrm{\phi}}-\dot{\theta}\sin \phi \hat{X}_{\mathrm{r}} \right)\\ \end{cases} \\ &=\left( \ddot{r}-r\phi \dot{\phi}-r\dot{\theta}^2\sin \phi ^2 \right) \hat{X}_{\mathrm{r}}+\left( 2\dot{r}\dot{\phi}+r\ddot{\phi}-r\dot{\theta}^2\sin \phi \cos \phi \right) \hat{X}_{\mathrm{\phi}}+\left[ \left( 2\dot{r}\dot{\theta}+r\ddot{\theta} \right) \sin \phi +\left( r\dot{\theta}\dot{\phi}+r\phi \dot{\theta} \right) \cos \phi \right] \hat{X}_{\mathrm{\theta}} \end{split}
aPS=V˙PS={r¨X^r+r˙X^˙r+r˙ϕ˙X^ϕ+rϕ¨X^ϕ+rϕX^˙ϕ+r˙θ˙sinϕX^θ+rθ¨sinϕX^θ+rθ˙ϕ˙cosϕX^θ+rθ˙sinϕX^˙θ=⎩
⎨
⎧r¨X^r+r˙(ϕ˙X^ϕ+θ˙sinϕX^θ)+(r˙ϕ˙+rϕ¨)X^ϕ+rϕ(θ˙cosϕX^θ−ϕ˙X^r)+(r˙θ˙sinϕ+rθ¨sinϕ+rθ˙ϕ˙cosϕ)X^θ+rθ˙sinϕ(−θ˙cosϕX^ϕ−θ˙sinϕX^r)=(r¨−rϕϕ˙−rθ˙2sinϕ2)X^r+(2r˙ϕ˙+rϕ¨−rθ˙2sinϕcosϕ)X^ϕ+[(2r˙θ˙+rθ¨)sinϕ+(rθ˙ϕ˙+rϕθ˙)cosϕ]X^θ
1.2.4 曲线坐标系:Frenet标架(详见微分几何-曲线论内容)

其中,
s
s
s 为曲线的弧长参数,
ρ
\rho
ρ 为曲线的曲率半径;则有:
α
⃗
˙
=
s
˙
ρ
β
⃗
\dot{\vec{\alpha}}=\frac{\dot{s}}{\rho}\vec{\beta}
α˙=ρs˙β,其中,
s
¨
\ddot{s}
s¨ 为切向加速度,
s
˙
2
ρ
\frac{\dot{s}^2}{\rho}
ρs˙2 为向心加速度,整理出:
{
V
⃗
P
F
=
R
⃗
˙
P
F
=
s
˙
α
⃗
a
⃗
P
F
=
V
⃗
˙
P
F
=
s
¨
α
⃗
+
s
˙
α
⃗
˙
=
s
¨
α
⃗
+
s
˙
2
ρ
β
⃗
\left\{ \begin{array}{c} \vec{V}_{\mathrm{P}}^{F}=\dot{\vec{R}}_{\mathrm{P}}^{F}=\dot{s}\vec{\alpha}\\ \vec{a}_{\mathrm{P}}^{F}=\dot{\vec{V}}_{\mathrm{P}}^{F}=\ddot{s}\vec{\alpha}+\dot{s}\dot{\vec{\alpha}}=\ddot{s}\vec{\alpha}+\frac{\dot{s}^2}{\rho}\vec{\beta}\\ \end{array} \right.
⎩
⎨
⎧VPF=R˙PF=s˙αaPF=V˙PF=s¨α+s˙α˙=s¨α+ρs˙2β
且有角速度 ω ⃗ = ω α α ⃗ + ω β β ⃗ + ω γ γ ⃗ \vec{\omega}=\omega _{\mathrm{\alpha}}\vec{\alpha}+\omega _{\beta}\vec{\beta}+\omega _{\mathrm{\gamma}}\vec{\mathrm{\gamma}} ω=ωαα+ωββ+ωγγ,求解下式: α ⃗ ˙ = ω γ β ⃗ = s ˙ ρ β ⃗ , β ⃗ ˙ = − ω α β ⃗ = s ˙ d γ ⃗ d s , ω β = 0 \dot{\vec{\alpha}}=\omega _{\mathrm{\gamma}}\vec{\beta}=\frac{\dot{s}}{\rho}\vec{\beta},\dot{\vec{\beta}}=-\omega _{\mathrm{\alpha}}\vec{\beta}=\dot{s}\frac{\mathrm{d}\vec{\mathrm{\gamma}}}{\mathrm{d}s},\omega _{\beta}=0 α˙=ωγβ=ρs˙β,β˙=−ωαβ=s˙dsdγ,ωβ=0
补充说明:
对于笛卡尔坐标系内的点 P P P 而言,其速度参数与加速度参数既可以在固定直角坐标系的标架 { F : ( I ^ , J ^ , K ^ ) } \left\{ F:\left( \hat{I},\hat{J},\hat{K} \right) \right\} {F:(I^,J^,K^)}下进行表示,也可以在运动坐标系的标架下 { C : ( X ^ r , X ^ θ , K ^ ) } \left\{ C:\left( \hat{X}_{\mathrm{r}},\hat{X}_{\mathrm{\theta}},\hat{K} \right) \right\} {C:(X^r,X^θ,K^)}(柱坐标系)、 { S : ( X ^ ϕ , X ^ θ , X ^ r ) } \left\{ S:\left( \hat{X}_{\mathrm{\phi}},\hat{X}_{\mathrm{\theta}},\hat{X}_{\mathrm{r}} \right) \right\} {S:(X^ϕ,X^θ,X^r)}(球坐标系)进行表示,甚至在轨迹的Frenet标架下表示。根据所给的运动参数,可以求得不同标架所对应不同运动的投影参数。
1.2.5 广义坐标系 Generalized coordinates system
对于不固定的单位矢量而言(如上述的柱坐标系中的
X
^
r
\hat{X}_{\mathrm{r}}
X^r与
X
^
θ
\hat{X}_{\theta}
X^θ,球坐标系中的
(
X
^
ϕ
,
X
^
θ
,
X
^
r
)
\left( \hat{X}_{\mathrm{\phi}},\hat{X}_{\mathrm{\theta}},\hat{X}_{\mathrm{r}} \right)
(X^ϕ,X^θ,X^r)),在表达运动时可能更为方便。认为广义坐标generalized coordinates是用来描述系统形位的相互独立广义坐标矢量的投影参数坐标,即在该广义坐标系下描述任意矢量,则有:
r
⃗
˙
p
e
=
(
q
˙
1
e
⃗
1
+
q
˙
2
e
⃗
2
+
⋯
+
q
˙
n
e
⃗
n
)
+
(
Q
1
e
⃗
˙
1
+
q
2
e
⃗
˙
2
+
⋯
+
q
n
e
⃗
˙
n
)
=
(
q
˙
1
e
⃗
1
+
q
˙
2
e
⃗
2
+
⋯
+
q
˙
n
e
⃗
n
)
+
ω
⃗
e
×
r
⃗
p
e
\dot{\vec{r}}_{\mathrm{p}}^{e}=\left( \dot{q}_1\vec{e}_1+\dot{q}_2\vec{e}_2+\cdots +\dot{q}_{\mathrm{n}}\vec{e}_{\mathrm{n}} \right) +\left( Q_1\dot{\vec{e}}_1+q_2\dot{\vec{e}}_2+\cdots +q_{\mathrm{n}}\dot{\vec{e}}_{\mathrm{n}} \right) =\left( \dot{q}_1\vec{e}_1+\dot{q}_2\vec{e}_2+\cdots +\dot{q}_{\mathrm{n}}\vec{e}_{\mathrm{n}} \right) +\vec{\omega}^e\times \vec{r}_{\mathrm{p}}^{e}
r˙pe=(q˙1e1+q˙2e2+⋯+q˙nen)+(Q1e˙1+q2e˙2+⋯+qne˙n)=(q˙1e1+q˙2e2+⋯+q˙nen)+ωe×rpe
对于三维空间而言,则有:
R
⃗
˙
P
E
=
(
q
˙
1
+
ω
2
q
3
−
ω
3
q
2
)
e
⃗
1
+
(
q
˙
2
+
ω
3
q
1
−
ω
1
q
3
)
e
⃗
2
+
(
q
˙
3
+
ω
1
q
2
−
ω
2
q
1
)
e
⃗
3
\dot{\vec{R}}_{\mathrm{P}}^{E}=\left( \dot{q}_1+\omega _2q_3-\omega _3q_2 \right) \vec{e}_1+\left( \dot{q}_2+\omega _3q_1-\omega _1q_3 \right) \vec{e}_2+\left( \dot{q}_3+\omega _1q_2-\omega _2q_1 \right) \vec{e}_3
R˙PE=(q˙1+ω2q3−ω3q2)e1+(q˙2+ω3q1−ω1q3)e2+(q˙3+ω1q2−ω2q1)e3
1.3 矢量Vector在坐标系下的表示与关系转换
对于质量点而言
P
P
P ,其可将其在固定坐标系
{
F
}
\left\{ F \right\}
{F}(默认为直角坐标系)下进行表示。同理,对于运动坐标系
{
M
}
\left\{ M \right\}
{M}而言,点
P
P
P 为运动刚体上一点,在运动坐标系下的表达为:
R
⃗
P
M
\vec{R}_{\mathrm{P}}^{M}
RPM,则其矢量在固定坐标系下的表达为:
(
R
⃗
P
M
)
F
\left( \vec{R}_{\mathrm{P}}^{M} \right) ^F
(RPM)F,或写成矢量表达形式为:
R
⃗
O
M
P
M
→
R
⃗
O
M
P
F
\vec{R}_{\mathrm{O}^{\mathrm{M}}\mathrm{P}}^{M}\rightarrow \vec{R}_{\mathrm{O}^{\mathrm{M}}\mathrm{P}}^{F}
ROMPM→ROMPF
R
⃗
P
M
=
P
1
M
i
^
M
+
P
2
M
j
^
M
+
P
3
M
k
^
M
=
[
i
^
M
j
^
M
k
^
M
]
T
[
P
1
M
P
2
M
P
3
M
]
=
(
[
Q
M
F
]
T
[
I
^
J
^
K
^
]
)
T
[
P
1
M
P
2
M
P
3
M
]
=
[
I
^
J
^
K
^
]
T
[
Q
M
F
]
[
P
1
M
P
2
M
P
3
M
]
=
(
R
⃗
P
M
)
F
\begin{split} \vec{R}_{\mathrm{P}}^{M}&={P_1}^M\hat{i}^M+{P_2}^M\hat{j}^M+{P_3}^M\hat{k}^M=\left[ \begin{array}{c} \hat{i}^M\\ \hat{j}^M\\ \hat{k}^M\\ \end{array} \right] ^{\mathrm{T}}\left[ \begin{array}{c} {P_1}^M\\ {P_2}^M\\ {P_3}^M\\ \end{array} \right] =\left( \left[ Q_{\mathrm{M}}^{F} \right] ^{\mathrm{T}}\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] \right) ^{\mathrm{T}}\left[ \begin{array}{c} {P_1}^M\\ {P_2}^M\\ {P_3}^M\\ \end{array} \right] \\ &=\left[ \begin{array}{c} \hat{I}\\ \hat{J}\\ \hat{K}\\ \end{array} \right] ^{\mathrm{T}}\left[ Q_{\mathrm{M}}^{F} \right] \left[ \begin{array}{c} {P_1}^M\\ {P_2}^M\\ {P_3}^M\\ \end{array} \right] =\left( \vec{R}_{\mathrm{P}}^{M} \right) ^F \end{split}
RPM=P1Mi^M+P2Mj^M+P3Mk^M=
i^Mj^Mk^M
T
P1MP2MP3M
=
[QMF]T
I^J^K^
T
P1MP2MP3M
=
I^J^K^
T[QMF]
P1MP2MP3M
=(RPM)F