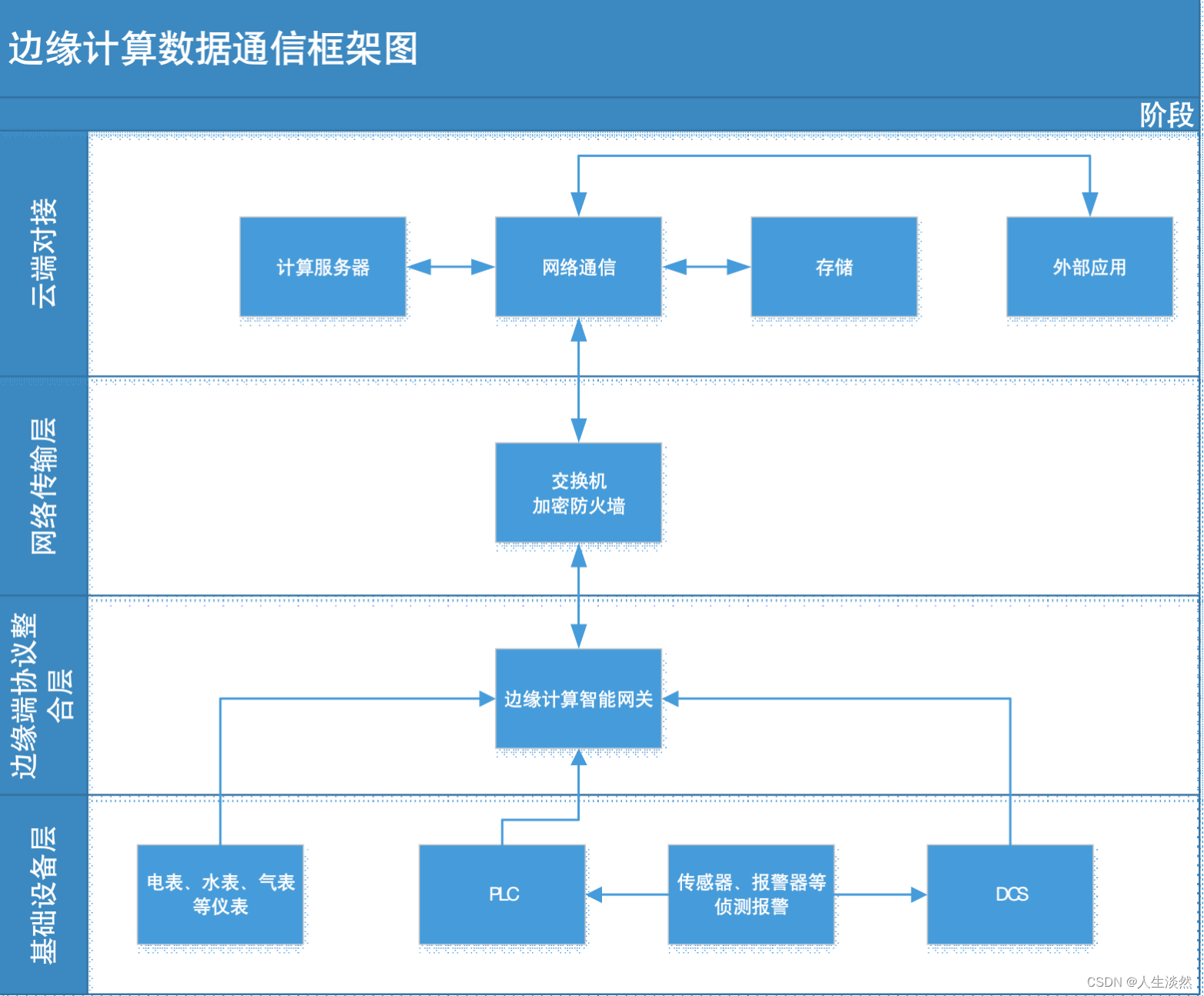
文章目录
- 数学原理
- 代码实现
- 测试
数学原理
平面方程可写为
A x + B y + C z + D = 0 Ax+By+Cz+D=0 Ax+By+Cz+D=0
假设 C C C不为0,则上式可以改写为
z = a x + b y + d z=ax+by+d z=ax+by+d
则现有一组点 { p i } \{p_i\} {pi},则根据 x i , y i x_i,y_i xi,yi以及平面方程,可以得到其对应的 z ^ i \hat z_i z^i
z ^ i = a x i + b y i + d \hat z_i=ax_i+by_i+d z^i=axi+byi+d
从而平面拟合就转换为了最小二乘问题
arg min ∑ ∣ z i − z ^ i ∣ \argmin \sum \vert z_i-\hat z_i\vert argmin∑∣zi−z^i∣
将其转换为矩阵形式,记
A = [ x 1 y 1 1 x 2 y 2 1 ⋮ ⋮ x n y n 1 ] , x = [ a b d ] , b = [ z 1 z 2 ⋮ z n ] A=\begin{bmatrix} x_1&y_1&1\\x_2&y_2&1\\\vdots&\vdots\\x_n&y_n&1 \end{bmatrix}, x=\begin{bmatrix}a\\b\\d\end{bmatrix}, b=\begin{bmatrix}z_1\\z_2\\\vdots\\z_n\end{bmatrix} A= x1x2⋮xny1y2⋮yn111 ,x= abd ,b= z1z2⋮zn
则拟合方程变为
A x = b Ax=b Ax=b
相应地 x x x可写为
x = ( A T A ) − 1 A T b x=(A^TA)^{-1}A^Tb x=(ATA)−1ATb
最小二乘法的原理可见:python实现最小二乘法
代码实现
其代码实现如下
import numpy as np
def planefit(points):
xs, ys, zs = list(zip(*points))
A = np.vstack([xs,ys,np.ones_like(xs)]).T
b = np.reshape(zs, [-1, 1])
abd = np.linalg.inv(A.T @ A) @ A.T @ b
return abd.reshape(-1)
其中输入参数points为一组点。第一步将这些点进行坐标拆分,得到一一对应的xs, ys, zs。然后通过这些点,构造矩阵A和向量b,最后输出 ( A T A ) − 1 A T b (A^TA)^{-1}A^Tb (ATA)−1ATb。
测试
首先做一个初始化平面的函数,其功能是随机生成一组在平面中的点,并且为其添加一些噪声。
# 初始化平面
def initPlane(a, b, d, N, err=0.1):
xs,ys = np.random.rand(2, N)
zs = a*xs + b*ys + d + np.random.rand(N)*err
return list(zip(xs, ys, zs))
然后用planefit函数对这些点进行拟合,通过对比二者之间的差异,来证实算法的有效性
import matplotlib.pyplot as plt
pts = initPlane(2,3,4,100,1)
a,b,d = planefit(pts)
xs, ys = np.indices([100,100])/100
zs = a*xs + b*ys + d
ax = plt.subplot(projection='3d')
ax.plot_surface(xs, ys, zs, cmap='jet')
ax.scatter(*np.array(pts).T, marker='*')
plt.show()

随着加入的噪声逐渐变大,拟合误差也越来越大
errs = [0.01, 0.1, 0.2, 0.5, 1, 3, 5]
fits = []
for err in errs:
pts = initPlane(2,3,4,100,1)
a,b,d = planefit(pts)
fits.append([abs(a-2),abs(b-3),abs(d-4)])
import pprint
pprint.pprint(fits)
[[0.09377971025135245, 0.023025216275622373, 0.4933931906466551],
[0.044310965250572654, 0.05681830483294226, 0.47952260969370997],
[0.051813469166934745, 0.017914573861143257, 0.47553046120193176],
[0.08578595894551588, 0.0464898508775029, 0.42791269232718054],
[0.011569662177250528, 0.15976404558136714, 0.4886516489062753],
[0.006829071411009302, 0.04832062421804073, 0.5193494695593301],
[0.1651263679674586, 0.0736367910618192, 0.44103226768552073]]



![[AutoSar]导出task mapping 表到excel](https://img-blog.csdnimg.cn/af7b6b3dbe9a493e913fce3af7d83a7e.png)


![[oeasy]python001_先跑起来_python_三大系统选择_windows_mac_linux](https://img-blog.csdnimg.cn/img_convert/f8a356f9bd35227eda97ec6b1151e81e.png)