文章目录
- 一【题目类别】
- 二【题目难度】
- 三【题目编号】
- 四【题目描述】
- 五【题目示例】
- 六【解题思路】
- 七【题目提示】
- 八【时间频度】
- 九【代码实现】
- 十【提交结果】
一【题目类别】
- 动态规划
二【题目难度】
- 中等
三【题目编号】
- 152.乘积最大子数组
四【题目描述】
- 给你一个整数数组 nums ,请你找出数组中乘积最大的非空连续子数组(该子数组中至少包含一个数字),并返回该子数组所对应的乘积。
- 测试用例的答案是一个 32-位 整数。
- 子数组 是数组的连续子序列。
五【题目示例】
-
示例 1:
- 输入: nums = [2,3,-2,4]
- 输出: 6
- 解释: 子数组 [2,3] 有最大乘积 6。
-
示例 2:
- 输入: nums = [-2,0,-1]
- 输出: 0
- 解释: 结果不能为 2, 因为 [-2,-1] 不是子数组。
六【解题思路】
- 本题的思路与【LeetCode每日一题】——剑指 Offer 42.连续子数组的最大和比较相似,只是本题做的是乘法,也就需要考虑到负负得正的情况
- 所以,我们分别维护前一次状态最大值和最小值,当遇到负数时,说明有可能发生负负得正,那么就交换最大值和最小值,这里不用担心最大值变小的问题,因为无非就两种情况
- 最小值是一个正数,这个时候最大值变为最小值,乘一个负数,不会影响之前的最大值
- 最小值是一个负数,这个时候最大值变为负数,很明显负负得正,相乘变大,正好可以更新
- 所以不管怎么样,都不会影响我们找到最大值
- 维护前一次状态的最大值和最小值的动态转移方程分别为:
- t e m p M a x = M a t h . m a x ( t e m p M a x ∗ n u m s [ i ] , n u m s [ i ] ) tempMax = Math.max(tempMax * nums[i],nums[i]) tempMax=Math.max(tempMax∗nums[i],nums[i])
- t e m p M i n = M a t h . m i n ( t e m p M i n ∗ n u m s [ i ] , n u m s [ i ] ) tempMin = Math.min(tempMin * nums[i],nums[i]) tempMin=Math.min(tempMin∗nums[i],nums[i])
- 每次都要记得更新最大值
- 最后返回结果即可
七【题目提示】
- 1 < = n u m s . l e n g t h < = 2 ∗ 1 0 4 1 <= nums.length <= 2 * 10^4 1<=nums.length<=2∗104
- − 10 < = n u m s [ i ] < = 10 -10 <= nums[i] <= 10 −10<=nums[i]<=10
- n u m s 的任何前缀或后缀的乘积都保证是一个 32 − 位整数 nums 的任何前缀或后缀的乘积都 保证 是一个 32-位 整数 nums的任何前缀或后缀的乘积都保证是一个32−位整数
八【时间频度】
- 时间复杂度: O ( n ) O(n) O(n),其中 n n n为数组大小
- 空间复杂度: O ( 1 ) O(1) O(1)
九【代码实现】
- Java语言版
class Solution {
public int maxProduct(int[] nums) {
int res = Integer.MIN_VALUE;
int tempMax = 1;
int tempMin = 1;
for(int i = 0;i<nums.length;i++){
if(nums[i] < 0){
int temp = tempMax;
tempMax = tempMin;
tempMin = temp;
}
tempMax = Math.max(tempMax * nums[i],nums[i]);
tempMin = Math.min(tempMin * nums[i],nums[i]);
res = Math.max(res,tempMax);
}
return res;
}
}
- C语言版
int maxProduct(int* nums, int numsSize)
{
int res = INT_MIN;
int tempMax = 1;
int tempMin = 1;
for(int i = 0;i<numsSize;i++)
{
if(nums[i] < 0)
{
int temp = tempMax;
tempMax = tempMin;
tempMin = temp;
}
tempMax = fmax(tempMax * nums[i],nums[i]);
tempMin = fmin(tempMin * nums[i],nums[i]);
res = fmax(res,tempMax);
}
return res;
}
- Python版
class Solution:
def maxProduct(self, nums: List[int]) -> int:
res = nums[0]
tempMax = 1
tempMin = 1
for i in range(0,len(nums)):
if nums[i] < 0:
temp = tempMax
tempMax = tempMin
tempMin = temp
tempMax = max(tempMax * nums[i],nums[i])
tempMin = min(tempMin * nums[i],nums[i])
res = max(res,tempMax)
return res
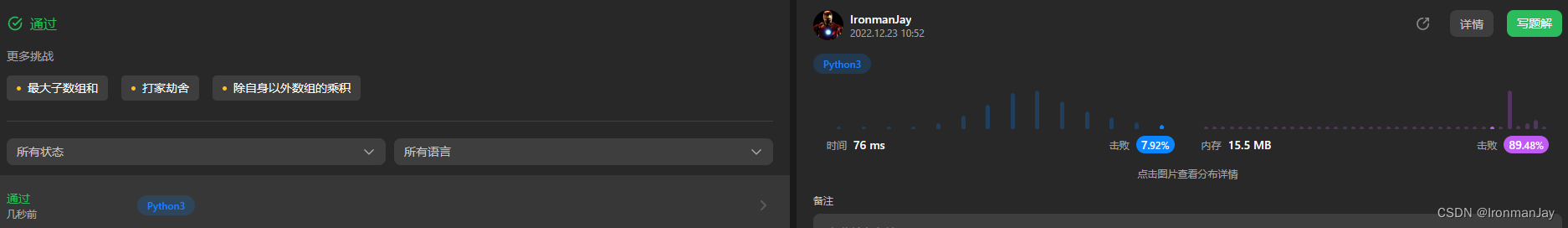
十【提交结果】
-
Java语言版
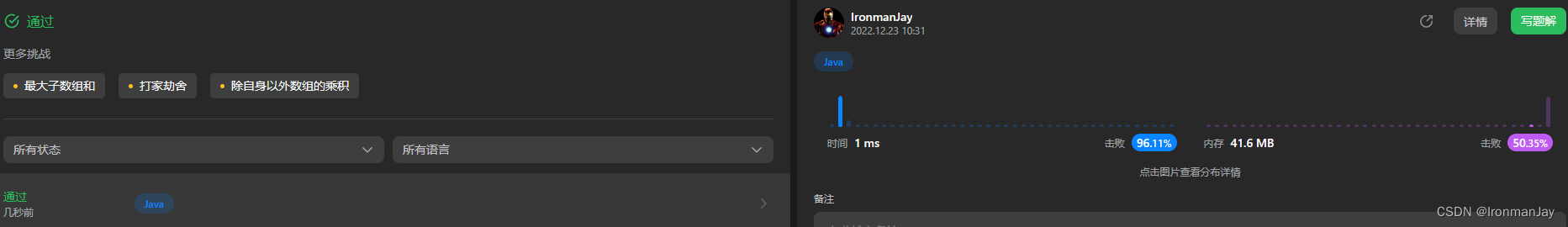
-
C语言版
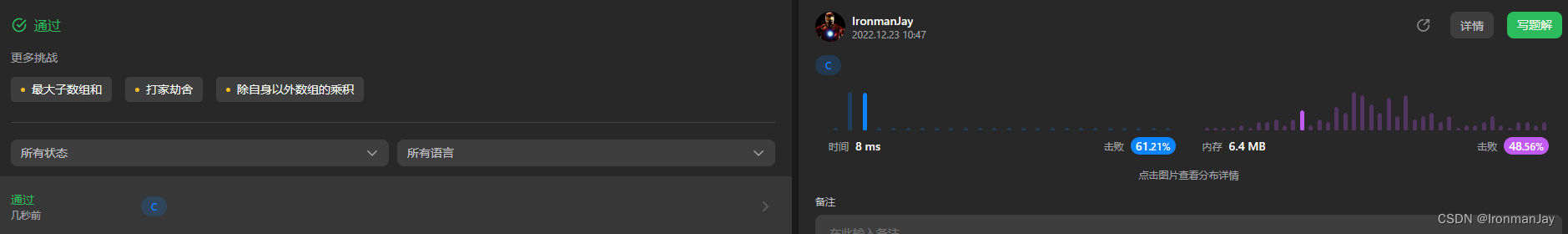
-
Python语言版