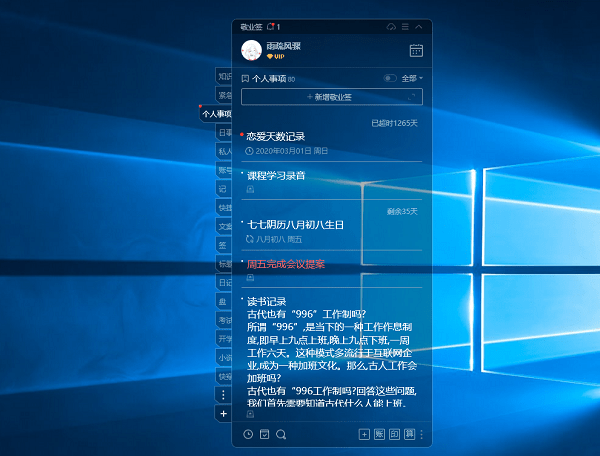
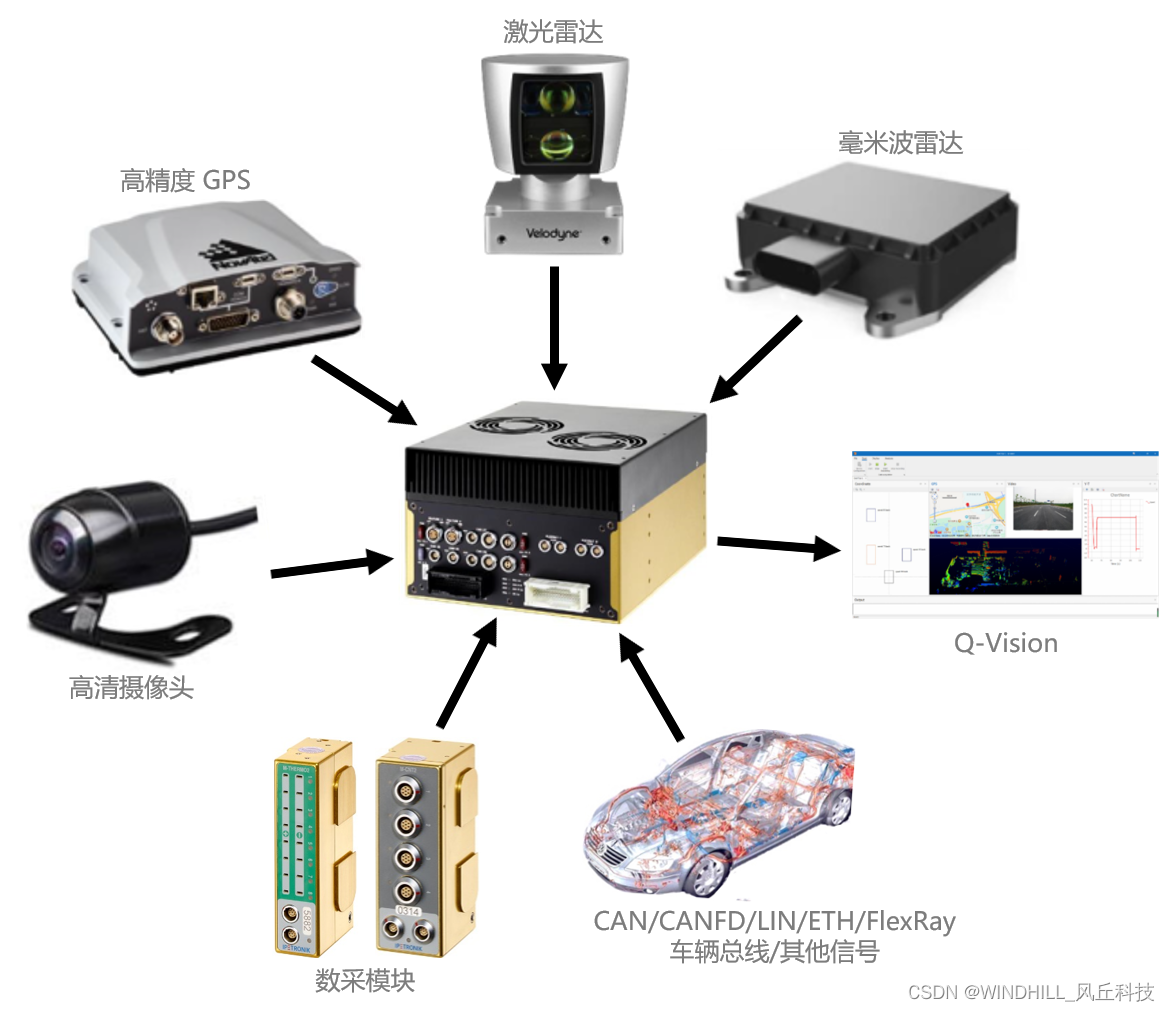
极坐标系下的交换积分次序

我把极坐标系下的交换积分次序总结为动静与静动之间的转换，下面通过一个例子感受一下
$$\rho =1、\rho =1+\cos \theta$$

$${\int }_0^{\pi /2}d\theta {\int }_1^{1+\cos \theta }f(\rho \cos \theta ,\rho \sin \theta )\rho d\rho$$
上例中先积 $\rho$ 后积$\theta$，
我们发现 $\rho$ 的上下限是 $1$ 到 $1+\cos \theta$，显然 $\theta$ 改变的话，上限也会发生变化，即变化的范围，我把它称为动
我们发现 $\theta$ 的上下限是 $0$ 到 $\pi /2$，显然是一个固定的范围，我把它称为静
我们交换积分次序，即动态范围的$\rho$、静态范围的$\theta$ 改变为 静态范围的$\rho$、动态范围的$\theta$
注意：我所说的动静是针对范围或者说界限的（积分上下限）

动态范围的$\rho$、静态范围的$\theta$
$${\int }_0^{\pi /2}d\theta {\int }_1^{1+\cos \theta }f(\rho \cos \theta ,\rho \sin \theta )\rho d\rho$$


静态范围的$\rho$、动态范围的$\theta$
$${\int }_1^2\rho d\rho {\int }_0^{\arccos (\rho -1)}f(\rho \cos \theta ,\rho \sin \theta )d\theta$$