文章目录
- 二叉搜索树
- 二叉搜索树的模拟实现
- 构造函数
- 拷贝构造函数
- 赋值运算符重载函数
- 析构函数
- Insert
- 循环
- 递归
- Erase
- 循环
- 递归
- Find
- 循环
- 递归
- 二叉搜索树的应用
- K模型
- KV模型
- 完整代码
- 普通版本
- 递归版本
二叉搜索树
二叉搜索树又称为二叉排序树,它或者是一棵空树,或者是具有以下性质的二叉树:
若它的左子树不为空,
则左子树上所有结点的值都小于根结点的值。
若它的右子树不为空,
则右子树上所有结点的值都大于根结点的值。
它的左右子树也分别是二叉搜索树。

二叉搜索树的模拟实现
构造函数
BSTree()
:_root(nullptr)
{
}
拷贝构造函数
BSTree(const BSTree<K>& t)
//BSTree( BSTree<K> *this , const BSTree<K> & t)
//t1 =t
{
_root = Copy(t._root);
}
private:
Node* Copy(Node* root)
{
if (root == nullptr)
{
return nullptr;
}
Node* copyNode = new Node(root->_key);
//递归
copyNode->_left = Copy(root->_left);
copyNode->_right = Copy(root->_right);
return copyNode;
}
赋值运算符重载函数
//赋值重载
BSTree<K>& operator= (BSTree<K>& t)
//t1 = t
//深拷贝
{
swap(_root, t._root);
return *this;
}
析构函数
~BSTree()
{
Destroy(_root);
}
private:
void Destroy(Node*& root) //引用的目的:将每个节点释放后同时置空
{
//后序遍历
if (root == nullptr)
{
return;
}
Destroy(root->_left);
Destroy(root->_right);
delete root;
root = nullptr;
}
Insert
核心思路:
如果是空树,则直接将插入结点作为二叉搜索树的根结点。
如果不是空树,则按照二叉搜索树的性质进行结点的插入。
如果待插入结点的值<根结点的值,则需要将结点插入到左子树当中。
如果待插入结点的值>根结点的值,则需要将结点插入到右子树当中。
如果待插入结点的值等于根结点的值,则插入失败。
循环
bool Insert(const K & key )
{
//空树
if (_root == nullptr)
{
_root = new Node(key);
return true;
}
//不是空树
Node* parent = nullptr;//找到父节点
Node* cur = _root;
while (cur)
{
//比较
if (cur->_key < key)
{
//往右子树走
parent = cur;
cur = cur->_right;
}
else if (cur->_key > key)
{
//往左子树走
parent = cur;
cur = cur->_left;
}
else
{
return false;
}
}
//插入节点
cur = new Node(key);
//不知道parent在那一边,需要进一步判断
if (parent->_key > key)
{
//parent在左边
parent->_left = cur;
}
else if (parent->_key < key)
{
//parent在右边
parent->_right = cur;
}
else
{
return false;
}
return true;
}
递归
bool InsertR(const K& key)//递归版本
{
return _InsertR(_root, key);
}
private:
bool _InsertR(Node*& root, const K& key) //引用的目的:不用找父节点,不需要用父节点比较大小
{
//结束条件
if (root == nullptr)
{
root = new Node(key);
return true;
}
//往左子树走
if (root->_key > key)
{
return _InsertR(root->_left, key);
}
//往右子树走
else if (root->_key < key)
{
return _InsertR(root->_right, key);
}
else
{
return false;
}
}
Erase
先找到需要删除的节点
需要删除的节点可能会有三种情况:
1、待删除结点的左子树为空(待删除结点的左右子树均为空包含在内)
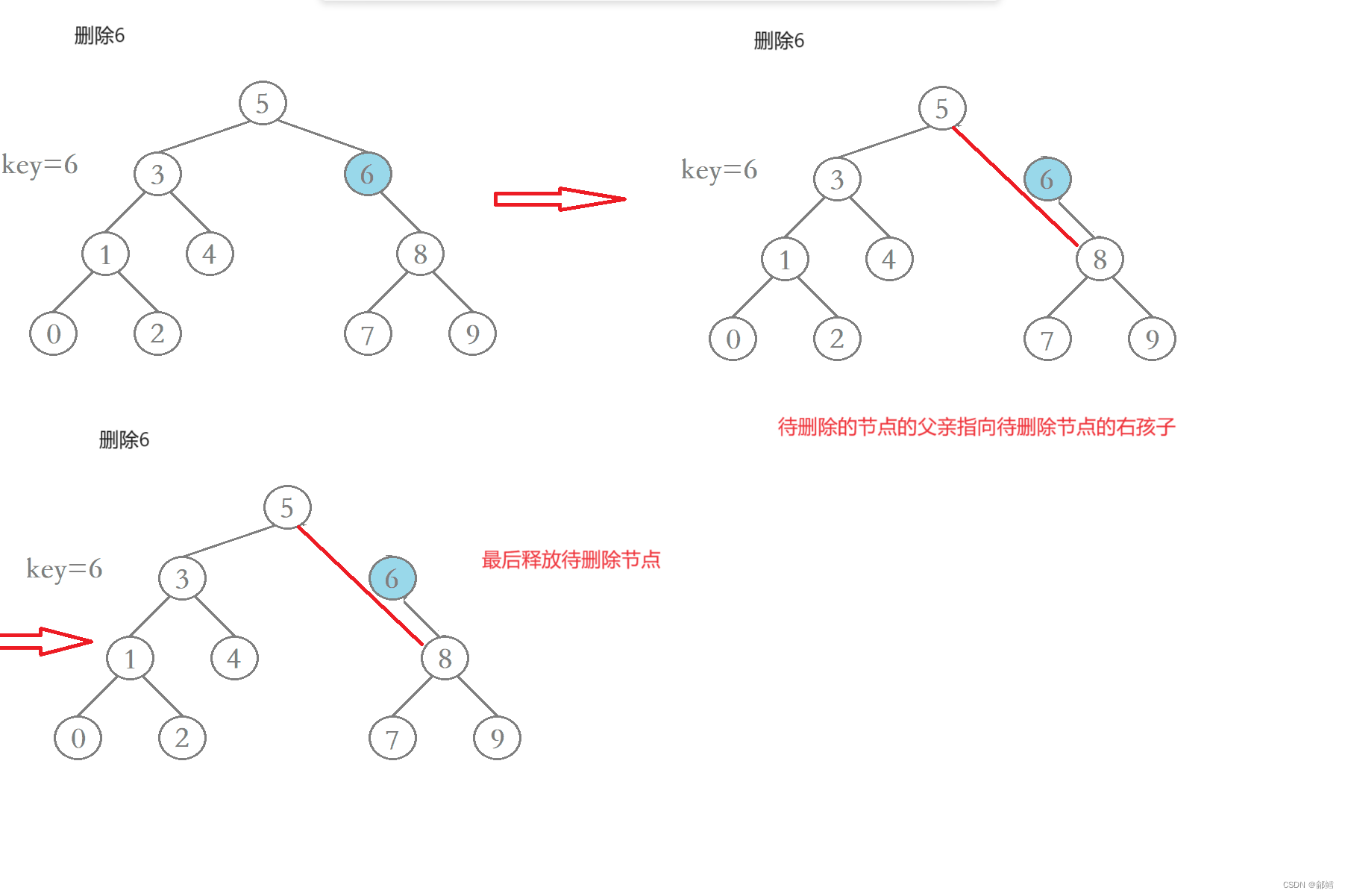
2、待删除结点的右子树为空。
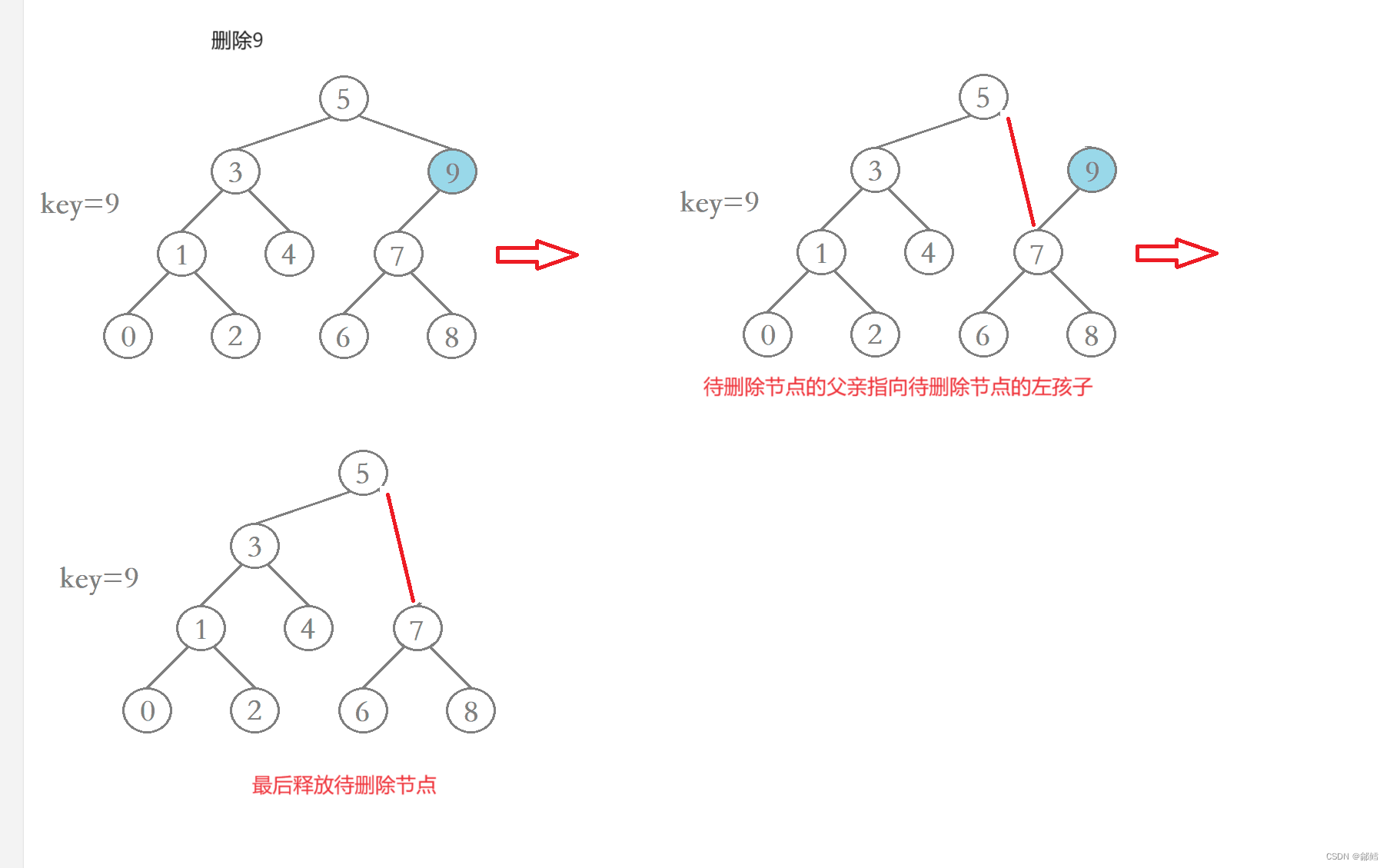
1、2 两种情况,被删除的节点都只有一个孩子
3、待删除结点的左右子树均不为空,即被删除节点有两个孩子
使用替换法处理第3中情况:
1、找替换节点:替换节点一般是左子树的最大节点(最右节点),或者是右子树的最小节点(最左节点)
2、将替换的节点删除
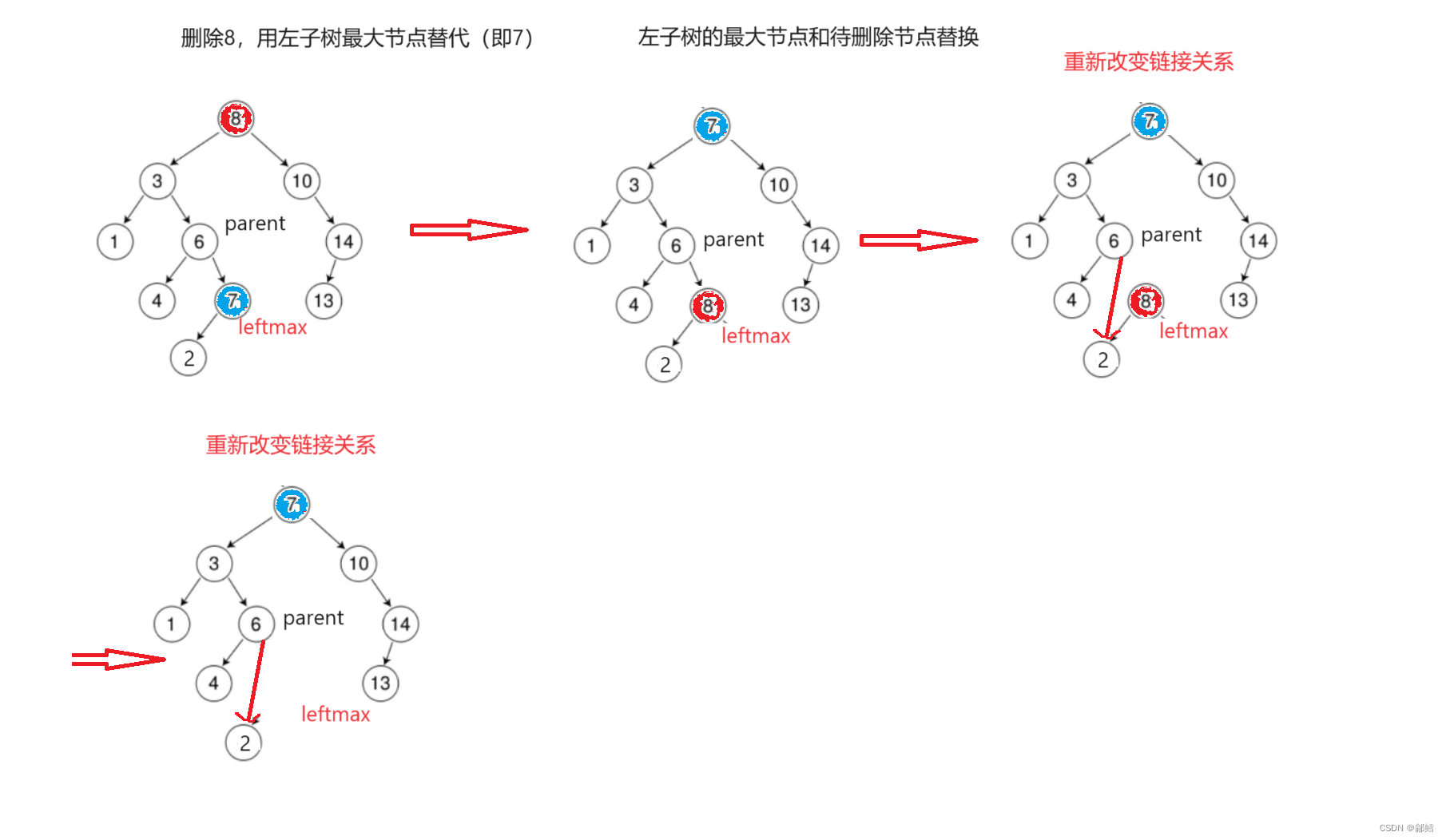
特殊情况:

循环
bool Erase(const K& key)
{
Node* parent = nullptr;//待删除节点的父节点
Node* cur = _root;//待删除的节点
//不是空树
while (cur)
{
//往左边走
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
//往右边走
else if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
//找到待删除的节点
else
{
//待删除节点的左子树为空 ,即一个孩子的情况
if (cur->_left == nullptr)
{
//待删除节点是根节点
if (cur == _root)
{
//将根节点改为待删除节点的右孩子
_root = cur->_right;
}
//待删除节点不是根节点,并且此时parent不为nullptr
else
{
if (parent->_right == cur)
{
parent->_right = cur->_right;
}
else//parent->_left ==cur
{
parent->_left = cur->_right;
}
}
}
//待删除节点的右子树为空 ,即一个孩子的情况
else if (cur->_right == nullptr)
{
//待删除节点是根节点
if (cur == _root)
{
//将根节点改为待删除节点的左孩子
_root = cur->_left;
}
//待删除节点不是根节点,并且此时parent不为nullptr
else
{
if (parent->_right == cur)
{
parent->_right = cur->_left;
}
else//parent->_left==cur
{
parent->_left = cur->_left;
}
}
}
else //待删除的节点的左右孩子都不为空 (替换法:左子树的最大节点即最右节点,或者右子树的最小节点即最左节点,并且将替换的节点删除)
{
//替换法
//找替代节点
Node* parent = cur;
//找左子树的最大节点,左子树的最大节点一定没有右孩子
Node* leftMax = cur->_left;
while (leftMax->_right)
{
parent = leftMax; //记录leftMax的父节点,防止删除leftMax时找不到该节点位置
//一直往右子树找
leftMax = leftMax->_right;
}
//左子树的最大节点和待删除节点替换
swap(cur->_key, leftMax->_key);
//重新改变链接关系
//特殊情况
if (parent->_left == leftMax)
{
parent->_left = leftMax->_left;
}
else//普通情况 parent->_right== leftMax
{
parent->_right = leftMax->_left;
}
cur = leftMax;
}
//删除左子树的最大节点
delete cur;
return true;
}
}
return false;
}
递归
bool EraseR(Node* _root, const K& key)//递归版本
{
return _EraseR(_root, key);
}
private:
bool _EraseR(Node*& root, const K& key)//引用的目的:不用找父节点,不需要用父节点比较大小
{
//结束条件
if (root == nullptr)
{
return false;
}
//往左树找
if (root->_key > key)
{
return _EraseR(root->_left, key);
}
//往右树找
else if (root->_key < key)
{
return _EraseR(root->_right, key);
}
else//找到,开始删除
{
Node* del = root;
//待删除节点的左子树为空 ,即一个孩子的情况
if (root->_left == nullptr)
{
root = root->_right;
}
//待删除节点的右子树为空 ,即一个孩子的情况
else if (root->_right == nullptr)
{
root = root->_left;
}
//待删除的节点的左右孩子都不为空 (替换法:左子树的最大节点即最右节点,或者右子树的最小节点即最左节点,并且将替换的节点删除)
else
{
//找左子树最大节点
Node* leftMax = root->_left;
//一直往左边找,直到找到左子树最大节点
while (root->_left)
{
root = root->_left;
}
//将左子树最大节点与被删除节点替换
swap(leftMax->_key, root->_key);
return _EraseR(root, key);
}
delete del;//?
return true;
}
}
Find
循环
bool Find(const K & key)
{
Node* cur = _root;
while (cur)
{
if (cur->_left > key)
{
cur = cur->_left;
}
else if (cur->_left < key)
{
cur = cur->_right;
}
else
{
return false;
}
return true;
}
}
递归
bool FindR(Node* _root, const K& key)//递归版本
{
return _FindR(_root, key);
}
private:
bool _FindR(Node* root, const K& key)
{
//结束条件
if (root == nullptr)
{
return false;
}
if (root->_key > key)
{
return _FindR(root->_left, key);
}
else if (root->_key < key)
{
return _FindR(root->_right, key);
}
else
{
return true;
}
}
二叉搜索树的应用
K模型
K模型,即只有key作为关键码,结构中只需存储key即可,关键码即为需要搜索到的值。
比如:给定一个单词,判断该单词是否拼写正确。具体方式如下:
void TestBSTree1()
{
BSTree<string, string > dict;
dict.InsertR("insert", "插入");
dict.InsertR("sort", "排序");
dict.InsertR("right", "右边");
dict.InsertR("date", "日期");
string str;
while (cin>>str)
{
auto * ret = dict.FindR(str);
//auto ret = dict.FindR(str);
if (ret)
{
cout << ret->_value << endl;
}
else
{
cout << "无此单词" << endl;
}
}
}
KV模型
KV模型,对于每一个关键码key,都有与之对应的值value,即<key, value>的键值对。
英汉词典就是英文与中文的对应关系,即<word, Chinese>就构成一种键值对。具体方式如下
1、以<单词, 中文含义>为键值对,构建一棵二叉搜索树。注意:二叉搜索树需要进行比较,键值对比较时只比较key。
2、查询英文单词时,只需给出英文单词就可以快速找到与其对应的中文含义。
完整代码
普通版本
#pragma once
template <class K>
struct BSTreeNode
{
BSTreeNode<K>* _left;
BSTreeNode<K>* _right;
K _key;
BSTreeNode(const K & key)
:_left(nullptr)
,_right(nullptr)
,_key(key)
{
}
};
template <class K>
class BSTree
{
typedef BSTreeNode<K> Node;
public:
BSTree()
:_root(nullptr)
{
}
bool Insert(const K & key )
{
//空树
if (_root == nullptr)
{
_root = new Node(key);
return true;
}
//不是空树
Node* parent = nullptr;//找到父节点
Node* cur = _root;
while (cur)
{
//比较
if (cur->_key < key)
{
//往右子树走
parent = cur;
cur = cur->_right;
}
else if (cur->_key > key)
{
//往左子树走
parent = cur;
cur = cur->_left;
}
else
{
return false;
}
}
//插入节点
cur = new Node(key);
//不知道parent在那一边,需要进一步判断
if (parent->_key > key)
{
//parent在左边
parent->_left = cur;
}
else if (parent->_key < key)
{
//parent在右边
parent->_right = cur;
}
else
{
return false;
}
return true;
}
bool Find(const K & key)
{
Node* cur = _root;
while (cur)
{
if (cur->_left > key)
{
cur = cur->_left;
}
else if (cur->_left < key)
{
cur = cur->_right;
}
else
{
return false;
}
return true;
}
}
bool Erase(const K& key)
{
Node* parent = nullptr;//待删除节点的父节点
Node* cur = _root;//待删除的节点
//不是空树
while (cur)
{
//往左边走
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
//往右边走
else if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
//找到待删除的节点
else
{
//待删除节点的左子树为空 ,即一个孩子的情况
if (cur->_left == nullptr)
{
//待删除节点是根节点
if (cur == _root)
{
//将根节点改为待删除节点的右孩子
_root = cur->_right;
}
//待删除节点不是根节点,并且此时parent不为nullptr
else
{
if (parent->_right == cur)
{
parent->_right = cur->_right;
}
else//parent->_left ==cur
{
parent->_left = cur->_right;
}
}
}
//待删除节点的右子树为空 ,即一个孩子的情况
else if (cur->_right == nullptr)
{
//待删除节点是根节点
if (cur == _root)
{
//将根节点改为待删除节点的左孩子
_root = cur->_left;
}
//待删除节点不是根节点,并且此时parent不为nullptr
else
{
if (parent->_right == cur)
{
parent->_right = cur->_left;
}
else//parent->_left==cur
{
parent->_left = cur->_left;
}
}
}
else //待删除的节点的左右孩子都不为空 (替换法:左子树的最大节点即最右节点,或者右子树的最小节点即最左节点,并且将替换的节点删除)
{
//替换法
//找替代节点
Node* parent = cur;
//找左子树的最大节点
Node* leftMax = cur->_left;
while (leftMax->_right)
{
parent = leftMax; //记录leftMax的父节点,防止删除leftMax时找不到该节点位置
//一直往右子树找
leftMax = leftMax->_right;
}
//左子树的最大节点和待删除节点替换
swap(cur->_key, leftMax->_key);
//重新改变链接关系
//特殊情况
if (parent->_left == leftMax)
{
parent->_left = leftMax->_left;
}
else//普通情况
{
parent->_right = leftMax->_left;
//parent->_right =nullptr;
}
cur = leftMax;
}
//删除左子树的最大节点
delete cur;
return true;
}
}
return false;
}
//中序遍历
void InOrder()
{
_InOrder(_root);
cout << endl;
}
void _InOrder(Node *root )
{
if (root == nullptr)
{
return;
}
_InOrder(root->_left);
cout << root->_key << " ";
_InOrder(root->_right);
}
private:
Node* _root;
};
void TestBSTree1()
{
int a[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };
BSTree<int> t;
for (auto e : a)
{
t.Insert(e);
}
t.InOrder();
t.Erase(4);
t.InOrder();
t.Erase(6);
t.InOrder();
t.Erase(7);
t.InOrder();
t.Erase(3);
t.InOrder();
for (auto e : a)
{
t.Erase(e);
}
t.InOrder();
}
递归版本
#pragma once
#include<string>
using namespace std;
namespace key
{
template <class K>
struct BSTreeNode
{
BSTreeNode<K>* _left;
BSTreeNode<K>* _right;
K _key;
BSTreeNode(const K& key)
:_left(nullptr)
, _right(nullptr)
, _key(key)
{
}
};
template <class K>
class BSTree
{
public:
typedef BSTreeNode<K> Node;
public:
BSTree()
:_root(nullptr)
{
}
~BSTree()
{
Destroy(_root);
}
//拷贝构造
BSTree(const BSTree<K>& t)
//BSTree( BSTree<K> *this , const BSTree<K> & t)
//t1 =t
{
_root = Copy(t._root);
}
//赋值重载
BSTree<K>& operator= (BSTree<K>& t)
//t1 = t
{
swap(_root, t._root);
return *this;
}
bool EraseR(Node* _root, const K& key)//递归版本
{
return _EraseR(_root, key);
}
bool InsertR(const K& key)//递归版本
{
return _InsertR(_root, key);
}
bool FindR(Node* _root, const K& key)//递归版本
{
return _FindR(_root, key);
}
private:
Node* Copy(Node* root)
{
if (root == nullptr)
{
return nullptr;
}
Node* copyNode = new Node(root->_key);
//递归
copyNode->_left = Copy(root->_left);
copyNode->_right = Copy(root->_right);
return copyNode;
}
void Destroy(Node*& root) //引用的目的:将每个节点释放后同时置空
{
//后序遍历
if (root == nullptr)
{
return;
}
Destroy(root->_left);
Destroy(root->_right);
delete root;
root = nullptr;
}
bool _InsertR(Node*& root, const K& key) //引用的目的:不用找父节点,不需要用父节点比较大小
{
//结束条件
if (root == nullptr)
{
root = new Node(key);
return true;
}
//往左子树走
if (root->_key > key)
{
return _InsertR(root->_left, key);
}
//往右子树走
else if (root->_key < key)
{
return _InsertR(root->_right, key);
}
else
{
return false;
}
}
bool _EraseR(Node*& root, const K& key)//引用的目的:不用找父节点,不需要用父节点比较大小
{
//结束条件
if (root == nullptr)
{
return false;
}
//往左树找
if (root->_key > key)
{
return _EraseR(root->_left, key);
}
//往右树找
else if (root->_key < key)
{
return _EraseR(root->_right, key);
}
else//找到,开始删除
{
Node* del = root;
//待删除节点的左子树为空 ,即一个孩子的情况
if (root->_left == nullptr)
{
root = root->_right;
}
//待删除节点的右子树为空 ,即一个孩子的情况
else if (root->_right == nullptr)
{
root = root->_left;
}
//待删除的节点的左右孩子都不为空 (替换法:左子树的最大节点即最右节点,或者右子树的最小节点即最左节点,并且将替换的节点删除)
else
{
//找左子树最大节点
Node* leftMax = root->_left;
//一直往左边找,直到找到左子树最大节点
while (root->_left)
{
root = root->_left;
}
//将左子树最大节点与被删除节点替换
swap(leftMax->_key, root->_key);
return _EraseR(root, key);
}
delete del;//?
return true;
}
}
bool _FindR(Node* root, const K& key)
{
//结束条件
if (root == nullptr)
{
return false;
}
if (root->_key > key)
{
return _FindR(root->_left, key);
}
else if (root->_key < key)
{
return _FindR(root->_right, key);
}
else
{
return true;
}
}
public:
//中序遍历
void InOrder()
{
_InOrder(_root);
cout << endl;
}
void _InOrder(Node* root)
{
if (root == nullptr)
{
return;
}
_InOrder(root->_left);
cout << root->_key << " ";
_InOrder(root->_right);
}
public:
Node* _root;
};
void TestBSTree1()
{
int a[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };
BSTree<int> t;
for (auto e : a)
{
t.InsertR(e);
}
t.InOrder();
//没有引用,释放了,只是指针没有置空,尤其是根节点_root,我们还能通过他找到
/*t.Destroy(t._root);*/
t.EraseR(t._root, 4);
t.InOrder();
t.EraseR(t._root, 6);
t.InOrder();
t.EraseR(t._root, 7);
t.InOrder();
t.EraseR(t._root, 3);
t.InOrder();
for (auto e : a)
{
t.EraseR(t._root, e);
}
t.InOrder();
}
void TestBSTree2()
{
int a[] = { 8, 3, 1, 10, 6, 4, 7, 14, 13 };
BSTree<int> t;
for (auto e : a)
{
t.InsertR(e);
}
t.InOrder();
BSTree<int> t1(t);
t.InOrder();
t1.InOrder();
}
}
namespace key_value
{
template<class K, class V>
struct BSTreeNode
{
BSTreeNode<K, V>* _left;
BSTreeNode<K, V>* _right;
K _key;
V _value;
BSTreeNode(const K& key, const V& value)
:_left(nullptr)
, _right(nullptr)
, _key(key)
, _value(value)
{}
};
template<class K, class V>
class BSTree
{
public:
typedef BSTreeNode<K, V> Node;
public:
BSTree()
:_root(nullptr)
{}
void InOrder()
{
_InOrder(_root);
cout << endl;
}
Node* FindR(const K& key)
{
return _FindR(_root, key);
}
bool InsertR(const K& key, const V& value)
{
return _InsertR(_root, key, value);
}
bool EraseR(const K& key)
{
return _EraseR(_root, key);
}
private:
bool _EraseR(Node*& root, const K& key)
{
if (root == nullptr)
return false;
if (root->_key < key)
{
return _EraseR(root->_right, key);
}
else if (root->_key > key)
{
return _EraseR(root->_left, key);
}
else
{
Node* del = root;
// 1、左为空
// 2、右为空
// 3、左右都不为空
if (root->_left == nullptr)
{
root = root->_right;
}
else if (root->_right == nullptr)
{
root = root->_left;
}
else
{
Node* leftMax = root->_left;
while (leftMax->_right)
{
leftMax = leftMax->_right;
}
swap(root->_key, leftMax->_key);
return _EraseR(root->_left, key);
}
delete del;
return true;
}
}
bool _InsertR(Node*& root, const K& key, const V& value)
{
if (root == nullptr)
{
root = new Node(key, value);
return true;
}
if (root->_key < key)
{
return _InsertR(root->_right, key, value);
}
else if (root->_key > key)
{
return _InsertR(root->_left, key, value);
}
else
{
return false;
}
}
Node* _FindR(Node* root, const K& key)
{
if (root == nullptr)
return nullptr;
if (root->_key < key)
{
return _FindR(root->_right, key);
}
else if (root->_key > key)
{
return _FindR(root->_left, key);
}
else
{
return root;
}
}
void _InOrder(Node* root)
{
if (root == NULL)
{
return;
}
_InOrder(root->_left);
cout << root->_key << ":" << root->_value << endl;
_InOrder(root->_right);
}
private:
Node* _root;
};
void TestBSTree1()
{
BSTree<string, string > dict;
dict.InsertR("insert", "插入");
dict.InsertR("sort", "排序");
dict.InsertR("right", "右边");
dict.InsertR("date", "日期");
string str;
while (cin>>str)
{
auto * ret = dict.FindR(str);
//auto ret = dict.FindR(str);
if (ret)
{
cout << ret->_value << endl;
}
else
{
cout << "无此单词" << endl;
}
}
}
void TestBSTree2()
{
string arr[] = {"西瓜", "西瓜", "苹果", "西瓜", "苹果", "苹果", "西瓜", "苹果", "香蕉", "苹果", "香蕉" };
BSTree<string, int > countTree;
for (auto &str : arr)
{
auto ret = countTree.FindR(str);
if (ret == nullptr)
{
countTree.InsertR(str,1);
}
else
{
ret->_value++;
}
}
countTree.InOrder();
}
}
如果你觉得这篇文章对你有帮助,不妨动动手指给点赞收藏加转发,给鄃鳕一个大大的关注你们的每一次支持都将转化为我前进的动力!!!