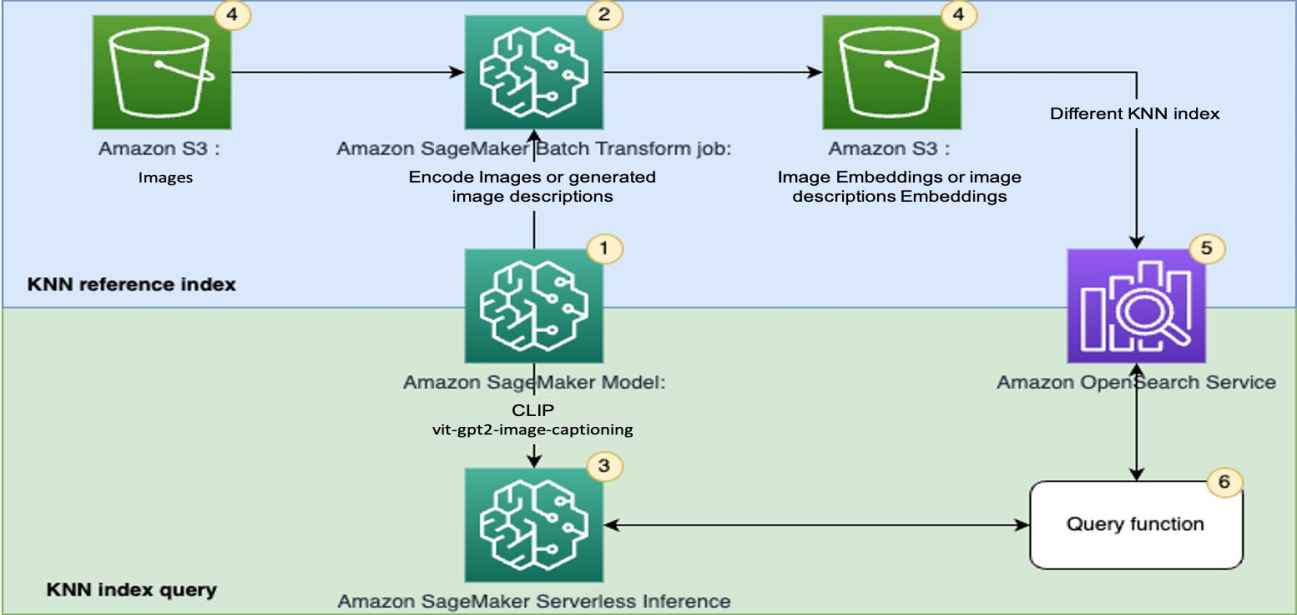
二叉搜索树（C++）

news2025/1/15 16:45:27

二叉搜索树

  • 概念
  • 二叉搜索树的应用
  • 二叉搜索树的实现
    • K模型
      • 基本结构和函数声明
      • 接口实现
        • ①find——查找关键码
        • ②Insert——插入关键码
        • ③Erase——删除关键码（==重点==）
        • 时间复杂度
      • 源码（整体）
        • 非递归
        • 递归
    • KV模型

在使用C语言写数据结构阶段时，对二叉树进行了讲解。本节内容是对二叉树的深入探索，也是二叉树部分的收尾

概念

二叉搜索树也称二叉排序树（BST，Binary Search Tree）：

  1. 空树
  2. 非空树（要具有以下性质）
    • 若它的左子树不为空，则左子树上所有节点的值都小于根节点的值
    • 若它的右子树不为空，则右子树上所有节点的值都大于根节点的值
    • 它的左右子树也分别为二叉搜索树

注意： 二叉搜索树的key值互不相同

eg:下图就属于二叉搜索树
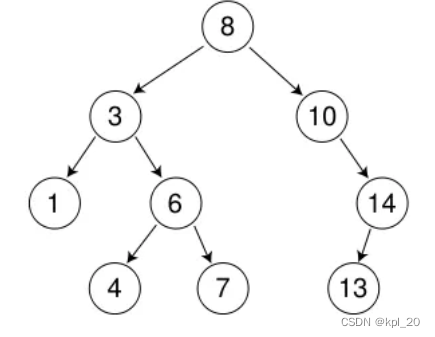
二叉搜索树

二叉搜索树的应用

  • K模型：即只有key作为关键码，结构中只需要存储key。关键码就是要搜索到的值

     eg: 给一个单词word，判断该单词是否拼写正确？
 
 具体步骤：
        1. 以词库中所有单词为基础，每个单词都是key，构建一颗搜索二叉树
        2. 在二叉树中搜索该单词是否存在，存在则返回true，否则返回false

  • KV模型：每一个关键码key，都有与之对应的value，即<Key, Value>的键值对

     eg1: 英汉词典 - 就是中文与英文的对应关系。通过英文可以快速找到与其对应的中文，英文单词和与其对应的中文<word, chinese>就构成键值对。
 eg2: 统计单词次数，统计成功后，给定单词就可以找到单词出现得次数。单词与其出现次数就是<word, count>就构成一种键值对

二叉搜索树的实现

注意： 通过二叉搜索树的应用，了解到其有两个模型，接下来对这两个模型分别进行实现。因为两个模型的接口都相似，所以仅对一个模型的接口进行详细介绍。

K模型

K模型的实现分为递归和非递归两种，但是接口的实现逻辑是一样的。为了便于理解把基本结构和函数声明先附上，然后对接口进行讲解，最后在贴上完整的源码

基本结构和函数声明

//二叉树节点
//节点使用struct，默认public访问
template<class K>
struct BSTreeNode   
{
	BSTreeNode<K>* _left;
	BSTreeNode<K>* _right;
	K _key;


	BSTreeNode(const K& key)
		:_left(nullptr)
		, _right(nullptr)
		, _key(key)
	{}
};

template<class K>
class BSTree
{
	typedef BSTreeNode<K> Node;
public:
	//默认构造
	BSTree()
		:_root(nullptr)
	{}

	//拷贝构造
	BSTree(const BSTree<K>& t);

	//赋值运算符重载
	BSTree<K>& operator=(BSTree<K> t);

	//析构函数
	~BSTree();

	//插入
	bool Insert(const K& key);

	//查找
	bool Find(const K& key);

	//删除
	bool Erase(const K& key);

	//中序遍历
	void InOrder();

private:
	Node* _root;
};

接口实现

①find——查找关键码

功能：查找关键码是否在树中，是返回真，否则返回假。
原理：

  1. 从根开始比较，比根大则往右边查找，比根小往左边查找
  2. 最多查找高度次，走到nullptr，则这个值不存在

实现代码

  1. 非递归版本
template<class K>
bool BSTree<K>::Find(const K& key)
{
	Node* cur = _root;
	while (cur)
	{
		if (cur->_key > key)
		{
			cur = cur->_left;
		}
		else if (cur->_key < key)
		{
			cur = cur->_right;
		}
		else
		{
			return true;
		}
	}
	return false;
}
  1. 递归版本
bool Find(const K& key)
{
	Node* cur = _root;
	while (cur)
	{
		if (cur->_key > key)
		{
			cur = cur->_left;
		}
		else if (cur->_key < key)
		{
			cur = cur->_right;
		}
		else
		{
			return true;
		}
	}
	return false;
}

②Insert——插入关键码

原理：

  1. 树为空，直接把要插入的节点赋值给root
  2. 树不为空，通过查找的思路，找到要插入的合适位置。插入成功返回true
    注意：如果要插入的值和树中的值冲突，则返回false

实现代码

  1. 非递归版本
//插入
bool Insert(const K& key)
{
	if (_root == nullptr)
	{
		_root = new Node(key);
		return true;
	}

	Node* parent = nullptr;
	Node* cur = _root;
	while (cur)
	{
		if (cur->_key > key)
		{
			parent = cur;
			cur = cur->_left;
		}
		else if (cur->_key < key)
		{
			parent = cur;
			cur = cur->_right;
		}
		else
		{
			return false;
		}
	}

	cur = new Node(key);
	if (parent->_key > key)
	{
		parent->_left = cur;
	}
	else
	{
		parent->_right = cur;
	}
	return true;
}
  1. 递归版本
bool InsertR(const K& key)
{
	return _InsertR(_root, key);
}
bool _FindR(Node* root, const K& key)
{
	if (root == nullptr)
		return false;

	if (root->_key > key)
		return _FindR(root->_left, key);
	else if (root->_key < key)
		return _FindR(root->_right, key);
	else
		return true;
}

③Erase——删除关键码（重点）

原理：

  1. 查找关键码是否在二叉搜索树中，不存在直接返回false
  2. 存在，则要分为四种情况

情况：
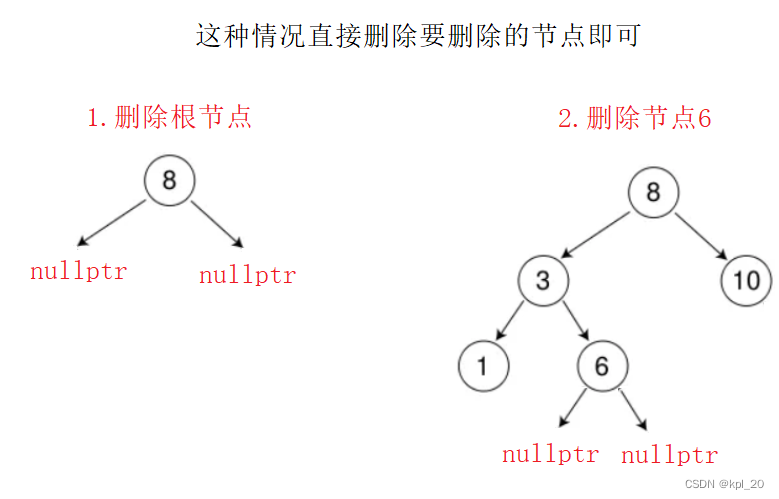
在考虑树的所有情况时，要把根节点的情况和普通情况，分离开来。哪怕最后根节点的情况和普通情况一样。（仅代表博主个人观点）

  1. 要删除的节点无孩子节点
    第一种情况
  1. 要删除的节点无左孩子节点
    第二种情况
  1. 要删除的节点无右孩子节点
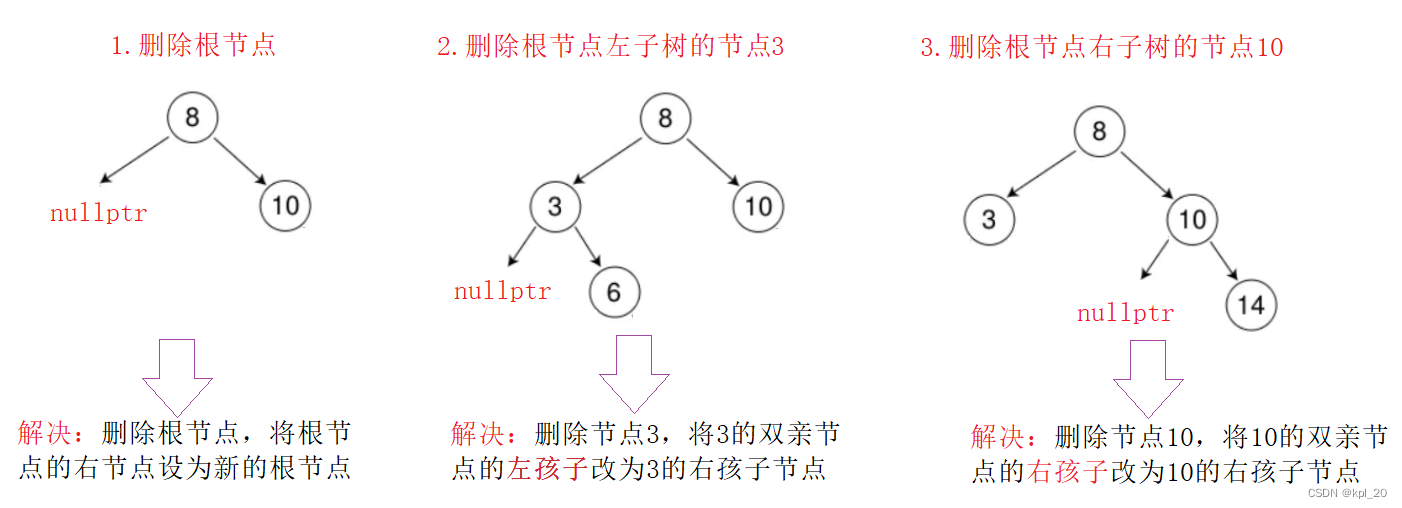
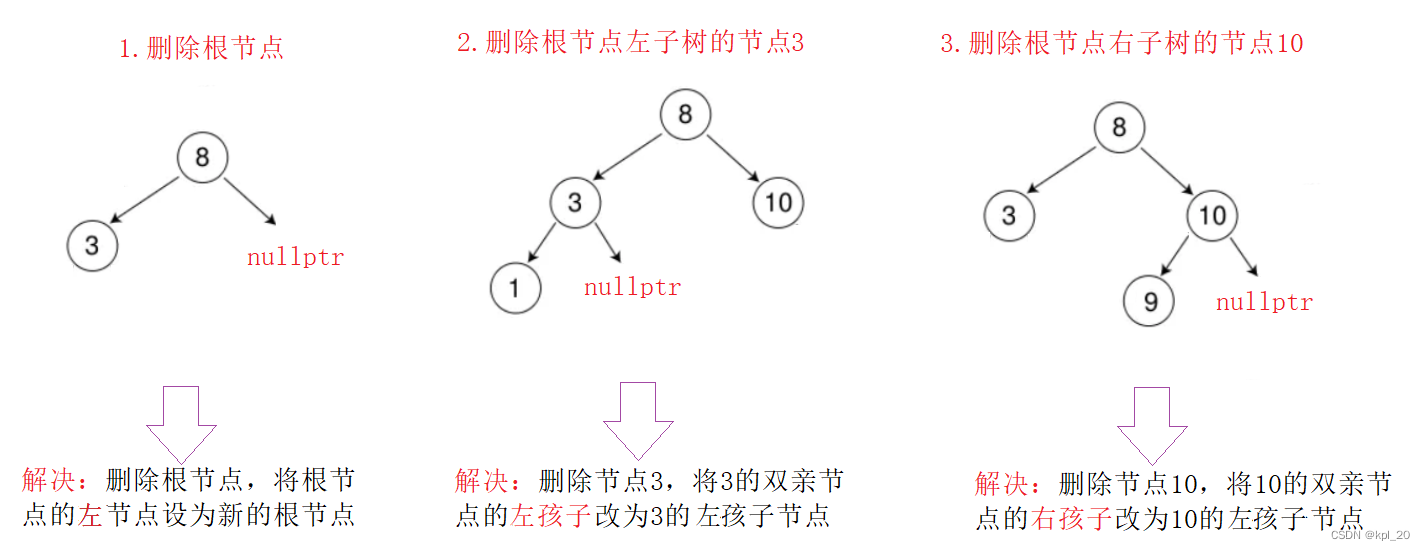
    第三种情况
  1. 要删除的节点左右都不为空 （采用的方法 一 替换法）
    替换法：找到删除节点左子树的最大值节点(leftMax)，然后与删除节点交换，再删除现在的leftMax。（也可以找删除节点右子树的最小值节点(rightMin)，原理相同）
    第四种情况

实现代码

  1. 非递归版本
//删除
bool Erase(const K& key)
{
	Node* parent = nullptr;
	Node* cur = _root;
	while (cur)
	{
		if (cur->_key > key)
		{
			parent = cur;
			cur = cur->_left;
		}
		else if (cur->_key < key)
		{
			parent = cur;
			cur = cur->_right;
		}
		else  //找到了要删除的值
		{
			//分情况

			//1.左为空
			if (cur->_left == nullptr)
			{
				if (cur == _root)
					_root = cur->_right;
				else
				{
					if (parent->_right == cur)
					{
						parent->_right = cur->_right;
					}
					else
					{
						parent->_left = cur->_right;
					}
				}
			}//2.右为空
			else if (cur->_right == nullptr)
			{
				if (cur == _root)
					_root = cur->_left;
				else
				{
					if (parent->_right == cur)
					{
						parent->_right = cur->_left;
					}
					else
					{
						parent->_left = cur->_left;
					}
				}
			} //3.左右都不为空
			else
			{
				parent = cur;
				Node* leftMax = cur->_left;
				while (leftMax->_right)
				{
					parent = leftMax;
					leftMax = leftMax->_right;
				}

				swap(cur->_key, leftMax->_key);
				if (parent->_left == leftMax)
				{
					parent->_left = leftMax->_left;
				}
				else
				{
					parent->_right = leftMax->_left;
				}
				cur = leftMax;
			}

			delete cur;
			cur = nullptr;
			return true;
		}
	}
	return false;
}
  1. 递归版本
//删除
bool EraseR(const K& key)
{
	return _EraseR(_root, key);
}

bool _EraseR(Node*& root, const K& key)
{
	if (root == nullptr)
		return false;

	if (root->_key < key)
		return _EraseR(root->_right, key);
	else if (root->_key > key)
		return _EraseR(root->_left, key);
	else
	{
		Node* del = root;
		if (root->_left == nullptr)
			root = root->_right;
		else if (root->_right == nullptr)
			root = root->_left;
		else
		{
			Node* leftMax = root->_left;
			while (leftMax->_right)
			{
				leftMax = leftMax->_right;
			}
			swap(root->_key, leftMax->_key);

			return _EraseR(root->_left, key);
		}
		delete del;
		del = nullptr;
		return true;
	}
}

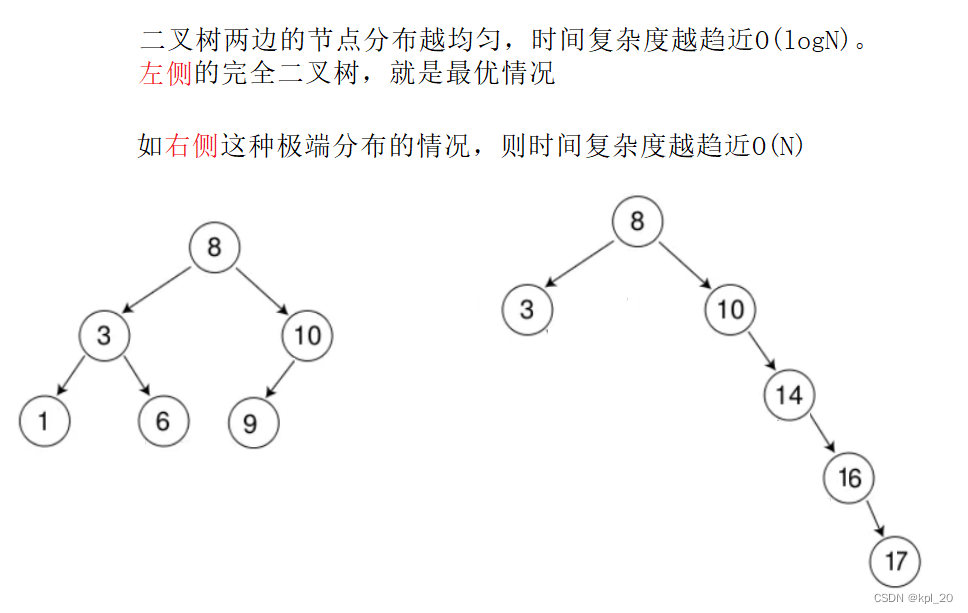
时间复杂度

二叉搜索树的操作时间复杂度：在O(logN)和O(N)之间。
画图解释时间复杂度：
时间复杂度

源码（整体）

非递归

//非递归
namespace key
{
	//二叉树节点
	//节点使用struct，默认public访问
	template<class K>
	struct BSTreeNode
	{
		BSTreeNode<K>* _left;
		BSTreeNode<K>* _right;
		K _key;


		BSTreeNode(const K& key)
			:_left(nullptr)
			, _right(nullptr)
			, _key(key)
		{}
	};

	template<class K>
	class BSTree
	{
		typedef BSTreeNode<K> Node;
	public:
		//默认构造
		BSTree()
			:_root(nullptr)
		{}

		//拷贝构造
		BSTree(const BSTree<K>& t)
		{
			_root = Copy(t._root);
		}

		//赋值运算符重载
		BSTree<K>& operator=(BSTree<K> t)
		{
			swap(_root, t._root);
			return *this;
		}

		//析构函数
		~BSTree()
		{
			Destroy(_root);
		}

		//插入
		bool Insert(const K& key)
		{
			if (_root == nullptr)
			{
				_root = new Node(key);
				return true;
			}

			Node* parent = nullptr;
			Node* cur = _root;
			while (cur)
			{
				if (cur->_key > key)
				{
					parent = cur;
					cur = cur->_left;
				}
				else if (cur->_key < key)
				{
					parent = cur;
					cur = cur->_right;
				}
				else
				{
					return false;
				}
			}

			cur = new Node(key);
			
			if (parent->_key > key)
			{
				parent->_left = cur;
			}
			else
			{
				parent->_right = cur;
			}
			return true;
		}

		//查找
		bool Find(const K& key)
		{
			Node* cur = _root;
			while (cur)
			{
				if (cur->_key > key)
				{
					cur = cur->_left;
				}
				else if (cur->_key < key)
				{
					cur = cur->_right;
				}
				else
				{
					return true;
				}
			}
			return false;
		}

		//删除
		bool Erase(const K& key)
		{
			Node* parent = nullptr;
			Node* cur = _root;
			while (cur)
			{
				if (cur->_key > key)
				{
					parent = cur;
					cur = cur->_left;
				}
				else if (cur->_key < key)
				{
					parent = cur;
					cur = cur->_right;
				}
				else  //找到了要删除的值
				{
					//分情况

					//1.左为空
					if (cur->_left == nullptr)
					{
						if (cur == _root)
							_root = cur->_right;
						else
						{
							if (parent->_right == cur)
							{
								parent->_right = cur->_right;
							}
							else
							{
								parent->_left = cur->_right;
							}
						}
					}//2.右为空
					else if (cur->_right == nullptr)
					{
						if (cur == _root)
							_root = cur->_left;
						else
						{
							if (parent->_right == cur)
							{
								parent->_right = cur->_left;
							}
							else
							{
								parent->_left = cur->_left;
							}
						}
					} //3.左右都不为空
					else
					{
						parent = cur;
						Node* leftMax = cur->_left;
						while (leftMax->_right)
						{
							parent = leftMax;
							leftMax = leftMax->_right;
						}

						swap(cur->_key, leftMax->_key);
						if (parent->_left == leftMax)
						{
							parent->_left = leftMax->_left;
						}
						else
						{
							parent->_right = leftMax->_left;
						}
						cur = leftMax;
					}

					delete cur;
					cur = nullptr;
					return true;
				}
			}
			return false;
		}

		//中序遍历
		void InOrder()
		{
			_InOrder(_root);
			cout << endl;
		}
	private:
		Node* Copy(Node* root)
		{
			if (root == nullptr)
				return nullptr;
			Node* copyRoot = new Node(root->_key);
			copyRoot->_left = Copy(root->_left);
			copyRoot->_right = Copy(root->_tight);

			return copyRoot;
		}

		void Destroy(Node*& root)
		{
			if (root == nullptr)
				return;
			Destroy(root->_left);
			Destroy(root->_right);
			delete root;
			root = nullptr;
		}

		void _InOrder(Node* root)
		{
			if (root == nullptr)
				return;
			_InOrder(root->_left);
			cout << root->_key << " ";
			_InOrder(root->_right);
		}

	private:
		Node* _root;
	};
}

递归

//递归
namespace keyR
{
	//二叉树节点
	//节点使用struct，默认public访问
	template<class K>
	struct BSTreeNode
	{
		BSTreeNode<K>* _left;
		BSTreeNode<K>* _right;
		K _key;


		BSTreeNode(const K& key)
			:_left(nullptr)
			, _right(nullptr)
			, _key(key)
		{}
	};

	template<class K>
	class BSTree
	{
		typedef BSTreeNode<K> Node;
	public:
		//默认构造
		BSTree()
			:_root(nullptr)
		{}

		//拷贝构造
		BSTree(const BSTree<K>& t)
		{
			_root = Copy(t._root);
		}

		//赋值运算符重载
		BSTree<K>& operator=(BSTree<K> t)
		{
			swap(_root, t._root);

			return *this;
		}

		//析构函数
		~BSTree()
		{
			Destroy(_root);
		}

		//插入
		bool InsertR(const K& key)
		{
			return _InsertR(_root, key);
		}

		//查找
		bool FindR(const K& key)
		{
			return _FindR(_root, key);
		}

		//删除
		bool EraseR(const K& key)
		{
			return _EraseR(_root, key);
		}

		//中序遍历
		void InOrder()
		{
			_InOrder(_root);
			cout << endl;
		}

	private:
		//在root添加引用很关键，要不然得是二级指针
		bool _InsertR(Node*& root, const K& key)
		{
			if (root == nullptr)
			{
				root = new Node(key);
				return true;
			}

			if (root->_key > key)
				return _InsertR(root->_left, key);
			else if (root->_key < key)
				return _InsertR(root->_right, key);
			else
				return false;
		}

		bool _EraseR(Node*& root, const K& key)
		{
			if (root == nullptr)
				return false;

			if (root->_key < key)
				return _EraseR(root->_right, key);
			else if (root->_key > key)
				return _EraseR(root->_left, key);
			else
			{
				Node* del = root;
				if (root->_left == nullptr)
					root = root->_right;
				else if (root->_right == nullptr)
					root = root->_left;
				else
				{
					Node* leftMax = root->_left;
					while (leftMax->_right)
					{
						leftMax = leftMax->_right;
					}
					swap(root->_key, leftMax->_key);

					return _EraseR(root->_left, key);
				}
				delete del;
				del = nullptr;
				return true;
			}
		}

		bool _FindR(Node* root, const K& key)
		{
			if (root == nullptr)
				return false;

			if (root->_key > key)
				return _FindR(root->_left, key);
			else if (root->_key < key)
				return _FindR(root->_right, key);
			else
				return true;
		}

		void Destroy(Node*& root)
		{
			if (root == nullptr)
				return;
			Destroy(root->_left);
			Destroy(root->_right);
			delete root;
			root = nullptr;
		}

		Node* Copy(Node* root)
		{
			if (root == nullptr)
				return nullptr;

			Node* copyRoot = new Node(root->_key);
			copyRoot->_left = CopyRoot(root->_left);
			copyRoot->_right = CopyRoot(root->_right);

			return copyRoot;
		}

		void _InOrder(Node* root)
		{
			if (root == nullptr)
				return;
			_InOrder(root->_left);
			cout << root->_key << " ";
			_InOrder(root->_right);
		}

	private:
		Node* _root;
	};
}

KV模型

直接贴出源码：

namespace key_value
{
	template<class K, class V>
	struct BSTreeNode
	{
		BSTreeNode<K, V>* _left;
		BSTreeNode<K, V>* _right;
		K _key;
		V _value;

		BSTreeNode(const K& key, const V& value)
			:_left(nullptr)
			, _right(nullptr)
			, _key(key)
			, _value(value)
		{}
	};

	template<class K, class V>
	class BSTree
	{
		typedef BSTreeNode<K, V> Node;
	public:
		BSTree()
			:_root(nullptr)
		{}

		BSTree(const BSTree<K, V>& t)
		{
			_root = Copy(t._root);
		}

		BSTree<K, V>& operator=(BSTree<K, V> t)
		{
			swap(_root, t._root);
			return *this;
		}

		~BSTree()
		{
			Destroy(_root);
		}

		void InOrder()
		{
			_InOrder(_root);
			cout << endl;
		}

		Node* FindR(const K& key)
		{
			return _FindR(_root, key);
		}

		bool InsertR(const K& key, const V& value)
		{
			return _InsertR(_root, key, value);
		}

		bool EraseR(const K& key)
		{
			return _EraseR(_root, key);
		}

	private:
		bool _EraseR(Node*& root, const K& key)
		{
			if (root == nullptr)
				return false;

			if (root->_key < key)
			{
				return _EraseR(root->_right, key);
			}
			else if (root->_key > key)
			{
				return _EraseR(root->_left, key);
			}
			else
			{
				Node* del = root;
				if (root->_left == nullptr)
				{
					//这个root的引用，非常好，
					root = root->_right;
				}
				else if (root->_right == nullptr)
				{
					root = root->_left;
				}
				else
				{
					Node* leftMax = root->_left;
					while (leftMax->_right)
					{
						leftMax = leftMax->_right;
					}
					swap(root->_key, leftMax->_key);

					//删的值在左边
					return _EraseR(root->_left, key);
				}
				delete del;
				return true;

			}

		}


		void Destroy(Node*& root)
		{
			if (root == nullptr)
				return;

			Destroy(root->_left);
			Destroy(root->_right);
			delete root;
			root = nullptr;
		}

		Node* Copy(Node* root)
		{
			if (root == nullptr)
				return nullptr;

			Node* copyRoot = new Node(root->_key, root->_value);
			copyRoot->_left = Copy(root->_left);
			copyRoot->_right = Copy(root->_right);
			return copyRoot;
		}


		void _InOrder(Node* root)
		{
			if (root == nullptr)
				return;

			_InOrder(root->_left);
			cout << root->_key << ":" << root->_value << endl;
			_InOrder(root->_right);
		}

		Node* _FindR(Node* root, const K& key)
		{
			if (root == nullptr)
				return nullptr;

			if (root->_key > key)
			{
				return _FindR(root->_left, key);
			}
			else if (root->_key < key)
			{
				return _FindR(root->_right, key);
			}
			else
			{
				return root;
			}
		}

		bool _InsertR(Node*& root, const K& key, const V& value)
		{
			if (root == nullptr)
			{
				root = new Node(key, value);
				return true;
			}

			if (root->_key > key)
			{
				return _InsertR(root->_left, key, value);
			}
			else if (root->_key < key)
			{
				return _InsertR(root->_right, key, value);
			}
			else
			{
				return false;
			}
		}

	private:
		Node* _root;
	};

}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.coloradmin.cn/o/944677.html

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈，一经查实，立即删除！

相关文章

【Linux】进程通信 — 信号（下篇）

【Linux】进程通信 — 信号（下篇）

文章目录 &#x1f4d6; 前言1. 阻塞信号1.1 信号其他相关常见概念&#xff1a;1.2 sigset_t&#xff1a;1.2 - 1 信号集操作函数 1.3 sigprocmask&#xff1a;1.4 sigpending&#xff1a; 2. 进程处理信号2.1 内核页表和用户页表&#xff1a;2.2 内核态和用户态&#xff1a;2.…
阅读更多...
华为OD机试 - VLAN资源池 - 回溯、双指针（Java 2023 B卷 100分）

华为OD机试 - VLAN资源池 - 回溯、双指针（Java 2023 B卷 100分）

目录 专栏导读一、题目描述二、输入描述三、输出描述四、解题思路1、核心思想2、具体解题思路 五、Java算法源码六、效果展示1、输入2、输出 华为OD机试 2023B卷题库疯狂收录中&#xff0c;刷题点这里 专栏导读 本专栏收录于《华为OD机试&#xff08;JAVA&#xff09;真题&…
阅读更多...
产品帮助中心对SaaS行业的作用

产品帮助中心对SaaS行业的作用

帮助中心是一款SaaS产品必不可少的一部分&#xff0c;为了帮助用户更好的解决产品相关问题&#xff0c;提高新用户的使用体验&#xff0c;并且引导其更好地使用产品。 所以今天我们就来谈谈帮助中心对SaaS行业的作用&#xff0c;以及制作帮助中心的方法&#xff0c;每个产品帮…
阅读更多...
基于 OV5640 的图像采集显示系统（DVP 接口时序逻辑设计）

基于 OV5640 的图像采集显示系统（DVP 接口时序逻辑设计）

文章目录 前言一、DVP 接口时序逻辑设计二、基本数据流接收三、像素位置输出四、舍弃前 N 张图像五、系统异常状态恢复控制六、完整代码展示七、仿真代码展示八、仿真波形展示前言 上一节，我们已经完成了 OV5640 初始化逻辑的介绍。接下来，将要开始完成 DVP 接口的时序设计。…
阅读更多...
Maven入门教程(一)：安装Maven环境

Maven入门教程(一)：安装Maven环境

Maven项目对象模型(POM)&#xff0c;可以通过一小段描述信息来管理项目的构建&#xff0c;报告和文档的软件项目管理工具。 ​ 在项目开发中Maven可以对jar包和对工程之间的依赖关系进行管理。maven仓库中存储jar包&#xff0c;可以一次下载&#xff0c;所有项目通用。 1. 安装…
阅读更多...
Java项目-苍穹外卖-Day07-redis缓存应用-SpringCache/购物车功能

Java项目-苍穹外卖-Day07-redis缓存应用-SpringCache/购物车功能

文章目录 前言缓存菜品问题分析和实现思路缓存菜品数据清理缓存数据功能测试 SpringCache介绍入门案例 缓存套餐 前言 本章节主要是进行用户端的购物车功能开发 和redis作为mysql缓存的应用以及SpringCache的介绍 因为很多人查询数据库会导致mysql的查询效率降低&#xff0c;可…
阅读更多...
解读亚马逊云科技语义搜图检索方案

解读亚马逊云科技语义搜图检索方案

图像检索&#xff08;包括文搜图和图搜图&#xff09;是各个行业中常见的一个应用场景。比如在电商场景中&#xff0c;基于以图搜图做相似商品查找&#xff1b;在云相册场景中&#xff0c;基于文搜图来找寻所需的图像素材。 传统基于标签的图像检索方式&#xff0c;即先使用目标…
阅读更多...
压力传感器的性能技术指标

压力传感器的性能技术指标

压力传感器采用第四代无线传输方式&#xff0c;加入用高性能的感压芯片&#xff0c;配合先进的电路处理和温度补偿技术&#xff0c;选用不锈钢外壳做隔离防腐&#xff0c;能够测量与接触部分材质相兼容的气体和液体等介质的表压和绝压。 应用场合&#xff1a;如供水、排水、消…
阅读更多...
如何空手套白狼？一口气省7K再抓住一个7K起步的工作？

如何空手套白狼？一口气省7K再抓住一个7K起步的工作？

今日话题&#xff0c;教你如何省七千再得到一个七千起步的技能&#xff01;现在网络行业已经是全世界重点发展的目标&#xff0c;开发行业更是各个企业重点培养&#xff0c;但是在学校教的网络知识太基础太老掉牙&#xff1f;报班随便就是小一万该如何是好呢&#xff1f;解决方…
阅读更多...
树莓派3b无屏幕登录

树莓派3b无屏幕登录

如果要无屏登录&#xff0c;烧写时最好设置&#xff0c;勾选WIFI &#xff0c;登录密码&#xff0c;和SSH 树莓派操作系统下载地址 树莓派资源下载 | 树莓派实验室 无屏幕无键盘登录&#xff1a;新版中可能要先SSH登录&#xff0c;然后才能在RASPI-CONFIG中打开串口控制台 登录…
阅读更多...
1A快恢复整流二极管型号汇总

1A快恢复整流二极管型号汇总

快恢复整流二极管是二极管中的一种&#xff0c;开关特性好、反向恢复时间短&#xff0c;在开关电源、PWM脉宽调制器、变频器等电子电路中经常能看到它的身影。快恢复整流二极管的内部结构与普通PN结二极管不同&#xff0c;它属于PIN结型二极管&#xff0c;即在P型硅材料与N型硅…
阅读更多...
浏览器渲染机制

浏览器渲染机制

学习渡一课程、参考 必须明白的浏览器渲染机制 - 掘金 渲染机制的流程 HTML解析 布局 分层 绘制 分块 光栅化 画 HTML解析 - Parse HTML 解析html会生成一个 dom树和cssom树 document.styleSheets 可以看到cssom树 渲染阻塞 在渲染的过程中&#xff0c;遇到一个scr…
阅读更多...
并发编程01（Lock Condition 生产者消费者）详细讲解

并发编程01（Lock Condition 生产者消费者）详细讲解

并发 并发编程∶并发、并行 并发&#xff08;多线程操作同一个资源) CPU一核&#xff0c;模拟出来多条线程&#xff0c;天下武功&#xff0c;唯快不破&#xff0c;快速交替并行(多个人一起行走) CPU 多核&#xff0c;多个线程可以同时执行; public class QuickSort {public …
阅读更多...
hive部署

hive部署

下载hive安装包&#xff1a;https://dlcdn.apache.org/hive/hive-2.3.9/解压及环境部署 tar -zxvf apache-hive-2.3.9-bin.tar.gz mv apache-hive-2.3.9-bin hivevim /etc/profile添加至环境变量 export HIVE_HOME/usr/local/hive export PATH$PATH:$HIVE_HOME/binsource /etc…
阅读更多...
技术分享 | RCU ：内核小“马达”，让你的产品弯道超车

技术分享 | RCU ：内核小“马达”，让你的产品弯道超车

在上一篇文章《编程界也内卷&#xff1f;浅析“斜杠青年”RCU 》中&#xff0c;鼎道智联带着大家一起认识了并行编程&#xff0c;了解了什么是 RCU &#xff0c;相信大家已经对 RCU 的特点和如何实现 Reader 无锁有了一定的了解。 今天就带着大家继续从 RCU 的实现入手&#xf…
阅读更多...
ESDA in PySal (2) localjoincounts

ESDA in PySal (2) localjoincounts

ESDA in PySal (2) localjoincounts 参考：https://blog.csdn.net/angel0929/article/details/128433265 https://blog.csdn.net/allenlu2008/article/details/49895387 PySAL有5种全局自相关检验：Gamma值、Join Count、Moran’s I、Geary’s C、和Getis and Ord’s G 在下…
阅读更多...
CVE-2023-36874 Windows错误报告服务本地权限提升漏洞分析

CVE-2023-36874 Windows错误报告服务本地权限提升漏洞分析

CVE-2023-36874 Windows错误报告服务本地权限提升漏洞分析 漏洞简介 Windows错误报告服务在提交错误报告前会创建wermgr.exe进程&#xff0c;而攻击者使用特殊手法欺骗系统创建伪造的wermgr.exe进程&#xff0c;从而以system权限执行代码。 影响版本 Windows10 1507 * Wind…
阅读更多...
LC1011. 在 D 天内送达包裹的能力(JAVA)

LC1011. 在 D 天内送达包裹的能力(JAVA)

在 D 天内送达包裹的能力 题目描述上期经典算法 题目描述 leetcode 1011. 在 D 天内送达包裹的能力 难度 - 中等 传送带上的包裹必须在 days 天内从一个港口运送到另一个港口。 传送带上的第 i 个包裹的重量为 weights[i]。每一天&#xff0c;我们都会按给出重量&#xff08;we…
阅读更多...
java主要的垃圾回收算法

java主要的垃圾回收算法

垃圾收集算法了解吗&#xff1f; 标记-清除算法 标记 : 标记出所有需要回收的对象 清除&#xff1a;回收所有被标记的对象 主要存在两个缺点&#xff1a; 执行效率不稳定&#xff0c;如果 Java 堆中包含大量对象&#xff0c;而且其中大部分是需要被回收的&#xff0c;这时必…
阅读更多...
macOS使用命令行连接Oracle（SQL*Plus）

macOS使用命令行连接Oracle（SQL*Plus）

Author: histonevonzohomail.com Date: 2023/08/25 文章目录 SQL\*Plus安装下载环境配置 SQL\*Plus远程连接数据库参考文献 原文地址&#xff1a;https://histonevon.top/archives/oracle-mac-sqlplus数据库安装&#xff1a;Docker安装Oracle数据库 (histonevon.top) SQL*Plus…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023