题目

标题和出处

标题：叶子相似的树

出处：872. 叶子相似的树

难度

3 级

题目描述

要求

考虑一个二叉树上所有的叶子，这些叶子的值按从左到右的顺序排列形成一个叶值序列。

例如，上图给定的树的叶值序列为 $\text{(6, 7, 4, 9, 8)}$。

如果有两个二叉树的叶值序列相同，那么它们是叶相似的。

当且仅当给定的两个根结点分别为 $\text{root1}$ 和 $\text{root2}$ 的树是叶相似的情况下，返回 $\text{true}$。

示例

示例 1：

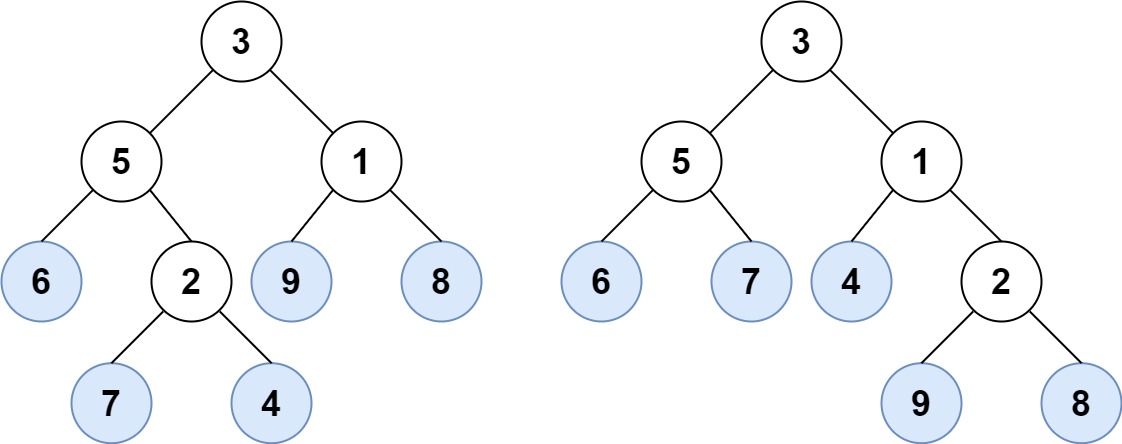
输入：$\text{root1 = [3,5,1,6,2,9,8,null,null,7,4], root2 = [3,5,1,6,7,4,2,null,null,null,null,null,null,9,8]}$
输出：$\text{true}$

示例 2：

输入：$\text{root1 = [1,2,3], root2 = [1,3,2]}$
输出：$\text{false}$

数据范围

• 每个树中结点数目在范围 $\text{[1, 200]}$ 内
• 每个树中结点值在范围 $\text{[0, 200]}$ 内

解法一

思路和算法

二叉树的叶值序列为所有叶结点值按照从左到右的顺序组成的序列。规定深度优先搜索的顺序为先遍历左子树再遍历右子树，则二叉树的叶值序列即为深度优先搜索遍历所有叶结点的顺序。

对于每个二叉树，从根结点开始深度优先搜索，访问根结点之后依次遍历左子树和右子树，并对左子树和右子树递归地遍历，访问到叶结点时将叶结点值加到叶值序列，遍历结束时即可得到二叉树的叶值序列。

两个二叉树都遍历结束之后，比较两个二叉树的叶值序列是否相等即可。

代码

class Solution {
    public boolean leafSimilar(TreeNode root1, TreeNode root2) {
        List<Integer> sequence1 = new ArrayList<Integer>();
        List<Integer> sequence2 = new ArrayList<Integer>();
        getLeafValueSequence(root1, sequence1);
        getLeafValueSequence(root2, sequence2);
        return sequence1.equals(sequence2);
    }

    public void getLeafValueSequence(TreeNode node, List<Integer> sequence) {
        if (node.left == null && node.right == null) {
            sequence.add(node.val);
        } else {
            if (node.left != null) {
                getLeafValueSequence(node.left, sequence);
            }
            if (node.right != null) {
                getLeafValueSequence(node.right, sequence);
            }
        }
    }
}

复杂度分析

• 时间复杂度：$O(m+n)$，其中 $m$ 和 $n$ 分别是两个二叉树的结点数。对两个二叉树深度优先搜索，每个结点都被访问一次。

• 空间复杂度：$O(m+n)$，其中 $m$ 和 $n$ 分别是两个二叉树的结点数。空间复杂度主要是递归调用的栈空间和两个二叉树的叶值序列空间。

解法二

思路和算法

也可以使用迭代实现深度优先搜索，需要使用栈存储结点。

从根结点开始遍历。每次访问一个结点之后，将当前结点入栈然后将当前结点移动到其左子结点，直到当前结点为空。然后将一个结点出栈，将当前结点设为出栈结点的右子结点。当所有结点都访问过时，遍历结束。

遍历过程中，访问到叶结点时将叶结点值加到叶值序列。两个二叉树都遍历结束之后，比较两个二叉树的叶值序列是否相等即可。

代码

class Solution {
    public boolean leafSimilar(TreeNode root1, TreeNode root2) {
        List<Integer> sequence1 = getLeafValueSequence(root1);
        List<Integer> sequence2 = getLeafValueSequence(root2);
        return sequence1.equals(sequence2);
    }

    public List<Integer> getLeafValueSequence(TreeNode root) {
        List<Integer> sequence = new ArrayList<Integer>();
        Deque<TreeNode> stack = new ArrayDeque<TreeNode>();
        TreeNode node = root;
        while (!stack.isEmpty() || node != null) {
            while (node != null) {
                if (node.left == null && node.right == null) {
                    sequence.add(node.val);
                }
                stack.push(node);
                node = node.left;
            }
            node = stack.pop().right;
        }
        return sequence;
    }
}

复杂度分析

• 时间复杂度：$O(m+n)$，其中 $m$ 和 $n$ 分别是两个二叉树的结点数。对两个二叉树深度优先搜索，每个结点都被访问一次。

• 空间复杂度：$O(m+n)$，其中 $m$ 和 $n$ 分别是两个二叉树的结点数。空间复杂度主要是栈空间和两个二叉树的叶值序列空间。