第十一届蓝桥杯国赛JavaB组题解

news2024/11/25 4:33:21

A. 美丽的2

在这里插入图片描述
思路：

枚举 1 到 2020 的每个数，依次判断即可。

代码：

public class Main {
	
	public static boolean check(int x) {
		while (x != 0) {
			if (x % 10 == 2) return true;
			x /= 10;
		}
		return false;
	}

	public static void main(String[] args) {
		// TODO Auto-generated method stub
		int cnt = 0;
		for (int i = 1; i <= 2020; i++) 
			if (check(i))
				cnt++;
		
		System.out.println(cnt);
	}

}

B. 扩散

在这里插入图片描述
解法一：（比较慢但是容易想）

$b f s$ ，先将给出的点加入队列中，并且加到集合里。记录扩散的步数，初始化为2020，然后对队列中的每一个同一层的节点在四个方向上扩散，当扩散的点不在集合里时，把它加到队列里，并加到集合里。每扩散一层就减1，当步数为0时，就结束 $b f s$ ，输出答案。

这种做法比较慢，当时跑了好几分钟，不过填空题也无关紧要了。

代码：

package L11;

import java.io.OutputStreamWriter;
import java.io.PrintWriter;
import java.util.HashSet;
import java.util.LinkedList;
import java.util.Objects;
import java.util.Queue;

public class B {
	
	static final int N = 10000;
	static long ans = 0;
	static int[] dx = {-1, 0, 1, 0}, dy = {0, 1, 0, -1};
	static HashSet<Point> set = new HashSet<Point>();
	static Queue<Point> queue = new LinkedList<Point>();
	static PrintWriter out = new PrintWriter(new OutputStreamWriter(System.out));
	
	public static void bfs() {
		int count = 2020;
		while (!queue.isEmpty()) {
			for (int i = queue.size(); i >= 1; i--) {
				Point p = queue.poll();
				int x = p.x, y = p.y;
				for (int u = 0; u < 4; u++) {
					int a = x + dx[u], b = y + dy[u];
					if (set.contains(new Point(a, b))) continue;
					set.add(new Point(a, b));
					queue.add(new Point(a, b));
				}
			}
			
			count--;
			System.out.println(count);
			if (count == 0) break;
		}
	}
	

	public static void main(String[] args) {
		// TODO Auto-generated method stub
		queue.add(new Point(0, 0));
		queue.add(new Point(2020, 11));
		queue.add(new Point(11, 14));
		queue.add(new Point(2000, 2000));
		
		set.add(new Point(0, 0));
		set.add(new Point(2020, 11));
		set.add(new Point(11, 14));
		set.add(new Point(2000, 2000));

		bfs();
		System.out.println(set.size());
		
	}
	
	static class Point {
		int x, y;
		Point(int x, int y) {
			this.x = x;
			this.y = y;
		}
		@Override
		public int hashCode() {
			return Objects.hash(x, y);
		}
		@Override
		public boolean equals(Object obj) {
			if (this == obj)
				return true;
			if (obj == null)
				return false;
			if (getClass() != obj.getClass())
				return false;
			Point other = (Point) obj;
			return x == other.x && y == other.y;
		}
		
	}

}

解法2：

统计在扩散范围内的点与那几个点距离小于2020的个数。

代码：

package L11;

public class B_2 {

	public static void main(String[] args) {
		// TODO Auto-generated method stub

		int ans = 0;
		for (int i = 0 - 2020; i <= 2020 + 2020; i++) 
			for (int j = 0 - 2020; j <= 2020 + 2020; j++) {
				if (Math.abs(i - 0) + Math.abs(j - 0) <= 2020 || Math.abs(i - 2020) + Math.abs(j - 11) <= 2020 || 
						Math.abs(i - 11) + Math.abs(j - 14) <= 2020 || Math.abs(i - 2000) + Math.abs(j - 2000) <= 2020)
					ans++;
			}
		
		System.out.println(ans);
	}

}

C. 阶乘约数

在这里插入图片描述

思路：

复习了一下试除法求质约数个数与约数个数。

给定一个数 $N$ ， $p_i$ 为 $N$ 的质约数，则可以表示为:
$N=p_{1}^{\alpha_1}\times p_{2}^{\alpha_2} \times p_{3}^{\alpha_3}\times... \times p_{k}^{\alpha_k}$
$N$ 的每一个约数 $d_i$ ，可以表示为:
$d_i=p_{1}^{\beta_1}\times p_{2}^{\beta_2} \times p_{3}^{\beta_3}\times... \times p_{k}^{\beta_k}$
其中， $\le \beta_i \le \alpha_i$ ， $\beta_i$ 的选法有 $\sim \alpha_i$ ，共有 $(\alpha_i + 1)$ 种选法，根据排列组合原理，约数个数为：
$(\alpha_1 + 1) \times (\alpha_2 + 1) \times (\alpha_3 + 1) \times ... \times (\alpha_k + 1)$

求 $\sim 100$ 的质因数以及每个质因数的个数，每个质因数的个数加一相乘即可，答案为 $\displaystyle\prod^{k}_{1}{(\alpha_i + 1)}$ 。

代码：

public class Main {
    
    static int[] p = new int[110];

    public static void main(String[] args) {
        // TODO Auto-generated method stub
        for (int i = 2; i <= 100; i++) {
            int x = i;
            for (int j = 2; j <= i / j; j++) {
                if (x % j == 0) {
                    while (x % j == 0) {
                        x /= j;
                        p[j]++;
                    }
                }
            }
            if (x > 1) p[x]++;
        }
        
        long ans = 1;
        for (int i = 2; i < 100; i++) ans *= (p[i] + 1);
        System.out.println(ans);
    }

}

D. 本质上升序列

在这里插入图片描述
解法1：

暴力枚举。因为本质上升序列最长为26，所有的序列个数为 $2^{26}$ ，可以二进制枚举所有的序列，判断在字符串中是否存在该序列，如果存在答案就加一，时间复杂度为 $O(2^{26} × 200)$ 。

代码：

public class D {

	public static void main(String[] args) {
		// TODO Auto-generated method stub
		int ans = 0;
		//String s = "lanqiao";
		String s = "tocyjkdzcieoiodfpbgcncsrjbhmugdnojjddhllnofawllbhfiadgdcdjstemphmnjihecoapdjjrprrqnhgccevdarufmliqijgihhfgdcmxvicfauachlifhafpdccfseflcdgjncadfclvfmadvrnaaahahndsikzssoywakgnfjjaihtniptwoulxbaeqkqhfwl";
		for (int i = 1; i < (1 << 26); i++) {
			StringBuffer sb = new StringBuffer();
 			for (int j = 0; j < 26; j++) {
				if (((i >> j) & 1) == 1)
					sb.append((char)('a' + j));
			}
 			int j = 0;
 			for (int k = 0; k < s.length(); k++) {
 				if (s.charAt(k) == sb.charAt(j)) {
 					j++;
 					if (j == sb.length()) {
 	 					ans++;
 	 					break;
 	 				}
 				}
 				
 			}
		}
		System.out.println(ans);
	}
}

解法2：

动态规划，分析如下，时间复杂度为 $O (N)$
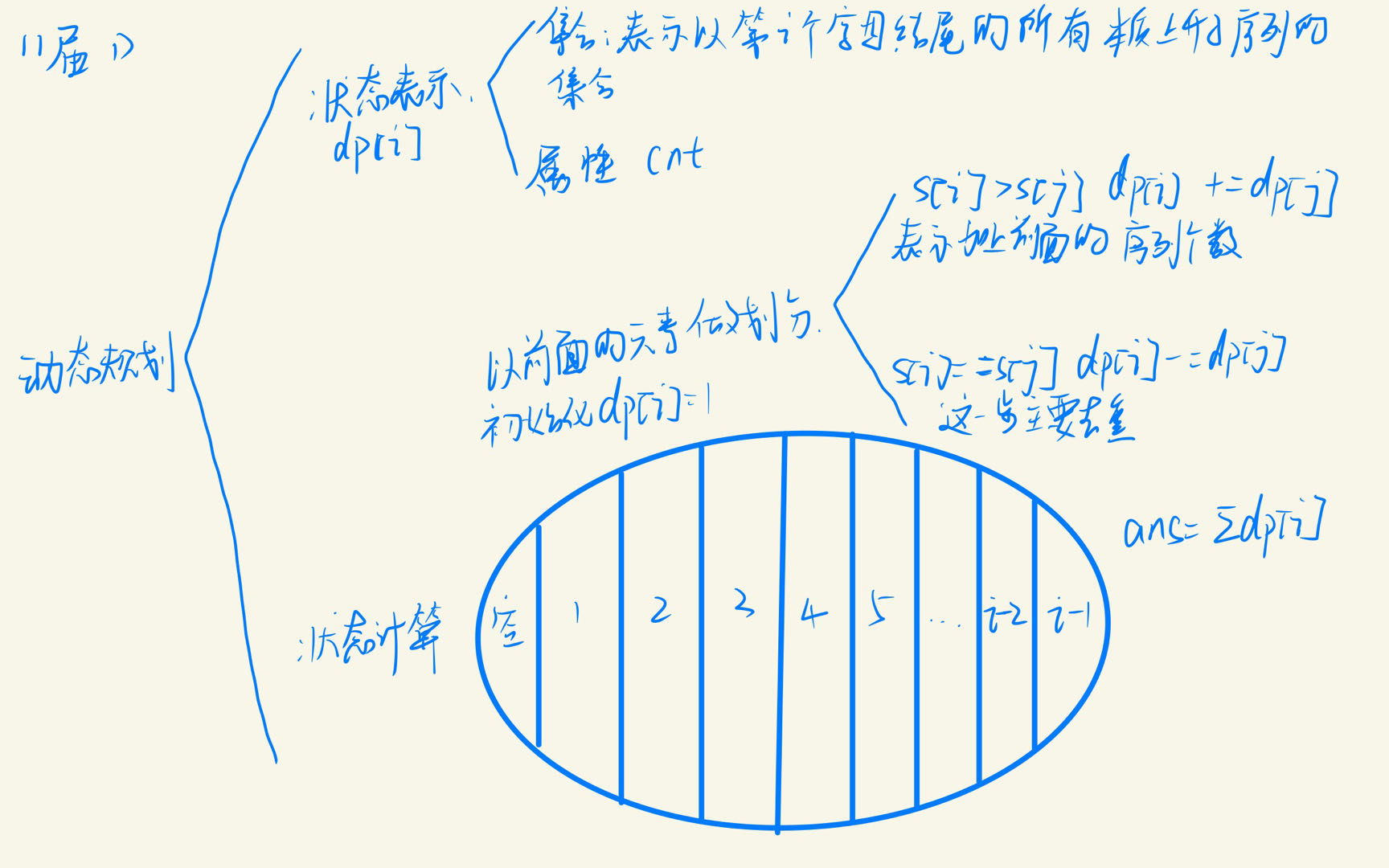
在这里插入图片描述

代码：

public class Main {
	
	static int[] dp = new int[200];

	public static void main(String[] args) {
		// TODO Auto-generated method stub
		String s = "tocyjkdzcieoiodfpbgcncsrjbhmugdnojjddhllnofawllbhfiadgdcdjstemphmnjihecoapdjjrprrqnhgccevdarufmliqijgihhfgdcmxvicfauachlifhafpdccfseflcdgjncadfclvfmadvrnaaahahndsikzssoywakgnfjjaihtniptwoulxbaeqkqhfwl";
		char[] str = s.toCharArray();
		for (int i = 0; i < 200; i++) {
			dp[i] = 1;
			for (int j = 0; j < i; j++) {
				if (str[i] > str[j]) dp[i] += dp[j];
				if (str[i] == str[j]) dp[i] -= dp[j];
			}
		}
		
		long ans = 0;
		for (int i = 0; i < 200; i++) ans += dp[i];
		System.out.println(ans);
	}
}

E. 玩具蛇

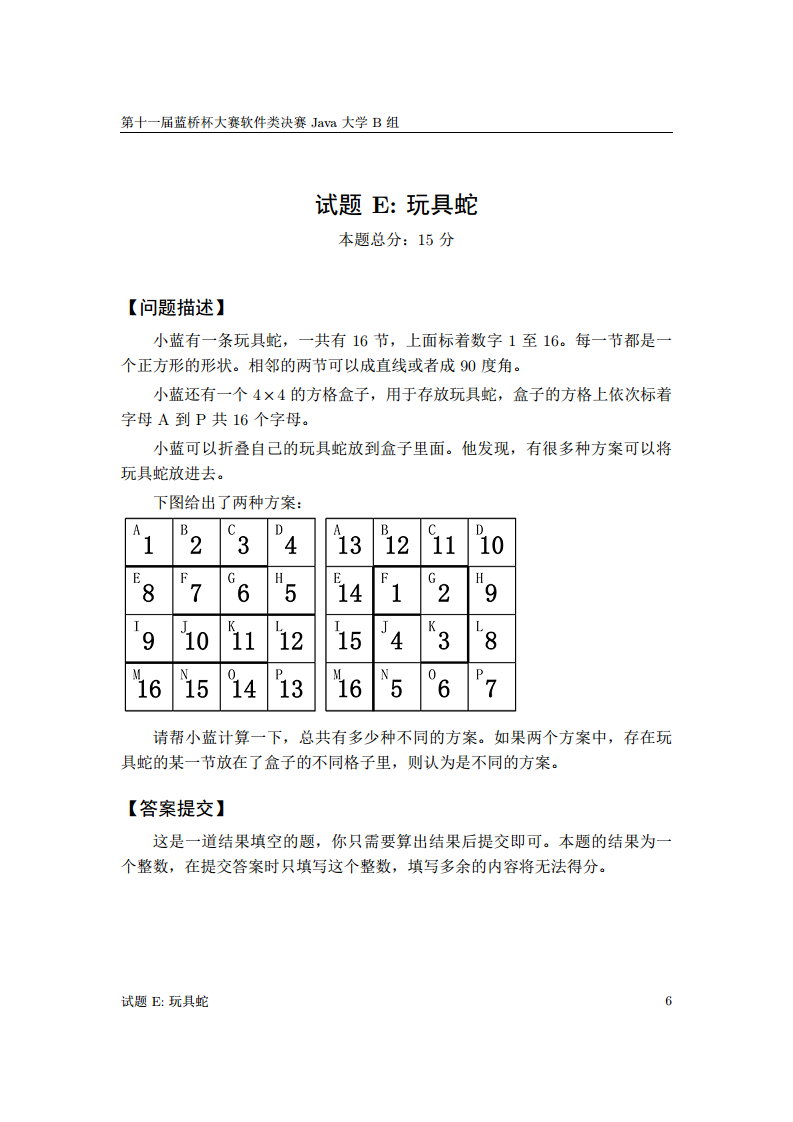
在这里插入图片描述
思路：

从 $\times 4$ 的小方格中每一个位置放置 $1$ , 接着按上下左右 4 个方向 $df s$ 。在搜索的过程中记得回溯，因为求的是方案的个数。枚举每一个位置都要先标记起点，搜索之后再删除标记。

代码：

public class Main {
	
	static int ans;
	static int[] dx = {-1, 0, 1, 0}, dy = {0, 1, 0, -1};
	static boolean[][] st = new boolean[4][4];
	
	public static void dfs(int x, int y, int ch) {
		if (ch == 16) {
			ans++;
			return;
		}
		for (int i = 0; i < 4; i++) {
			int a = x + dx[i], b = y + dy[i];
			if (a < 0 || a >= 4 || b < 0 || b >= 4) continue;
			if (st[a][b]) continue;
			
			st[a][b] = true;
			dfs(a, b, ch + 1);
			st[a][b] = false;
		}
	}

	public static void main(String[] args) {
		// TODO Auto-generated method stub
		for (int i = 0; i < 4; i++) 
			for (int j = 0; j < 4; j++) {
				st[i][j] = true;
				dfs(i, j, 1);
				st[i][j] = false;
			}
				
		System.out.println(ans);
	}

}