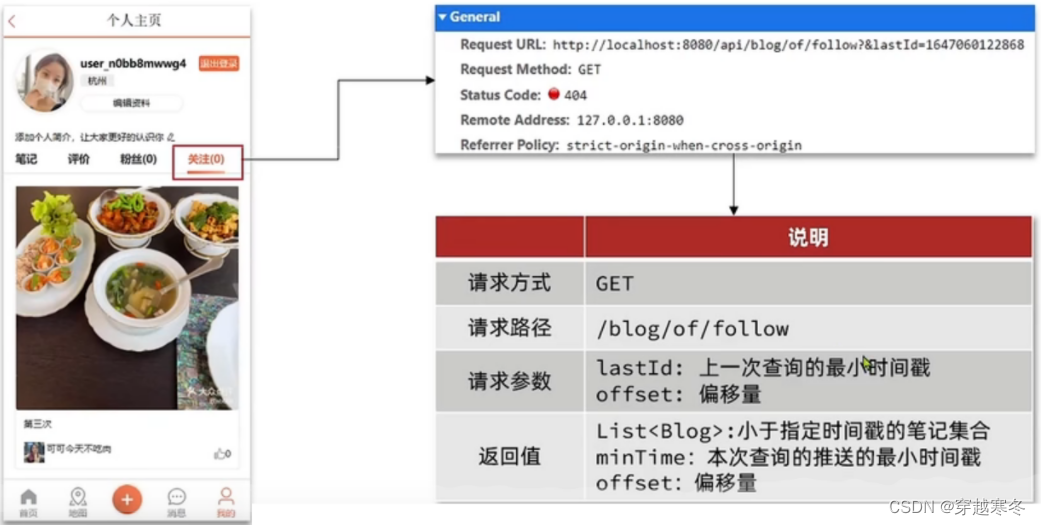
Problem - D - Codeforces

思路：

1. 注意到bi+bj<=2*n，所以ai*aj<=2*n，即我们实际只需要枚举个a的匹配即可
2. 为了不重不漏，我们可以枚举x从1到，寻找所有与x匹配且大于等于x的[a,b]对
3. 这样复杂度就是

#include <bits/stdc++.h>
using namespace std;
#define ll               long long
#define endl             "\n"
typedef pair<int, int> pii;
const int N = 2e5 + 10;
void mysolve()
{
	int n;
	cin>>n;
	vector<pii>a(n);
	for(int i=0; i<n; ++i)cin>>a[i].first;
	for(int i=0; i<n; ++i)cin>>a[i].second;
	sort(a.begin(),a.end());
	ll ans=0;
	for(int x=1; x*x<=2*n; ++x)
		{
			vector<int>cnt(n+1);//枚举x，x只与大于大于他的a匹配，显然在遇到pip前，如果真的存在a=x，我们可以存入每个x=a对应的b
			for(auto [k,v]:a)
				{
					int b=k*x-v;
					if(b>0&&b<=n)///b符合范围
						{
							ans+=cnt[b];
						}
					if(k==x)cnt[v]++;//在遇到a匹配前，先计入a=x的各个b的数目
				}
		}
	cout<<ans<<endl;
}

int32_t main()
{
	std::ios::sync_with_stdio(0), cin.tie(0), cout.tie(0);//使用read请把解绑注释了
	int t=1;
	cin >> t;
	//read(t);
	while (t--)
		{
			mysolve();
		}
	system("pause");
	return 0;
}

Problem - E - Codeforces

思路：

1. 设左括号为1，右括号为-1
2. 如果一个括号序列是合法的，必须满足所以前缀都是>=0的,而且所有后缀都是<=0的
3. 要求[l,r]是合法序列，如果没有[l,r]之间的区间交，显然答案就是被割裂开的每个偶数段（存在奇数段就是无解）的卡特兰数乘积
4. 如果[l1,r1]与[l2,r2]相交，观察出[l2,r1]也必须是合法括号序列，因为[l2,r1]同时满足第一个的后缀和<=0，又同时满足第二个区间的前缀和>=0，所以它同样是合法括号序列
5. 那么存在相交，相当于在割裂出一段，变为[l1,l2],[l2,r1],[r1,r2]都要求是合法序列
6. 所以最后答案就是所有割裂出的合法序列乘积
7. 一个区间被操作多次最后属于哪个区间，我们可以使用异或的思想，显然，如果几个数异或值相同，他们属于一个区间。
8. 为了避免异或出现冲突，我们可以在unsigned long long 的范围内取随机数取异或。操作进行差分即可。

#include <bits/stdc++.h>
using namespace std;
#define ll               long long
#define endl             "\n"
#define inf 0x3f3f3f3f
#define INF 0x3f3f3f3f3f3f3f3f
#define eps 1e-9
#define int              long long
typedef unsigned long long ull;
typedef pair<int, int> pii;
inline int read(int &x);
//double 型memset最大127，最小128
//---------------------------------------------------------------------------------------------------------------------//
//---------------------------------------------------------------------------------------------------------------------//
const int N = 3e5 + 10;
const int mod = 998244353;

ll f[N];

ll fastmi(ll base,ll power)
{
	ll ans=1;
	while(power)
		{
			if(power&1)ans=ans*base%mod;
			power>>=1,base=base*base%mod;
		}
	return ans;
}
void mysolve()
{
	mt19937_64 rnd(random_device{}());
	uniform_int_distribution<ull> dist(0, ULLONG_MAX);
	int n,k;
	cin>>n>>k;
	vector<int>b(n+5);
	int l,r;
	for(int i=1; i<=k; ++i)
		{
			cin>>l>>r;
			ull h=dist(rnd);
			b[l]^=h,b[r+1]^=h;
		}
	if(n&1)
		{
			cout<<0<<endl;
			return;
		}
	map<ull,int>mp;
	for(int i=1; i<=n; ++i)
		{
			b[i]^=b[i-1];
			mp[b[i]]++;
		}
	int ans=1;
	for(auto [k,v]:mp)
		{
			if(v&1)//要求被割裂的每个段都是偶数长度
				{
					cout<<0<<endl;
					return;
				}
			ans=ans*f[v/2]%mod;
		}
	cout<<ans<<endl;
}

int32_t main()
{
	std::ios::sync_with_stdio(0), cin.tie(0), cout.tie(0);//使用read请把解绑注释了
	int t=1;
	cin >> t;
	//read(t);
	f[0]=1;
	for(int i=1; i<=3e5; ++i)
		f[i]=f[i-1]*(4*i-2)%mod*fastmi(i+1,mod-2)%mod;//预处理卡特兰数
	while (t--)
		{
			mysolve();
		}
	system("pause");
	return 0;
}

inline int read(int &x)
{
	x = 0;
	char ch = 0;
	while (ch < '0' || ch > '9')ch = getchar();
	while (ch >= '0' && ch <= '9')
		{
			x = x * 10 + ch - '0';
			ch = getchar();
		}
	return x;
}