简介

SUNTANS中 tidal forcing 算例的全称是 Example tidal forcing in Monterey Bay。该算例模拟了 Monterey Bay 地区在潮汐作用下的水流；但由于模型网格的水平分辨率较粗，该模型只能模拟正压流动，而无法模拟该区域的内波。通过该算例，我们可以学习SUNTANS模型中tide模块的应用及潮汐边界条件的设置。
该算例的网格和参数文件位于 /examples/tides，其中还包含一个简单的说明文件 README。

网格配置与地形

本例采用一个三维网格。从水平面上看，计算域如下图所示，其网格均为三角形。从垂向看，网格被分为了10层（suntans.dat： Nkmax=10, rstretch=1）。
注意，下图所示网格仅是输入时候的网格；在模型中，网格的 Voronoi 点需要被校正，之后才会进行模拟。当要对网格的 Voronoi 点进行校正时，suntans.dat 文件中的 CorrectVoronoi 要被设置成 -1，校正参数 VoronoiRatio 需要被指定。在本算例中， VoronoiRatio = 85，即对于任何最大角大于85°的三角形网格，其 Voronoi 点需要被校正。
在这里插入图片描述
注意，上图中不同颜色的计算域边界线表示了不同类型的边界。其中，紫红色线的编号为1（marker=1），表示陆地边界（闭边界）；绿色线的编号为2（marker=2），表示受潮汐作用的开边界；

计算域的地形数据存储在 /examples/tides/rundata/mbay_bathy.dat 文件中。在运行SUNTANS时，该文件中的数据将被插值到每个网格的 Voronoi 点上。因为这个过程可能会花费相当长的时间，所以最好只在必要时运行它，并在之后的运行中使用预插值的水深测量（/examples/tides/rundata/mbay_bathy-voro）。
如果在模型运行过程中进行了地形数据插值，一个名为 mbay_bathy.dat-voro 将会被输出，并出现在结果文件夹 /examples/tides/data 中。是否进行地形插值计算可通过设置输入文件中 suntans.dat 来实现。当 suntans.dat 中的 IntDepth 被设置为1时，插值过程将会进行，即模型将在 rundata 文件夹中读取 mbay_bathy.dat 文件，并输出插值后的数据文件 mbay_bathy.dat-voro；若 IntDepth 被设置为2，模型则会直接读取 mbay_bathy.dat-voro 的数据，即不进行插值计算。由于自带算例文件中已经包含 mbay_bathy.dat-voro 文件，故本算例的 IntDepth 被设置为2。
请注意，若Voronoi点发生改变，地形数据都应该被重新插值。

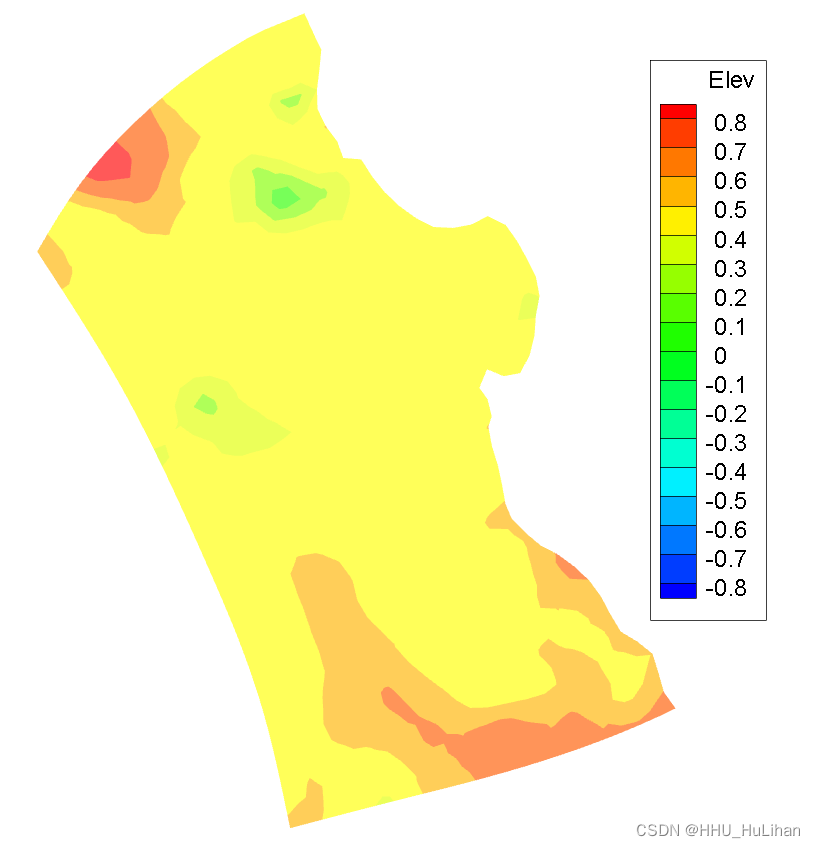
插值后的静水深如下图所示。
在这里插入图片描述

定解条件设置

初始条件设置

在本算例中，初始的水位和流速值需要设定。初始条件的相关设置参见 initialization.c 文件。

函数GetDZ：对本算例，此函数不重要，略作介绍
该函数用于确定垂向网格。对于本算例，rstretch=1，所以垂向网格通过水面、最深点和垂向最大网格数推算得出。
函数ReturnDepth：对本算例，此函数并没有被调用，因为仅当IntDepth=0时此函数才被调用；此处不讲解
函数ReturnFreeSurface（重要）：用于给定水位的初始条件。
对于本算例，初始水位为0；故设置如下：
```
REAL ReturnFreeSurface(REAL x, REAL y, REAL d) {
	return 0.0;
}
```
函数ReturnSalinity和ReturnTemperature：用于确定初始时刻的盐度/温度场（不重要）
由于state.c中的设置：
```
REAL StateEquation(const propT *prop, const REAL s, const REAL T, const REAL p) {
	return prop->beta*s;
}
```
水体密度仅和水体盐度相关。而系数beta=0（suntans.dat中设定），故可认为本例中盐度和温度设置对流动过程没有影响。

函数ReturnHorizontalVelocity：设定了初始时的水平流速场

REAL ReturnHorizontalVelocity(REAL x, REAL y, REAL n1, REAL n2, REAL z) {
	return 0.0;
}

边界条件设置

在本算例中，需要设定的边界条件有两部分.一是陆地边界（marker=1），二是受潮汐驱动的开边界（marker=2）。相关设置都在 initialization.c 文件中。

开边界处的通量计算（OpenBoundaryFluxes）

void OpenBoundaryFluxes(REAL **q, REAL **ub, REAL **ubn, gridT *grid, physT *phys, propT *prop) {
  int j, jptr, ib, k, forced;
  REAL **uc = phys->uc, **vc = phys->vc, **ucold = phys->uold, **vcold = phys->vold;
  REAL z, c0, c1, C0, C1, dt=prop->dt, u0, u0new, uc0, vc0, uc0old, vc0old, ub0;

  for(jptr=grid->edgedist[2];jptr<grid->edgedist[3];jptr++) {
    j = grid->edgep[jptr];

    ib = grid->grad[2*j];

    for(k=grid->etop[j];k<grid->Nke[j];k++) {    
      ub[j][k] = phys->boundary_u[jptr-grid->edgedist[2]][k]*grid->n1[j] 
    	+ phys->boundary_v[jptr-grid->edgedist[2]][k]*grid->n2[j]; 
    }
  }
}

上面的代码指定了，在所有marker=2的边上（grid->edgedist[2]），速度ub为是 (boundary_u, boundary_v) 在边界法相量 (n₁, n₂)上的投影：

      ub[j][k] = phys->boundary_u[jptr-grid->edgedist[2]][k]*grid->n1[j] 
    	+ phys->boundary_v[jptr-grid->edgedist[2]][k]*grid->n2[j];

(boundary_u, boundary_v) 将在函数BoundaryVelocities中设定。

开边处的速度、水位（BoundaryVelocities）

void BoundaryVelocities(gridT *grid, physT *phys, propT *prop, int myproc, MPI_Comm comm) {
  int i, j, jind, iptr, jptr, n, k;
  REAL h, u, v, toffSet, secondsPerDay = 86400.0;

  if(prop->n==prop->nstart+1) 
    SetTideComponents(grid,myproc);

  // Tidal data is from the start of a particular year, so an offset 
  // needs to be used to start the simulation on a particular date.
  // Note that the offset time is in days, and must be converted to seconds
  // using the secondsPerDay variable.
  toffSet = MPI_GetValue(DATAFILE,"toffSet","BoundaryVelocities",myproc)*secondsPerDay;

  for(jptr=grid->edgedist[2];jptr<grid->edgedist[3];jptr++) {
    jind = jptr-grid->edgedist[2];
    j = grid->edgep[jptr];

    u=v=h=0;
    for(n=0;n<numtides;n++) {
      h = h + h_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + h_phase[jind][n]);
      u = u + u_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + u_phase[jind][n]);
      v = v + v_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + v_phase[jind][n]);
    }

    // Velocities from tides.c are in cm/s and h is in cm!
    phys->boundary_h[jind]=h*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
    for(k=grid->etop[j];k<grid->Nke[j];k++) {
      phys->boundary_u[jind][k]=u*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
      phys->boundary_v[jind][k]=v*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
      phys->boundary_w[jind][k]=0;
    }
  }
  for(iptr=grid->celldist[1];iptr<grid->celldist[2];iptr++) {
    jind = iptr-grid->celldist[1]+grid->edgedist[3]-grid->edgedist[2];
    i = grid->cellp[iptr];

    u=v=h=0;
    for(n=0;n<numtides;n++) {
      h = h + h_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + h_phase[jind][n]);
      u = u + u_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + u_phase[jind][n]);
      v = v + v_amp[jind][n]*cos(omegas[n]*(toffSet+prop->rtime) + v_phase[jind][n]);
    }

    // Velocities from tides.c are in cm/s and h is in cm!
    phys->h[i]=h*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
    for(k=grid->ctop[i];k<grid->Nk[i];k++) {
      phys->uc[i][k]=u*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
      phys->vc[i][k]=v*(1-exp(-prop->rtime/prop->thetaramptime))/100.0;
      phys->w[i][k]=0;
    }
  }
}

首先，调用tide.c中的SetTideComponents函数。该函数会读取输入文件夹中的潮汐参数文件，该参数文件的名字在suntans.dat中被指定：

TideInput               tidecomponents.dat
TideOutput              tidexy.dat

即潮汐参数文件的文件名为tidecomponents.dat。该数据文件是二进制格式，包含了分潮数 numtides、开边界的edges数 numboundaryedges，以及对应的各个分潮的角频率 omega，水平流速、水位振幅 amp 和相位角 phase。
在这里插入图片描述
此外，BoundaryVelocities还会读取 suntans.dat 中的一个数据 toffSet。这个数据表示模拟起始时间与tidecomponents.dat起始时间的偏移量，单位为天。在本算例中，toffSet=7。

prop->nctime = prop->toffSet*86400.0 + prop->nstart*prop->dt;

随后，第一个循环 for(jptr=grid->edgedist[2];jptr< grid->edgedist[3];jptr++) 中根据不同分潮的参数，求出了水位h和水平流速u、v的值；并用这些值确定了开边界的boundary_u、boundary_v、boundary_w。同时，为了避免潮汐边界条件在脉冲启动时的瞬态振荡，潮汐边界的添加还涉及了“缓坡启动”，设置的启动时间 thetaramptime=86400s （suntans.dat）。

在第二个循环 for(iptr=grid->celldist[1];iptr< grid->celldist[2];iptr++) 中，设定边界处的网格中心的流速uc、vc和w与边界处相等，即 uc = boundary_u、vc = boundary_v、wc = boundary_w。

其它参数配置

该算例采用静压模拟（suntans.dat: nonhydrostatic = 0），时间步长为Δt=90s （suntans.dat: dt = 90），总共运行3000个时间步（suntans.dat: nstep = 13440），并每隔120步输出一次结果（suntans.dat: ntout = 1344）。
水体的分子粘度采用 0.1 m²/s，垂向粘度通过求解MY-2.5紊流模型得到。
动量平流项采用中心差分格式（suntans.dat: nonlinear = 2）。
此外，模拟考虑了科氏力效应，设置科氏力系数Coriolis_f = 8.7e-5。