Problem - E - Codeforces

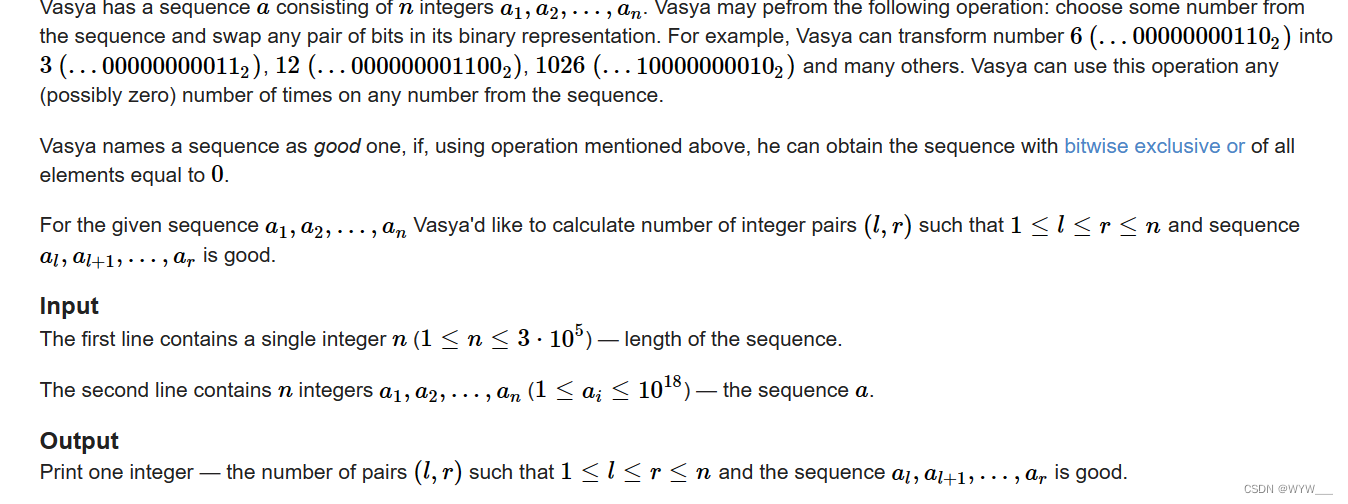
Vasya有一个由n个整数组成的序列a。 Vasya可以执行以下操作：从序列中选择一些数字，并交换其二进制表示中的任意一对位。例如，Vasya可以将数字6（… 000000001102）转换为3（… 000000000112），12（… 0000000011002），1026（… 100000000102）等等。 Vasya可以在任何数字上使用此操作任意（可能为零）次。

如果使用上述操作，Vasya可以获得按位异或所有元素均等于0的序列，则Vasya将序列命名为好序列。

对于给定的序列a1，a2，…，an，Vasya想计算整数对（l，r），使得1≤l≤r≤n且序列al，al + 1，…，ar是好的。

输入 第一行包含一个整数n（1≤n≤3⋅105） - 序列的长度。

第二行包含n个整数a1，a2，…，an（1≤ai≤1018）-序列a。

输出 打印一个整数 - 对于1≤l≤r≤n且序列al，al + 1，…，ar很好，返回配对（l，r）的数量。

Examples

input

Copy

3
6 7 14

output

Copy

2

input

Copy

4
1 2 1 16

output

Copy

4

在第一个示例中，(2,3)和(1,3)是有效的。当a2=7→11，a3=14→11且11 ⊕ 11 = 0时，对(2,3)进行配对是有效的，其中⊕表示按位异或运算。当a1=6→3，a2=7→13，a3=14→14且3 ⊕ 13 ⊕ 14 = 0时，对(1,3)进行配对是有效的。

在第二个示例中，配对(1,2)，(2,3)，(3,4)和(1,4)都是有效的。

题解:

对于这种求好区间有多少的题目,一般都是先枚举左区间,再枚举右区间,同时题目隐藏一些性质,大大减少了枚举的段数

我们可以任意改变一个数中一的位置,如果一段异或和为0,应该满足两个条件

1.1的数目为偶数

2.1的数目大于等于区间内1数目最大的两倍(不太好看出来,题目的关键)

有了这个条件,我们就可以枚举段数了,由于题目说,1<=ai<=1e18,

也就是说每个位置1的最小数目为1,最大数目大概为64,

如果一个区间长度为64,最小1的数目也有64个,肯定大于等于区间内1数目最大的两倍,也就是说每个i顶多往后数64个区间即可,不会t

枚举区间时,符合条件的++,对于超过最大限制区间的第二个条件肯定满足,我们只需通过后缀和,来看,后面多少加上后奇偶性满足即可 

#include <cstdio>
#include <cstring>
#include <algorithm>
#include<iostream>
#include<vector>
#include<set>
#include<map>
#include<cmath>
#include<queue>
using namespace std;
typedef long long ll;
#define int long long
typedef pair<int,int> PII;
int mod = 1e9 + 7;
vector<int> p[200050];
int a[300050];
int c[300050];
int s[300050];
void solve()
{
	int n;
	cin >> n;
	for(int i = 1;i <= n;i++)
	{
		int x;
		cin >> x;
		while(x)
		{
			if(x%2)
			a[i]++;
			x /= 2;
		}
	}
	for(int i = 1;i <= n;i++)
	{
		s[i] = s[i - 1] + a[i];
	}
	for(int i = n;i >= 1;i --)
	{
		c[i] = c[i + 1];
		if(s[i]%2 == 0)
		{
			c[i]++;
		}
	}
	int ans = 0;
	for(int i = 1;i <= n;i++)
	{
		int ma = a[i];
		int sum = a[i];
		for(int j = i + 1;j <= n;j++)
		{
			sum += a[j];
			ma = max(ma,a[j]);
			if(sum >= 2*ma&&sum%2 == 0)
			{
				ans++;
			}
			if(sum > 128)
			{
				if(sum%2 == 0)
				{
					if(s[j]%2 == 0)
					{
						ans += c[j + 1];
					}
					else
					{
						ans += n - j - c[j + 1];
					}
				}
				else
				{
					if(s[j]%2 == 0)
					{
						ans += n - j - c[j + 1];
					}
					else
					{
						ans += c[j + 1];
					}
				}
				break;
			}
		}
	}
	cout << ans;
}

signed main()
{
//	ios::sync_with_stdio(0 );
//	cin.tie(0);cout.tie(0);
	int t = 1;
//	cin >> t;
	while(t--)
	{
		solve(); 
	}
}