算法思想 - 回溯算法

news2024/11/30 1:34:52
Backtracking(回溯)属于 DFS, 本文主要介绍算法中Backtracking算法的思想。回溯算法实际上一个类似枚举的搜索尝试过程，主要是在搜索尝试过程中寻找问题的解，当发现已不满足求解条件时，就“回溯”返回，尝试别的路径。回溯法是一种选优搜索法，按选优条件向前搜索，以达到目标。但当探索到某一步时，发现原先选择并不优或达不到目标，就退回一步重新选择，这种走不通就退回再走的技术为回溯法。

Backtracking

  • 普通 DFS 主要用在 可达性问题 ，这种问题只需要执行到特点的位置然后返回即可。

  • 而 Backtracking 主要用于求解 排列组合 问题，例如有 { 'a','b','c' } 三个字符，求解所有由这三个字符排列得到的字符串，这种问题在执行到特定的位置返回之后还会继续执行求解过程。

因为 Backtracking 不是立即就返回，而要继续求解，因此在程序实现时，需要注意对元素的标记问题:

  • 在访问一个新元素进入新的递归调用时，需要将新元素标记为已经访问，这样才能在继续递归调用时不用重复访问该元素；

  • 但是在递归返回时，需要将元素标记为未访问，因为只需要保证在一个递归链中不同时访问一个元素，可以访问已经访问过但是不在当前递归链中的元素。

数字键盘组合

17. Letter Combinations of a Phone Number (Medium)

Input:Digit string "23"
Output: ["ad", "ae", "af", "bd", "be", "bf", "cd", "ce", "cf"].
private static final String[] KEYS = {"", "", "abc", "def", "ghi", "jkl", "mno", "pqrs", "tuv", "wxyz"};

public List<String> letterCombinations(String digits) {
    List<String> combinations = new ArrayList<>();
    if (digits == null || digits.length() == 0) {
        return combinations;
    }
    doCombination(new StringBuilder(), combinations, digits);
    return combinations;
}

private void doCombination(StringBuilder prefix, List<String> combinations, final String digits) {
    if (prefix.length() == digits.length()) {
        combinations.add(prefix.toString());
        return;
    }
    int curDigits = digits.charAt(prefix.length()) - '0';
    String letters = KEYS[curDigits];
    for (char c : letters.toCharArray()) {
        prefix.append(c);                         // 添加
        doCombination(prefix, combinations, digits);
        prefix.deleteCharAt(prefix.length() - 1); // 删除
    }
}

IP 地址划分

93. Restore IP Addresses(Medium)

Given "25525511135",
return ["255.255.11.135", "255.255.111.35"].
public List<String> restoreIpAddresses(String s) {
    List<String> addresses = new ArrayList<>();
    StringBuilder tempAddress = new StringBuilder();
    doRestore(0, tempAddress, addresses, s);
    return addresses;
}

private void doRestore(int k, StringBuilder tempAddress, List<String> addresses, String s) {
    if (k == 4 || s.length() == 0) {
        if (k == 4 && s.length() == 0) {
            addresses.add(tempAddress.toString());
        }
        return;
    }
    for (int i = 0; i < s.length() && i <= 2; i++) {
        if (i != 0 && s.charAt(0) == '0') {
            break;
        }
        String part = s.substring(0, i + 1);
        if (Integer.valueOf(part) <= 255) {
            if (tempAddress.length() != 0) {
                part = "." + part;
            }
            tempAddress.append(part);
            doRestore(k + 1, tempAddress, addresses, s.substring(i + 1));
            tempAddress.delete(tempAddress.length() - part.length(), tempAddress.length());
        }
    }
}

在矩阵中寻找字符串

79. Word Search (Medium)

For example,
Given board =
[
  ['A','B','C','E'],
  ['S','F','C','S'],
  ['A','D','E','E']
]
word = "ABCCED", -> returns true,
word = "SEE", -> returns true,
word = "ABCB", -> returns false.
private final static int[][] direction = {{1, 0}, {-1, 0}, {0, 1}, {0, -1}};
private int m;
private int n;

public boolean exist(char[][] board, String word) {
    if (word == null || word.length() == 0) {
        return true;
    }
    if (board == null || board.length == 0 || board[0].length == 0) {
        return false;
    }

    m = board.length;
    n = board[0].length;
    boolean[][] hasVisited = new boolean[m][n];

    for (int r = 0; r < m; r++) {
        for (int c = 0; c < n; c++) {
            if (backtracking(0, r, c, hasVisited, board, word)) {
                return true;
            }
        }
    }

    return false;
}

private boolean backtracking(int curLen, int r, int c, boolean[][] visited, final char[][] board, final String word) {
    if (curLen == word.length()) {
        return true;
    }
    if (r < 0 || r >= m || c < 0 || c >= n
            || board[r][c] != word.charAt(curLen) || visited[r][c]) {

        return false;
    }

    visited[r][c] = true;

    for (int[] d : direction) {
        if (backtracking(curLen + 1, r + d[0], c + d[1], visited, board, word)) {
            return true;
        }
    }

    visited[r][c] = false;

    return false;
}

输出二叉树中所有从根到叶子的路径

257. Binary Tree Paths (Easy)

  1
 /  \
2    3
 \
  5
["1->2->5", "1->3"]
public List<String> binaryTreePaths(TreeNode root) {
    List<String> paths = new ArrayList<>();
    if (root == null) {
        return paths;
    }
    List<Integer> values = new ArrayList<>();
    backtracking(root, values, paths);
    return paths;
}

private void backtracking(TreeNode node, List<Integer> values, List<String> paths) {
    if (node == null) {
        return;
    }
    values.add(node.val);
    if (isLeaf(node)) {
        paths.add(buildPath(values));
    } else {
        backtracking(node.left, values, paths);
        backtracking(node.right, values, paths);
    }
    values.remove(values.size() - 1);
}

private boolean isLeaf(TreeNode node) {
    return node.left == null && node.right == null;
}

private String buildPath(List<Integer> values) {
    StringBuilder str = new StringBuilder();
    for (int i = 0; i < values.size(); i++) {
        str.append(values.get(i));
        if (i != values.size() - 1) {
            str.append("->");
        }
    }
    return str.toString();
}

排列

46. Permutations (Medium)

[1,2,3] have the following permutations:
[
  [1,2,3],
  [1,3,2],
  [2,1,3],
  [2,3,1],
  [3,1,2],
  [3,2,1]
]
public List<List<Integer>> permute(int[] nums) {
    List<List<Integer>> permutes = new ArrayList<>();
    List<Integer> permuteList = new ArrayList<>();
    boolean[] hasVisited = new boolean[nums.length];
    backtracking(permuteList, permutes, hasVisited, nums);
    return permutes;
}

private void backtracking(List<Integer> permuteList, List<List<Integer>> permutes, boolean[] visited, final int[] nums) {
    if (permuteList.size() == nums.length) {
        permutes.add(new ArrayList<>(permuteList)); // 重新构造一个 List
        return;
    }
    for (int i = 0; i < visited.length; i++) {
        if (visited[i]) {
            continue;
        }
        visited[i] = true;
        permuteList.add(nums[i]);
        backtracking(permuteList, permutes, visited, nums);
        permuteList.remove(permuteList.size() - 1);
        visited[i] = false;
    }
}

含有相同元素求排列

47. Permutations II (Medium)

[1,1,2] have the following unique permutations:
[[1,1,2], [1,2,1], [2,1,1]]

数组元素可能含有相同的元素，进行排列时就有可能出现重复的排列，要求重复的排列只返回一个。

在实现上，和 Permutations 不同的是要先排序，然后在添加一个元素时，判断这个元素是否等于前一个元素，如果等于，并且前一个元素还未访问，那么就跳过这个元素。

public List<List<Integer>> permuteUnique(int[] nums) {
    List<List<Integer>> permutes = new ArrayList<>();
    List<Integer> permuteList = new ArrayList<>();
    Arrays.sort(nums);  // 排序
    boolean[] hasVisited = new boolean[nums.length];
    backtracking(permuteList, permutes, hasVisited, nums);
    return permutes;
}

private void backtracking(List<Integer> permuteList, List<List<Integer>> permutes, boolean[] visited, final int[] nums) {
    if (permuteList.size() == nums.length) {
        permutes.add(new ArrayList<>(permuteList));
        return;
    }

    for (int i = 0; i < visited.length; i++) {
        if (i != 0 && nums[i] == nums[i - 1] && !visited[i - 1]) {
            continue;  // 防止重复
        }
        if (visited[i]){
            continue;
        }
        visited[i] = true;
        permuteList.add(nums[i]);
        backtracking(permuteList, permutes, visited, nums);
        permuteList.remove(permuteList.size() - 1);
        visited[i] = false;
    }
}

组合

77. Combinations (Medium)

If n = 4 and k = 2, a solution is:
[
  [2,4],
  [3,4],
  [2,3],
  [1,2],
  [1,3],
  [1,4],
]
public List<List<Integer>> combine(int n, int k) {
    List<List<Integer>> combinations = new ArrayList<>();
    List<Integer> combineList = new ArrayList<>();
    backtracking(combineList, combinations, 1, k, n);
    return combinations;
}

private void backtracking(List<Integer> combineList, List<List<Integer>> combinations, int start, int k, final int n) {
    if (k == 0) {
        combinations.add(new ArrayList<>(combineList));
        return;
    }
    for (int i = start; i <= n - k + 1; i++) {  // 剪枝
        combineList.add(i);
        backtracking(combineList, combinations, i + 1, k - 1, n);
        combineList.remove(combineList.size() - 1);
    }
}

组合求和

39. Combination Sum (Medium)

given candidate set [2, 3, 6, 7] and target 7,
A solution set is:
[[7],[2, 2, 3]]
public List<List<Integer>> combinationSum(int[] candidates, int target) {
    List<List<Integer>> combinations = new ArrayList<>();
    backtracking(new ArrayList<>(), combinations, 0, target, candidates);
    return combinations;
}

private void backtracking(List<Integer> tempCombination, List<List<Integer>> combinations,
                          int start, int target, final int[] candidates) {

    if (target == 0) {
        combinations.add(new ArrayList<>(tempCombination));
        return;
    }
    for (int i = start; i < candidates.length; i++) {
        if (candidates[i] <= target) {
            tempCombination.add(candidates[i]);
            backtracking(tempCombination, combinations, i, target - candidates[i], candidates);
            tempCombination.remove(tempCombination.size() - 1);
        }
    }
}

含有相同元素的求组合求和

40. Combination Sum II (Medium)

For example, given candidate set [10, 1, 2, 7, 6, 1, 5] and target 8,
A solution set is:
[
  [1, 7],
  [1, 2, 5],
  [2, 6],
  [1, 1, 6]
]
public List<List<Integer>> combinationSum2(int[] candidates, int target) {
    List<List<Integer>> combinations = new ArrayList<>();
    Arrays.sort(candidates);
    backtracking(new ArrayList<>(), combinations, new boolean[candidates.length], 0, target, candidates);
    return combinations;
}

private void backtracking(List<Integer> tempCombination, List<List<Integer>> combinations,
                          boolean[] hasVisited, int start, int target, final int[] candidates) {

    if (target == 0) {
        combinations.add(new ArrayList<>(tempCombination));
        return;
    }
    for (int i = start; i < candidates.length; i++) {
        if (i != 0 && candidates[i] == candidates[i - 1] && !hasVisited[i - 1]) {
            continue;
        }
        if (candidates[i] <= target) {
            tempCombination.add(candidates[i]);
            hasVisited[i] = true;
            backtracking(tempCombination, combinations, hasVisited, i + 1, target - candidates[i], candidates);
            hasVisited[i] = false;
            tempCombination.remove(tempCombination.size() - 1);
        }
    }
}

1-9 数字的组合求和

216. Combination Sum III (Medium)

Input: k = 3, n = 9

Output:

[[1,2,6], [1,3,5], [2,3,4]]

从 1-9 数字中选出 k 个数不重复的数，使得它们的和为 n。

public List<List<Integer>> combinationSum3(int k, int n) {
    List<List<Integer>> combinations = new ArrayList<>();
    List<Integer> path = new ArrayList<>();
    backtracking(k, n, 1, path, combinations);
    return combinations;
}

private void backtracking(int k, int n, int start,
                          List<Integer> tempCombination, List<List<Integer>> combinations) {

    if (k == 0 && n == 0) {
        combinations.add(new ArrayList<>(tempCombination));
        return;
    }
    if (k == 0 || n == 0) {
        return;
    }
    for (int i = start; i <= 9; i++) {
        tempCombination.add(i);
        backtracking(k - 1, n - i, i + 1, tempCombination, combinations);
        tempCombination.remove(tempCombination.size() - 1);
    }
}

子集

78. Subsets (Medium)

找出集合的所有子集，子集不能重复，[1, 2] 和 [2, 1] 这种子集算重复

public List<List<Integer>> subsets(int[] nums) {
    List<List<Integer>> subsets = new ArrayList<>();
    List<Integer> tempSubset = new ArrayList<>();
    for (int size = 0; size <= nums.length; size++) {
        backtracking(0, tempSubset, subsets, size, nums); // 不同的子集大小
    }
    return subsets;
}

private void backtracking(int start, List<Integer> tempSubset, List<List<Integer>> subsets,
                          final int size, final int[] nums) {

    if (tempSubset.size() == size) {
        subsets.add(new ArrayList<>(tempSubset));
        return;
    }
    for (int i = start; i < nums.length; i++) {
        tempSubset.add(nums[i]);
        backtracking(i + 1, tempSubset, subsets, size, nums);
        tempSubset.remove(tempSubset.size() - 1);
    }
}

含有相同元素求子集

90. Subsets II (Medium)

For example,
If nums = [1,2,2], a solution is:

[
  [2],
  [1],
  [1,2,2],
  [2,2],
  [1,2],
  []
]
public List<List<Integer>> subsetsWithDup(int[] nums) {
    Arrays.sort(nums);
    List<List<Integer>> subsets = new ArrayList<>();
    List<Integer> tempSubset = new ArrayList<>();
    boolean[] hasVisited = new boolean[nums.length];
    for (int size = 0; size <= nums.length; size++) {
        backtracking(0, tempSubset, subsets, hasVisited, size, nums); // 不同的子集大小
    }
    return subsets;
}

private void backtracking(int start, List<Integer> tempSubset, List<List<Integer>> subsets, boolean[] hasVisited,
                          final int size, final int[] nums) {

    if (tempSubset.size() == size) {
        subsets.add(new ArrayList<>(tempSubset));
        return;
    }
    for (int i = start; i < nums.length; i++) {
        if (i != 0 && nums[i] == nums[i - 1] && !hasVisited[i - 1]) {
            continue;
        }
        tempSubset.add(nums[i]);
        hasVisited[i] = true;
        backtracking(i + 1, tempSubset, subsets, hasVisited, size, nums);
        hasVisited[i] = false;
        tempSubset.remove(tempSubset.size() - 1);
    }
}

分割字符串使得每个部分都是回文数

131. Palindrome Partitioning (Medium)

For example, given s = "aab",
Return

[
  ["aa","b"],
  ["a","a","b"]
]
public List<List<String>> partition(String s) {
    List<List<String>> partitions = new ArrayList<>();
    List<String> tempPartition = new ArrayList<>();
    doPartition(s, partitions, tempPartition);
    return partitions;
}

private void doPartition(String s, List<List<String>> partitions, List<String> tempPartition) {
    if (s.length() == 0) {
        partitions.add(new ArrayList<>(tempPartition));
        return;
    }
    for (int i = 0; i < s.length(); i++) {
        if (isPalindrome(s, 0, i)) {
            tempPartition.add(s.substring(0, i + 1));
            doPartition(s.substring(i + 1), partitions, tempPartition);
            tempPartition.remove(tempPartition.size() - 1);
        }
    }
}

private boolean isPalindrome(String s, int begin, int end) {
    while (begin < end) {
        if (s.charAt(begin++) != s.charAt(end--)) {
            return false;
        }
    }
    return true;
}

数独

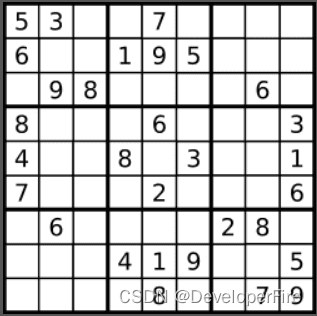
37. Sudoku Solver (Hard)

private boolean[][] rowsUsed = new boolean[9][10];
private boolean[][] colsUsed = new boolean[9][10];
private boolean[][] cubesUsed = new boolean[9][10];
private char[][] board;

public void solveSudoku(char[][] board) {
    this.board = board;
    for (int i = 0; i < 9; i++)
        for (int j = 0; j < 9; j++) {
            if (board[i][j] == '.') {
                continue;
            }
            int num = board[i][j] - '0';
            rowsUsed[i][num] = true;
            colsUsed[j][num] = true;
            cubesUsed[cubeNum(i, j)][num] = true;
        }

    for (int i = 0; i < 9; i++) {
        for (int j = 0; j < 9; j++) {
            backtracking(i, j);
        }
    }
}

private boolean backtracking(int row, int col) {
    while (row < 9 && board[row][col] != '.') {
        row = col == 8 ? row + 1 : row;
        col = col == 8 ? 0 : col + 1;
    }
    if (row == 9) {
        return true;
    }
    for (int num = 1; num <= 9; num++) {
        if (rowsUsed[row][num] || colsUsed[col][num] || cubesUsed[cubeNum(row, col)][num]) {
            continue;
        }
        rowsUsed[row][num] = colsUsed[col][num] = cubesUsed[cubeNum(row, col)][num] = true;
        board[row][col] = (char) (num + '0');
        if (backtracking(row, col)) {
            return true;
        }
        board[row][col] = '.';
        rowsUsed[row][num] = colsUsed[col][num] = cubesUsed[cubeNum(row, col)][num] = false;
    }
    return false;
}

private int cubeNum(int i, int j) {
    int r = i / 3;
    int c = j / 3;
    return r * 3 + c;
}

N 皇后

51. N-Queens (Hard)

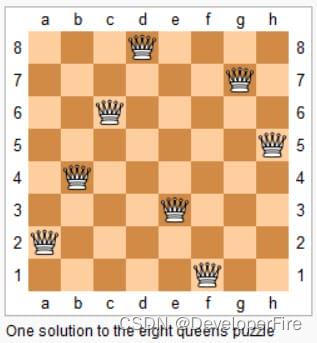
在 n*n 的矩阵中摆放 n 个皇后，并且每个皇后不能在同一行，同一列，同一对角线上，求所有的 n 皇后的解。

一行一行地摆放，在确定一行中的那个皇后应该摆在哪一列时，需要用三个标记数组来确定某一列是否合法，这三个标记数组分别为: 列标记数组、45 度对角线标记数组和 135 度对角线标记数组。

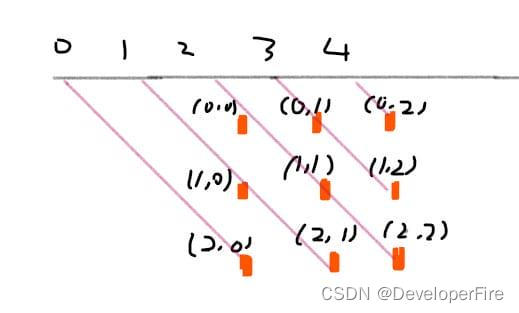
45 度对角线标记数组的维度为 2 * n - 1，通过下图可以明确 (r, c) 的位置所在的数组下标为 r + c。

135 度对角线标记数组的维度也是 2 * n - 1，(r, c) 的位置所在的数组下标为 n - 1 - (r - c)。

private List<List<String>> solutions;
private char[][] nQueens;
private boolean[] colUsed;
private boolean[] diagonals45Used;
private boolean[] diagonals135Used;
private int n;

public List<List<String>> solveNQueens(int n) {
    solutions = new ArrayList<>();
    nQueens = new char[n][n];
    for (int i = 0; i < n; i++) {
        Arrays.fill(nQueens[i], '.');
    }
    colUsed = new boolean[n];
    diagonals45Used = new boolean[2 * n - 1];
    diagonals135Used = new boolean[2 * n - 1];
    this.n = n;
    backtracking(0);
    return solutions;
}

private void backtracking(int row) {
    if (row == n) {
        List<String> list = new ArrayList<>();
        for (char[] chars : nQueens) {
            list.add(new String(chars));
        }
        solutions.add(list);
        return;
    }

    for (int col = 0; col < n; col++) {
        int diagonals45Idx = row + col;
        int diagonals135Idx = n - 1 - (row - col);
        if (colUsed[col] || diagonals45Used[diagonals45Idx] || diagonals135Used[diagonals135Idx]) {
            continue;
        }
        nQueens[row][col] = 'Q';
        colUsed[col] = diagonals45Used[diagonals45Idx] = diagonals135Used[diagonals135Idx] = true;
        backtracking(row + 1);
        colUsed[col] = diagonals45Used[diagonals45Idx] = diagonals135Used[diagonals135Idx] = false;
        nQueens[row][col] = '.';
    }
}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.coloradmin.cn/o/344576.html

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈，一经查实，立即删除！

相关文章

ChatGPT 类 AI 软件供应链的安全及合规风险

ChatGPT 类 AI 软件供应链的安全及合规风险

AIGC将成为重要的软件供应链近日&#xff0c;OpenAI推出的ChatGPT通过强大的AIGC&#xff08;人工智能生产内容&#xff09;能力让不少人认为AI的颠覆性拐点即将到来&#xff0c;基于AI将带来全新的软件产品体验&#xff0c;而AI也将会成为未来软件供应链中非常重要的一环。在O…
阅读更多...
【项目精选】基于WEB的仓库管理系统的设计与实现（论文+视频+源码）

【项目精选】基于WEB的仓库管理系统的设计与实现（论文+视频+源码）

点击下载源码 仓库物品的管理是与我们的日常生活息息相关的一个重大问题。随着我国经济飞速的发展&#xff0c;改革开放的不断深入&#xff0c;企业要想在激烈的市场竞争中立于不败之地&#xff0c;要想继续的发展与生存&#xff0c;没有现代化的管理方式与方法是万万不行的&am…
阅读更多...
torch.nn.parameter 生成可更新的 tensor （requires_grad = True）

torch.nn.parameter 生成可更新的 tensor （requires_grad = True）

torch.nn.parameter 是 PyTorch 中的一种特殊类型的 tensor&#xff0c;它主要用于存储神经网络中的参数。这些参数可以被自动求导和被优化器自动更新。使用 torch.nn.Parameter 定义的tensor 会被自动添加到模型的参数列表中。 \quadtorch.nn.Parameter 是继承自 torch.Tensor…
阅读更多...
vite+vue3搭建的工程热更新失效问题

vite+vue3搭建的工程热更新失效问题

前段时间开发新的项目&#xff0c;由于没有技术上的限制&#xff0c;所以选择了vitevue3ts来开发新的项目&#xff0c;一开始用vite来开发新项目过程挺顺利&#xff0c;确实比vue2webpack的项目高效些&#xff08;为什么选择vite&#xff09;,但是过了一段时间后&#xff0c;不…
阅读更多...
android组件化

android组件化

学习流程&#xff1a;1.开源最佳实践&#xff1a;Android平台页面路由框架ARouter-阿里云开发者社区 (aliyun.com)2.中文ARouter使用API&#xff1a;https://github.com/alibaba/ARouter/blob/master/README_CN.md3.看当前文档后面的代码4.这是通俗易懂的文章&#xff1a;https…
阅读更多...
使用 Nodejs、Express、Postgres、Docker 在 JavaScript 中构建 CRUD Rest API

使用 Nodejs、Express、Postgres、Docker 在 JavaScript 中构建 CRUD Rest API

让我们在 JavaScript 中创建一个 CRUD rest API&#xff0c;使用&#xff1a;节点.js表达续集Postgres码头工人码头工人组成介绍这是我们将要创建的应用程序架构的架构&#xff1a;我们将为基本的 CRUD 操作创建 5 个端点&#xff1a;创造阅读全部读一个更新删除我们将使用以下…
阅读更多...
【H.264】码流解析 annexb vs avcc

【H.264】码流解析 annexb vs avcc

H264码流解析及NALUAVCC和ANNEXB 前者是FLV容器、mp4 常用的。后者 是实时传输使用，所以是TS 一类的标准。VLC显示AVC1就是AVCC AVCC格式 也叫AVC1格式，MPEG-4格式，字节对齐，因此也叫Byte-Stream Format。用于mp4/flv/mkv, VideoToolbox。 – Annex-B格式 也叫MPEG-2 trans…
阅读更多...
微信公众号扫码授权登录思路

微信公众号扫码授权登录思路

引言 上学期研究了一下微信登录相关内容&#xff0c;也写了两三篇笔记&#xff0c;但是最后实际登录流程没有写&#xff0c;主要因为感觉功能完成有所欠缺&#xff0c;一直也没有好的思路&#xff1b;这两天我又看了看官方文档&#xff0c;重新构思了一下微信公众号登录相关的…
阅读更多...
操作系统综合实验

操作系统综合实验

实验目的 加深对进程概念理解&#xff0c;进一步认识进程并发执行掌握Linux系统的进程创建和终止操作掌握文件系统调用及文件标准子例程的编程方法掌握Linux下终端图形编程方法&#xff0c;能编写基于文本的图形界面掌握proc文件系统的使用 相关知识 Linux C编程中的头文件 …
阅读更多...
知识点整合

知识点整合

⭐面试 自我介绍&#xff08;优势岗位匹配度&#xff09; 为什么来我们公司&#xff08;对公司的了解&#xff09; 讲讲做的项目&#xff08;为什么这么做&#xff0c;思路和贡献&#xff09; 跨部门涉案的业务流程 我们跨部门涉案业务主要是本系统、配合物流系统和罚没系…
阅读更多...
二战字节跳动成功上岸，准备了小半年，拿27k也算不上很高吧~

二战字节跳动成功上岸，准备了小半年，拿27k也算不上很高吧~

先说下我基本情况&#xff0c;本科不是计算机专业&#xff0c;现在是学通信&#xff0c;然后做图像处理&#xff0c;可能面试官看我不是科班出身没有问太多计算机相关的问题&#xff0c;因为第一次找工作&#xff0c;字节的游戏专场又是最早开始的&#xff0c;就投递了&#xf…
阅读更多...
SpringMVC传值

SpringMVC传值

实现步骤 先看后台代码如何获取前端传过来的数据&#xff0c;直接在方法的参数列表中添加RequestParam(xxx)&#xff0c;再在后面加上参数列表即可 不过这样的方式会出现一个问题&#xff1a;当前端页面没有提交相应的数据过来的时候&#xff0c;后台会出现异常&#xff0c;所…
阅读更多...
Elasticsearch7.8.0版本进阶——数据读流程

Elasticsearch7.8.0版本进阶——数据读流程

目录一、数据读流程概述二、数据读流程步骤2.1、数据读流程图2.2、数据读流程步骤&#xff08;从主分片或者副本分片检索文档的步骤顺序&#xff09;2.3、数据读流程注意事项一、数据读流程概述 从主分片或者从其它任意副本分片检索文档。 二、数据读流程步骤 2.1、数据读流…
阅读更多...
5_机试_递归和分治

5_机试_递归和分治

一、递归 本章介绍程序设计中的另一个非常重要的思想一递归策略。递归是指函数直接或间接调用自身的一种方法&#xff0c;它通常可把一个复杂的大型问题层层转化为与原问题相似但规模较小的问题来求解。递归策略只需少量的程序就可描述解题过程所需的多次重复计算&#xff0c;…
阅读更多...
谈谈Java多线程离不开的AQS

谈谈Java多线程离不开的AQS

如果你想深入研究Java并发的话&#xff0c;那么AQS一定是绕不开的一块知识点&#xff0c;Java并发包很多的同步工具类底层都是基于AQS来实现的&#xff0c;比如我们工作中经常用的Lock工具ReentrantLock、栅栏CountDownLatch、信号量Semaphore等&#xff0c;而且关于AQS的知识点…
阅读更多...
DDR4 信号说明

DDR4 信号说明

SDRAM Differential Clock :Differential clocks signal pairs , pair perrank . The crossing of the positive edgeand the negative edge of theircomplement are used to sample thecommand and control signals on theSDRAMSDRAM差分时钟&#xff1a;差分时钟信号对&#…
阅读更多...
MagicThoughts｜让ChatGPT变得更智能的Finetuned数据集

MagicThoughts｜让ChatGPT变得更智能的Finetuned数据集

近两个月&#xff0c;ChatGPT无疑都是AI领域最炙手可热的话题。而它的成功&#xff0c;也引发了行业内外对于对话式AI、LLM模型商业化应用可能性的思考。诚然&#xff0c;尽管就目前来看ChatGPT对大部分问答都能基本做到“对答如流”。但是&#xff0c;ChatGPT本质上依旧是预训…
阅读更多...
Flutter Modul集成到IOS项目

Flutter Modul集成到IOS项目

Flutter Modul集成到IOS项目中1. 创建一个Flutter Modul2.在既有应用中集成Flutter Modul2.1 Flutter的构建模式选择2.1.1 debug模式2.1.2 Release模式2.1.3 Podfile 模式2.2 Cocoapods管理依赖库集成方式2.3 直接在Xcode中集成framework2.4 Local Network Privacy Permissions…
阅读更多...
采用 spring 配置文件管理Bean

采用 spring 配置文件管理Bean

文章目录采用 spring 配置文件管理Bean一、安装配置Maven二、Spring 框架1、Spring 官网三、Spring 容器演示-采用Spring配置文件管理Bean1、创建Manev项目2、添加Spring依赖3、创建杀龙骑士类4、创建勇敢骑士类5、采用传统方式让勇敢骑士完成杀龙任务6、采用Spring 容器让勇敢…
阅读更多...
创建Ubuntu虚拟机与Windows共享的文件夹

创建Ubuntu虚拟机与Windows共享的文件夹

目录 1、Windows创建一个共享文件夹 2、在虚拟机的设置中选择Windows下的共享文件夹 3、在Ubuntu中查看共享文件夹 1、Windows创建一个共享文件夹 该共享文件夹可以被Windows和Ubuntu访问&#xff0c;需要注意的是&#xff0c;Ubuntu在共享目录下的一些操作会受到限制&…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023