参考视频:MIT微积分
如何得到的自然对数 l n ln ln
-
首先我们知道以 e e e 为底的指数函数 e x e^x ex
-
其次,我们引入反函数(逆函数)的概念 f − 1 ( y ) f^{-1}(y) f−1(y)
对于任意的 x x x 如果 f ( x ) = y f(x)=y f(x)=y 那么 x = f − 1 ( x ) x=f^{-1}(x) x=f−1(x)
-
举个例子来说:
y = a x + b → f ( x ) = y = a x + b y=ax+b \rightarrow f(x)=y=ax+b y=ax+b→f(x)=y=ax+b
x = y − b a = f − 1 ( y ) x=\frac{y-b}{a}=f^{-1}(y) x=ay−b=f−1(y)
- 因此我们定义 y = e x y=e^x y=ex 的反函数是 f − 1 ( y ) = l n y f^{-1}(y)=lny f−1(y)=lny 即 l n ( e x ) = x ln(e^x)=x ln(ex)=x
l n ln ln 函数的导数
- 因为我们现在知道 l n ln ln 是 e x e^x ex 的反函数,我们尝试对他进行求导
- 首先
l
n
(
e
x
)
=
x
ln(e^x)=x
ln(ex)=x 两边同时求导:左边根据链式法则
d ( l n y ) d y ⋅ d y d x = 1 \frac{d(lny)}{dy}\cdot \frac{dy}{dx}=1 dyd(lny)⋅dxdy=1
d ( l n y ) d y ⋅ d e x d x = 1 \frac{d(lny)}{dy}\cdot \frac{de^x}{dx}=1 dyd(lny)⋅dxdex=1
d ( l n y ) d y ⋅ e x = 1 \frac{d(lny)}{dy}\cdot e^x=1 dyd(lny)⋅ex=1
d ( l n y ) d y ⋅ = 1 e x \frac{d(lny)}{dy}\cdot =\frac{1}{e^x} dyd(lny)⋅=ex1 - 关键来了,因为现在我们的反函数的主题是
y
y
y,也就是
f
−
1
(
y
)
f^{-1}(y)
f−1(y) 我们关心的是
y
y
y,所以在上述式子中需要把
e
x
e^x
ex 替换成
y
y
y,所以可以得到:
d ( l n y ) d y ⋅ = 1 y \frac{d(lny)}{dy}\cdot =\frac{1}{y} dyd(lny)⋅=y1
a r c s i n arcsin arcsin 的导数
-
我们知道 a r c s i n arcsin arcsin 是 s i n sin sin 的反函数,即 y = s i n ( x ) , s i n − 1 ( y ) = x y=sin(x), sin^{-1}(y)=x y=sin(x),sin−1(y)=x
-
为了方便观察,我们暂且把这个 s i n sin sin 的反函数写成 A ( y ) A(y) A(y),所以 A ( y ) = A ( s i n ( x ) ) = x A(y)=A(sin(x))=x A(y)=A(sin(x))=x
-
两边同时求导:(左边还是根据链式法则)
d A ( y ) d y ⋅ d y d x = 1 \frac{dA(y)}{dy}\cdot \frac{dy}{dx}=1 dydA(y)⋅dxdy=1
d A ( y ) d y ⋅ d s i n ( x ) d x = 1 \frac{dA(y)}{dy}\cdot \frac{dsin(x)}{dx}=1 dydA(y)⋅dxdsin(x)=1
d A ( y ) d y ⋅ c o s ( x ) = 1 \frac{dA(y)}{dy}\cdot cos(x)=1 dydA(y)⋅cos(x)=1 -
这时候要把 c o s ( x ) cos(x) cos(x) 化成使用 y y y 表示的形式
-
又因为 y = s i n ( x ) y=sin(x) y=sin(x) 我们知道 s i n 2 ( x ) + c o s 2 ( x ) = 1 sin^2(x)+cos^2(x)=1 sin2(x)+cos2(x)=1 所以 c o s ( x ) = 1 − s i n 2 ( x ) cos(x)=\sqrt{1-sin^2(x)} cos(x)=1−sin2(x)
-
所以:
d A ( y ) d y = 1 1 − s i n 2 ( x ) = 1 1 − y 2 \frac{dA(y)}{dy}=\frac{1}{\sqrt{1-sin^2(x)}}=\frac{1}{1-y^2} dydA(y)=1−sin2(x)1=1−y21 -
也就是 s i n − 1 ( y ) sin^{-1}(y) sin−1(y) 的导数是 1 1 − y 2 \frac{1}{\sqrt{1-y^2}} 1−y21
arccos 的导数
-
与上面的步骤完全一样 a r c c o s arccos arccos 是 c o s cos cos 的反函数,即 y = c o s ( x ) , c o s − 1 ( y ) = x y=cos(x), cos^{-1}(y)=x y=cos(x),cos−1(y)=x
-
我们暂且把这个 c o s cos cos 的反函数写成 A ( y ) A(y) A(y),所以 A ( y ) = A ( c o s ( x ) ) = x A(y)=A(cos(x))=x A(y)=A(cos(x))=x
-
两边同时求导:(左边还是根据链式法则)
d A ( y ) d y ⋅ d y d x = 1 \frac{dA(y)}{dy}\cdot \frac{dy}{dx}=1 dydA(y)⋅dxdy=1
d A ( y ) d y ⋅ d c o s ( x ) d x = 1 \frac{dA(y)}{dy}\cdot \frac{dcos(x)}{dx}=1 dydA(y)⋅dxdcos(x)=1
d A ( y ) d y ⋅ − s i n ( x ) = 1 \frac{dA(y)}{dy}\cdot -sin(x)=1 dydA(y)⋅−sin(x)=1 -
s i n 2 ( x ) + c o s 2 ( x ) = 1 sin^2(x)+cos^2(x)=1 sin2(x)+cos2(x)=1 所以 − s i n ( x ) = − 1 − c o s 2 ( x ) -sin(x)=-\sqrt{1-cos^2(x)} −sin(x)=−1−cos2(x)
-
所以:
d A ( y ) d y = − 1 1 − c o s 2 ( x ) = − 1 1 − y 2 \frac{dA(y)}{dy}=\frac{-1}{\sqrt{1-cos^2(x)}}=\frac{-1}{\sqrt{1-y^2}} dydA(y)=1−cos2(x)−1=1−y2−1 -
因此 c o s − 1 ( y ) cos^{-1}(y) cos−1(y) 的导数为: − 1 1 − y 2 \frac{-1}{\sqrt{1-y^2}} 1−y2−1
有趣的现象
- 有没有发现 ( a r c s i n ( y ) ) ′ = 1 1 − y 2 (arcsin(y))^{'}=\frac{1}{\sqrt{1-y^2}} (arcsin(y))′=1−y21, ( a r c c o s ( y ) ) ′ = − 1 1 − y 2 (arccos(y))^{'}=\frac{-1}{\sqrt{1-y^2}} (arccos(y))′=1−y2−1 这两个加起来结果是 0 0 0
( a r c c o s ( y ) ) ′ + ( a r c s i n ( y ) ) ′ = 0 (arccos(y))^{'}+(arcsin(y))^{'}=0 (arccos(y))′+(arcsin(y))′=0
- 也就是
( a r c c o s ( y ) + a r c s i n ( y ) ) ′ = 0 (arccos(y)+arcsin(y))^{'}=0 (arccos(y)+arcsin(y))′=0 - 所以肯定 a r c c o s ( y ) + a r c s i n ( y ) ) = C arccos(y)+arcsin(y))=C arccos(y)+arcsin(y))=C 其中 C C C 是常数
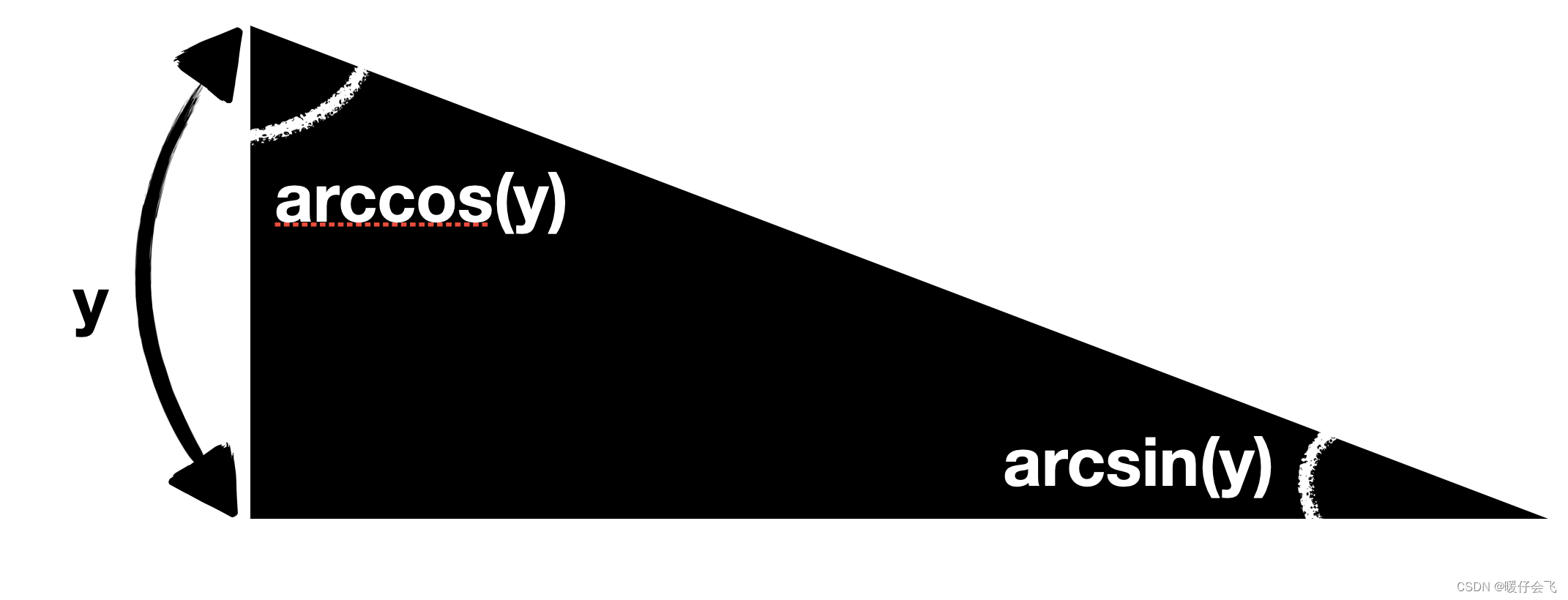
- 事实上,如上图所示, a r c s i n arcsin arcsin 和 a r c c o s arccos arccos 对应的两个角之和为 π 2 \frac{\pi}{2} 2π 确实为常数!



![[附源码]计算机毕业设计JAVA基于协同过滤算法的网上招聘系统](https://img-blog.csdnimg.cn/6bda8b0bba514b84b53b5e5a2a627a34.png)