目录
详细布置:
1. 93. 复原 IP 地址
2. 78. 子集
3. 90. 子集 II
详细布置:
1. 93. 复原 IP 地址
有效 IP 地址 正好由四个整数(每个整数位于
0到255之间组成,且不能含有前导0),整数之间用'.'分隔。
- 例如:
"0.1.2.201"和"192.168.1.1"是 有效 IP 地址,但是"0.011.255.245"、"192.168.1.312"和"192.168@1.1"是 无效 IP 地址。给定一个只包含数字的字符串
s,用以表示一个 IP 地址,返回所有可能的有效 IP 地址,这些地址可以通过在s中插入'.'来形成。你 不能 重新排序或删除s中的任何数字。你可以按 任何 顺序返回答案。
有点类似LC 131,这个切割问题就可以使用回溯搜索法把所有可能性搜出来
class Solution:
def restoreIpAddresses(self, s: str) -> List[str]:
result = []
self.backtracking(s, 0, 0, "", result)
return result
def backtracking(self, s, start_index, point_num, current, result):
if point_num == 3: # 逗点数量为3时,分隔结束
if self.is_valid(s, start_index, len(s) - 1): # 判断第四段子字符串是否合法
current += s[start_index:] # 添加最后一段子字符串
result.append(current)
return
for i in range(start_index, len(s)):
if self.is_valid(s, start_index, i): # 判断 [start_index, i] 这个区间的子串是否合法
sub = s[start_index:i + 1]
self.backtracking(s, i + 1, point_num + 1, current + sub + '.', result)
else:
break
def is_valid(self, s, start, end):
if start > end:
return False
if s[start] == '0' and start != end: # 0开头的数字不合法
return False
num = 0
for i in range(start, end + 1):
if not s[i].isdigit(): # 遇到非数字字符不合法
return False
num = num * 10 + int(s[i])
if num > 255: # 如果大于255了不合法
return False
return True2. 78. 子集
给你一个整数数组
nums,数组中的元素 互不相同 。返回该数组所有可能的子集(幂集)。解集 不能 包含重复的子集。你可以按 任意顺序 返回解集。
建议:子集问题,就是收集树形结构中,每一个节点的结果。 整体代码其实和 回溯模板都是差不多的。
如果把 子集问题、组合问题、分割问题都抽象为一棵树的话,那么组合问题和分割问题都是收集树的叶子节点,而子集问题是找树的所有节点!
其实子集也是一种组合问题,因为它的集合是无序的,子集{1,2} 和 子集{2,1}是一样的。
那么既然是无序,取过的元素不会重复取,写回溯算法的时候,for就要从startIndex开始,而不是从0开始!
class Solution:
def subsets(self, nums):
result = []
path = []
self.backtracking(nums, 0, path, result)
return result
def backtracking(self, nums, startIndex, path, result):
result.append(path[:]) # 收集子集,要放在终止添加的上面,否则会漏掉自己
# if startIndex >= len(nums): # 终止条件可以不加
# return
for i in range(startIndex, len(nums)):
path.append(nums[i])
self.backtracking(nums, i + 1, path, result)
path.pop()3. 90. 子集 II
给你一个整数数组
nums,其中可能包含重复元素,请你返回该数组所有可能的子集(幂集)。解集 不能 包含重复的子集。返回的解集中,子集可以按 任意顺序 排列。
建议:大家之前做了 40.组合总和II 和 78.子集 ,本题就是这两道题目的结合,建议自己独立做一做,本题涉及的知识,之前都讲过,没有新内容。
这道题和上一题区别就是集合里有重复元素了,而且求取的子集要去重。
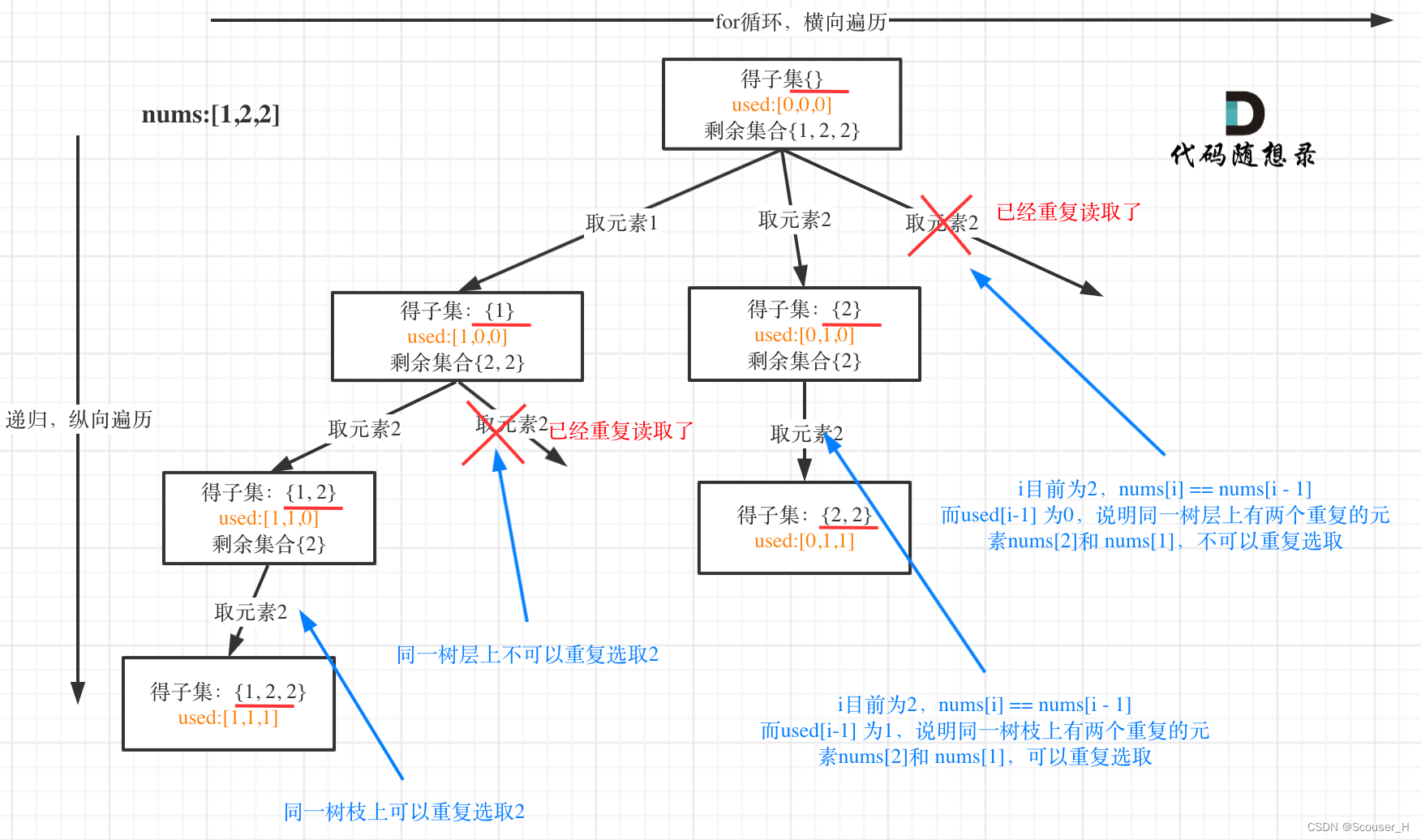
从图中可以看出,同一树层上重复取2 就要过滤掉,同一树枝上就可以重复取2,因为同一树枝上元素的集合才是唯一子集!
class Solution:
def subsetsWithDup(self, nums):
result = []
path = []
used = [False] * len(nums)
nums.sort() # 去重需要排序
self.backtracking(nums, 0, used, path, result)
return result
def backtracking(self, nums, startIndex, used, path, result):
result.append(path[:]) # 收集子集
for i in range(startIndex, len(nums)):
# used[i - 1] == True,说明同一树枝 nums[i - 1] 使用过
# used[i - 1] == False,说明同一树层 nums[i - 1] 使用过
# 而我们要对同一树层使用过的元素进行跳过
if i > 0 and nums[i] == nums[i - 1] and not used[i - 1]:
continue
path.append(nums[i])
used[i] = True
self.backtracking(nums, i + 1, used, path, result)
used[i] = False
path.pop()