三维变换矩阵实战——三维点云的旋转、缩放、镜像、错切、平移、正交投影

一、旋转矩阵（右手坐标系）

绕x轴旋转

旋转矩阵：右边矩阵是点云的原始坐标，左边的是旋转矩阵

$\begin{bmatrix} 1&0 &0 \\ 0&cos\beta &-sin\beta \\ 0&sin\beta & cos\beta \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}$

可视化：绕x轴旋转90度

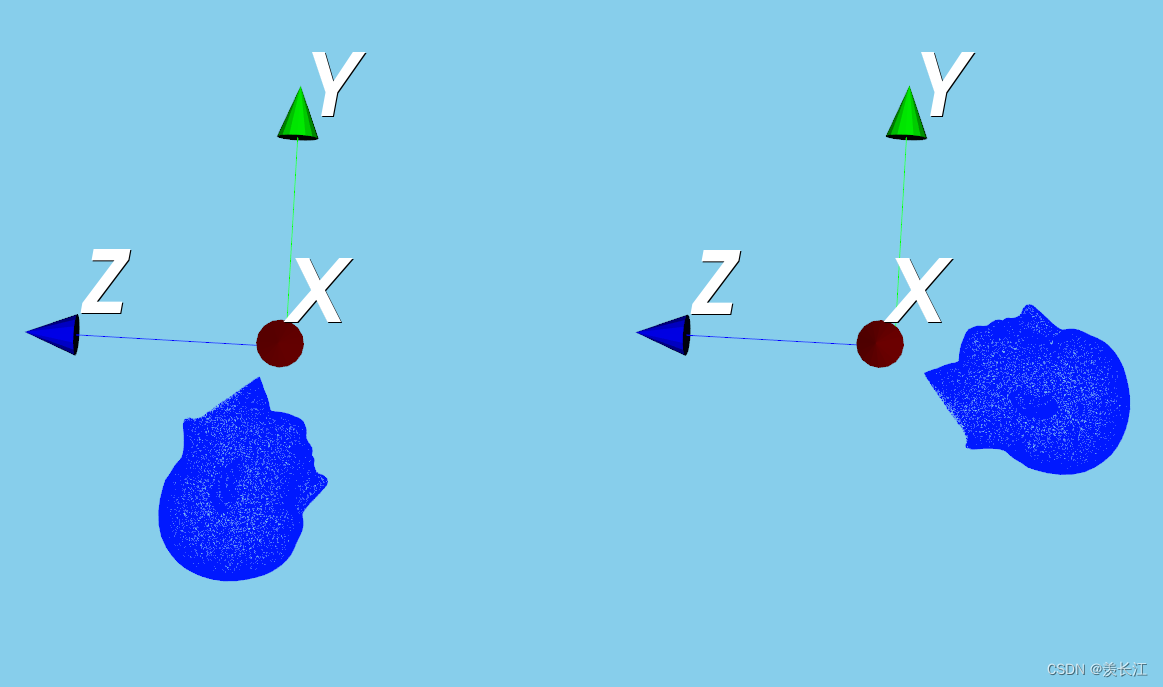
代码：

import vtk
import numpy as np
import math

def pointPolydataCreate(pointCloud):
    points = vtk.vtkPoints()
    cells = vtk.vtkCellArray()
    i = 0
    for point in pointCloud:
        points.InsertPoint(i, point[0], point[1], point[2])
        cells.InsertNextCell(1)
        cells.InsertCellPoint(i)
        i += 1
    PolyData = vtk.vtkPolyData()
    PolyData.SetPoints(points)
    PolyData.SetVerts(cells)

    mapper = vtk.vtkPolyDataMapper()
    mapper.SetInputData(PolyData)

    actor = vtk.vtkActor()
    actor.SetMapper(mapper)
    actor.GetProperty().SetColor(0.0, 0.1, 1.0)

    return actor

def visiualize(pointCloud, pointCloud2):
    colors = vtk.vtkNamedColors()
    actor1 = pointPolydataCreate(pointCloud)
    actor2 = pointPolydataCreate(pointCloud2)
    Axes = vtk.vtkAxesActor()

    # 可视化
    renderer1 = vtk.vtkRenderer()
    renderer1.SetViewport(0.0, 0.0, 0.5, 1)
    renderer1.AddActor(actor1)
    renderer1.AddActor(Axes)
    renderer1.SetBackground(colors.GetColor3d('skyblue'))

    renderer2 = vtk.vtkRenderer()
    renderer2.SetViewport(0.5, 0.0, 1.0, 1)
    renderer2.AddActor(actor2)
    renderer2.AddActor(Axes)
    renderer2.SetBackground(colors.GetColor3d('skyblue'))

    renderWindow = vtk.vtkRenderWindow()
    renderWindow.AddRenderer(renderer1)
    renderWindow.AddRenderer(renderer2)
    renderWindow.SetSize(1040, 880)
    renderWindow.Render()
    renderWindow.SetWindowName('PointCloud')

    renderWindowInteractor = vtk.vtkRenderWindowInteractor()
    renderWindowInteractor.SetRenderWindow(renderWindow)
    renderWindowInteractor.Initialize()
    renderWindowInteractor.Start()


pointCloud = np.loadtxt("C:/Users/A/Desktop/pointCloudData/model.txt") #读取点云数据

angel_x = 90  # 旋转角度
radian = angel_x * np.pi / 180  # 旋转弧度
Rotation_Matrix_1 = [  # 绕x轴三维旋转矩阵 
    [1, 0, 0],
    [0, math.cos(radian), -math.sin(radian)],
    [0, math.sin(radian), math.cos(radian)]]

Rotation_Matrix_1 = np.array(Rotation_Matrix_1)

p = np.dot(Rotation_Matrix_1, pointCloud.T) # 计算
p = p.T
visiualize(pointCloud, p)

绕y轴旋转

旋转矩阵：

$\begin{bmatrix} cos\beta &0 &sin\beta \\ 0&1 &0 \\ -sin\beta &0 &cos\beta \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}$

可视化：绕y轴旋转180度

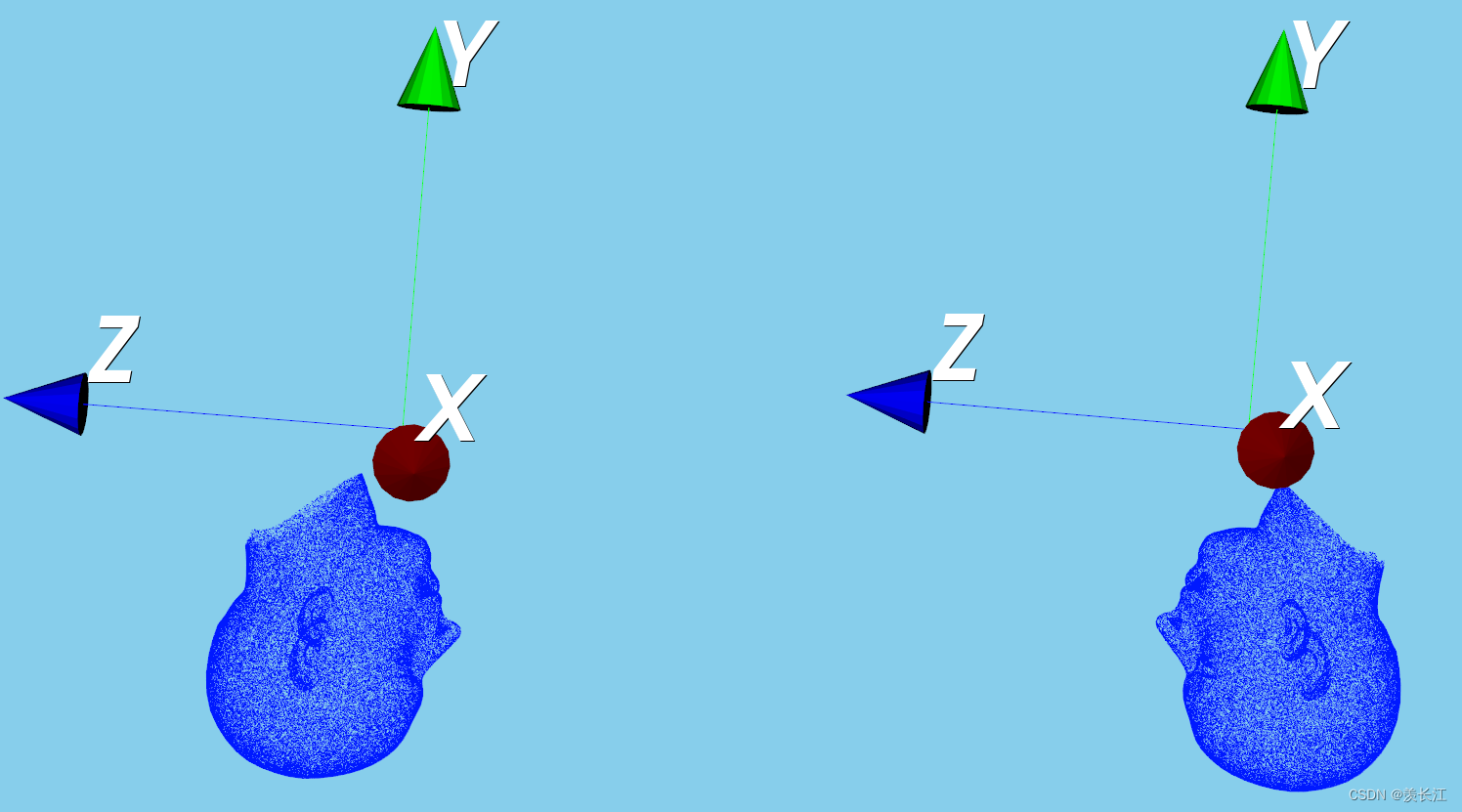
代码：

angel_y = 180  # 旋转角度
radian = angel_y * np.pi / 180  # 旋转弧度
Rotation_Matrix_2 = [  # 绕y轴三维旋转矩阵
    [math.cos(radian), 0, math.sin(radian)],
    [0, 1, 0],
    [-math.sin(radian), 0, math.cos(radian)]]

Rotation_Matrix_1 = np.array(Rotation_Matrix_1)

p = np.dot(Rotation_Matrix_1, pointCloud.T) # 计算
p = p.T
visiualize(pointCloud, p)

绕z轴旋转

旋转矩阵：

$\begin{bmatrix} cos\beta &-sin\beta &0 \\ sin\beta &cos\beta & 0\\ 0&0 & 1 \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}$

可视化：绕z轴旋转90度

代码：

angel_z = 90  # 旋转角度
radian = angel_z * np.pi / 180  # 旋转弧度
Rotation_Matrix_1 = [  # 绕z轴三维旋转矩阵
    [math.cos(radian), -math.sin(radian), 0],
    [math.sin(radian), math.cos(radian), 0],
    [0, 0, 1]]

Rotation_Matrix_1 = np.array(Rotation_Matrix_1)

p = np.dot(Rotation_Matrix_1, pointCloud.T) # 计算
p = p.T
visiualize(pointCloud, p)

线绕z轴旋转，再绕x轴旋转：

旋转矩阵: 线绕哪个轴转，xyz矩阵就和哪和轴的旋转矩阵先计算

$\begin{bmatrix} 1&0 &0 \\ 0&cos\beta &-sin\beta \\ 0&sin\beta & cos\beta \end{bmatrix}$ $\begin{bmatrix} cos\beta &-sin\beta &0 \\ sin\beta &cos\beta & 0\\ 0&0 & 1 \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}$

可视化：先绕z轴旋转90度，再绕x轴旋转90度

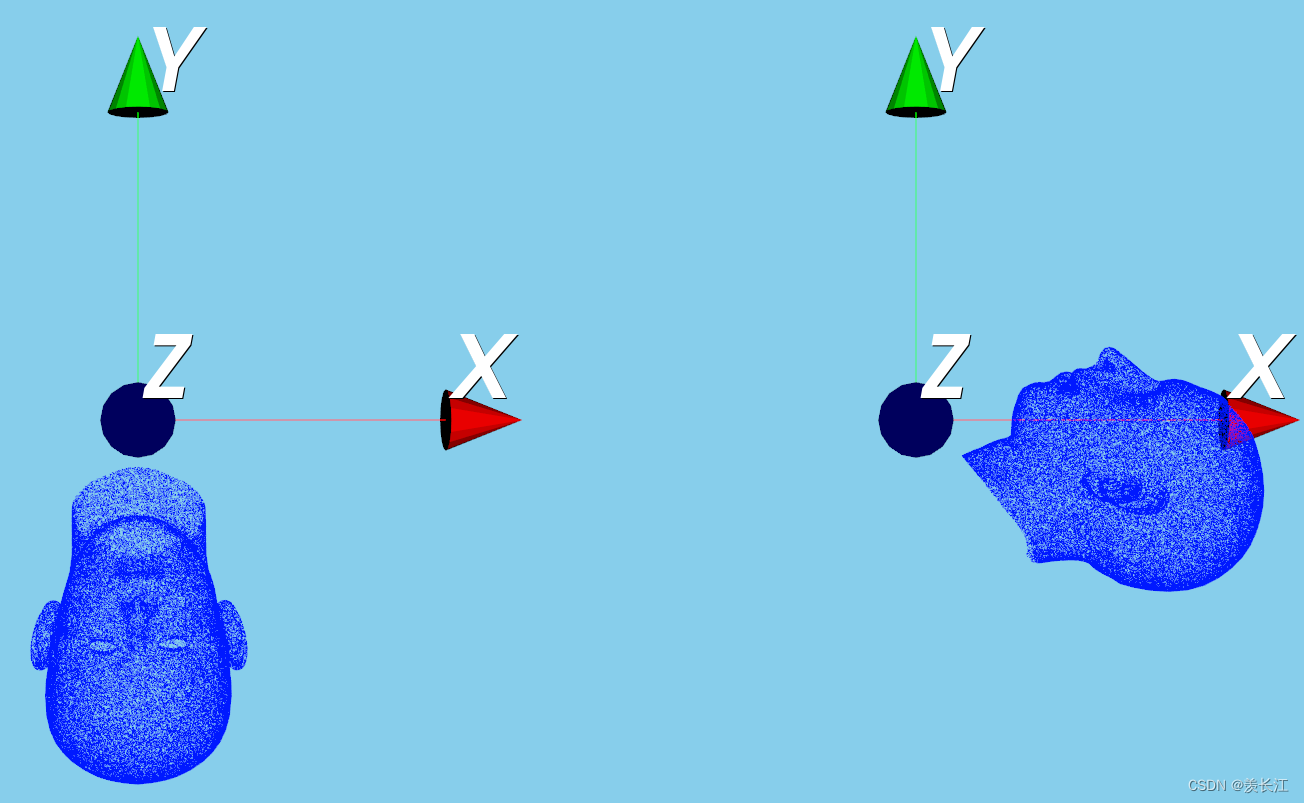
代码：

angel_z = 90  # 旋转角度
radian = angel_z * np.pi / 180  # 旋转弧度
Rotation_Matrix_z = [  # 绕z轴三维旋转矩阵
    [math.cos(radian), -math.sin(radian), 0],
    [math.sin(radian), math.cos(radian), 0],
    [0, 0, 1]]

angel_x = 90  # 旋转角度
radian = angel_x * np.pi / 180  # 旋转弧度
Rotation_Matrix_x = [  # 绕x轴三维旋转矩阵
    [1, 0, 0],
    [0, math.cos(radian), -math.sin(radian)],
    [0, math.sin(radian), math.cos(radian)]]


Rotation_Matrix_z = np.array(Rotation_Matrix_z)
Rotation_Matrix_x = np.array(Rotation_Matrix_x)

p = np.dot(Rotation_Matrix_z, pointCloud.T) # 计算
p = np.dot(Rotation_Matrix_x, p) # 计算
p = p.T
visiualize(pointCloud, p)

二、缩放矩阵

缩放矩阵：

$\begin{bmatrix} k_{x}&0 &0 \\ 0& k_{y} &0 \\ 0&0 &k_{z} \end{bmatrix}$

计算过程：三个k是xyz对应的缩放系数

$\begin{bmatrix} k_{x}&0 &0 \\ 0& k_{y} &0 \\ 0&0 &k_{z} \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}=$ $\begin{bmatrix} k_{x}x\\k_{y} y \\k_{z}z \end{bmatrix}$

x坐标变为原来的1.5倍，y变为0.7倍，z不变

$\begin{bmatrix} 1.5&0 &0 \\ 0& 0.7 &0 \\ 0&0 &1 \end{bmatrix}$ $\begin{bmatrix} x\\ y \\z \end{bmatrix}=$ $\begin{bmatrix} 1.5x\\0.7 y \\1z \end{bmatrix}$

可视化：

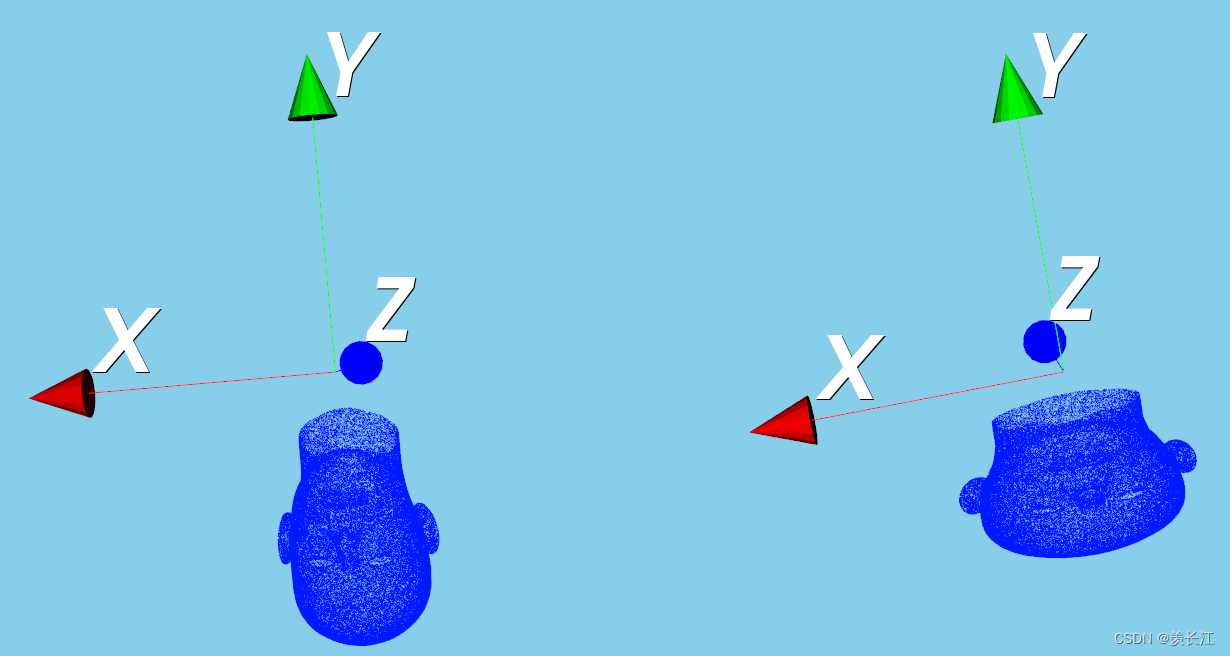
三、镜像矩阵

3D镜像矩阵：

$\begin{bmatrix} 1-2n_x{}^{2} & -2n_x{}n_{y} &-2n_x{}n_{z} \\ -2n_x{}n_{y} &1-2n_y{}^{2} &-2n_y{}n_{z} \\ -2n_x{}n_{z} &-2n_y{}n_{z} & 1-2n_z{}^{2} \end{bmatrix}$

$n=\begin{bmatrix} n_{x} &n_{y} & n_{z} \end{bmatrix}$

向量n是垂直于镜像平面的单位向量

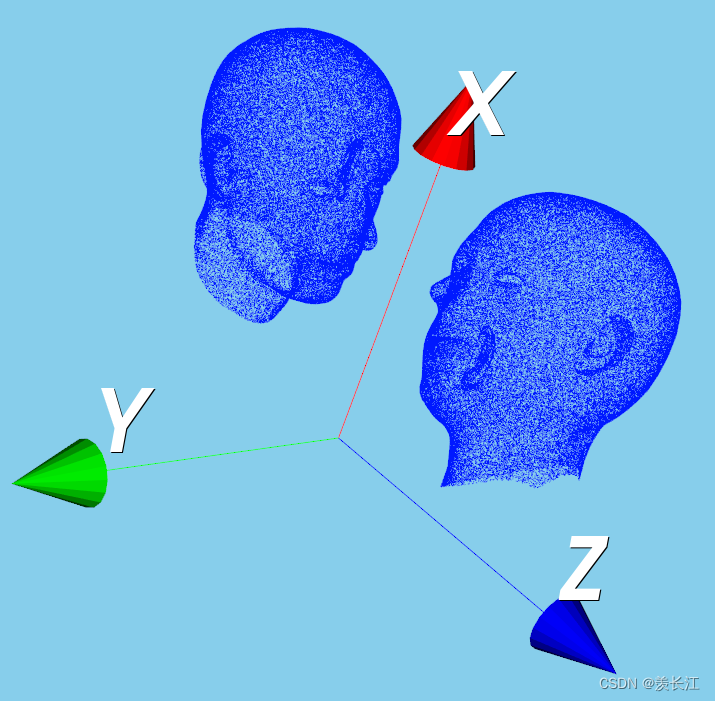
三维点云对xz平面的镜像：

①首先，确定一个垂直于xz平面的单位向量 n=[0, 1, 0]

②将该单位向量带入上述3D镜像矩阵

可视化：

代码：

import vtk
import numpy as np
import math

def pointPolydataCreate(pointCloud):
    points = vtk.vtkPoints()
    cells = vtk.vtkCellArray()
    i = 0
    for point in pointCloud:
        points.InsertPoint(i, point[0], point[1], point[2])
        cells.InsertNextCell(1)
        cells.InsertCellPoint(i)
        i += 1
    PolyData = vtk.vtkPolyData()
    PolyData.SetPoints(points)
    PolyData.SetVerts(cells)

    mapper = vtk.vtkPolyDataMapper()
    mapper.SetInputData(PolyData)

    actor = vtk.vtkActor()
    actor.SetMapper(mapper)
    actor.GetProperty().SetColor(0.0, 0.1, 1.0)

    return actor

def visiualize(pointCloud, pointCloud2):
    colors = vtk.vtkNamedColors()
    actor1 = pointPolydataCreate(pointCloud)
    actor2 = pointPolydataCreate(pointCloud2)
    Axes = vtk.vtkAxesActor()

    # 可视化
    renderer1 = vtk.vtkRenderer()
    renderer1.SetViewport(0.0, 0.0, 0.5, 1)
    renderer1.AddActor(actor1)
    renderer1.AddActor(Axes)
    renderer1.SetBackground(colors.GetColor3d('skyblue'))

    renderer2 = vtk.vtkRenderer()
    renderer2.SetViewport(0.5, 0.0, 1.0, 1)
    renderer2.AddActor(actor1)
    renderer2.AddActor(actor2)
    renderer2.AddActor(Axes)
    renderer2.SetBackground(colors.GetColor3d('skyblue'))

    renderWindow = vtk.vtkRenderWindow()
    renderWindow.AddRenderer(renderer1)
    renderWindow.AddRenderer(renderer2)
    renderWindow.SetSize(1040, 880)
    renderWindow.Render()
    renderWindow.SetWindowName('PointCloud')

    renderWindowInteractor = vtk.vtkRenderWindowInteractor()
    renderWindowInteractor.SetRenderWindow(renderWindow)
    renderWindowInteractor.Initialize()
    renderWindowInteractor.Start()


pointCloud = np.loadtxt("C:/Users/A/Desktop/pointCloudData/model.txt") #读取点云数据

nx = 0
ny = 0
nz = 1
n = [nx, ny, nz] # 垂直xy平面的单位向量
# 镜像矩阵
Mirror_Matrix = [
    [1-2*nx**2, -2*nx*ny, -2*nx*nz],
    [-2*nx*ny, 1-2*ny**2, -2*ny*nz],
    [-2*nx*nz, -2*ny*nz, 1-2*nz**2]]

Mirror_Matrix = np.array(Mirror_Matrix)

p = np.dot(Mirror_Matrix, pointCloud.T)  # 计算
p = p.T
visiualize(pointCloud, p)

四、错切矩阵

沿xy平面错切（z不变）

矩阵计算过程

$H_{xy}(s, t)=\begin{bmatrix} 1 &0 &s \\ 0&1 &t \\ 0&0 &1 \end{bmatrix}$ $\begin{bmatrix} 1 &0 &s \\ 0&1 &t \\ 0&0 &1 \end{bmatrix} \begin{bmatrix} x\\ y \\ z \end{bmatrix}=\begin{bmatrix} x+sz\\y+tz \\ z \end{bmatrix}$

沿xz平面错切（y不变）

矩阵计算过程

$H_{xz}(s, t)=\begin{bmatrix} 1 &0 &s \\ 0&1 &0 \\ 0&t &1 \end{bmatrix}$ $\begin{bmatrix} 1 &0 &s \\ 0&1 &0 \\ 0&t &1 \end{bmatrix} \begin{bmatrix} x\\ y \\ z \end{bmatrix}=\begin{bmatrix} x+sz\\y \\ z+ty \end{bmatrix}$

沿yz平面错切（x不变）

矩阵计算过程

$H_{yz}(s, t)=\begin{bmatrix} 1 &0 &0 \\ s&1 &0 \\ t&0 &1 \end{bmatrix}$ $\begin{bmatrix} 1 &0 &0 \\ s&1 &0 \\ t&0 &1 \end{bmatrix} \begin{bmatrix} x\\ y \\ z \end{bmatrix}=\begin{bmatrix} x\\y+sx \\ z+tx \end{bmatrix}$

可视化：沿yz平面错切

代码：

pointCloud = np.loadtxt("C:/Users/A/Desktop/pointCloudData/model.txt") #读取点云数据

s = 0.3
t = 0.3
# 沿yz平面错切矩阵
Shear_Matrix = [
    [1, 0, 0],
    [s, 1, 0],
    [t, 0, 1]]

Shear_Matrix = np.array(Shear_Matrix)

p = np.dot(Shear_Matrix, pointCloud.T)  # 计算
p = p.T
visiualize(pointCloud, p)

五、正交投影

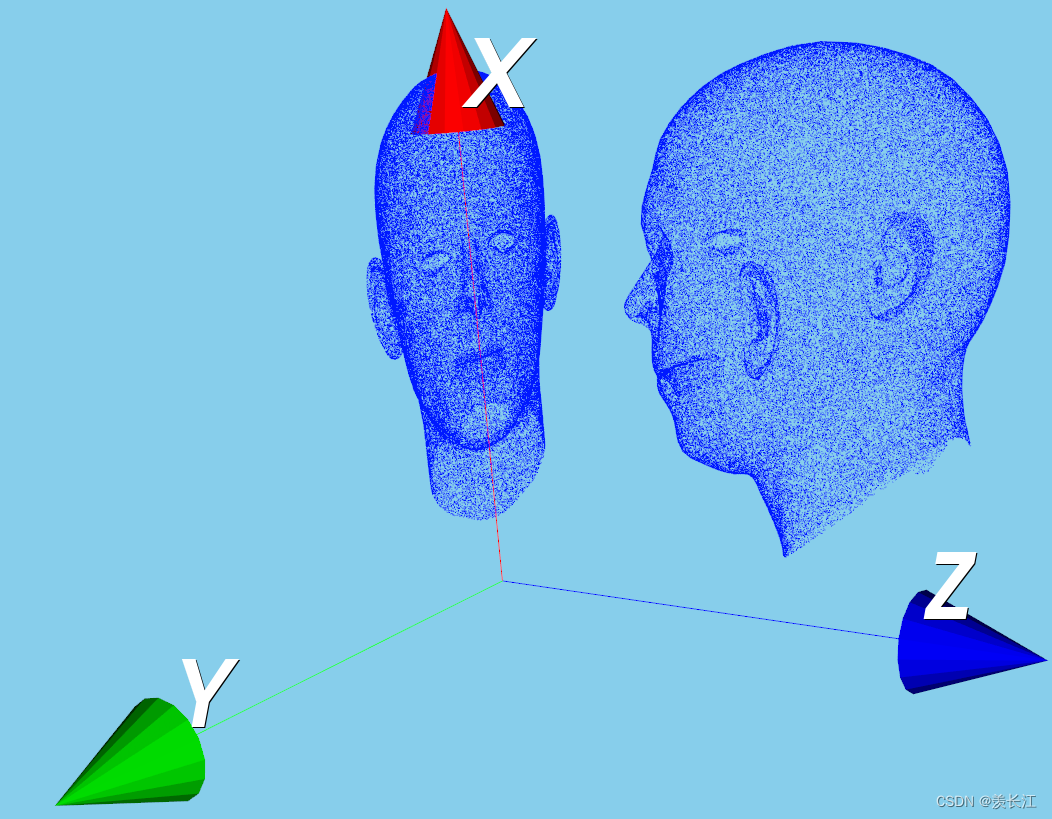
正交投影矩阵（投影到三维空间任意平面）：

$\begin{bmatrix} 1-n_{x}^{2} &-n_{x}n_{y} &-n_{x}n_{z} \\ -n_{x}n_{y}&1-n_{y}^{2} &-n_{y}n_{z} \\ -n_{x}n_{z}&-n_{y}n_{z} & 1-n_{z}^{2} \end{bmatrix}$

$n=\begin{bmatrix} n_{x} &n_{y} & n_{z} \end{bmatrix}$

向量n是垂直于投影平面的单位向量

可视化：点云在xy平面上的正交投影

六、平移矩阵

平移矩阵需要利用齐次矩阵(4*4矩阵)，下面是一个平移矩阵

最右边一列是xyz的位移量

$\begin{bmatrix} 1 &0 &0 & \Delta x \\ 0&1 &0 &\Delta y \\ 0&0 &1 & \Delta z\\ 0&0 & 0 & 1 \end{bmatrix}$

计算过程：

$\begin{bmatrix} 1 &0 &0 & \Delta x \\ 0&1 &0 &\Delta y \\ 0&0 &1 & \Delta z\\ 0&0 & 0 & 1 \end{bmatrix}$ $\begin{bmatrix} x\\y \\z \\ 1 \end{bmatrix}=\begin{bmatrix} x+\Delta x\\ y+\Delta y \\ z+\Delta z \\ 1 \end{bmatrix}$