E. Pokémon Arena:
题目大意:


思路解析:
可以想到的是,可以用最短路来解决这个问题,但是如果简单的建图的话,时间复杂度将会达到 O(n*n*m),我们考虑怎么减少图中边的个数。
我们考虑一个颜色,从这个点能去的点,能不能去这个点可以用一个代价数组判断,然后我们再考虑到达的点,是否之前通过其他颜色到达过,如果没有,我们衍生这个点其他的颜色。点移动时,我们让他移动到在这个颜色上离他最近的点,如果让他混乱移动,可能第一次到达这个点时代价不是最低的情况,那么这样的衍生并不是好的操作。
那么每个点我们最多只需要访问一次,一个点最多有m个颜色,那么最多可能包含 n*m种情况。那么便可以在此基础上利用最短路即可。
代码实现:
import java.io.*;
import java.math.BigInteger;
import java.util.*;
import static java.util.Collections.*;
public class Main {
static long inf = (long) 2e18;
public static void main(String[] args) throws IOException {
int t = f.nextInt();
while (t > 0) {
solve();
t--;
}
w.flush();
w.close();
br.close();
}
public static void solve() {
int n = f.nextInt();
int m = f.nextInt();
int[] c = new int[n+1];
int[][] a = new int[n+1][m+1];
int[][] rk = new int[n+1][m+1];
int[][] dec = new int[n+1][m+1];
long[][] dist = new long[n+1][m+1];
for (int i = 0; i < n + 1; i++) {
Arrays.fill(dist[i], inf);
}
for (int i = 0; i < m + 1; i++) {
dist[1][i] = 0;
}
for (int i = 0; i < n; i++) {
c[i+1] = f.nextInt();
}
for (int i = 0; i < n; i++) {
for (int j = 0; j < m; j++) {
a[i+1][j+1] = f.nextInt();
}
}
for (int i = 1; i <= m; i++) {
ArrayList<int[]> bq = new ArrayList<>();
for (int j = 1; j <= n; j++) {
bq.add(new int[] {a[j][i], j});
}
bq.sort(new Comparator<int[]>() {
@Override
public int compare(int[] o1, int[] o2) {
return o1[0] - o2[0];
}
});
int cnt = 1;
for (int[] cur : bq) {
dec[cnt][i] = cur[1];
rk[cur[1]][i] = cnt++;
}
}
int[] vis = new int[n+1];
vis[1] = 1;
PriorityQueue<long[]> q = new PriorityQueue<>(new Comparator<long[]>() {
@Override
public int compare(long[] o1, long[] o2) {
return Long.compare(o1[0], o2[0]);
}
});
for (int i = 1; i <= m; i++) {
q.add(new long[] {dist[1][i], 1, i});
}
long ans = inf;
while (!q.isEmpty()){
long[] cur = q.poll();
int x = (int) cur[1]; int t = (int) cur[2]; long w = cur[0];
if (w > dist[x][t]) continue;
if (x == n) ans = Math.min(ans, w + c[n]);
if (rk[x][t] < n){
int y = dec[rk[x][t] + 1][t];
if (w < dist[y][t]){
dist[y][t] = w;
q.add(new long[] {dist[y][t], y, t});
}
}
if (rk[x][t] > 1){
int y = dec[rk[x][t] - 1][t];
if (w + a[x][t] - a[y][t]< dist[y][t]){
dist[y][t] = w + a[x][t] - a[y][t];
q.add(new long[] {dist[y][t], y, t});
}
}
if (vis[x] == 0){
vis[x] = 1;
for (int i = 1; i <= m; i++) {
if (w + c[x] < dist[x][i]){
dist[x][i] = w + c[x];
q.add(new long[] {dist[x][i], x, i});
}
}
}
}
w.println(ans);
}
static PrintWriter w = new PrintWriter(new OutputStreamWriter(System.out));
static Input f = new Input(System.in);
static BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
static class Input {
public BufferedReader reader;
public StringTokenizer tokenizer;
public Input(InputStream stream) {
reader = new BufferedReader(new InputStreamReader(stream), 32768);
tokenizer = null;
}
public String next() {
while (tokenizer == null || !tokenizer.hasMoreTokens()) {
try {
tokenizer = new StringTokenizer(reader.readLine());
} catch (IOException e) {
throw new RuntimeException(e);
}
}
return tokenizer.nextToken();
}
public String nextLine() {
String str = null;
try {
str = reader.readLine();
} catch (IOException e) {
// TODO 自动生成的 catch 块
e.printStackTrace();
}
return str;
}
public int nextInt() {
return Integer.parseInt(next());
}
public long nextLong() {
return Long.parseLong(next());
}
public Double nextDouble() {
return Double.parseDouble(next());
}
public BigInteger nextBigInteger() {
return new BigInteger(next());
}
}
}


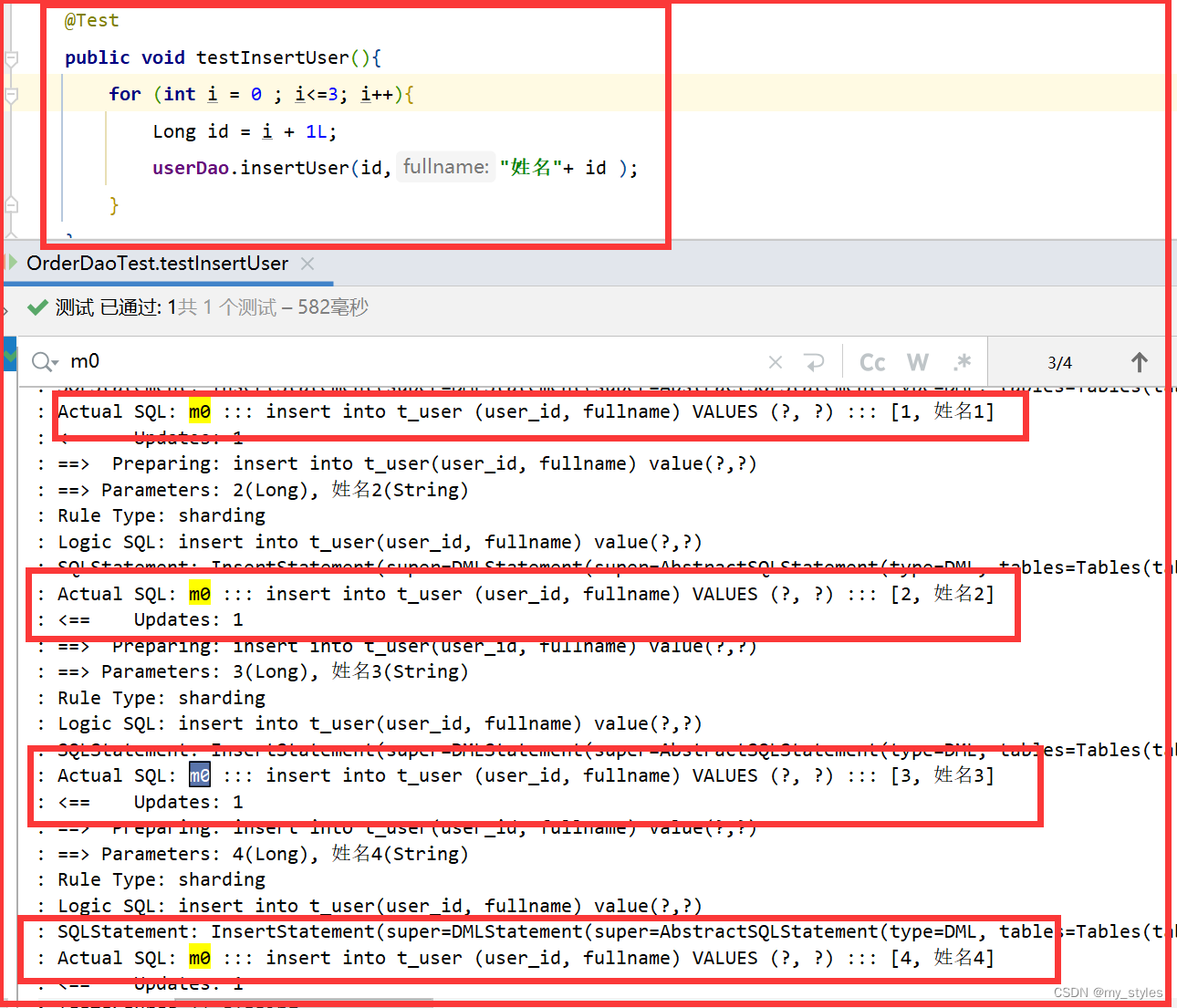





![[调度算法]](https://img-blog.csdnimg.cn/direct/7288f360996243cd802cd55284f9c532.png)