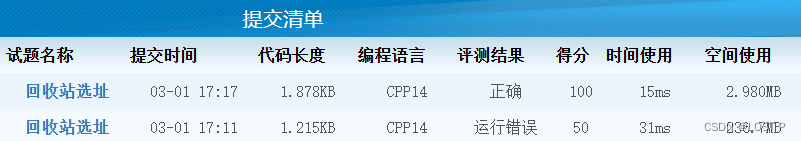
CSP-201912-2-回收站选址
【50分思路-暴力枚举】
#include <iostream>
#include <vector>
#include <algorithm>
using namespace std;
struct trashPoint
{
int x;
int y;
};
vector<trashPoint>trashList;
vector<int>grade(5);
int main() {
int n, max_x = -1, max_y = -1, min_x = 999, min_y = 999;
cin >> n;
for (int i = 0; i < n; i++)
{
trashPoint t;
cin >> t.x >> t.y;
max_x = max(max_x, t.x), max_y = max(max_y, t.y);
min_x = min(min_x, t.x), min_y = min(min_y, t.y);
trashList.push_back(t);
}
int deltaX = max_x - min_x, deltaY = max_y - min_y;
int moveX = min_x, moveY = min_y;
vector<vector<bool>>tashMatrix(deltaX + 1, vector<bool>(deltaY + 1));
for (const auto& it : trashList)
{
tashMatrix[it.x - moveX][it.y - moveY] = 1;
}
for (int i = 1; i < deltaX; i++)
{
for (int j = 1; j < deltaY; j++)
{
int myGrade = 0;
if (tashMatrix[i][j] && tashMatrix[i][j + 1] && tashMatrix[i][j - 1] && tashMatrix[i + 1][j] && tashMatrix[i - 1][j])
{
if (tashMatrix[i - 1][j - 1])myGrade++;
if (tashMatrix[i + 1][j + 1])myGrade++;
if (tashMatrix[i - 1][j + 1])myGrade++;
if (tashMatrix[i + 1][j - 1])myGrade++;
grade[myGrade]++;
}
}
}
for (const auto& it : grade) {
cout << it << endl;
}
return 0;
}
【100分思路】
-
读取数据:初始化一个二维向量
coords存储每个垃圾点的坐标。对于每个垃圾点,代码读取其横纵坐标(x, y)并存储在coords中。 -
计算相邻点数量:对于每个垃圾点,代码计算其直接相邻的垃圾点数量(即上下左右四个方向)。这是通过比较每个点与其他所有点的坐标来完成的,如果两个点在水平或垂直方向上相邻(差一个单位距离),则它们被认为是相邻的。对于每个点,它的相邻垃圾点的数量被记录在
adjacentCount向量中。 -
评分回收站选址:根据题目的要求,一个地点适合建立回收站的条件是它有四个直接相邻的垃圾点。对于每个符合这一条件的垃圾点,代码会进一步检查其四个对角线方向上的垃圾点的数量。对角线上的垃圾点数量将决定该地点的评分,评分范围是 0 到 4。每个可能的评分值都有一个对应的计数,记录在
diagonalCounts向量中。 -
输出结果:最后,代码输出每个评分值的回收站选址数量。对于每个从 0 到 4 的评分值,它打印出相应的计数值,这些值表示评分为该值的合适回收站选址的数量。
完整代码
#include<iostream>
#include<vector>
using namespace std;
int main() {
int n;
cin >> n;
vector<vector<long long>> coords(n, vector<long long>(2)); // 二维向量存储坐标
vector<int> adjacentCount(n, 0); // 创建一个向量存储每个点相邻点的数量
vector<int> diagonalCounts(5, 0); // 创建一个向量存储对角线上点的数量分布
// 读取坐标数据
for (int i = 0; i < n; i++) {
cin >> coords[i][0] >> coords[i][1]; // 读取每个坐标
}
// 计算每个点的相邻点数量
for (int i = 0; i < n; i++) {
for (int j = i + 1; j < n; j++) {
// 检查是否为相邻点(水平或垂直相邻)
if ((coords[i][0] == coords[j][0] && abs(coords[i][1] - coords[j][1]) == 1) ||
(coords[i][1] == coords[j][1] && abs(coords[i][0] - coords[j][0]) == 1)) {
adjacentCount[i]++;
adjacentCount[j]++;
}
}
}
// 统计每个点对角线上的点的数量
for (int i = 0; i < n; i++) {
int diagCount = 0; // 对角线上的点数量
if (adjacentCount[i] == 4) { // 如果一个点有4个相邻点,则检查其对角线上的点
for (int j = 0; j < n; j++) {
// 检查是否为对角线上的点
if (abs(coords[i][0] - coords[j][0]) == 1 && abs(coords[i][1] - coords[j][1]) == 1) {
diagCount++;
}
}
diagonalCounts[diagCount]++; // 根据对角线上的点的数量增加对应的计数
}
}
for (int i = 0; i <= 4; i++) {
cout << diagonalCounts[i] << endl;
}
return 0; // 程序结束
}