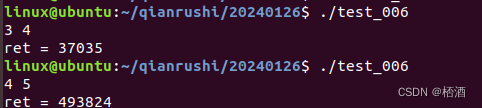
林浩然矩阵江湖历险记

Lin Haoran’s Matrix Adventures

---

在那充满神秘色彩的矩阵世界里，林浩然面对的挑战是驯服一个具有六个个性元素的2×3矩阵——“小三儿”。这个矩阵由两行三列组成，每一个元素都像是棋盘上的一枚棋子，它们紧密排列在一起，形成了一种微妙而复杂的阵势。

In the enigmatic realm of matrices, Lin Haoran faced the challenge of taming a 2×3 matrix with six distinct personality elements—dubbed “Little Three.” This matrix, composed of two rows and three columns, resembled chess pieces tightly arranged, creating a subtle yet intricate formation.

首先，林浩然采用加减法这一基础招式。他将两个大小相同的2×3矩阵进行叠加，就像是玩俄罗斯方块那样精准对接，对应位置上的数字或符号如同武士般一对一地比拼力量，通过逐个相加或相减后，一个新的矩阵就此诞生，其形态各异，展现出不同于原矩阵的独特风貌。

Initially, Lin Haoran employed the basic techniques of addition and subtraction. Like playing Tetris with precision, he overlaid two identically sized 2×3 matrices, where each element, akin to warriors on a chessboard, engaged in one-on-one battles at corresponding positions. Through meticulous addition or subtraction, a new matrix emerged, exhibiting diverse forms and showcasing unique characteristics distinct from the original.

接下来，他对矩阵乘法进行了细致入微的探索。矩阵乘法并非简单的元素对应相乘，而是类似一场精心编排的舞蹈，其中第一个矩阵的每一列（代表伸出的手）必须与第二个矩阵的每一行（代表待握的脚）一一匹配才能产生交互作用。只有在这种情况下，每个位置的新元素才是前一行对应的数与当前列所有数按照一定规则相乘后求和的结果，由此创造出了一个全新的、大小不同的矩阵后代。

Next, he delved into the intricacies of matrix multiplication. It wasn’t a simple element-wise product but rather a choreographed dance. Each column of the first matrix (representing extended hands) had to match with each row of the second matrix (representing awaiting feet) to interact. Only in this scenario did each new element at every position result from the product and sum of the previous row’s corresponding number and all numbers in the current column, creating a completely new, differently sized matrix offspring.

当林浩然施展“转置大法”时，整个矩阵世界的法则发生了戏剧性的变化。原本的小三儿被瞬间改头换面，它的行与列互换位置，从一个2×3的矩阵转变成了一个3×2的矩阵，也就是它的转置矩阵$A^{\text{T}}$。这一变化揭示了矩阵空间变换的奥秘，让人对矩阵的内在结构有了更深的认识。

When Lin Haoran unleashed the “Transpose Grand Technique,” the rules of the entire matrix world underwent a dramatic change. The original Little Three transformed instantly, swapping its rows and columns. It morphed from a 2×3 matrix into a 3×2 matrix, known as its transpose matrix $A^{\text{T}}$. This transformation revealed the mysteries of matrix space alteration, providing a deeper understanding of the matrix’s intrinsic structure.

随着剧情的推进，林浩然运用了矩阵世界中更为深邃的武器：行列式和特征值。对于2×2或更大尺寸的方阵来说，行列式不仅能够判断矩阵是否可逆，而且能反映矩阵对空间扭曲的程度。而特征值则代表着矩阵在特定方向上的缩放因子，通过特征向量可以揭示矩阵变换的核心特性。

As the plot progressed, Lin Haoran wielded more profound weapons in the matrix world: determinants and eigenvalues. For square matrices of size 2×2 or larger, determinants not only determined invertibility but also reflected the extent of the matrix’s spatial distortion. Eigenvalues represented scaling factors in specific directions, unveiling the core characteristics of matrix transformations through eigenvectors.

最后，为了彻底降服小三儿，林浩然使出初等变换这一系列连环招数：

Finally, to completely subdue Little Three, Lin Haoran employed a series of successive techniques, known as elementary transformations:

• 交换大法：他毫不犹豫地调换矩阵中的任意两行（或列），就像两位武林高手在比武场上的位置瞬息万变，从而改变矩阵的整体布局。

• Exchange Grand Technique: Without hesitation, he swiftly swapped any two rows (or columns) in the matrix, akin to the swift changes in position of two martial arts experts in a arena, thereby altering the overall layout of the matrix.

• 倍增术：对某一行（或列）的所有元素乘以同一个非零常数，仿佛为该行赋予了神奇的力量，使得矩阵内部的能量分布发生重大转变。

• Duplication Art: Multiplying all elements of a particular row (or column) by the same non-zero constant endowed that row with a magical power, leading to a significant transformation of energy distribution within the matrix.

• 加减合并功：选取一行元素，然后将其倍数巧妙地添加到另一行中，这种融合之术让矩阵一步步简化，最终化繁为简，形成了阶梯形矩阵，甚至是最简形矩阵，犹如经过艰苦修炼后的武林高手褪去冗余招式，仅保留核心内力。

• Summation and Subtraction Merge Art: Selecting one row’s elements and judiciously adding its multiples to another row, this fusion technique gradually simplified the matrix, ultimately reducing it to row echelon form and even the simplest form, much like a martial artist discarding redundant moves after rigorous training, retaining only the core strength.

通过这一系列操作，林浩然展示了矩阵之间的奇妙等价关系——不论矩阵最初有多么复杂难解，只要灵活应用基本运算和初等变换，就能揭示矩阵背后的深层规律，并将其应用于解决实际的数学问题，如线性方程组的求解等。

Through this series of operations, Lin Haoran demonstrated the fascinating equivalence relationships between matrices. No matter how initially complex or challenging a matrix might be, adept application of basic operations and elementary transformations could reveal the deep-seated patterns behind it. These insights could then be applied to solve practical mathematical problems, such as systems of linear equations.

这就是林浩然在矩阵江湖的历险记，他的每一次出手，都是对数学智慧的生动诠释，每一步探索，都引领着我们一同领略矩阵世界的无尽魅力与深邃内涵。

This is Lin Haoran’s adventure in the matrix world—a vivid interpretation of mathematical wisdom with each move, guiding us to appreciate the endless charm and profound connotations of the matrix realm.